Understanding Exponential Functions and Inverse Functions

This document explains the concepts of exponential functions and inverse functions. It covers topics such as domain, range, and finding the inverse of a function. The document also includes examples and explanations for better understanding.

1.
a)
h(t) = 5t + 7
h(t) is basically the function 5t shifted up by 7 units.It is therefore essentially an
exponential function.Exponential functions accept all real numbers as input, there-
fore domain of h(t) is:
Domain:(−∞, +∞)
Exponentialfunctions decay and tend to zero as t → −∞.Since 7 is added to the
exponential term, it will tend to 7 as t → −∞.Exponential functions tend to ∞ as
they grow.Therefore, range of h(t) is:
Range:(7, +∞)
b)
r(m) = 2 +
√ 3 − m
Square root of negative numbers is undefined, so 3−m cannot become less than zero.
3 − m = 0=⇒ m = 3, for m > 3,r(m) is undefined,so the function can accept
numbers less than 3.
Domain:[3, −∞)
As m → −∞, r(m) tends to +∞.The least value r(m) achieves when the square
root term becomes zero, at which its value is 2.
Range:[2, +∞)
2.
f (x) = 3
2x + 1
a) Inverse
Step 1:y = 3
2x + 1
Step 2:Interchange x and y
x = 3
2y + 1

Step 3:Solve for y
2y + 1 =
3
x =⇒ y = 1
2
3
x − 1
Step 4:y = f−1(x)
f −1(x) = 1
2
3
x − 1
Step 5:Verify:(f −1 o f )(x) = x
(f −1 o f ) =1
2
3
f (x) − 1
= 1
2
3
3
2x+1
− 1
!
= 1
2(2x + 1 − 1) = x =⇒ Verified
Therefore, inverse of f (x) is f−1(x) = 1
2
3
x − 1
b)
f (x) accepts allvalues ofx except the one for which 2x + 1 = 0,because at this
point value of f (x) is undefined.This point is x = −1
2. Therefore,
Domain: −∞, −1
2 ∪ −1
2, +∞
f (x) attains allvalues possible except 0 because the function tends to 0 as x gets
bigger and bigger but never actually reaches it.f (x) = 0 is an horizontal assymptote.
Range:(−∞, 0) ∪ (0, +∞)
c)
For an inverse function, the domain is just the range of f (x) and the range is domain
of f (x).Therefore,
Domain:(−∞, 0) ∪ (0, +∞)
Range: −∞, −1
2 ∪ −1
2, +∞

3.
f (x) = 3x2 + 1
a)
f (1) is the value of f (x) at x = 1.Therefore,
f (1) = 3(1)2 + 1 = 3 + 1 = 4
b)
f (1 + h) = 3(1 + h)2 + 1 = 3(1 + 2h + h2) + 1 = 3h2 + 6h + 4
c)
f (1) − f (1 + h)
h = 4 − (3h2 + 6h + 4)
h
= −3h2 − 6h
h
= −3h − 6
4.
x2 + y2 − 4x + 8y + 16 = 0
We want to reduce this equation into standard form of circle equation:(x − h)2 +
(y − k)2 = a2, where (h, k) is the circle center while a is the radius.

0 = x2 + y2 − 4x + 8y + 16
0 = x2 − 4x + y2 + 8y + 16
[Split 16 as 4+12]
0 = (x2 − 4x + 4) + y2 + 8y + 12
[Add 4 to both sides of the equation]
4 = (x2 − 4x + 4) + (y2 + 8y + 16)
[Terms in brackets are squares]
22 = (x − 2)2 + (y + 4)2
Comparing with standard form,we get:a = 2,h = 2 and k = −4.Therefore,the
circle has a center at (2, −4) and has a radius=2.
5.
a)
10 − 6x ≤ 14 − 4x
[Add 6x to both sides]
10 ≤ 14 + 2x
14 + 2x ≥ 10
[Subtract 14 from both sides]
2x ≥ −4
[Divide throughout by 2]
x ≥ −2
Therefore, solution to the inequality is:
x ∈ [−2, +∞)

b)
x2 − 3x ≥ 10
[Subtract 10 from both sides]
x2 − 3x − 10 ≥ 0
x2 − 5x + 2x + −10 ≥ 0
(x − 5)(x + 2) ≥ 0
This condition (x − 5)(x + 2) ≥ 0 is satisfied when both the terms in the brackets
are positive, or both are negative or either one or both are zero.
i) Both terms positive:Implies x > 5 and x > −2.Therefore for x > 5, this condi-
tion is always true.
ii) Both terms negative:Implies x < 5 and x < −2.Therefore for x < −2,this
condition is always true.
iii) Zero condition:
x = 5 and/or x = −2 satisfies this condition, that is (x−5)(x+2) = 0 iv) −2 < x < 5
:
Between x = −2 and x = 5, the term x + 2 is positive but x − 5 is negative, therefore
this interval fails the inequality.
Solution to the inequality x2 − 3x ≥ 10, therefore, is:
x ∈ (−∞, −2] ∪ [5, +∞)
6.
The sine function:
f(t) = A sin (Bt + C) + D
has, amplitude = A, phase shift =C
D (to the left), and period =2π
B .
The given function is:
f (t) = 5 sin (3t − π) + 7
Comparing with standard form, we get:
a)

Amplitude = A = 5
b)
Period =2π
B = 2π
3
c)
Phase shift =
C
B = −π
3, or π
3 to the right.
d)
f π
3 = 5 sin (3 ·
π
3 − π) + 7 = 5 sin (0) + 7 = 7
7.
a)
13 + 25e−x = 63
[Subtract 13 from both sides]
25e−x = 50
[Divide throughout by 25]
e−x = 2
[Apply log to both sides ]
ln (e−x) = ln (2)
[Property:ln (ea) = a]
−x = ln (2)=⇒ x = − ln (2)
[Property:ln (ab) = b ln (a)]
x = ln (2−1) = ln 1
2
b)

log(x − 7) + log(x − 10) = 1
[Property:log(a) + log(b) = log(ab)]
log((x − 7) · (x − 10)) = 1
[log(10) = 1]
log((x − 7) · (x − 10)) = log(10)
[Taking terms out of logarithms]
(x − 7)(x − 10) = 10
x2 − 17x + 70 = 10
x2 − 17x + 60 = 0
x2 − 12x − 5x + 60 = 0
(x − 12)(x − 5) = 0 =⇒ x = 12 or x = 5
x = 5 is discarded as it creates negative logarithms (undefined).Therefore,the so-
lution to the equation is x = 12.
8.
Cost of production for x number of cars is:
C(x) = x2 − 36x + 10, 400
a)
At minimum Cost of production:
dC
dx = 0
dC
dx = d
dx(x2 − 36x + 10, 400) = 2x − 36
Therefore, for cost to be minimum:
2x − 36 = 0 =⇒ x = 18
18 cars produced daily will minimize cost of production.
b)

The minimum cost is:
C(18) = (18)2 − 36(18) + 10400 = 10076 $
9.
P = (−4, 6) and Q = (2, −2)
a)
Vertical line passing through Q.Vertical line passing through a point (a, b) is given
by the equation x = a,therefore for Q = (2, −2),vertical line passing through it is
x = 2
b)
Equation of line passing through P and Q.
Equation of a line passing through (x1, y1) is:
y − y1 = m(x − x1)
where, m is the slope of the line.
Since both P and Q lie on the line, slope of the line can be calculated as:
m = yP − yQ
xP − xQ
where, xP , yP are co-ordinates of P and similarly for Q.Therefore,
m = 6 − (−2)
−4 − 2 = −4
3
Therefore, equation of line passing through P = (−4, 6) and Q is:
y − 6 = −
4
3(x − (−4))=⇒ y − 6 = −
4
3(x + 4)
In the slope-intercept form, it is given as:
y = −
4
3(x + 4) + 6
c)
Slope of the line 5x − 2y = 4 is obtained by expressing it in standard slope-intercept

for.
5x − 2y = 4 =⇒ 2y = 5x − 4 =⇒ y =
5
2x − 2
Slope, therefore is:m = 5
2.
Line perpendicular to the above line has a slope m0 which satisfies the condition:
m × m0 = −1
Implies, 5
2 m0 = −1 =⇒ m0 = −2
5
Equation of line passing through P = (−4, 6) with slope m0 = −2
5 is given by:
y − 6 = −
2
5(x − (−4)=⇒ y = −2
5(x + 4) + 6
10.
f (x) = ln x & g(x) = 10 − x
a)
(f o g)(x):
(f o g)(x) = ln g(x) = ln (10 − x)
b)
Domain of (f o g)(x) is the set of numbers for which 10 − x is greater than 0, because
the function is not defined for numbers less than or equal to 0.Therefore, 10 − x > 0
or x < 10 is valid input.Domain of (f o g)(x) is:
x ∈ (−∞, 10)
c)
(f o g)(9) = ln (10 − 9) = ln (1) = 0

11.
f (x) = (x + 1)(x − 2)(x − 4)2
a) x- intercept(s):
x- intercept(s) is the point(s) at which the function intersects the x- axis.It is found
by setting y = f (x) = 0, which is the equation of x- axis.
Therefore,
0 = (x + 1)(x − 2)(x − 4)2
This equation is satisfied when:x = −1, x = 2 or x = 4.This can be verified by
substituting for x in the equation.
Eg.
x = 4 =⇒ f(x) = (4 + 1)(4 − 2)(4 − 4)2 = 5 × 2 × 0 = 0
Similarly,other two values can also be verified.Therefore,the x- intercepts are
points −1, 2 and 4, on the x- axis.
b) y- intercept:
y- intercept(s) is the point(s) at which the function intersects the y- axis.It is found
by setting x = 0 in the function, which is the equation of y- axis.
Therefore, substituting x = 0 in f (x), we get:
f (x) = (0 + 1)(0 − 2)(0 − 4)2 = 1 × −2 × 16 = −32
Therefore, the y- intercept is the point −32 on the y- axis.
c) End behaviour:
In order to analyze f (x) let us first express it in the expanded form:
f (x) = (x + 1)(x − 2)(x − 4)2 = (x2 − x − 2)(x2 − 8x + 16) = x4 − 9x3 + 22x2 − 32
f (x) has a leading term with even degree (x4) having a positive coefficient (+1).
As x → ±∞, the highest degree term in the function f (x) will dominate and therefore
f (x) will behave like y = x4.
Since the leading term is an even degree term with positive coefficient:
x → +∞ =⇒ f(x) → +∞
x → −∞ =⇒ f(x) → +∞

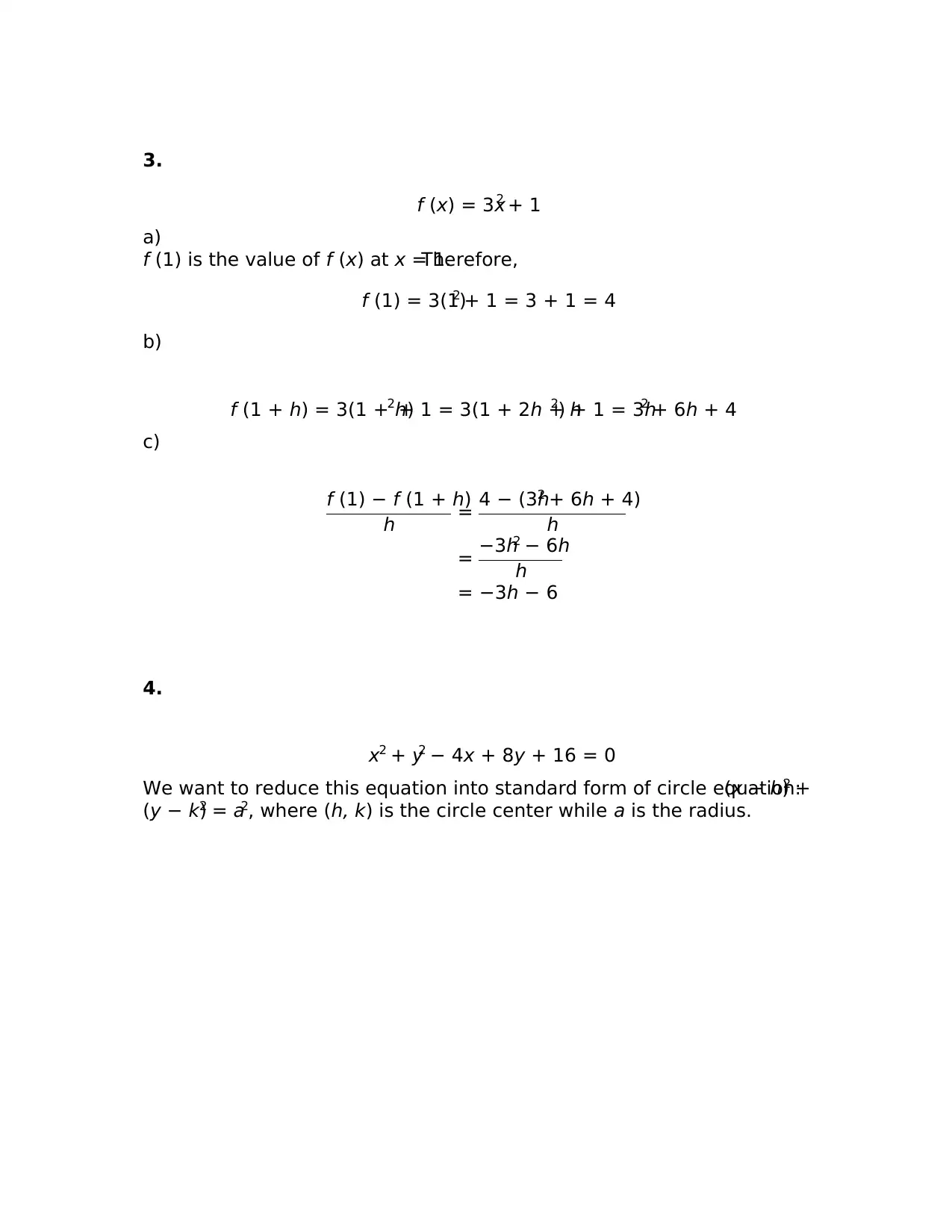
d) Sketch of f (x):
f (x) = (x + 1)(x − 2)(x − 4)2
Sign off (x) is the result ofsign ofthree terms:x + 1,x − 2 and (x − 4)2. For
example, if x < −1, then x − 1 is negative, x − 2 is negative and (x − 4)2 is positive,
therefore, sign of f (x) is (−)(−)(+) which is positive (+).
x f (x) sign
x < −1 (−)(−)(+) +
−1 < x < 2 (+)(−)(+) −
2 < x < 4 (+)(+)(+) +
x > 4 (+)(+)(+) +
Few points to plot on the graph:
x f (x) value
x = 0 (0 + 1)(0 − 2)(0 − 4)2 −32
x = 3 (3 + 1)(3 − 2)(3 − 4)2 4
Using these sample points,the intercept points and information on end behaviour,
f (x) is plotted and shown in figure below:

12.
To prove: 1
sin θ− sin θ = cot θ cos θ
1
sin θ− sin θ =
1 − sin2 θ
sin θ
[Identity: 1 = sin2 θ + cos2 θ]
= sin2 θ + cos2 θ − sin2 θ
sin θ
= cos2 θ
sin θ
= cos θ
sin θcos θ
= cot θ cos θ =⇒ Proved
13.
2 sin t cos t + cos t = 0∀ t ∈ [0, 2π)
0 = 2 sin t cos t + cos t
= cos t(2 sin t + 1)
=⇒ cos t = 0 or 2 sin t + 1 = 0
cos tbecomes 0 at t= 0.5π & 1.5π for t ∈ [0, 2π). The second condition is :
2 sin t + 1 = 0 or sin t = −0.5.This is true for t = 7π/6 & t = 11π/6 fort ∈ [0, 2π).
Therefore solution for the equation is:
t = {0.5π, 1.5π, 7π/6, 11π/6}
for t ∈ [0, 2π)

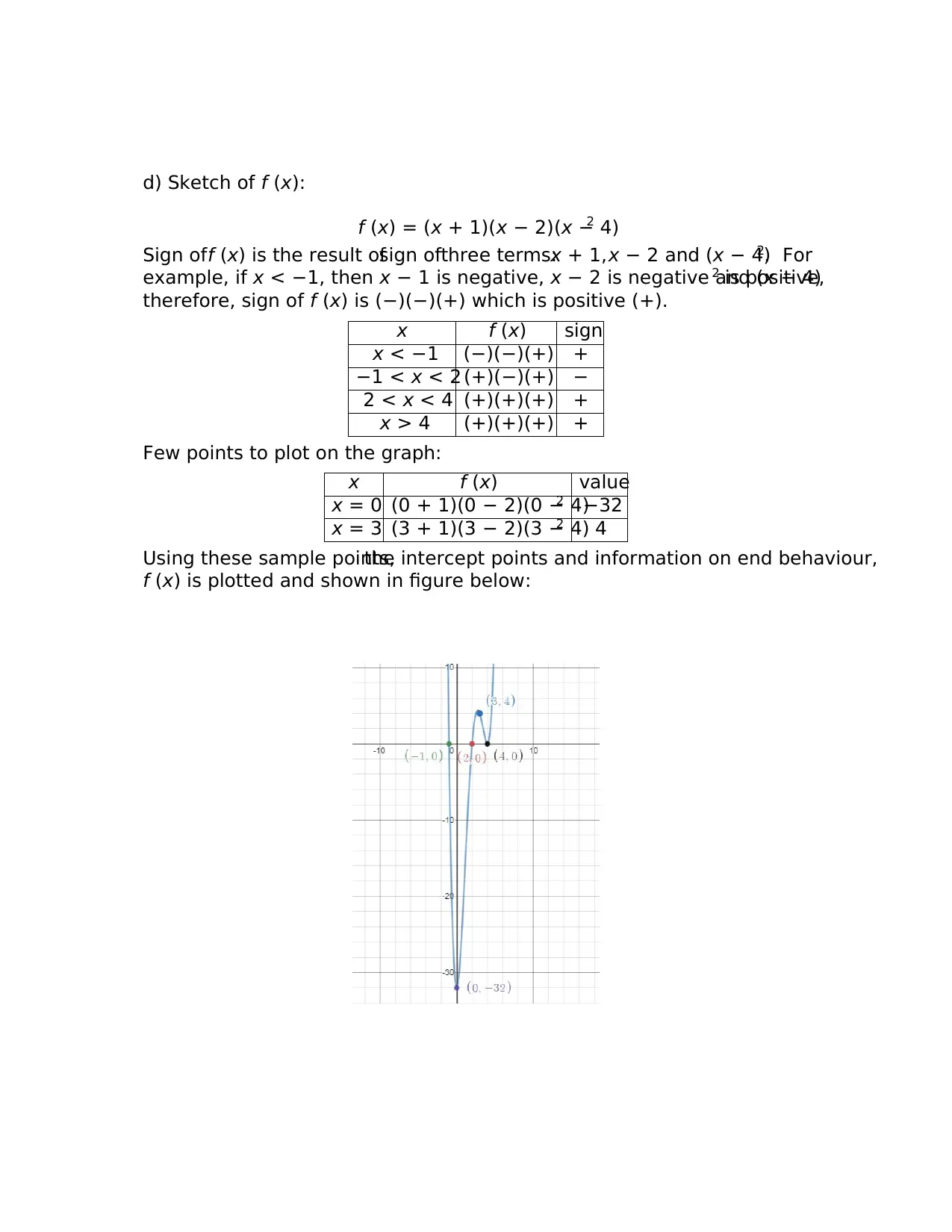
14.
a)
h(x) = |x + 3| − 2
For f (x) = |x|, the transformations required to obtain h(x) are:
1. Horizontal shift to the left by 3
2. Vertical shift downwards by 2
b)
15.
f (x) = 2x − 1
(x + 1)(x − 2)
a)
x- intercept:It is the value for which f (x) = 0, that is:
0 = 2x − 1
(x + 1)(x − 2)
. Implies, 2x − 1 = 0, therefore, the point x = 0.5 on x- axis is the x- intercept.
y- intercept:It is obtained by setting x = 0 in f (x).
f (x) = 0 − 1
(0 + 1)(0 − 2)
= 0.5

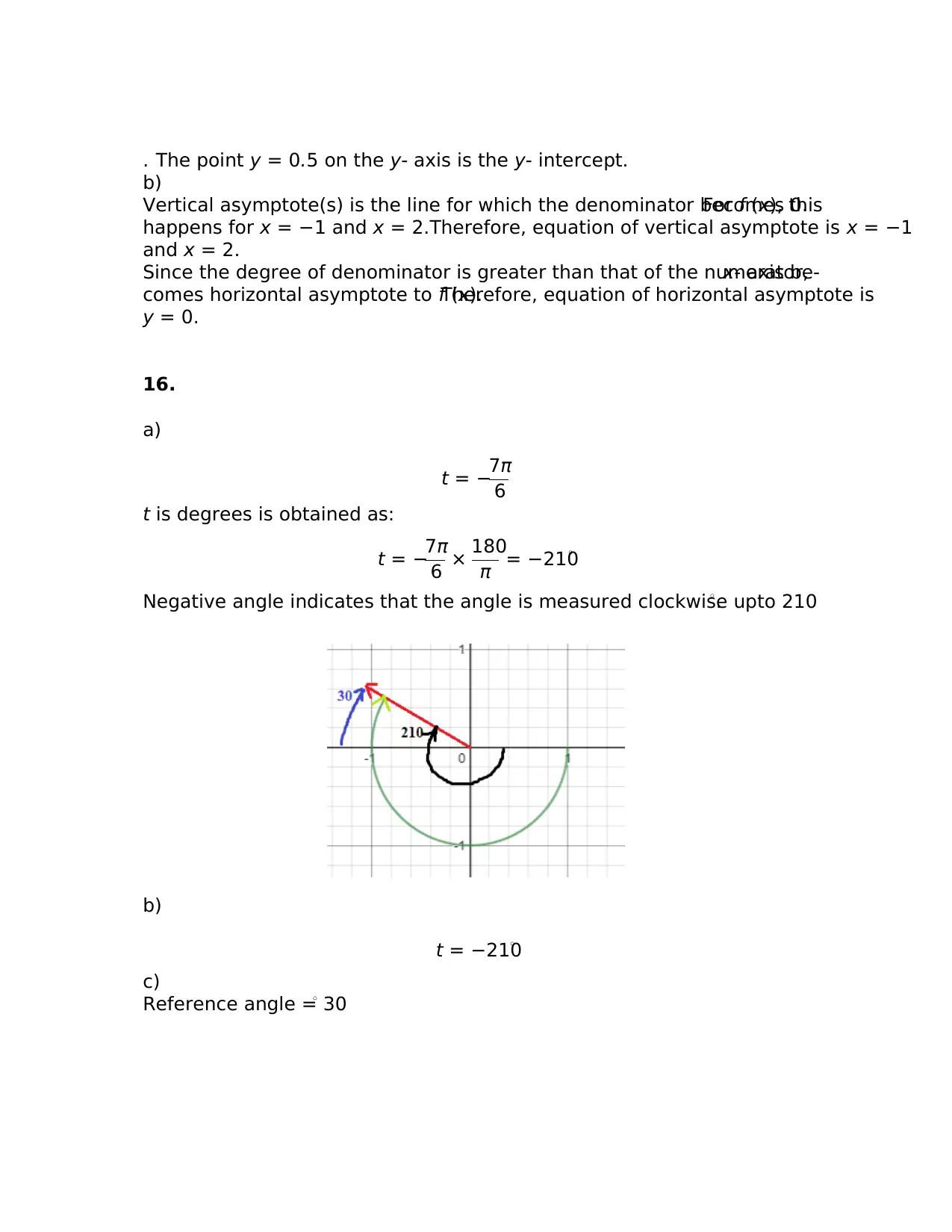
. The point y = 0.5 on the y- axis is the y- intercept.
b)
Vertical asymptote(s) is the line for which the denominator becomes 0.For f (x), this
happens for x = −1 and x = 2.Therefore, equation of vertical asymptote is x = −1
and x = 2.
Since the degree of denominator is greater than that of the numerator,x- axis be-
comes horizontal asymptote to f (x).Therefore, equation of horizontal asymptote is
y = 0.
16.
a)
t = −7π
6
t is degrees is obtained as:
t = −7π
6 × 180
π = −210◦
Negative angle indicates that the angle is measured clockwise upto 210◦.
b)
t = −210◦
c)
Reference angle = 30◦

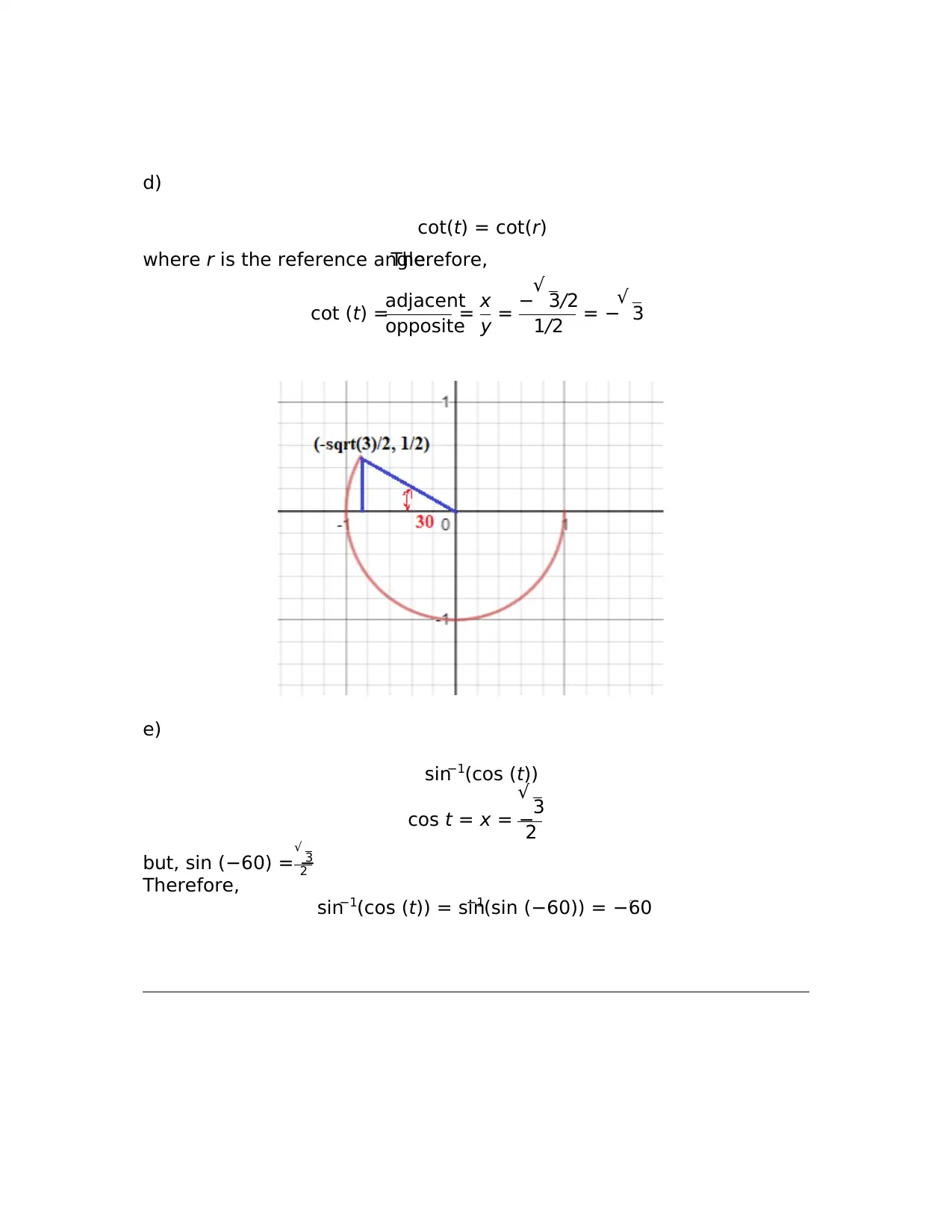
d)
cot(t) = cot(r)
where r is the reference angle.Therefore,
cot (t) =
adjacent
opposite
= x
y = −√ 3/2
1/2 = −√ 3
e)
sin−1(cos (t))
cos t = x = −
√ 3
2
but, sin (−60) = −
√ 3
2
Therefore,
sin−1(cos (t)) = sin−1(sin (−60)) = −60◦

References:
1. Sepanski,M. R. (2010). Algebra. Providence,R.I., American Mathematical
Society.
http://public.eblib.com/choice/publicfullrecord.aspx?p=4718110.
2. Cohen, L., Theodore B.,Sklar, D. (2017).Precalculus.Cengage Learning.