Calculus Assignment: Proving Integral Convergence/Divergence

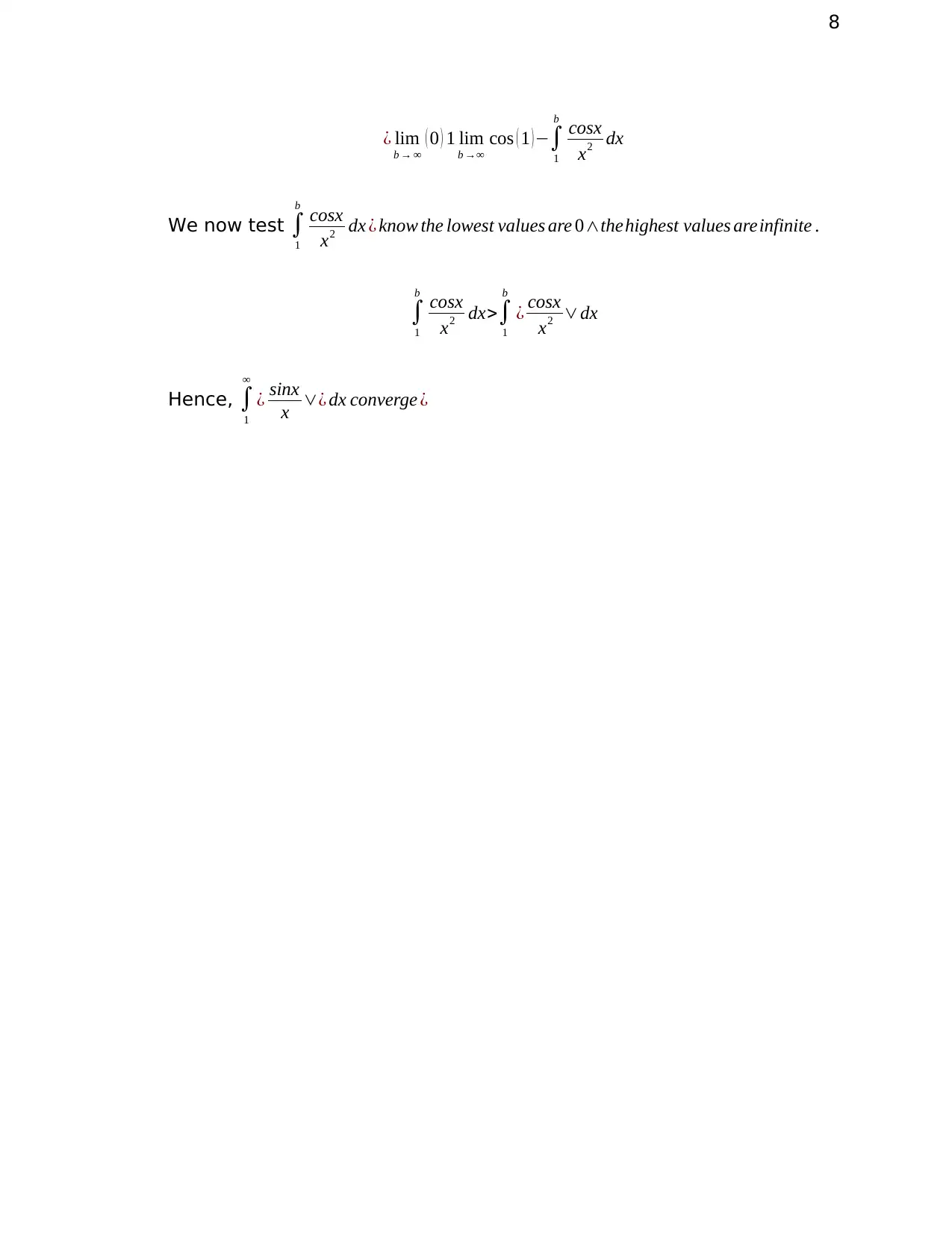
This calculus assignment delves into the analysis of integral convergence and divergence. The student provides detailed solutions to prove whether various improper integrals converge or diverge. The assignment utilizes techniques such as integration by parts and limit evaluation to determine the be...Read More

1
Calculus
Student’s Name:
University Affiliation:

2
Part a
If f(x)=|f(x)|
Then a ≤ f ( x ) ≤ b∨x ≥ 1
Now let us determine whether f(x) converges or not
∫
1
∞
f ( x ) dx=¿ lim
b → ∞
∫
1
b
f ( x ) dx ¿
¿ lim
b → ∞
¿
¿ lim
b → ∞ [ f ( b )a+1
a+1 ]−[ f ( 1 )a +1
a+ 1 ]
¿ lim
b → ∞ [ −f ( 1 ) a +1
a+1 ] +[ f ( b ) a +1
a+1 ]
¿ [ 1
a+1 ]
Therefore, |f(x)| diverge since P<1
Part b
Prove that ∫
1
∞
cosx
x2 dx converges .
Solution
Using integration by parts
∫u v' dx=uv −∫ u' vdx

3
Where u= 1
x2 , v'=∫ cosxdx ,u'=−2
x3 , v=sinx
∫ −2
x3 ∗cosxdx = sinx
x2 +2∫ sinx
x3 dx
Introducing limits
∫
1
∞
cosx
x2 =lim
b → ∞
∫
1
b
cosx
x3 dx
¿ lim
b → ∞
¿
¿ lim
b → ∞ {[ sin ( b )
( b )3 ]− [ sin ( 1 )
( 1 )3 ] }+ lim
b → ∞
2∫
1
b
sinx
x3 dx
¿ lim
b → ∞
[ 0 ]−sin [ 1 ] +lim
b →∞
2∫
1
b
sinx
x3 dx
From the equation above, lim
b → ∞
2∫
1
b
sinx
x3 dx ≤ lim
b →∞
2∫
1
b
¿ sinx∨ ¿
x3 dx ¿
The absolute value |sin x| when graphed produces a positive graph
with 1 maximum and 0 minimum.
Considering the highest value: 1, it becomes
≤ lim
b →∞
2∫
1
b
1
x3 dx which converges.
Therefore, the ∫
1
∞
cosx
x2 dx converge .
Part c

4
Prove that ∫
1
∞
sinx
x dx converges .
Solution
We will use integration by parts which is stated as:
∫u v' dx=uv −∫ u' vdx
Let u= 1
x , v'=sinxdx , v=−cosx
∫ 1
x . sinxdx= [ 1
x ∗( −cosx ) ] −∫ 1/ x2 (cosx) dx
¿ [ −cosx
x ]−∫(cosx )/ x2 dx
Introducing the limits
∫
1
∞
sinx
x dx= lim
n→ ∞
∫
1
n
sinx
x dx
¿ lim
n → ∞ ([−cosx
x2 ) ] b
1−∫
1
n
cosx
x2 dx ¿
¿ lim
n → ∞
{
([ −cos (n)
(n)2 ) ] +¿
lim
n → ∞
( 0 ) +cos ( 1 ) −lim
n →∞
∫
1
n
cosx
x2 dx

5
Now taking lim
n → ∞
∫
1
n
cosx
x2 dxand testing, we will find that
lim
n → ∞
∫
1
n
cosx
x2 dx ≤ lim
n → ∞
∫
1
n
¿ cosx ∨ ¿
x2 dx ¿
And the absolute values of |cos x| ranges between 0 and 1.
Taking the highest value i.e. 1, it will become
≤ lim
n →∞
∫
1
n
1
x2 dx which converges.
Since, lim
n → ∞
∫
1
n
cosx
x2 dx ≤ lim
n → ∞
∫
1
n
¿ cosx∨ ¿
x2 dx ¿ , hence ∫
1
∞
sinx
x dx converges .
Part d
Prove that ∫
1
∞
1−cos 2 x
x dx di verges .
Hint: Split the expression inside the integral into two parts.
Solution.
∫
1
∞
1−cos 2 x
x dx =∫
1
∞
1
x dx−∫
1
∞
cos 2 x
x dx
The second part of the equation would be solved using integration by
parts ∫u v' dx=uv −∫ u' vdx
u= 1
x , u' = 1
x2 , v'=∫cos 2 x dx , v =sin 2 x
2

6
∫
1
∞
1−cos 2 x
x = [ −1
x2 ] −{( sin 2 x
2 x )−∫ sin 2 x
2 x2 dx }
Let us introduce limits
∫
1
∞
1−cos 2 x
x =lim
b → ∞
∫
1
b
1
x dx−lim
b → ∞
∫
1
b
cos 2 x
x dx
¿ [−1
x2 ]−{( sin 2 x
2 x )−∫ sin 2 x
2 x2 dx }
¿ lim
b → ∞ [−1
x2 ] b
1−{lim
b → ∞
( sin 2 x
2 x )
b
1− lim
b →∞ ∫
1
b
sin 2 x
2 x2
dx }
¿ lim
b → ∞
[ 0 ] −1−lim
b →∞
[ 0 ] −sin [ 2 ] −lim
b → ∞
∫
1
b
sin2 x
2 x2 dx
Now takin
lim
b → ∞
∫
1
b
sin2 x
2 x2 dx∧testing , we will find that lim
b → ∞
∫
1
b
sin2 x
2 x2 dx ≥ lim
b → ∞
∫
1
b
¿ sin 2 x∨ ¿
2 x2 dx ¿
The absolute |sin 2x| ranges between 0 and 2.5
Taking the largest value, we will obtain
≥ lim
b →∞
∫
1
b
5
4 x2 dx
Hence ∫
1
∞
1−cos 2 x
x dx diverges .

7
Part e
Prove that ∫
1
∞
¿ sinx
x ∨¿ dx converges . ¿
Hint: Use the fact that |sin x| ≤ Sin2 x ∀ ϵR
Solutions.
When dealing with absolute values, we would only consider positive
values.
We use integration by parts to obtain or solutions
∫u v' dx=uv −∫ u' vdx
∫ 1
x . sinxdx=[ cox
x ¿ ]−∫ cosx
x2 dx ¿
We now introduce our limits
∫
1
∞
¿ sinx
x ∨¿=lim
b →∞
∫
1
b
sinx
x2 dx ¿
Solving the above we obtain
¿ lim
b → ∞
¿
¿ lim
b → ∞
( [ cos ( b )
b2 ] + cos ( 1 )
1 )−∫
1
b
cosx
x2 dx

8
¿ lim
b → ∞
( 0 ) 1 lim
b →∞
cos ( 1 ) −∫
1
b
cosx
x2 dx
We now test ∫
1
b
cosx
x2 dx ¿ know the lowest values are 0∧thehighest values areinfinite .
∫
1
b
cosx
x2 dx>∫
1
b
¿ cosx
x2 ∨dx
Hence, ∫
1
∞
¿ sinx
x ∨¿ dx converge ¿

9
References
Grosser, D. (2014). Improper Integrals.
Lay, D. (2012). Linear Algebra and Its Applications. University of Mary Land.

10