Trigonometric Function Properties

This document explains the properties of trigonometric functions, including amplitude, period, and maximum/minimum values. It also provides examples and graphs to illustrate these concepts.

1. Consider the trigonometric function f ( t )=−1+4 sin ( 1
2 π ( t−1 ) )
a) What is the amplitude of f ( t )?
b) What is the period of f ( t )?
c) What are the maximum and minimum values attained by f ( t )?
d) Sketch the graph of f ( t ) for t ∈ [−1,3 ]
Answer:
A sine wave of the form (Miller, 2010):
y ( t ) =D+ A sin ( B ( t +C ) )
has:
Amplitude = A
Period = 2 π
B
Phase shift = C (positive value means shift is to the left)
Vertical shift = D
f ( t )=−1+ 4 sin ( 1
2 π ( t−1 ) )
Comparing with the standard form ( y ( t ) ), we get:
A=4 , B= π
2 , C=−1 , D=−1
a) Amplitude of f ( t ) is A that is 4
b) Period of f ( t ) is:
Period= 2 π
B =2 π
π
2
=4
c) Maximum and minimum values of f ( t ):
f ( t )=−1+ 4 sin ( 1
2 π ( t−1 ) )
Maximum value of sin ( 1
2 π ( t −1 ) ) is 1 and minimum is -1 (Property of sine function)
therefore,
Max f ( t )=−1+ 4 · Max sin ( π
2 ( t−1 ) )=−1+4 · 1=3

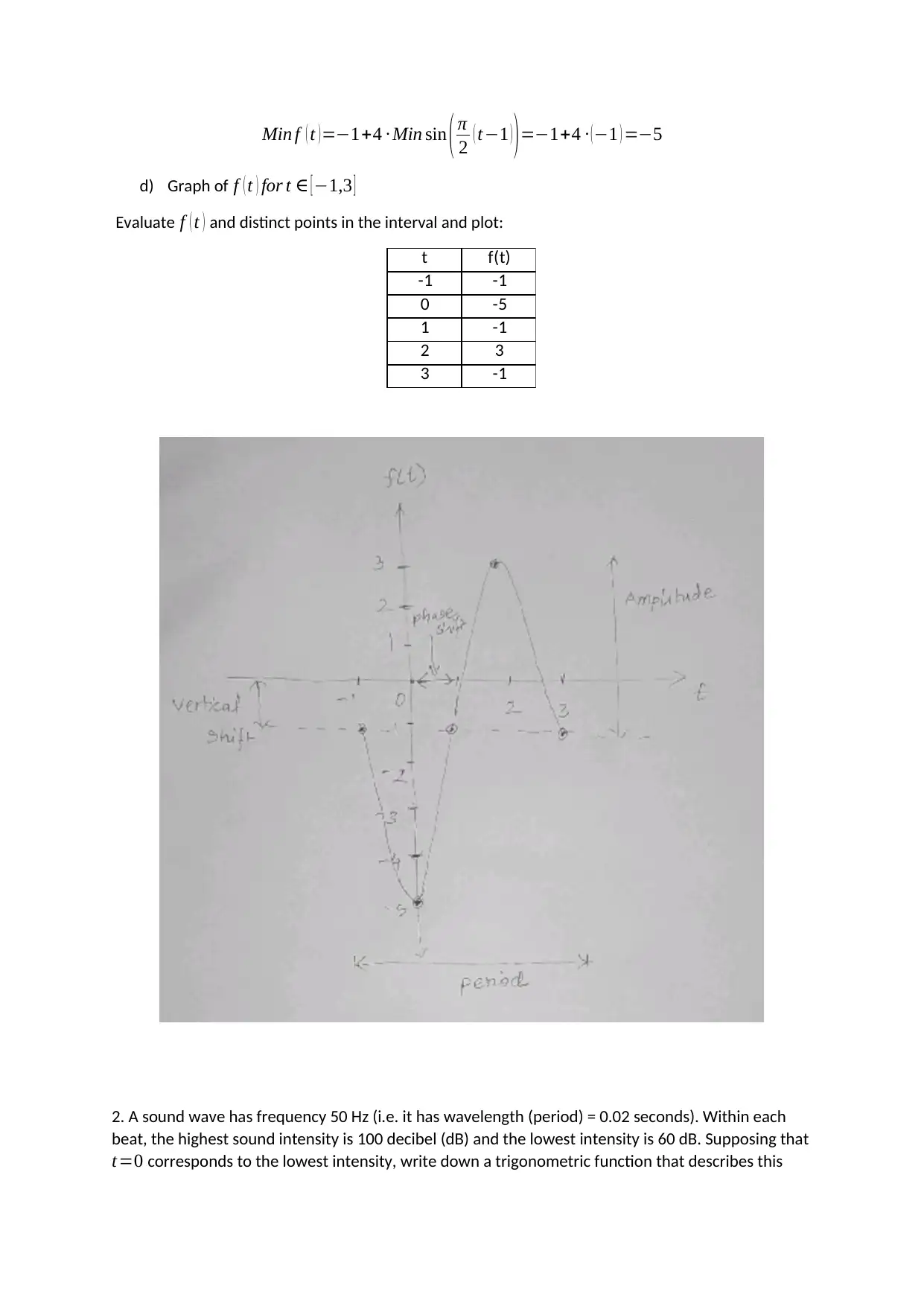
Min f ( t )=−1+4 · Min sin ( π
2 ( t−1 ) )=−1+4 · (−1 ) =−5
d) Graph of f ( t ) for t ∈ [−1,3 ]
Evaluate f ( t ) and distinct points in the interval and plot:
t f(t)
-1 -1
0 -5
1 -1
2 3
3 -1
2. A sound wave has frequency 50 Hz (i.e. it has wavelength (period) = 0.02 seconds). Within each
beat, the highest sound intensity is 100 decibel (dB) and the lowest intensity is 60 dB. Supposing that
t=0 corresponds to the lowest intensity, write down a trigonometric function that describes this

sound wave. Sketch the sound wave over two periods. (Hind: Since period = 0.02 seconds which is
very small, make the scale on the horizontal axis really big when you sketch the graph).
Answer:
A sine wave of the form (Miller, 2010):
y ( t ) =D+ A sin ( B ( t +C ) )
has:
Amplitude = A
Period = 2 π
B
Phase shift = C (positive value means shift is to the left)
Vertical shift = D
In order to fit the given function into trigonometric (sine) function, we need to determine the
constants A , B , C and D
Amplitude of the function is also defined as:
A= ymax − ymin
2
where, ymax is the maximum value (intensity) of the function which is given as 100. Similarly,
ymin=60. Therefore,
A=100−60
2 =20
The shift is obtained as:
D= ymax− ymin
2 = 100+60
2 =80
Period is 0.02, which means:
B= 2 π
P = 2 π
0.02 =100 π
Therefore,
y ( t ) =80+20 sin ( 100 π ( t +C ) )
The horizontal shift ( C ) is determined in the following way:
At t=0, the function has lowest intensity (=60)
⇒ 60=80+20 sin ( 100 πC ) ⇒−1=sin ( 100 πC )
Taking inverse on both sides:

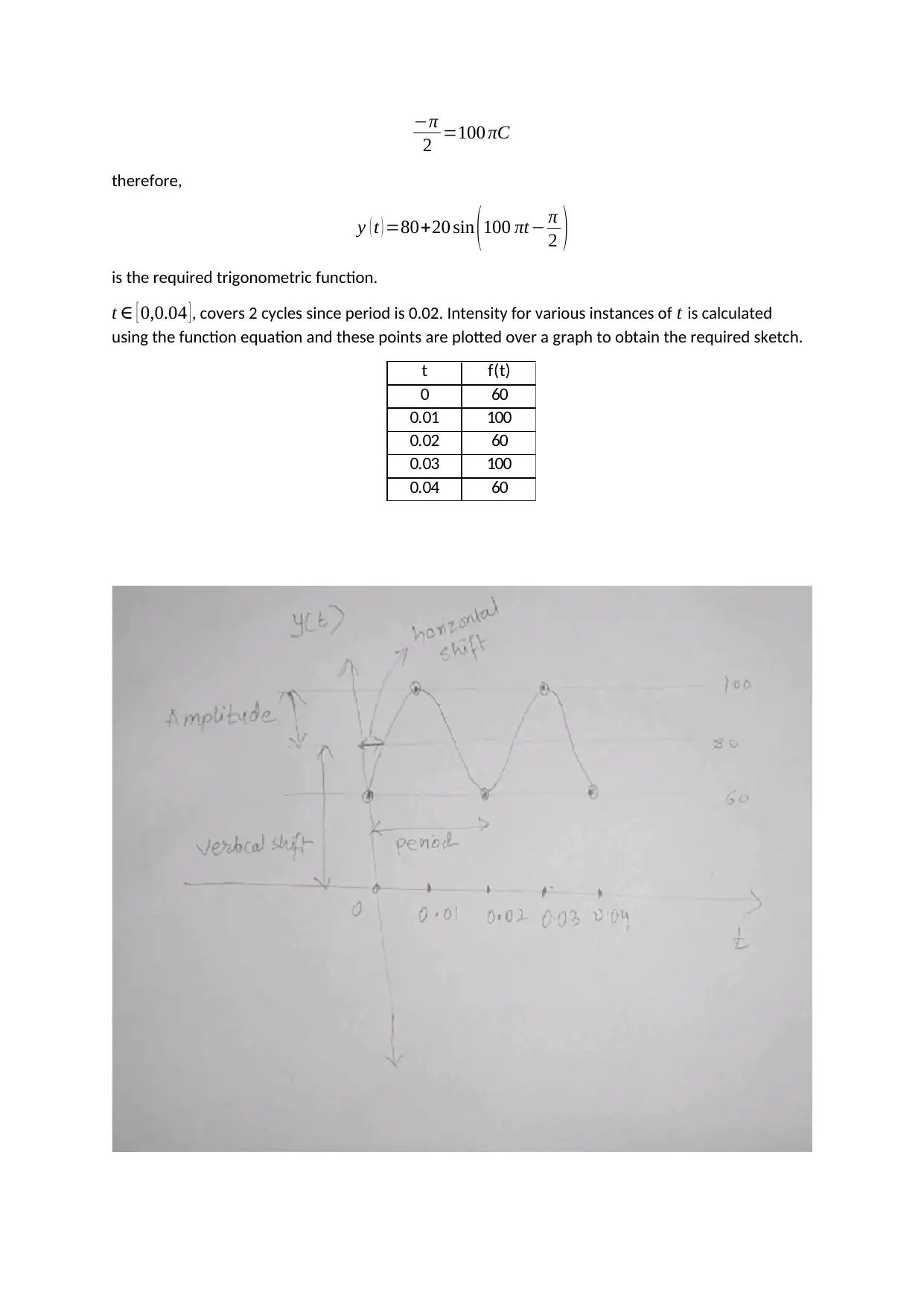
−π
2 =100 πC
therefore,
y ( t ) =80+20 sin (100 πt− π
2 )
is the required trigonometric function.
t ∈ [ 0,0.04 ] , covers 2 cycles since period is 0.02. Intensity for various instances of t is calculated
using the function equation and these points are plotted over a graph to obtain the required sketch.
t f(t)
0 60
0.01 100
0.02 60
0.03 100
0.04 60

3. The volume of water in a tank at a particular time (measured in seconds) is given by
V ( t ) =5 t ( 2−t ) m3. Find the rate of change of the volume of water in the tank from first principles
(using definition of rate of change).
Answer:
Volume of water in tank at any instant of time (t) is given by:
V ( t ) =5 t ( 2−t ) m3
¿ 10 t−5 t2
Rate of change of volume is (Stewart, 2012, 105-203):
d
dt V m3
sec
From the first principles:
d
dt V =li mh →0
V ( t +h )−V ( t )
h
Substitute the expression for V and evaluate the limit.
d
dt V =li mh →0
[ 10 ( t +h ) −5 ( t+h )2 ]− ( 10 t −5 t2 )
h =li mh →0
[ 10 t+10 h−5 ( t2 +h2 +2 th ) ]− ( 10 t−5 t 2 )
h =li mh → 0
[ 10 t+
¿ li mh →0 10−5 h−10 t
Taking limit to each term eliminates 5 h, as it becomes 0.
Therefore,
Rate of change of volume (Stewart, 2012, 105-203):
d
dt V =10−10 t m3
sec
4. Consider the function f ( x )=x2−1. Find the equation of the tangent to the graph of f ( x ) at x=3,
[NOTE: when calculating f ' ( 3 ), use first principles.]
Answer:
f ( x)=x2 – 1
Equation of tangent line to a curve at point ( x0 , y0 ) is given by the expression (Stewart, 2012, 105-
203):
y− y0=f ' ( x0 ) ( x−x0 )

Where f ' ( x0 ) is derivative of the function at x=x0, and y=f ( x )
The equation of tangent line is obtained from the fact that slope of curve at a point is same as the
slope of tangent to the curve at that point.
x0=3 ⇒ y0 =f ( x0 )=x0
2−1=32−1=8
Derivative of f ( x ) from first principles is obtained as:
f ' ( x )= d
dx f =li mh → 0
f ( x +h ) −f ( x )
h
Therefore, for f ( x )=x2−1,
f ' ( x)=li mh → 0
( ( x+h ) 2−1 ) − ( x2−1 )
h
¿ li mh →0
( x +h )2 −x2
h
¿ li mh →0
x2 +2 xh+h2−x2
h
¿ li mh →0
2 xh+h2
h =li mh → 0 2 x+h
Taking limit of each term in the last expression eliminates h, as it goes to 0, therefore,
f ' ( x)=2 x
At x=x0:
f ' ( x0 ) =2 ( x0 )=2 ( 3 )=6
Now, as all the terms in the tangent line equation are determined, we get:
y− y0=f ' ( x0 ) ( x−x0 ) ⇒ y−8=6 ( x−3 )
⇒ y =6 ( x−3 ) +8
Is the equation of tangent line to curve f ( x ) =x2 – 1, at point x=3
References:
1) Stewart, James. “Derivatives.” In Calculus, 105-203. Boston: Cengage Learning, 2012.
2) Miller, Haynes. “Sinusoidal Functions.” MITOPENCOURSWARE. Spring, 2010.
https://ocw.mit.edu/courses/mathematics/18-03sc-differential-equations-fall-2011/unit-i-
first-order-differential-equations/sinusoidal-functions/MIT18_03SCF11_s7_1text.pdf