Relationship between Retention Rate and Graduation Rate in Universities
VerifiedAdded on 2023/04/21
|9
|1992
|360
AI Summary
This paper explores the relationship between retention rate and graduation rate in universities and provides recommendations for improving graduation rates. The analysis is based on data from 29 universities and includes descriptive analysis, scatter diagram, regression equation, and statistical significance of the relationship.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

1
ECONOMICS ASSIGNMENT
ECONOMICS ASSIGNMENT
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

2
Contents
Purpose.......................................................................................................................................3
Background................................................................................................................................3
Methods......................................................................................................................................3
Results........................................................................................................................................3
a) Descriptive analysis of the variable....................................................................................3
b) Scatter diagram...................................................................................................................4
c) Regression Equation...........................................................................................................4
d) Meaning of slope coefficient..............................................................................................5
e) Statistical significance of the relationship..........................................................................5
f) The goodness of fit of the regression..................................................................................6
g) The case of South University.............................................................................................6
h) The case of the University of Phoenix...............................................................................6
Discussion..................................................................................................................................7
Recommendations......................................................................................................................7
Reference....................................................................................................................................8
Contents
Purpose.......................................................................................................................................3
Background................................................................................................................................3
Methods......................................................................................................................................3
Results........................................................................................................................................3
a) Descriptive analysis of the variable....................................................................................3
b) Scatter diagram...................................................................................................................4
c) Regression Equation...........................................................................................................4
d) Meaning of slope coefficient..............................................................................................5
e) Statistical significance of the relationship..........................................................................5
f) The goodness of fit of the regression..................................................................................6
g) The case of South University.............................................................................................6
h) The case of the University of Phoenix...............................................................................6
Discussion..................................................................................................................................7
Recommendations......................................................................................................................7
Reference....................................................................................................................................8
3
Purpose
Student retention and rate of graduation is an important parameter to measure the
performance of the university. The purpose of this paper is to find out how the retention rate
in universities is related to the graduation rate. The answers to this question will enable the
universities to improve the rate of graduation which depends on an independent variable.
Background
The graduation rate is referred to as the percentage of students of the first year successfully
complete the course within the time stipulated to the student. On the other hand, the
Retention rate is the rate at which the first year students of the universities continue their
classes in the next year (Tchamdjeu, Steenkamp and Nel, 2018). To an economist, these to
variables pertaining to the operation of universities are important as it would help in
improving the performance of the universities. If the retention of students can be increased
through improving the quality of education or by reducing the cost, there is a higher chance
of having a huge number of students to become graduate from the university (Cancado,
Reisel and Walker, 2018).
Methods
This paper uses data of retention rate and the graduation rate from 29 universities. The
numerical data has been analyzed through the process of data analysis. Statistical regression
has been done on the data collected where the graduation rate of the universities have been
taken as the dependent variable. On the other hand, the retention rate variable has been
considered as the independent variable. MS Excel has been used as a tool to analyze the data
that has presented an ANOVA table. This table shows how the independent variable retention
rate influences the value of the dependent variable graduation rate.
Results
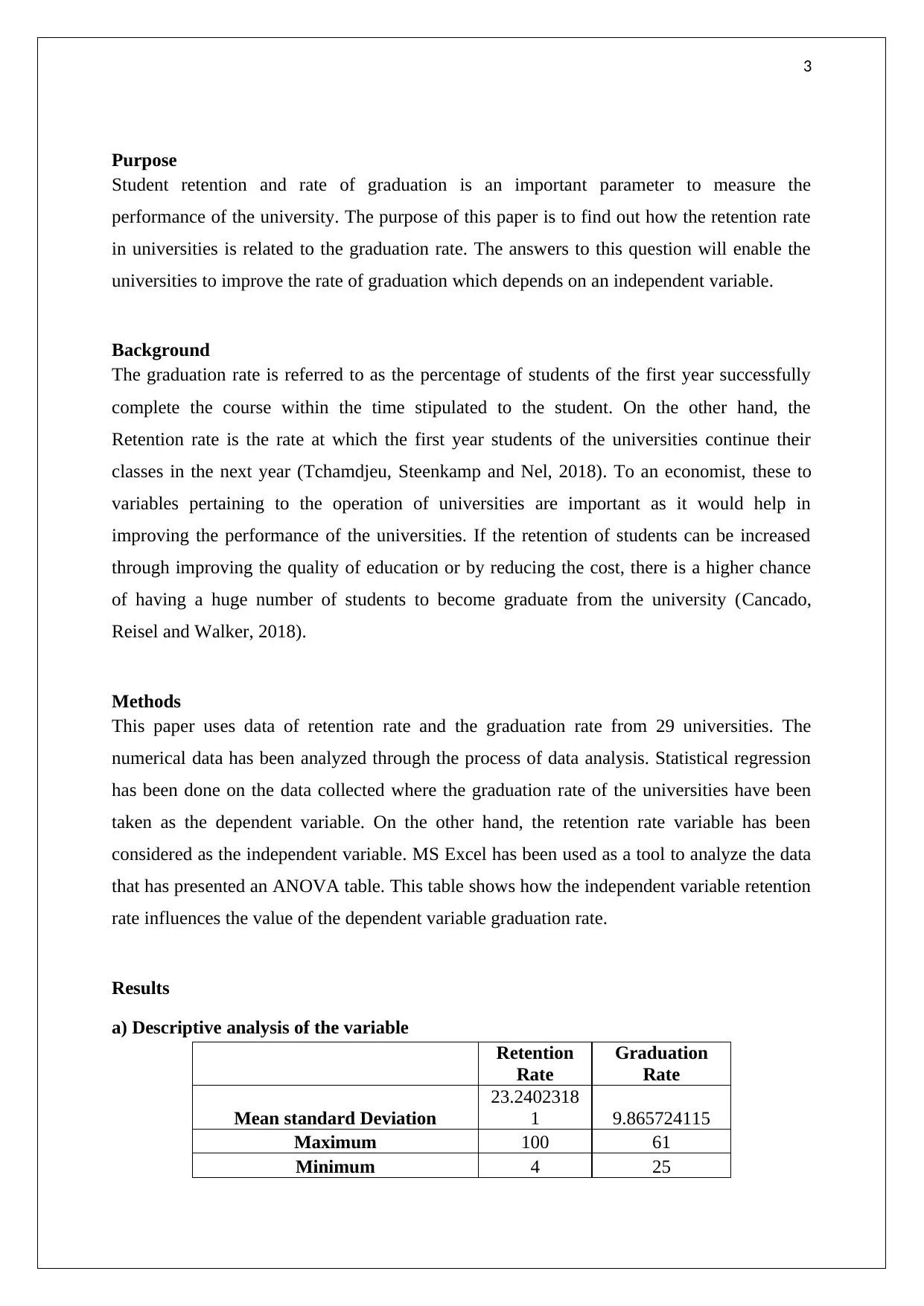
a) Descriptive analysis of the variable
Retention
Rate
Graduation
Rate
Mean standard Deviation
23.2402318
1 9.865724115
Maximum 100 61
Minimum 4 25
Purpose
Student retention and rate of graduation is an important parameter to measure the
performance of the university. The purpose of this paper is to find out how the retention rate
in universities is related to the graduation rate. The answers to this question will enable the
universities to improve the rate of graduation which depends on an independent variable.
Background
The graduation rate is referred to as the percentage of students of the first year successfully
complete the course within the time stipulated to the student. On the other hand, the
Retention rate is the rate at which the first year students of the universities continue their
classes in the next year (Tchamdjeu, Steenkamp and Nel, 2018). To an economist, these to
variables pertaining to the operation of universities are important as it would help in
improving the performance of the universities. If the retention of students can be increased
through improving the quality of education or by reducing the cost, there is a higher chance
of having a huge number of students to become graduate from the university (Cancado,
Reisel and Walker, 2018).
Methods
This paper uses data of retention rate and the graduation rate from 29 universities. The
numerical data has been analyzed through the process of data analysis. Statistical regression
has been done on the data collected where the graduation rate of the universities have been
taken as the dependent variable. On the other hand, the retention rate variable has been
considered as the independent variable. MS Excel has been used as a tool to analyze the data
that has presented an ANOVA table. This table shows how the independent variable retention
rate influences the value of the dependent variable graduation rate.
Results
a) Descriptive analysis of the variable
Retention
Rate
Graduation
Rate
Mean standard Deviation
23.2402318
1 9.865724115
Maximum 100 61
Minimum 4 25
4
Table 1: The descriptive analysis of the variables
(Source: Developed by the learner)
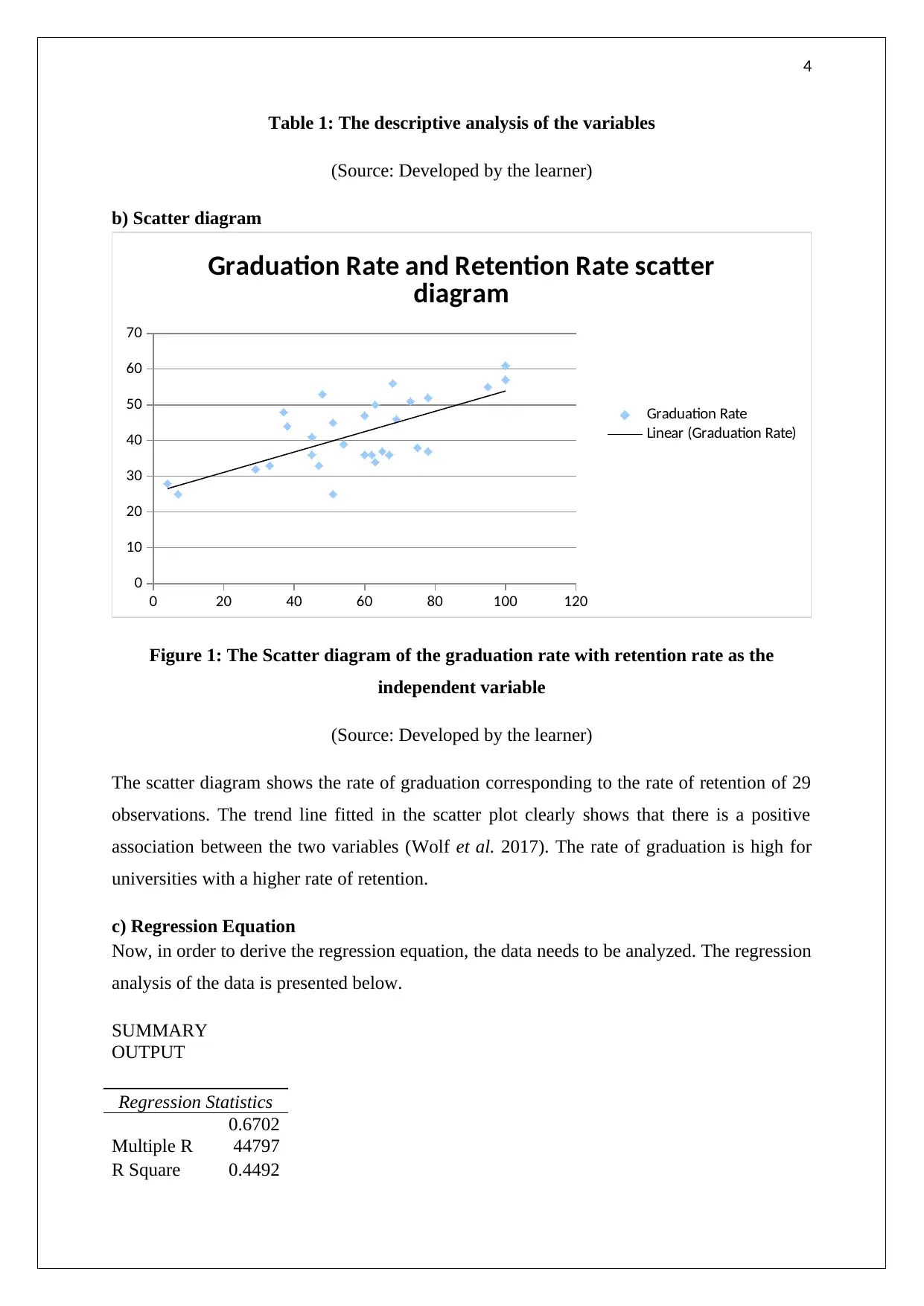
b) Scatter diagram
0 20 40 60 80 100 120
0
10
20
30
40
50
60
70
Graduation Rate and Retention Rate scatter
diagram
Graduation Rate
Linear (Graduation Rate)
Figure 1: The Scatter diagram of the graduation rate with retention rate as the
independent variable
(Source: Developed by the learner)
The scatter diagram shows the rate of graduation corresponding to the rate of retention of 29
observations. The trend line fitted in the scatter plot clearly shows that there is a positive
association between the two variables (Wolf et al. 2017). The rate of graduation is high for
universities with a higher rate of retention.
c) Regression Equation
Now, in order to derive the regression equation, the data needs to be analyzed. The regression
analysis of the data is presented below.
SUMMARY
OUTPUT
Regression Statistics
Multiple R
0.6702
44797
R Square 0.4492
Table 1: The descriptive analysis of the variables
(Source: Developed by the learner)
b) Scatter diagram
0 20 40 60 80 100 120
0
10
20
30
40
50
60
70
Graduation Rate and Retention Rate scatter
diagram
Graduation Rate
Linear (Graduation Rate)
Figure 1: The Scatter diagram of the graduation rate with retention rate as the
independent variable
(Source: Developed by the learner)
The scatter diagram shows the rate of graduation corresponding to the rate of retention of 29
observations. The trend line fitted in the scatter plot clearly shows that there is a positive
association between the two variables (Wolf et al. 2017). The rate of graduation is high for
universities with a higher rate of retention.
c) Regression Equation
Now, in order to derive the regression equation, the data needs to be analyzed. The regression
analysis of the data is presented below.
SUMMARY
OUTPUT
Regression Statistics
Multiple R
0.6702
44797
R Square 0.4492
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
5
28088
Adjusted R
Square
0.4288
29129
Standard
Error
7.4561
04604
Observation
s 29
ANOVA
df SS MS F
Signific
ance F
Regression 1
1224.28
5956
1224.2
85956
22.022
10775
6.9549
1E-05
Residual 27
1501.02
4388
55.593
49587
Total 28
2725.31
0345
Coeffic
ients
Standar
d Error t Stat
P-
value
Lower
95%
Upper
95%
Lower
95.0%
Upper
95.0%
Intercept
25.422
90363
3.74628
3822
6.7861
65928
2.7441
E-07
17.736
16425
33.109
64301
17.736
16425
33.109
64301
RR(%)
0.2845
26003
0.06063
0691
4.6927
71862
6.9549
1E-05
0.1601
22101
0.4089
29904
0.1601
22101
0.4089
29904
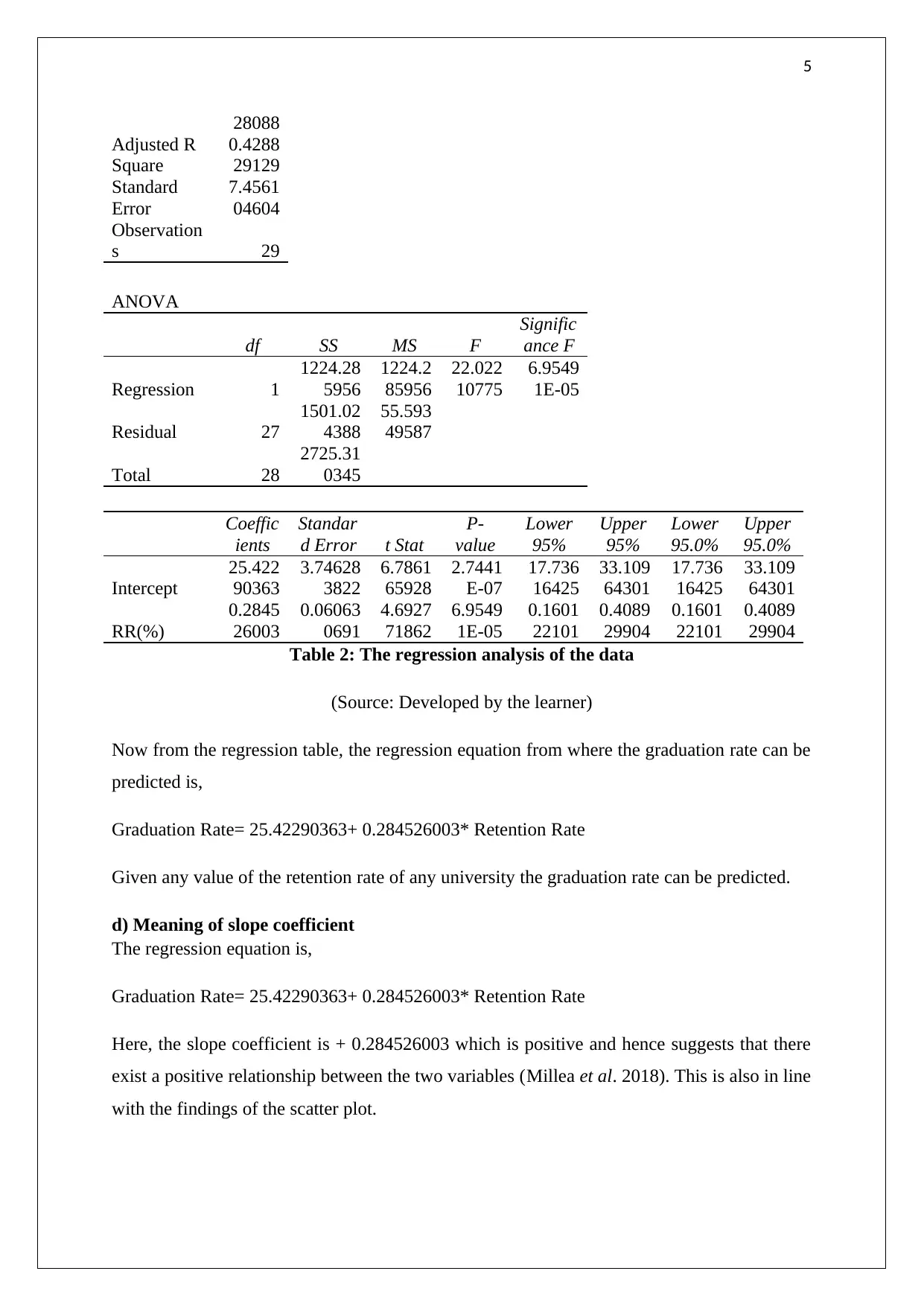
Table 2: The regression analysis of the data
(Source: Developed by the learner)
Now from the regression table, the regression equation from where the graduation rate can be
predicted is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Given any value of the retention rate of any university the graduation rate can be predicted.
d) Meaning of slope coefficient
The regression equation is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Here, the slope coefficient is + 0.284526003 which is positive and hence suggests that there
exist a positive relationship between the two variables (Millea et al. 2018). This is also in line
with the findings of the scatter plot.
28088
Adjusted R
Square
0.4288
29129
Standard
Error
7.4561
04604
Observation
s 29
ANOVA
df SS MS F
Signific
ance F
Regression 1
1224.28
5956
1224.2
85956
22.022
10775
6.9549
1E-05
Residual 27
1501.02
4388
55.593
49587
Total 28
2725.31
0345
Coeffic
ients
Standar
d Error t Stat
P-
value
Lower
95%
Upper
95%
Lower
95.0%
Upper
95.0%
Intercept
25.422
90363
3.74628
3822
6.7861
65928
2.7441
E-07
17.736
16425
33.109
64301
17.736
16425
33.109
64301
RR(%)
0.2845
26003
0.06063
0691
4.6927
71862
6.9549
1E-05
0.1601
22101
0.4089
29904
0.1601
22101
0.4089
29904
Table 2: The regression analysis of the data
(Source: Developed by the learner)
Now from the regression table, the regression equation from where the graduation rate can be
predicted is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Given any value of the retention rate of any university the graduation rate can be predicted.
d) Meaning of slope coefficient
The regression equation is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Here, the slope coefficient is + 0.284526003 which is positive and hence suggests that there
exist a positive relationship between the two variables (Millea et al. 2018). This is also in line
with the findings of the scatter plot.

6
The value of the slope coefficient is 0.284526003 which means that with one unit increase in
the retention rate, the graduation rate increases by 0.284526003 units.
e) Statistical significance of the relationship
The statistical significance of the association between the two variables can be checked
through the p-value of the variable and the overall significance of the regression analysis. The
variable retention rate, as shown by the regression table reflects a p-value of 0.0000695491
which is extremely low (Dagley et al. 2016). That means the probability of random error is
low and hence the association is statistically significant. The overall F value is also low
which confirms that the association between the variables are statistically significant.
f) The goodness of fit of the regression
The goodness of the fit of the analysis is checked by studying the R square value of the
analysis. This value shows the extent to which the variability in the dependent variable is
explained by the independent variable. This value lies between 0 and 1 where 1 suggests that
variability in the dependent variable is completely explained by the independent variable
(Mancini, Cipher and Ganji, 2018). Here, in this case, the value of R square is 0.449228088
which is not impressive but good to arrive at the conclusion that retention rate slightly
explains the variations in the graduation rates in the universities. That means, retention rate
does not fully explain the changes in the value of the dependent variable and hence there
might be other variables which may influence the value of graduation rate as well (Chiyaka et
al. 2016).
g) The case of South University
The Regression equation is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Now putting the value of Retention rate in the case of South University we get the value of
Graduation Rate as
25.42290363+ 0.284526003* 51 = 39.93372978
In reality, the graduation rate of the university is 25. Thus, as per the prediction, the
graduation rate should have been more given the retention rate of the university (Callahan
and King, 2018).
The value of the slope coefficient is 0.284526003 which means that with one unit increase in
the retention rate, the graduation rate increases by 0.284526003 units.
e) Statistical significance of the relationship
The statistical significance of the association between the two variables can be checked
through the p-value of the variable and the overall significance of the regression analysis. The
variable retention rate, as shown by the regression table reflects a p-value of 0.0000695491
which is extremely low (Dagley et al. 2016). That means the probability of random error is
low and hence the association is statistically significant. The overall F value is also low
which confirms that the association between the variables are statistically significant.
f) The goodness of fit of the regression
The goodness of the fit of the analysis is checked by studying the R square value of the
analysis. This value shows the extent to which the variability in the dependent variable is
explained by the independent variable. This value lies between 0 and 1 where 1 suggests that
variability in the dependent variable is completely explained by the independent variable
(Mancini, Cipher and Ganji, 2018). Here, in this case, the value of R square is 0.449228088
which is not impressive but good to arrive at the conclusion that retention rate slightly
explains the variations in the graduation rates in the universities. That means, retention rate
does not fully explain the changes in the value of the dependent variable and hence there
might be other variables which may influence the value of graduation rate as well (Chiyaka et
al. 2016).
g) The case of South University
The Regression equation is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Now putting the value of Retention rate in the case of South University we get the value of
Graduation Rate as
25.42290363+ 0.284526003* 51 = 39.93372978
In reality, the graduation rate of the university is 25. Thus, as per the prediction, the
graduation rate should have been more given the retention rate of the university (Callahan
and King, 2018).

7
Therefore, as the president of the university, I would be concerned about the performance
compared to the other universities.
h) The case of the University of Phoenix
The Regression equation is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Now putting the value of Retention rate in case of University of Phoenix we get the value of
Graduation Rate as
25.42290363+ 0.284526003*4= 26.56100764
In reality, the graduation rate for the university is 28 which are more than the predicted value
(Queener and Ford, 2019). Therefore as a president of the university, I would have no
concern regarding the performance of the university.
Discussion
Therefore the results show that the variables used in the study are moderately associated with
each other. However, there are limitations in data which has reduced the robustness of the
findings (Stout et al. 2018). The number of samples for the analysis was very low and hence
reliability of the result is low compared to the other analyses in the same topic. Although the
results are roughly the same compared to the other studies, the data points and the
coefficients are less reliable compared to other studies owing to the limited number of
observation in the study.
Recommendations
There are factors that influence the variable Retention rate of the university. These
include quality of the study, number of professors, cost of education and many more.
The universities need to focus on this to increase the retention rate and hence the
graduation rate.
The universities with a high retention rate need to conduct counseling for the students
in order to improve the performance.
The presidents of the universities should monitor the performance using the prediction
of the regression analysis in order to understand the situation.
Therefore, as the president of the university, I would be concerned about the performance
compared to the other universities.
h) The case of the University of Phoenix
The Regression equation is,
Graduation Rate= 25.42290363+ 0.284526003* Retention Rate
Now putting the value of Retention rate in case of University of Phoenix we get the value of
Graduation Rate as
25.42290363+ 0.284526003*4= 26.56100764
In reality, the graduation rate for the university is 28 which are more than the predicted value
(Queener and Ford, 2019). Therefore as a president of the university, I would have no
concern regarding the performance of the university.
Discussion
Therefore the results show that the variables used in the study are moderately associated with
each other. However, there are limitations in data which has reduced the robustness of the
findings (Stout et al. 2018). The number of samples for the analysis was very low and hence
reliability of the result is low compared to the other analyses in the same topic. Although the
results are roughly the same compared to the other studies, the data points and the
coefficients are less reliable compared to other studies owing to the limited number of
observation in the study.
Recommendations
There are factors that influence the variable Retention rate of the university. These
include quality of the study, number of professors, cost of education and many more.
The universities need to focus on this to increase the retention rate and hence the
graduation rate.
The universities with a high retention rate need to conduct counseling for the students
in order to improve the performance.
The presidents of the universities should monitor the performance using the prediction
of the regression analysis in order to understand the situation.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

8
Reference
Callahan, M.T. and King, K.P., 2018, March. Florida Virtual School Impact on the
Graduation Rate of a Higher Education Honors Program. In 42 and Annual Conference
Proceedings, pp. 21.
Cancado, L., Reisel, J. and Walker, C., 2018. Impacts of a summer bridge program in
engineering on student retention and graduation. Journal of STEM Education, 19(2), pp. 34
Chiyaka, E.T., Sithole, A., Manyanga, F., McCarthy, P. and Bucklein, B.K., 2016.
Institutional Characteristics and Student Retention: What Integrated Postsecondary Education
Data Reveals about Online Learning. Online Journal of Distance Learning
Administration, 19(2), pp. 34-69.
Dagley, M., Georgiopoulos, M., Reece, A. and Young, C., 2016. Increasing retention and
graduation rates through a STEM learning community. Journal of College Student Retention:
Research, Theory & Practice, 18(2), pp.167-182.
Mancini, M.E., Cipher, D.J. and Ganji, D., 2018. Maximizing Retention and Progression to
Graduation in Online Programs: A Case Study in “Designing With the End in Mind”.
In Critical Assessment and Strategies for Increased Student Retention, pp. 211-225.
Millea, M., Wills, R., Elder, A. and Molina, D., 2018. What Matters in College Student
Success? Determinants of College Retention and Graduation Rates. Education, 138(4),
pp.309-322.
Queener, J.E. and Ford, B.A., 2019. Culturally Responsive Mentoring Programs: Impacting
Retention/Graduation Rates of African American Males Attending Predominately White
Institutions. In Overcoming Challenges and Creating Opportunity for African American Male
Students,pp. 120-132
Stout, R., Archie, C., Cross, D. and Carman, C.A., 2018. The relationship between faculty
diversity and graduation rates in higher education. Intercultural Education, 29(3), pp.399-
417..
Tchamdjeu, R.M., Steenkamp, H. and Nel, A.L., 2018, April. Retention based on graduation
rate of male and female industrial engineering students. In Global Engineering Education
Conference (EDUCON), 2018 IEEE (pp. 738-745). IEEE.
Reference
Callahan, M.T. and King, K.P., 2018, March. Florida Virtual School Impact on the
Graduation Rate of a Higher Education Honors Program. In 42 and Annual Conference
Proceedings, pp. 21.
Cancado, L., Reisel, J. and Walker, C., 2018. Impacts of a summer bridge program in
engineering on student retention and graduation. Journal of STEM Education, 19(2), pp. 34
Chiyaka, E.T., Sithole, A., Manyanga, F., McCarthy, P. and Bucklein, B.K., 2016.
Institutional Characteristics and Student Retention: What Integrated Postsecondary Education
Data Reveals about Online Learning. Online Journal of Distance Learning
Administration, 19(2), pp. 34-69.
Dagley, M., Georgiopoulos, M., Reece, A. and Young, C., 2016. Increasing retention and
graduation rates through a STEM learning community. Journal of College Student Retention:
Research, Theory & Practice, 18(2), pp.167-182.
Mancini, M.E., Cipher, D.J. and Ganji, D., 2018. Maximizing Retention and Progression to
Graduation in Online Programs: A Case Study in “Designing With the End in Mind”.
In Critical Assessment and Strategies for Increased Student Retention, pp. 211-225.
Millea, M., Wills, R., Elder, A. and Molina, D., 2018. What Matters in College Student
Success? Determinants of College Retention and Graduation Rates. Education, 138(4),
pp.309-322.
Queener, J.E. and Ford, B.A., 2019. Culturally Responsive Mentoring Programs: Impacting
Retention/Graduation Rates of African American Males Attending Predominately White
Institutions. In Overcoming Challenges and Creating Opportunity for African American Male
Students,pp. 120-132
Stout, R., Archie, C., Cross, D. and Carman, C.A., 2018. The relationship between faculty
diversity and graduation rates in higher education. Intercultural Education, 29(3), pp.399-
417..
Tchamdjeu, R.M., Steenkamp, H. and Nel, A.L., 2018, April. Retention based on graduation
rate of male and female industrial engineering students. In Global Engineering Education
Conference (EDUCON), 2018 IEEE (pp. 738-745). IEEE.

9
Wolf, P.S., David, A., Butler-Barnes, S.T. and Zile-Tamsen, V., 2017. American
Indian/Alaskan Native College Dropout: Recommendations for Increasing Retention and
Graduation. Journal on Race, Inequality, and Social Mobility in America, 1(1), pp.12-36.
Wolf, P.S., David, A., Butler-Barnes, S.T. and Zile-Tamsen, V., 2017. American
Indian/Alaskan Native College Dropout: Recommendations for Increasing Retention and
Graduation. Journal on Race, Inequality, and Social Mobility in America, 1(1), pp.12-36.
1 out of 9
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





