Pricing Zero-Coupon Bonds and Forward Contracts
VerifiedAdded on 2023/01/20
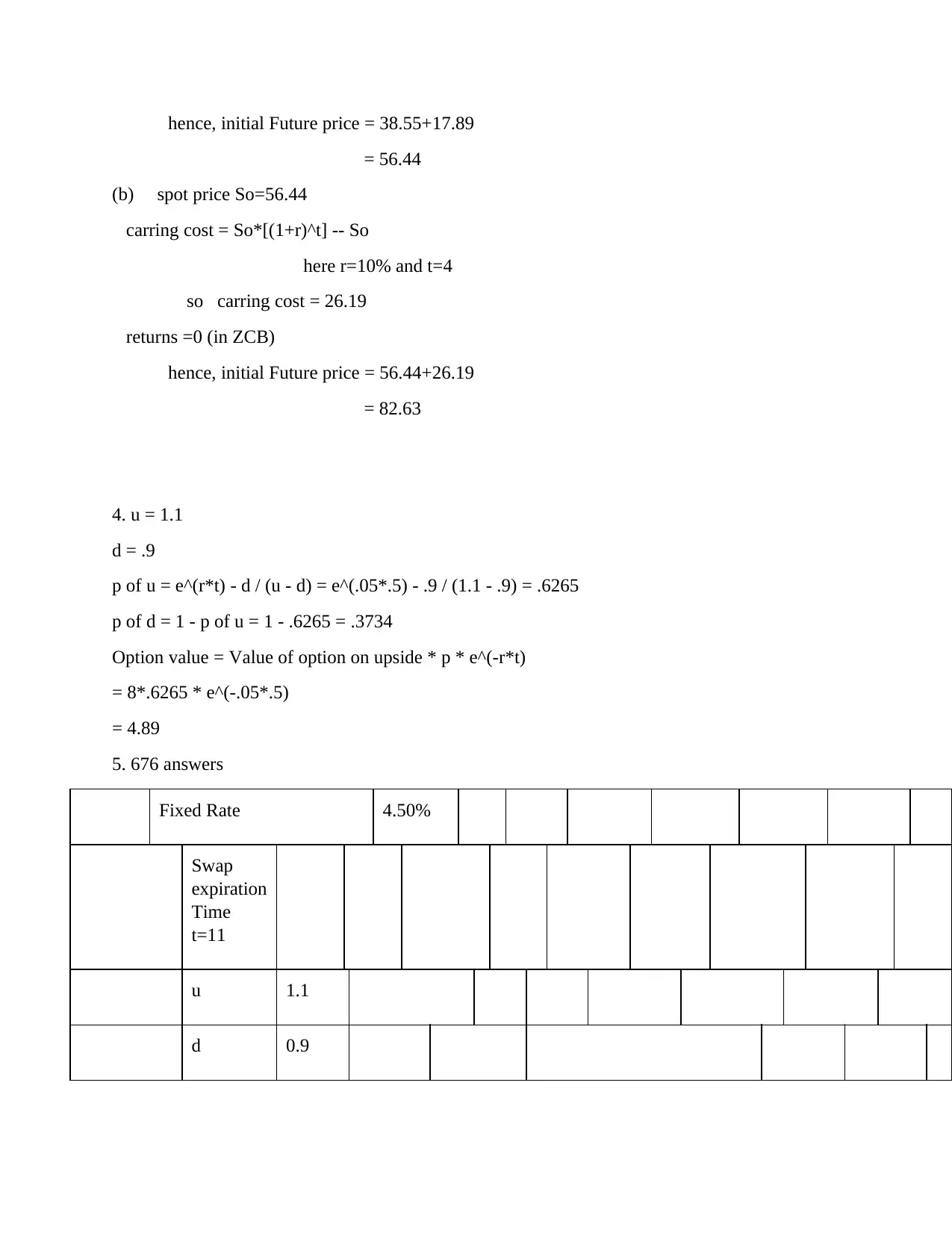
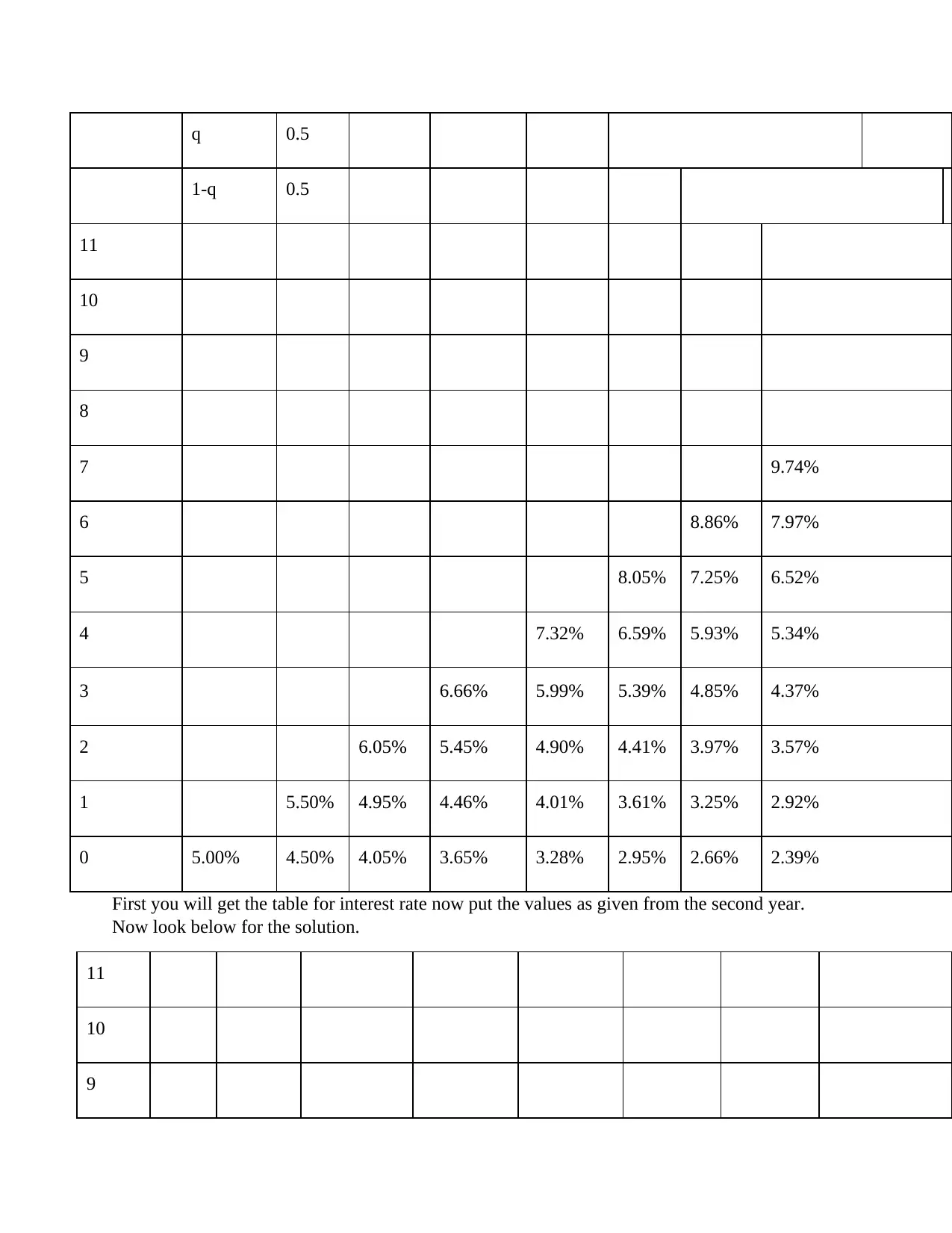
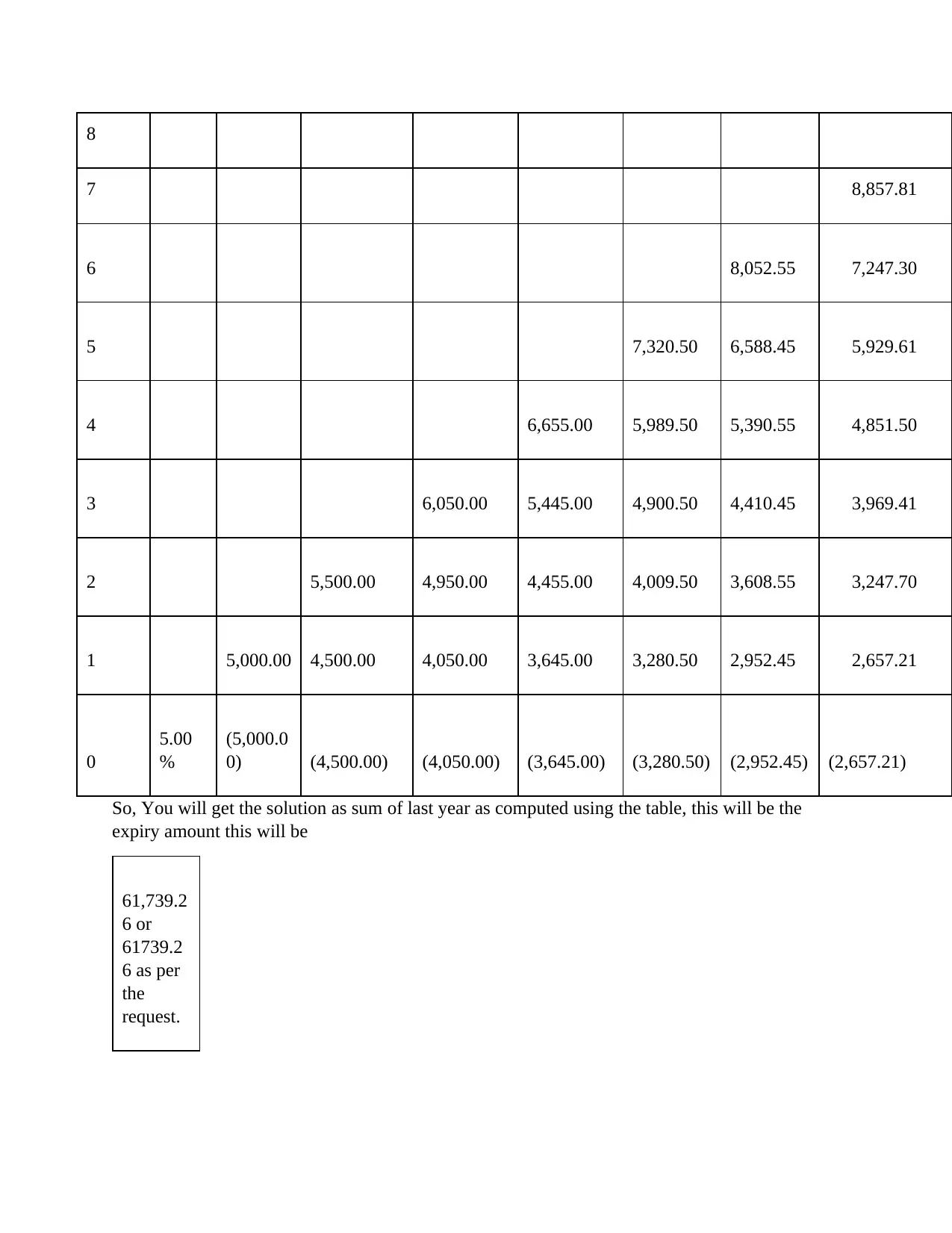
|6
|1097
|27
AI Summary
This document explains the process of pricing zero-coupon bonds and forward contracts using interest rates and time to maturity. It provides step-by-step calculations and formulas for determining the prices of these financial instruments. The document also includes references for further reading on the topic.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
1 out of 6






![[object Object]](/_next/static/media/star-bottom.7253800d.svg)