Desklib Study Material Solutions

Desklib is an online library that provides a wide range of solved assignments, essays, dissertations and study material. The library offers solutions for various document types and assignment types. Get access to high-quality study material for different subjects, courses and colleges/universities.

1 Question 1.
Apply complex arithmetic rule :(a + bi) (c + di) = (ac − bd) + (ad + bc) i
(8 − 9i)(2 − 4i) + (5 − 6i) = (8 · 2 − (−9) · (−4)) + (8 · (−4) + (−9) · 2)i + 5 − 6i
= (16 − 36) + (−50)i + 5 − 6i
= −15 − 56i
2 Question 2.
Apply complex arithmetic rule :a+bi
c+di = (c−di)(a+bi)
(c−di)(c+di) = (ac+bd)+(bc−ad)i
c2 +d2
3 −√ 5i
2 +√ 3i = 3 · 2 + −√ 5 √ 3 + − √ 5 · 2 − 3
√ 3 i
22 + √ 3 2
= 6 −√ 15
7 + −2
√ 5 − 3
√ 3
7 i
3 Question 3.
For a maximum or a minimum f’(x)=0.
d
dx(−2x2 + 4x + 7) = 0
−4x + 4 = 0
x = 1
At this value of x=1,d2
dx2 f (x) = −4 < 0 and hence this gives a maximum
value of f(1)=−2(1)2 + 4(1) + 7 or f(1)=9.
4 Question 4.
5x5y−4 2 = 5x5
y4
2
Apply exponent rule:
a
b
c
= ac
bc we get :
5x5y−4 2 = 5x5 2
(y4)2
= 25x10
y8
1

5 Question 5.
According to the graph the domain ofthe function is [-3,3) ∪[4, ∞) and the
range is [−9, ∞).
6 Question 6.
Subtracting elements in matching positions :

4 7 4
9 9 6
6 9 7

 −


−9 5 7
3 2 4
9 −1 4

 =


4 − (−9) 7 − 5 4 − 7
9 − 3 9 − 2 6 − 4
6 − 9 9 − (−1) 7 − 4



 4 7 4
9 9 6
6 9 7

 −

 −9 5 7
3 2 4
9 −1 4

 =

 13 2 −3
6 7 2
−3 10 3


7 Question 7.
There is no question of continuity at x=3 since the function is not defined at
x=3.
8 Question 8.
By average rate of change we calculate∆f(x)
∆x , where ∆x = (14 − 5) = 9,and
∆f (x) = f (14) − f (5) = −702 hence average rate of change is−702
9 or -78.
9 Question 9.
For the function f ◦ g(x),we need
√ x − 6 6= 0 also for square root we need
x − 6 ≥ 0. Hence we need x − 6 > 0 or x > 6.Hence our Domain is (6, ∞).
And our function f ◦ g(x) =−3√ x−6 , x > 6.
10 Question 10.
The critical points i.e the points where the derivatives are zero are x=0,1,2.5
The point x=0 is a local maxima, x=1 is an inflection point and x=2.5 is a
local minima.
2

11 Question 11.
p {x − 11} −
p {x} = −1
=⇒ p {x − 11} = −1 +
p {x}
=⇒ p {x − 11}
2
= −1 +p {x} 2
=⇒ x − 11 = 1 − 2
p {x} + x
=⇒ (−12)2 = −2p {x} 2
=⇒ 4x= 144
=⇒ x = 36
12 Question 12.
The possible number ofzeros are 7 including repeated roots.The possible
number ofturning points are 6 including repeated points.x7 + 7x6 + 10x5 :
x5 (x + 2) (x + 5) = 0 x = 0, −2, −5.
13 Question 13.
In order to obtain a function with real coefficients with the given roots we need
a function which has an additionalroot with a solution which is the complex
conjugate of -5 -3i.Hence our required function is
x + 4 +
√ 3 x + 4 −
√ 3 (x + 5 + 3i) (x + 5 − 3i) or
x4 + 18x3 + 127x2 + 402x + 442
14 Question 14.
− 3
y − 8+ − 2
−5 − y= −10y + 5
y2 − 3y − 40
Factoringy2 − 3y − 40 as (y + 5)(y − 8)
=⇒ − 3
y − 8+ 2
y + 5= −10y + 5
(y − 8)(y + 5)
Multiplying (y-8)(y+5) on both sides.
=⇒ −3(y + 5) + 2(y − 8) = −10y + 5
=⇒ y = 4
15 Question 15.
Since the value depreciates by 15% every year, the value after n years is 245000 ·
(1 − 0.15)n . So after 4 years, it is 245000 · (0.85)4 or $ 127891.53.
3

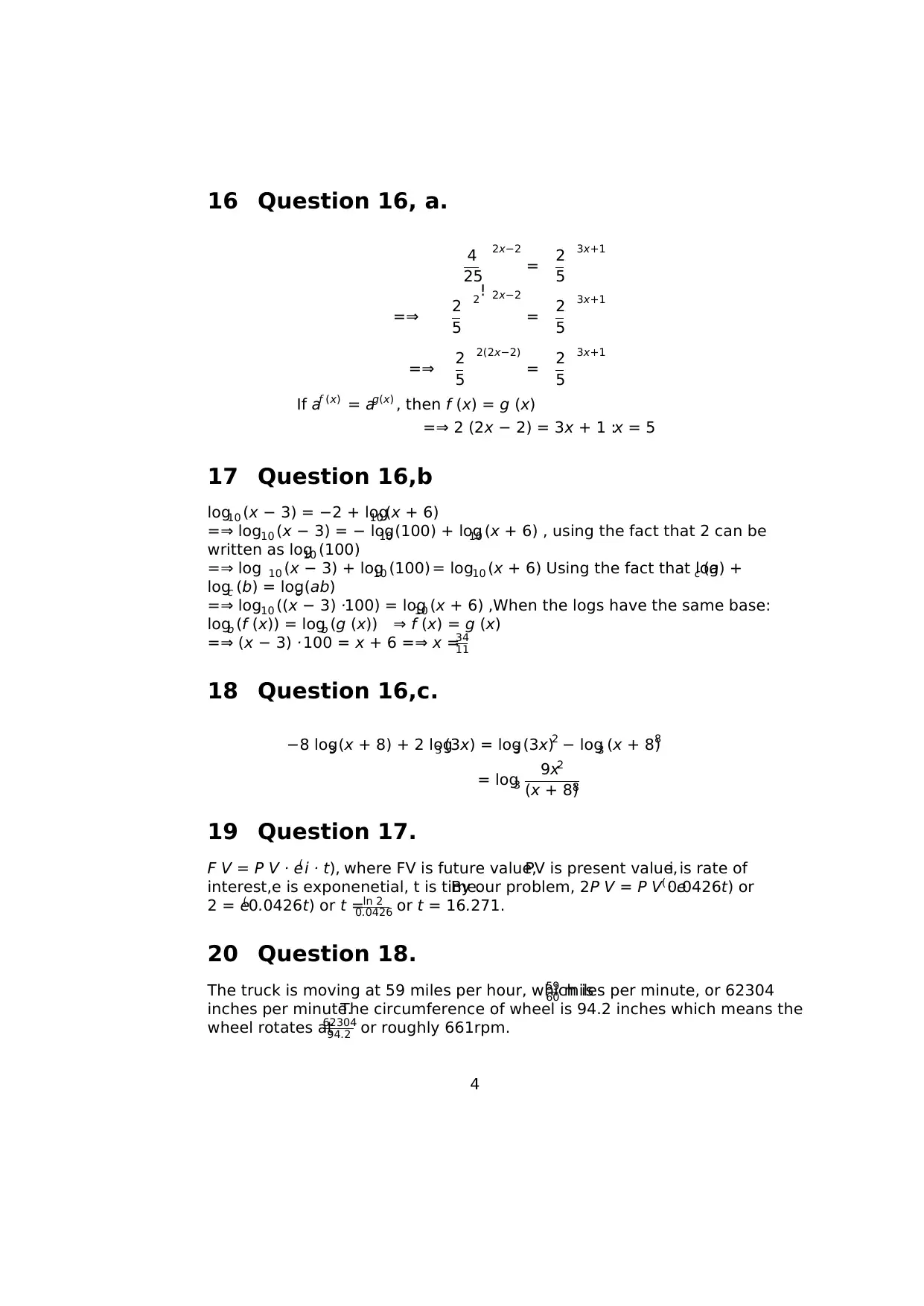
16 Question 16, a.
4
25
2x−2
= 2
5
3x+1
=⇒ 2
5
2! 2x−2
= 2
5
3x+1
=⇒ 2
5
2(2x−2)
= 2
5
3x+1
If af (x) = ag(x) , then f (x) = g (x)
=⇒ 2 (2x − 2) = 3x + 1 :x = 5
17 Question 16,b
log10 (x − 3) = −2 + log10 (x + 6)
=⇒ log10 (x − 3) = − log10 (100) + log10 (x + 6) , using the fact that 2 can be
written as log10 (100)
=⇒ log 10 (x − 3) + log10 (100) = log10 (x + 6) Using the fact that logc (a) +
logc (b) = logc (ab)
=⇒ log10 ((x − 3) ·100) = log10 (x + 6) ,When the logs have the same base:
logb (f (x)) = logb (g (x)) ⇒ f (x) = g (x)
=⇒ (x − 3) ·100 = x + 6 =⇒ x =34
11
18 Question 16,c.
−8 log3 (x + 8) + 2 log3 (3x) = log3 (3x)2 − log3 (x + 8)
8
= log3
9x2
(x + 8)8
19 Question 17.
F V = P V · e( i · t), where FV is future value,PV is present value,i is rate of
interest,e is exponenetial, t is time.By our problem, 2P V = P V · e( 0.0426t) or
2 = e( 0.0426t) or t =ln 2
0.0426 or t = 16.271.
20 Question 18.
The truck is moving at 59 miles per hour, which is59
60 miles per minute, or 62304
inches per minute.The circumference of wheel is 94.2 inches which means the
wheel rotates at62304
94.2 or roughly 661rpm.
4

21 Question 19.
If y = A cos(B(x + C)) + D
Amplitude Is A Period is 2π/B Phase shift is C (positive is to the left)
Vertical shift is D.
So our required function is y = 3 cos1
3 (x + 9π
2 ) − 4
22 Question 20,a.
tan2 (x) sec2 (x) + 2 sec2 (x) − tan2 (x) = 2
tan2 (x) sec2 (x) + 2 sec2 (x) − tan2 (x) − 2 = 0
sec2 (x) tan2 (x) + 2 sec2 (x) + − tan2 (x) − 2 = 0
− tan2 (x) + 2 + sec2 (x) tan2 (x) + 2 = 0
tan2 (x) + 2 sec2 (x) − 1 = 0
tan2 (x) + 2 (sec (x) + 1) (sec (x) − 1) = 0
Now tan2 (x) + 2 = 0 has no solutions since tan (x) =
√ 2i, tan (x) = −
√ 2i
has no solutions.
Now sec (x) = −1 gives x = π + 2πn
and sec (x) = 1 gives x = 2πn.
23 Question 20,b.
cos (x + π) − sin (x − π) = 0
− cos (x) − (− sin (x)) = 0
tan (x) = 1
x = π
4 + πn
5