SAS Similarity Test

This article explains the SAS similarity test for triangles, which states that two triangles are similar if two sides in one triangle are in the same proportion to corresponding sides in the other, and the included angles are equal. It provides examples and step-by-step explanations of how to apply the SAS test to determine triangle similarity.

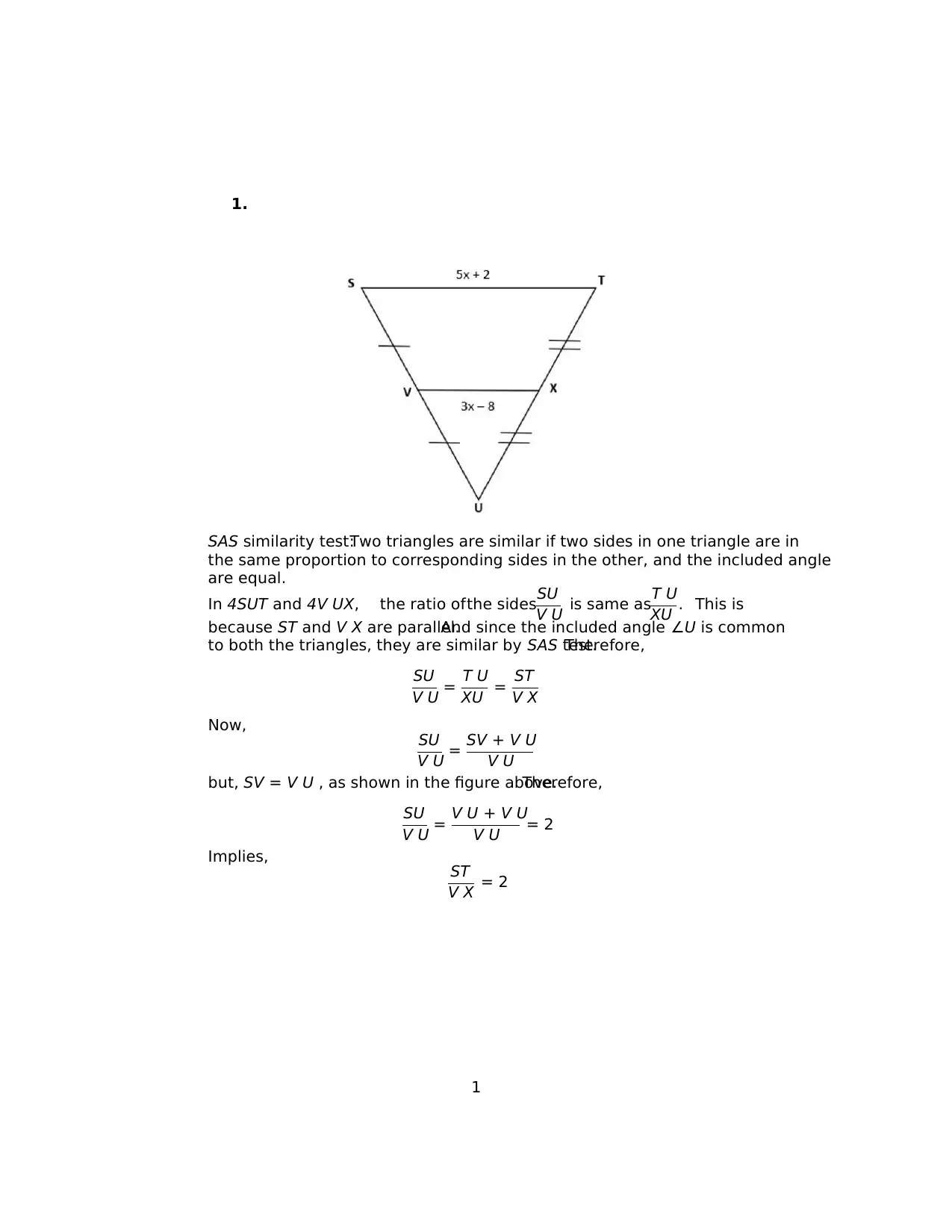
1.
SAS similarity test:Two triangles are similar if two sides in one triangle are in
the same proportion to corresponding sides in the other, and the included angle
are equal.
In 4SUT and 4V UX, the ratio ofthe sidesSU
V U is same asT U
XU . This is
because ST and V X are parallel.And since the included angle ∠U is common
to both the triangles, they are similar by SAS test.Therefore,
SU
V U = T U
XU = ST
V X
Now, SU
V U = SV + V U
V U
but, SV = V U , as shown in the figure above.Therefore,
SU
V U = V U + V U
V U = 2
Implies, ST
V X = 2
1

or,
5x + 2
3x − 8= 2
∴ 5x + 2 = 2(3x − 8)
=⇒ 5x − 6x = −16 − 2
=⇒ x = 18
¯V X = 3x − 8 = 3(18) − 8 = 46
Therefore, length of mid-segment¯V X is 46.
2.
In right-4:
sin (∠θ) =
side opposite to ∠θ
hypotenuse
4GP K and 4KP H are right angle triangles.Hypotenuse is the side opposite
to 90◦. Therefore, ¯KP is the hypotenuse for both the triangles.
In right-4GP K:
sin (∠GP K) =GK
KP
2

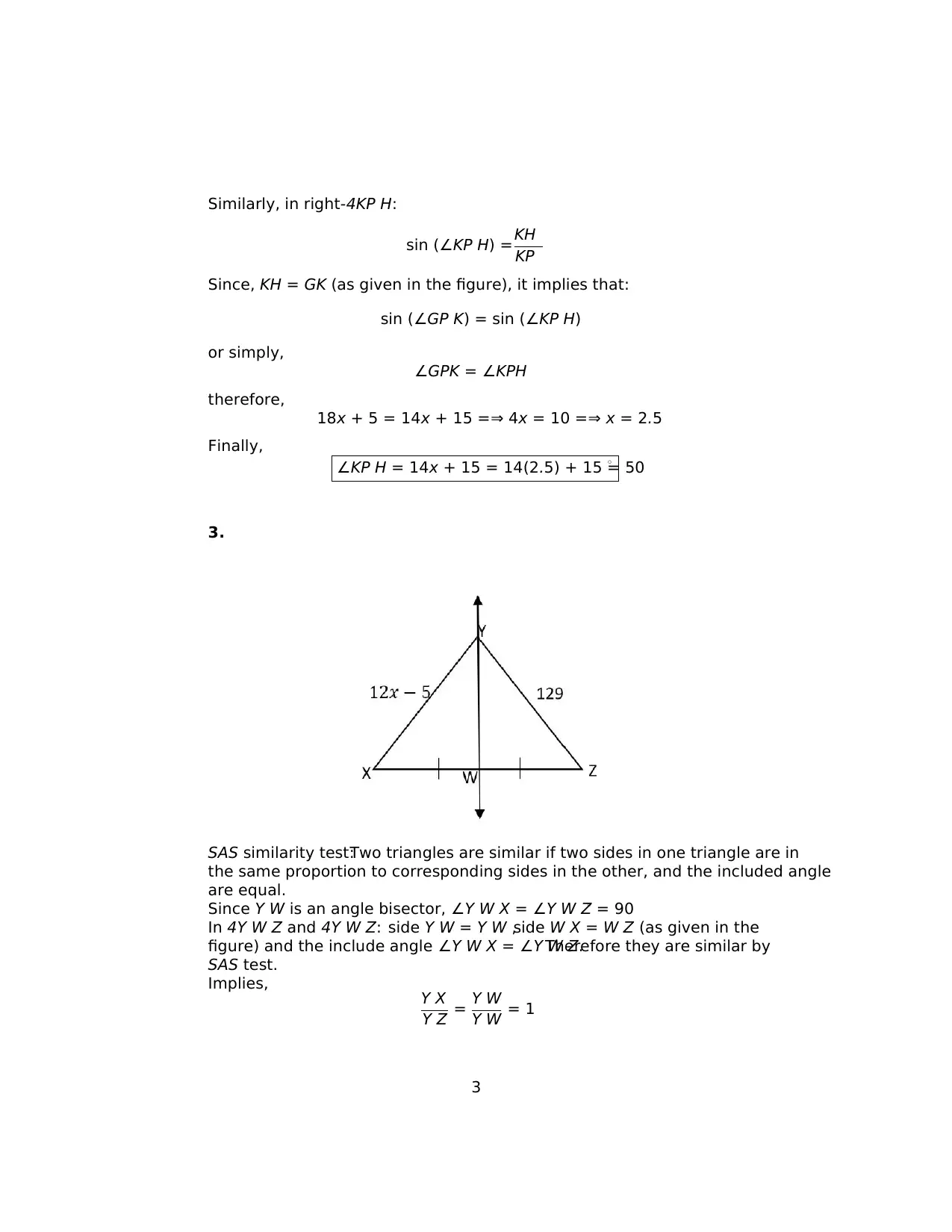
Similarly, in right-4KP H:
sin (∠KP H) =KH
KP
Since, KH = GK (as given in the figure), it implies that:
sin (∠GP K) = sin (∠KP H)
or simply,
∠GPK = ∠KPH
therefore,
18x + 5 = 14x + 15 =⇒ 4x = 10 =⇒ x = 2.5
Finally,
∠KP H = 14x + 15 = 14(2.5) + 15 = 50◦
3.
SAS similarity test:Two triangles are similar if two sides in one triangle are in
the same proportion to corresponding sides in the other, and the included angle
are equal.
Since Y W is an angle bisector, ∠Y W X = ∠Y W Z = 90◦
In 4Y W Z and 4Y W Z: side Y W = Y W ,side W X = W Z (as given in the
figure) and the include angle ∠Y W X = ∠Y W Z.Therefore they are similar by
SAS test.
Implies, Y X
Y Z = Y W
Y W = 1
3

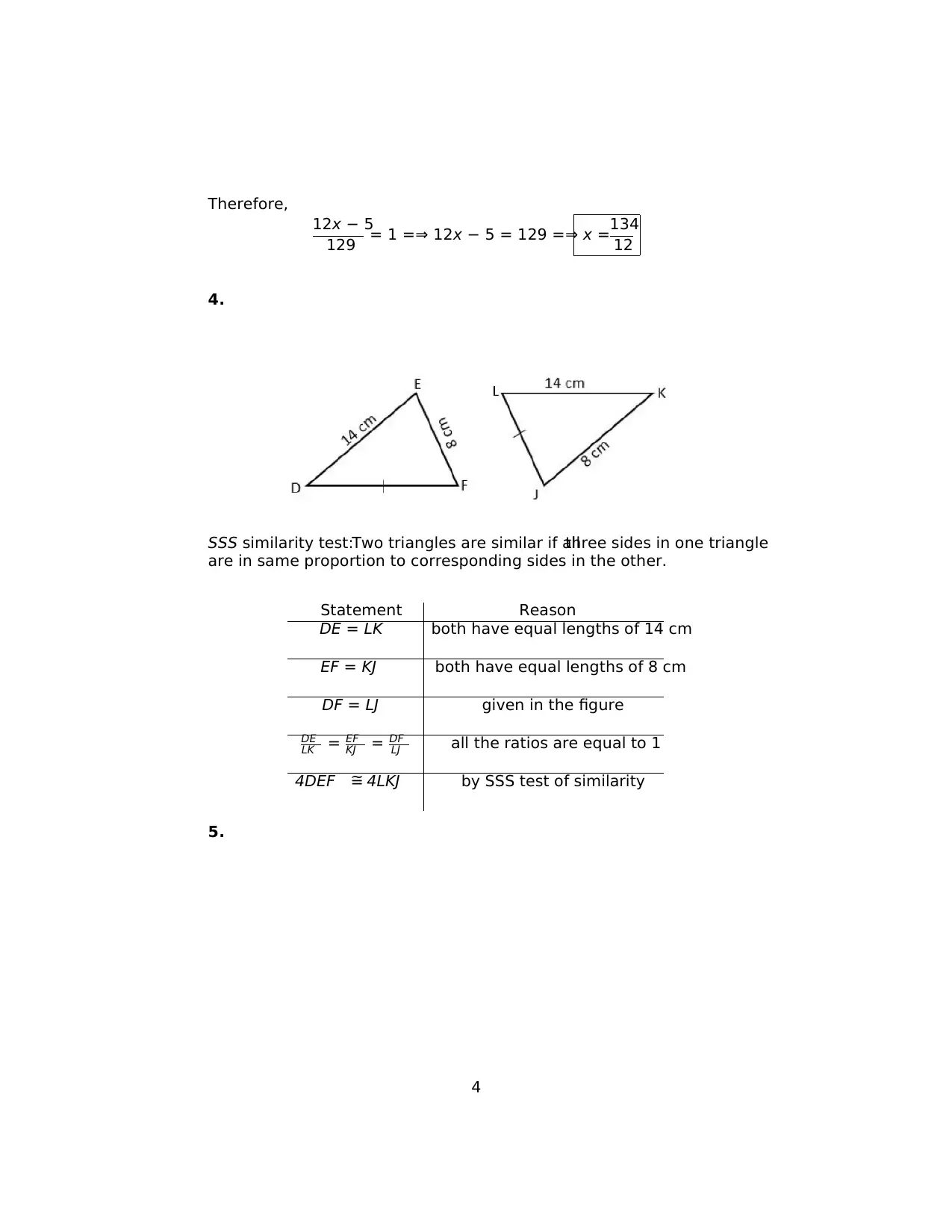
Therefore,
12x − 5
129 = 1 =⇒ 12x − 5 = 129 =⇒ x =134
12
4.
SSS similarity test:Two triangles are similar if allthree sides in one triangle
are in same proportion to corresponding sides in the other.
Statement Reason
DE = LK both have equal lengths of 14 cm
EF = KJ both have equal lengths of 8 cm
DF = LJ given in the figure
DE
LK = EF
KJ = DF
LJ all the ratios are equal to 1
4DEF ∼= 4LKJ by SSS test of similarity
5.
4

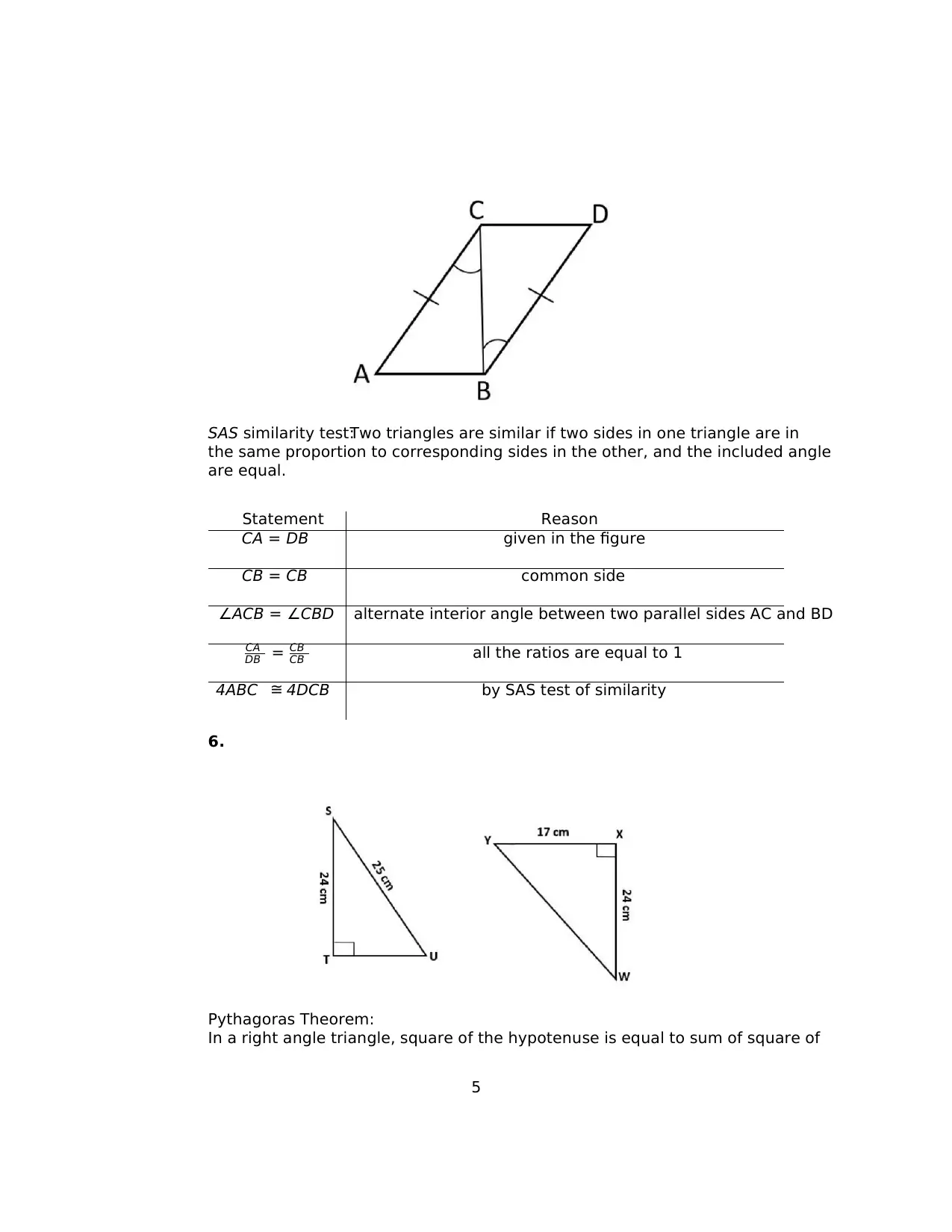
SAS similarity test:Two triangles are similar if two sides in one triangle are in
the same proportion to corresponding sides in the other, and the included angle
are equal.
Statement Reason
CA = DB given in the figure
CB = CB common side
∠ACB = ∠CBD alternate interior angle between two parallel sides AC and BD
CA
DB = CB
CB all the ratios are equal to 1
4ABC ∼= 4DCB by SAS test of similarity
6.
Pythagoras Theorem:
In a right angle triangle, square of the hypotenuse is equal to sum of square of
5

the other two sides.
4ST U and 4W XY are right angle triangles.Hypotenuse is the side oppo-
site to 90◦ angle.Therefore,SU and Y W are the hypotenuse for 4ST U and
4W XY respectively.
In 4ST U , by Pythagoras Theorem we have:
SU 2 = ST2 + T U2 =⇒ TU 2 = SU2 − ST2 = 252 − 242
therefore,
T U =p 252 − 242 = √ 49 = 7
Now, ST
XW = 24
24 = 1 & T U
XY = 7
17
Since, ST
XW 6= T U
XY
the corresponding sides of the triangles are not in same proportion, so the two
triangles cannot be similar.
7.
E: an event occurs.n(E): number of favourable outcomes for E.
Probability of E = P (E) = n(E)
total number of possible outcomes
(a)
E: a student studies for 1 − 2 hours.n(E): 29 students study for 1 − 2 hours.
Total number of students = 130
Therefore,
Probability that a student studies for 1-2 hours =
29
130
(b)
E: a student studies for more than 3 hours and earn a 71−90.n(E): 18 students
study for more than 3 hours and earn a 71 − 90.Total number of students =
130
Therefore,
Probability that a student studies for more than 3 hours and earns 71-90 =
18
130= 9
65
(c)
Conditionalprobability (Lavalle):Probability that an event A occurs given
event B:
P (A|B) = P (A and B)
P (B)
6

A: Student scores 91 − 100.B: Student studies for 2 − 3 hours.
n(B) = 45, therefore, P (B) =45
130
n(A and B) = 22, therefore, P (A and B) =22
130
Therefore,
P (91 − 100 | 2 − 3 hours) =
22/130
45/130= 22
45
8.
F : reads a fiction.P (F ) = 0.45
NF : reads a non-fiction.P (N F ) = 0.28
F ∩ NF : reads fiction and non-fiction.P (F ∩ NF ) = 0.1
F ∪ NF : reads fiction or a non fiction.
Probability that the student reads a fiction or a non-fiction = P (F ∪ N F ) = P (F ) + P (N F ) − P (
= 0.45 + 0.28 − 0.1 = 0.63
9.
There are 52 cards in a deck.These cards are divided into 4 suits (hearts,
spades, diamonds, clubs), each with 13 cards.
Each suit has a number 2 card.So, there are 4 number 2 cards.
P (two) = 4
52 = 1
13
Each suit has 5 odd number cards (1,3,5,7,9).So, there are 20 odd number
cards in a deck.
P (odd) =20
52 = 5
13
Therefore,
Probability of selecting a two and then an odd = P (two)×P (odd) =
1
13× 5
13 = 5
169
Figure below illustrates the entire process of drawing the cards.
7

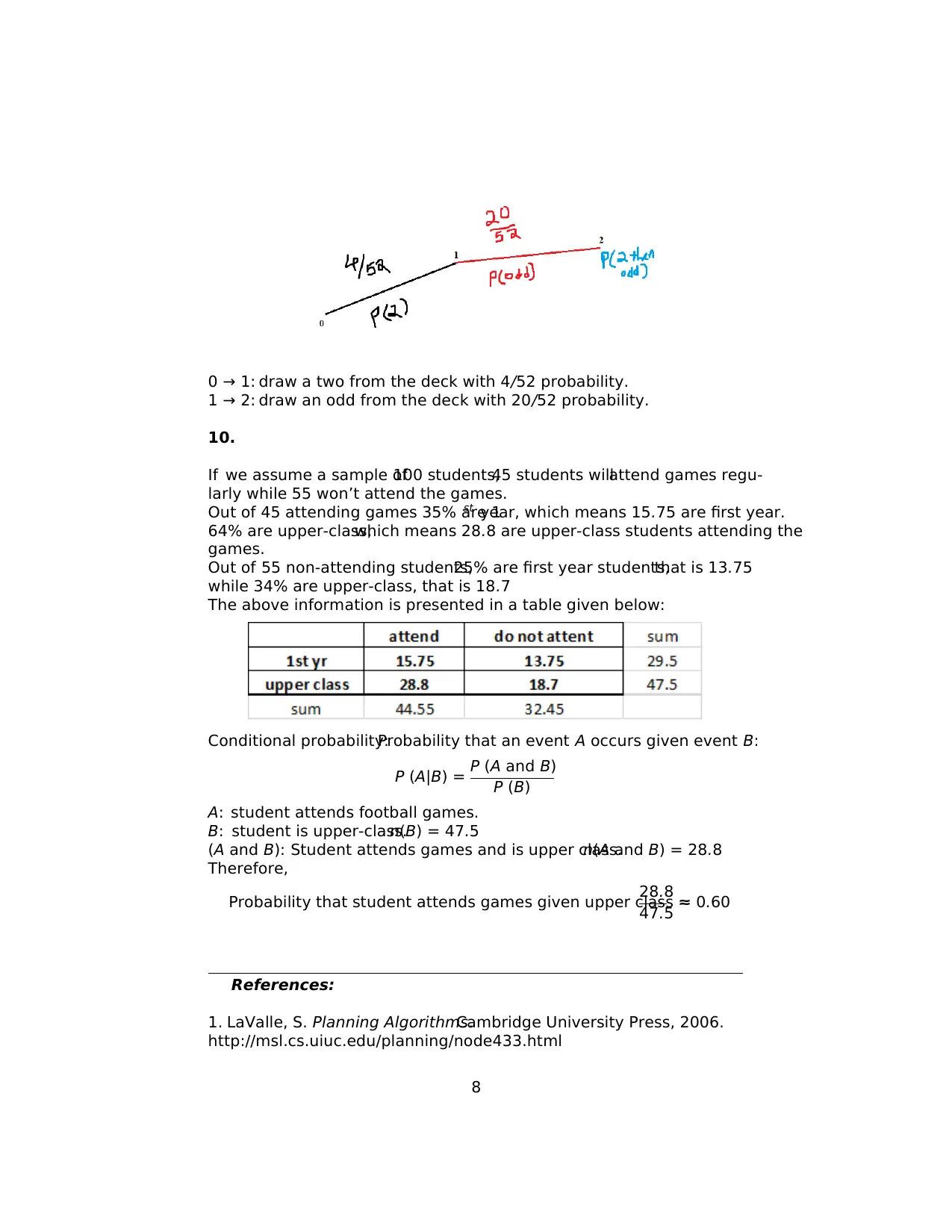
0 → 1: draw a two from the deck with 4/52 probability.
1 → 2: draw an odd from the deck with 20/52 probability.
10.
If we assume a sample of100 students,45 students willattend games regu-
larly while 55 won’t attend the games.
Out of 45 attending games 35% are 1st year, which means 15.75 are first year.
64% are upper-class,which means 28.8 are upper-class students attending the
games.
Out of 55 non-attending students,25% are first year students,that is 13.75
while 34% are upper-class, that is 18.7
The above information is presented in a table given below:
Conditional probability:Probability that an event A occurs given event B:
P (A|B) = P (A and B)
P (B)
A: student attends football games.
B: student is upper-class.n(B) = 47.5
(A and B): Student attends games and is upper class.n(A and B) = 28.8
Therefore,
Probability that student attends games given upper class =
28.8
47.5 ≈ 0.60
References:
1. LaValle, S. Planning Algorithms.Cambridge University Press, 2006.
http://msl.cs.uiuc.edu/planning/node433.html
8