University Complex Analysis Homework on Series and Functions

This document presents solutions to a complex analysis homework assignment. The solutions cover several key areas, including determining the radius of convergence for series, specifically using the ratio test. The assignment involves the manipulation and analysis of complex functions, including fi...Read More

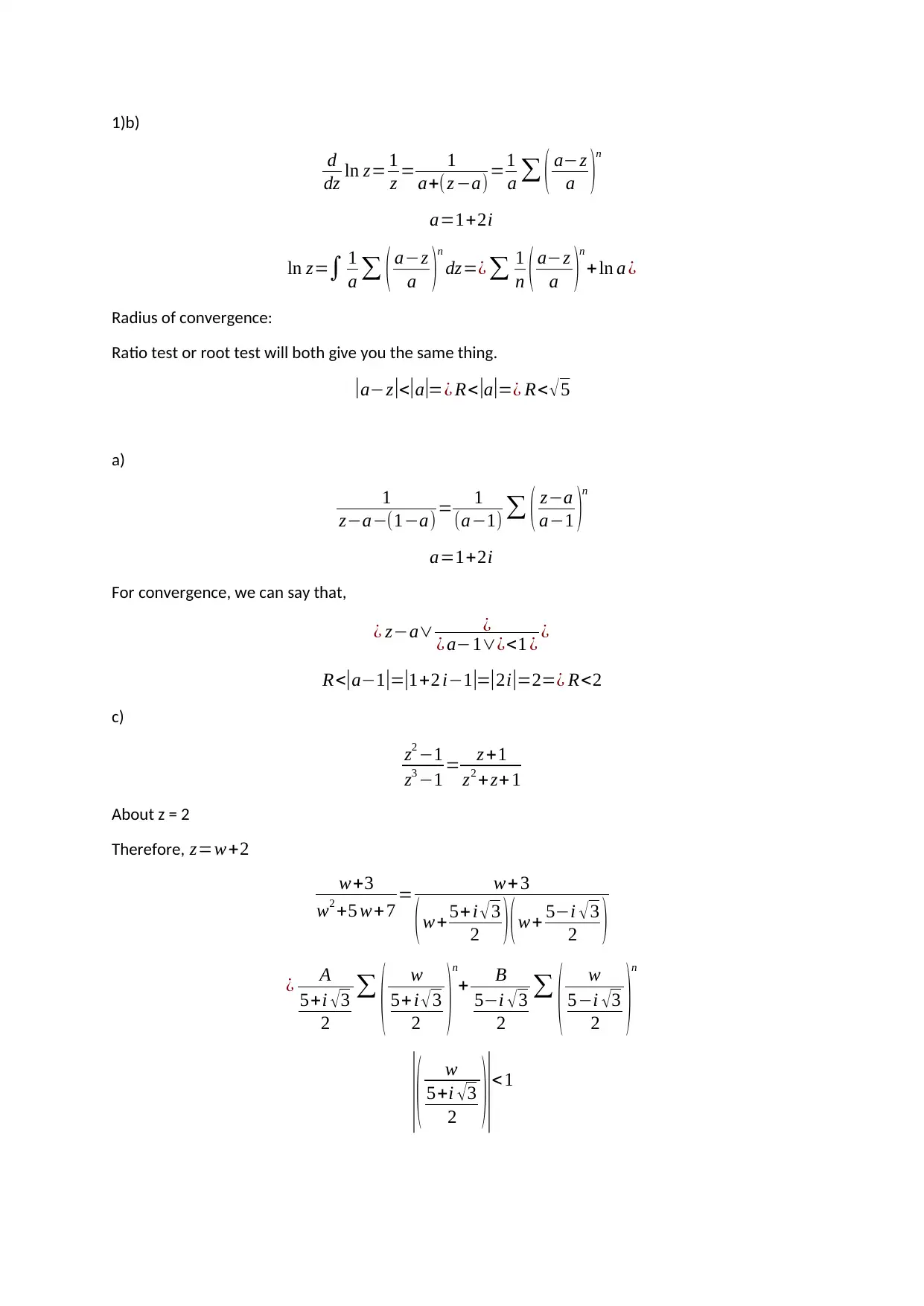
1)b)
d
dz ln z= 1
z = 1
a+(z −a)=1
a ∑ ( a−z
a )
n
a=1+2i
ln z=∫ 1
a ∑ ( a−z
a )
n
dz=¿ ∑ 1
n ( a−z
a )
n
+ln a ¿
Radius of convergence:
Ratio test or root test will both give you the same thing.
|a−z|<|a|=¿ R<|a|=¿ R< √ 5
a)
1
z−a−(1−a)= 1
(a−1) ∑ ( z−a
a−1 )n
a=1+2i
For convergence, we can say that,
¿ z−a∨ ¿
¿ a−1∨¿<1 ¿ ¿
R<|a−1|=|1+2 i−1|=|2i|=2=¿ R<2
c)
z2 −1
z3 −1 = z +1
z2 +z+ 1
About z = 2
Therefore, z=w+2
w+3
w2 +5 w+7 = w+3
( w+ 5+ i √ 3
2 )( w+ 5−i √ 3
2 )
¿ A
5+i √3
2
∑ ( w
5+ i √3
2 )n
+ B
5−i √3
2
∑ ( w
5−i √3
2 )n
|( w
5+i √3
2 )|<1

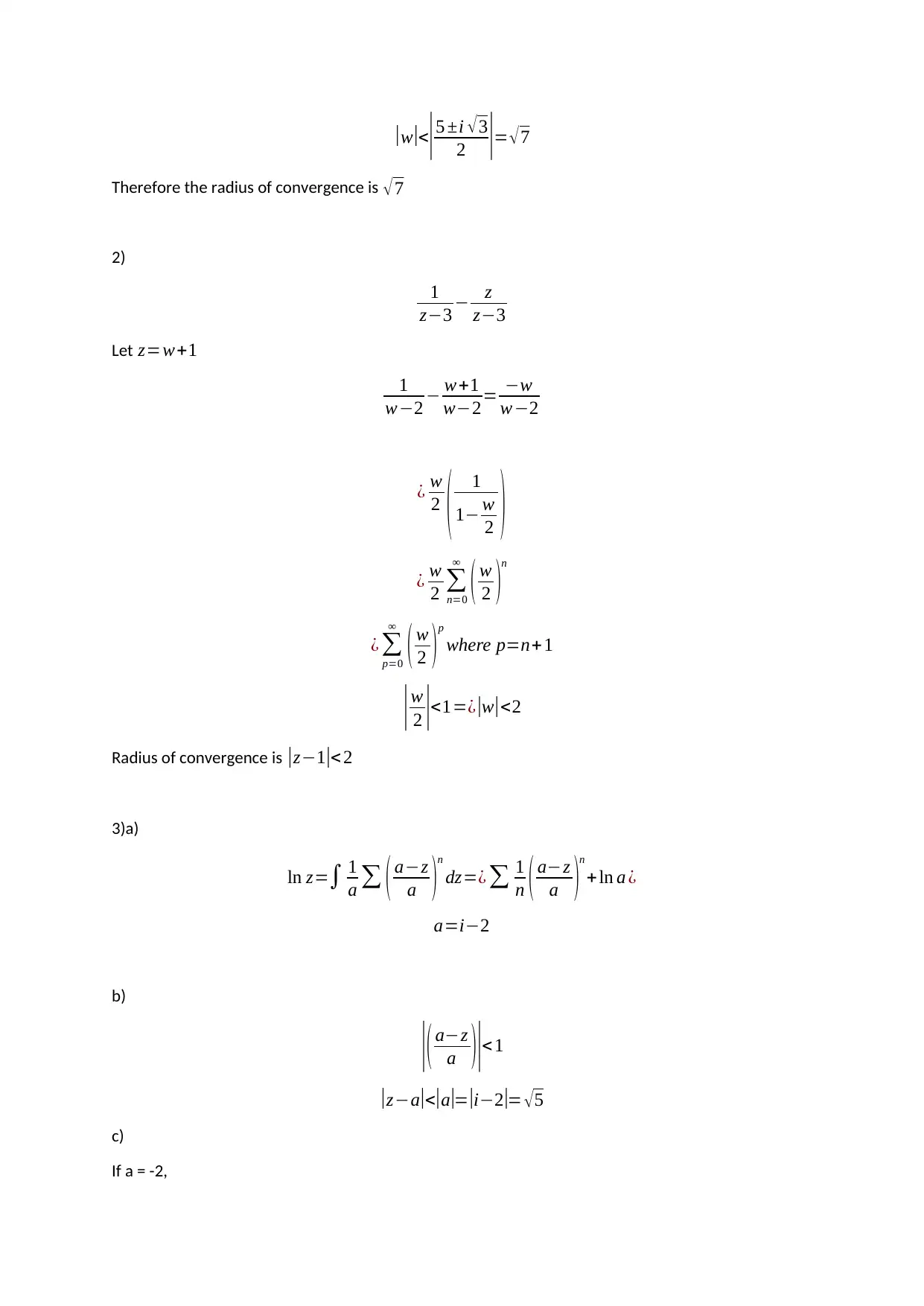
|w|<| 5 ±i √ 3
2 |= √ 7
Therefore the radius of convergence is √7
2)
1
z−3 − z
z−3
Let z=w+1
1
w−2 − w+1
w−2= −w
w−2
¿ w
2 ( 1
1− w
2 )
¿ w
2 ∑
n=0
∞
( w
2 )n
¿ ∑
p=0
∞
( w
2 )p
where p=n+1
|w
2 |<1=¿|w|<2
Radius of convergence is |z−1|<2
3)a)
ln z=∫ 1
a ∑ ( a−z
a )
n
dz=¿ ∑ 1
n ( a−z
a )
n
+ln a ¿
a=i−2
b)
|( a−z
a )|< 1
|z−a|<|a|=|i−2|= √5
c)
If a = -2,

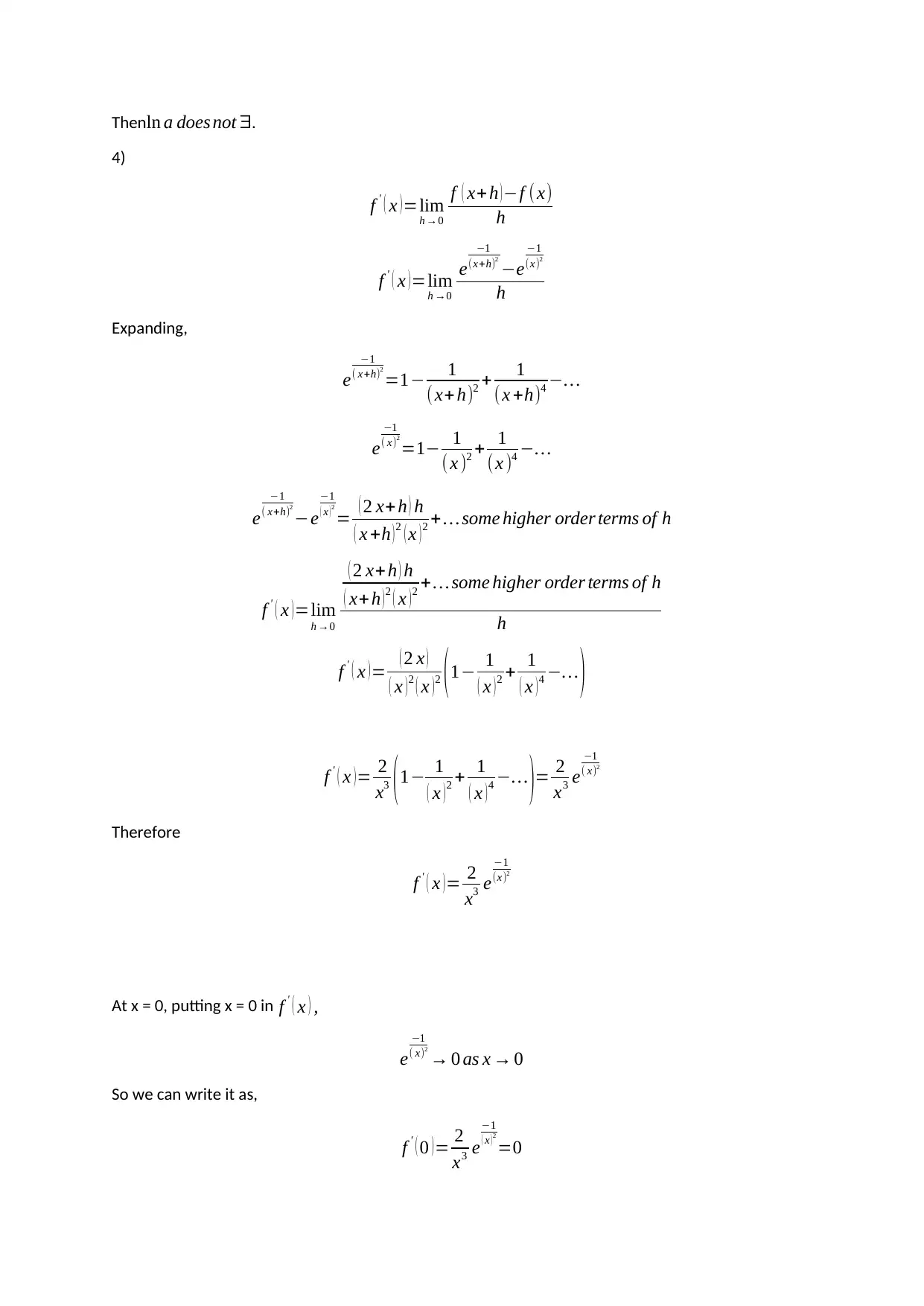
Thenln a does not ∃.
4)
f ' ( x ) =lim
h →0
f ( x+ h ) −f (x)
h
f ' ( x ) =lim
h →0
e
−1
(x+h)2
−e
−1
(x)2
h
Expanding,
e
−1
( x+h)2
=1− 1
(x+ h)2 + 1
(x +h)4 −…
e
−1
( x)2
=1− 1
( x )2 + 1
(x )4 −…
e
−1
( x+h)2
−e
−1
( x ) 2
= ( 2 x+ h ) h
( x +h ) 2 ( x ) 2 + … some higher order terms of h
f ' ( x )=lim
h →0
( 2 x+ h ) h
( x+ h )2 ( x )2 + … some higher order terms of h
h
f ' ( x ) = ( 2 x )
( x ) 2 ( x ) 2 ( 1− 1
( x ) 2 + 1
( x ) 4 −… )
f ' ( x )= 2
x3 (1− 1
( x )2 + 1
( x )4 −… )= 2
x3 e
−1
( x)2
Therefore
f ' ( x )= 2
x3 e
−1
(x)2
At x = 0, putting x = 0 in f ' ( x ) ,
e
−1
( x)2
→ 0 as x → 0
So we can write it as,
f ' ( 0 ) = 2
x3 e
−1
( x ) 2
=0

b)
With the help of induction we can show that higher order derivatives are also zero so,
f ' ( 0 )=f ' ' ( 0 )=f '' ' ( 0 )=…=0
Now if we find the Taylor series of f ( x) at 0,
f ( 0+ h ) =f ( 0 ) +h f ' ( 0 ) + h2
2 f ' ' ( 0 ) + h3
6 f ' ' ' ( 0 ) + …
Putting the values of the derivatives we get,
f ( 0+ h )=0
c)
lim
h→ 0
f ( 0+h ) −f ( 0 ) =lim
h→ 0
e
−1
h2
As h is imaginary so h2 will give a negative value so it will become -h2
lim
h→ 0
e
1
h2
=∞ ≠ f ( 0 )
Therefore it is not continuous proved.
d)
e
−1
z2
=1+−1
z2 + 1
2 ! z4 + −1
3 ! z6 + …
¿ ∑
n=0
∞ (−1)n
n !(z2 )n =∑
n=0
∞ 1
n ! ( −1
z2 )
n
It is valid in the domain of |z| > 0
5)
1
z2 −5 z +6 = 1
( z−3 ) ( z−2 ) = 1
z−3 − 1
z−2
If |Z|> 3
1
z−3 = 1
z ( 1
1−3 z−1 )=1
z ∑
n =0
∞
( 3
z )n
=∑
n=0
∞ 3n
zn +1

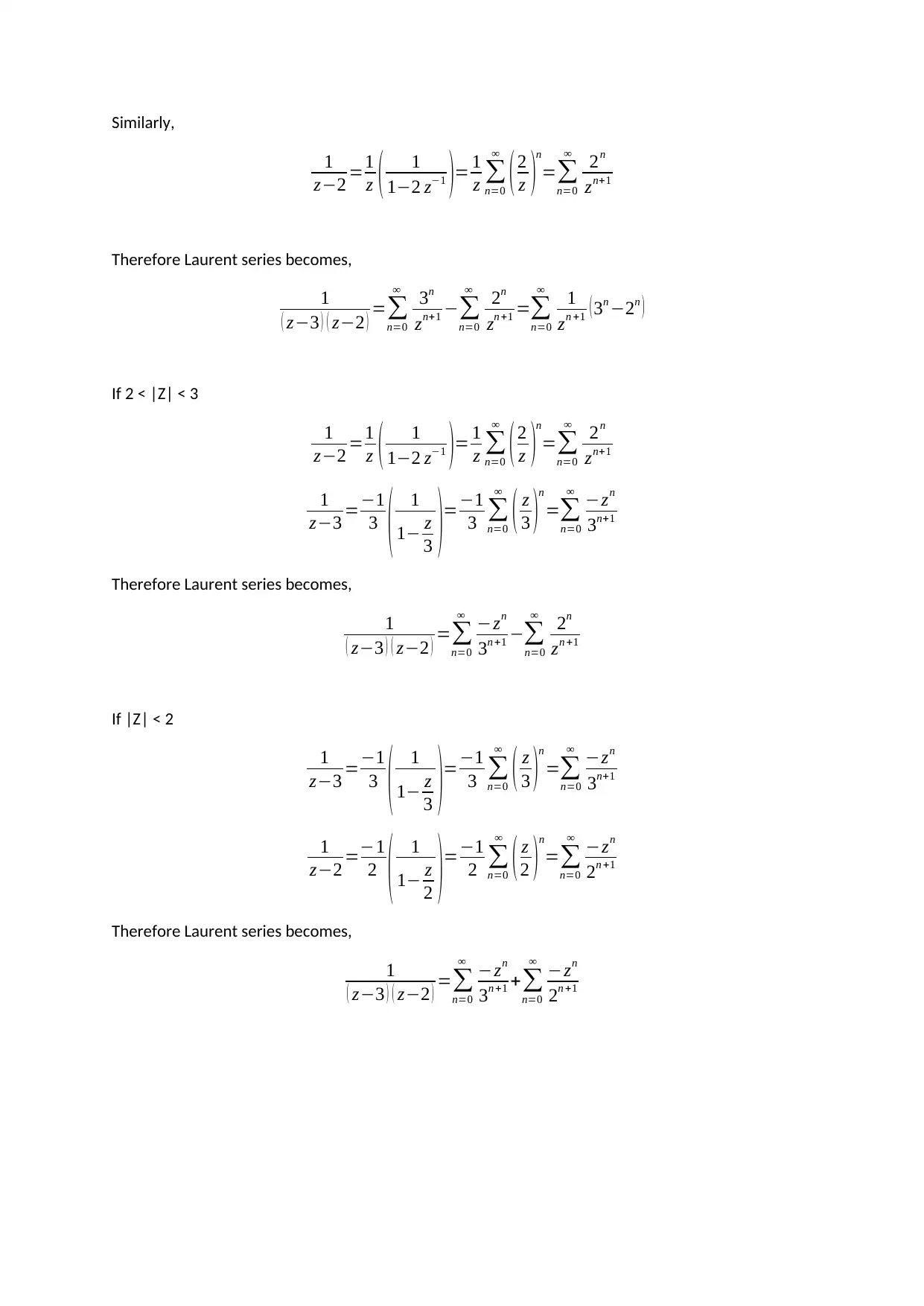
Similarly,
1
z−2 =1
z ( 1
1−2 z−1 )= 1
z ∑
n=0
∞
( 2
z )
n
=∑
n=0
∞ 2n
zn+1
Therefore Laurent series becomes,
1
( z−3 ) ( z−2 ) =∑
n=0
∞ 3n
zn+1 −∑
n=0
∞ 2n
zn +1 =∑
n=0
∞ 1
zn +1 ( 3n −2n )
If 2 < |Z| < 3
1
z−2 =1
z ( 1
1−2 z−1 )= 1
z ∑
n=0
∞
(2
z )n
=∑
n=0
∞ 2n
zn+1
1
z−3 =−1
3 ( 1
1− z
3 )=−1
3 ∑
n=0
∞
( z
3 )
n
=∑
n=0
∞ −zn
3n+1
Therefore Laurent series becomes,
1
( z−3 ) ( z−2 ) =∑
n=0
∞ −zn
3n +1 −∑
n=0
∞ 2n
zn +1
If |Z| < 2
1
z−3 =−1
3 ( 1
1− z
3 )=−1
3 ∑
n=0
∞
( z
3 )n
=∑
n=0
∞ −zn
3n+1
1
z−2 =−1
2 ( 1
1− z
2 )=−1
2 ∑
n=0
∞
( z
2 )n
=∑
n=0
∞ −zn
2n +1
Therefore Laurent series becomes,
1
( z−3 ) ( z−2 ) =∑
n=0
∞ −zn
3n +1 +∑
n=0
∞ −zn
2n +1