Math Assignment - Linear Algebra

This document is a solved math assignment on linear algebra. It includes questions on expressing vectors as linear combinations, finding numbers in matrix equations, computing matrices, and solving systems of equations.

3/22/2019 Math Assignment
Linear Algebra
[Student Name]
[STUDENT ID]

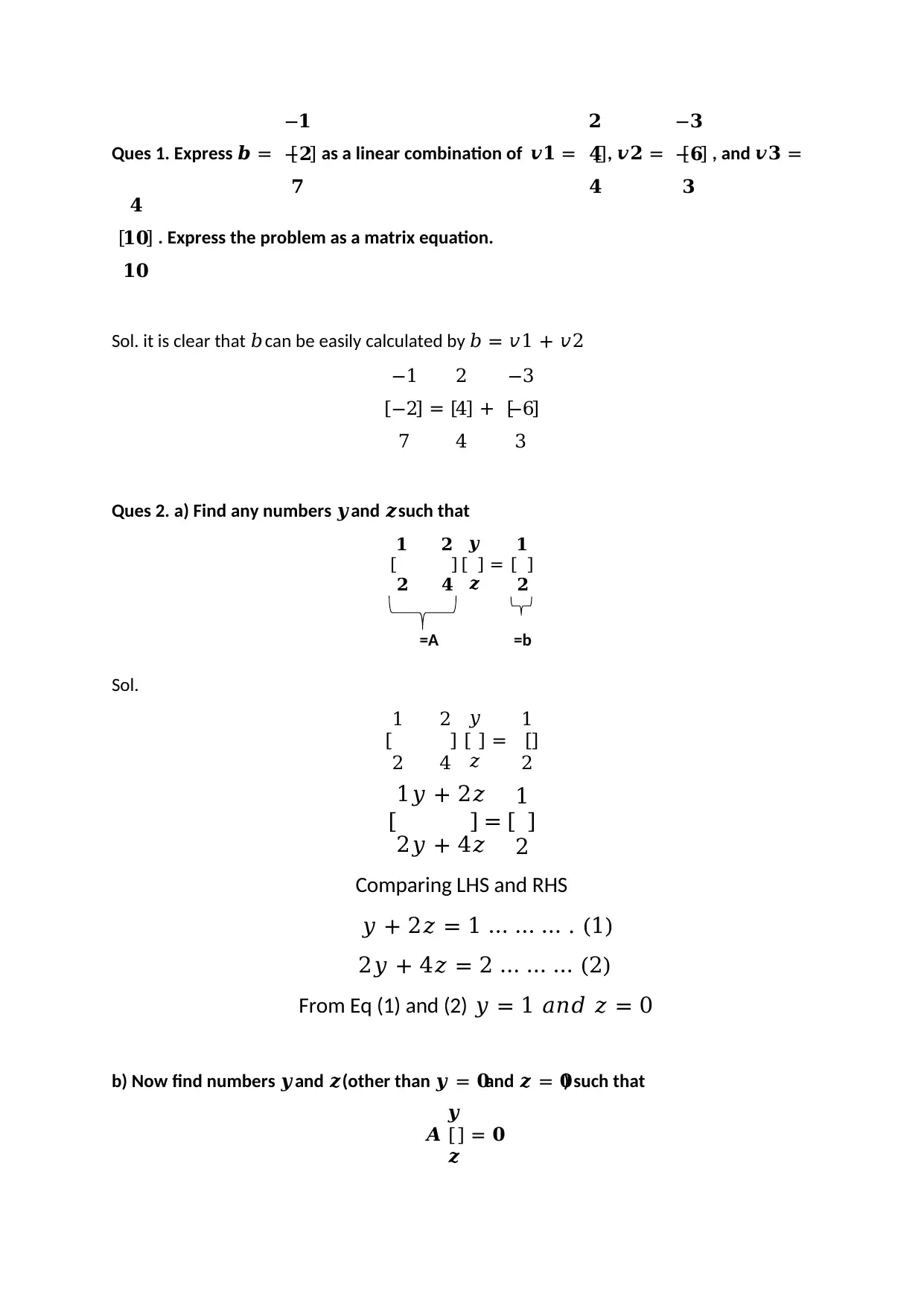
Ques 1. Express 𝒃 = [
−𝟏
−𝟐
𝟕
] as a linear combination of 𝒗𝟏 = [
𝟐
𝟒
𝟒
], 𝒗𝟐 = [
−𝟑
−𝟔
𝟑
] , and 𝒗𝟑 =
[
𝟒
𝟏𝟎
𝟏𝟎
] . Express the problem as a matrix equation.
Sol. it is clear that 𝑏 can be easily calculated by 𝑏 = 𝑣1 + 𝑣2
[
−1
−2
7
] = [
2
4
4
] + [
−3
−6
3
]
Ques 2. a) Find any numbers 𝒚and 𝒛such that
[
𝟏 𝟐
𝟐 𝟒
] [
𝒚
𝒛
] = [
𝟏
𝟐
]
Sol.
[
1 2
2 4
] [
𝑦
𝑧
] = [
1
2
]
[
1𝑦 + 2𝑧
2𝑦 + 4𝑧
] = [
1
2
]
Comparing LHS and RHS
𝑦 + 2𝑧 = 1 … … … . (1)
2𝑦 + 4𝑧 = 2 … … … (2)
From Eq (1) and (2) 𝑦 = 1 𝑎𝑛𝑑 𝑧 = 0
b) Now find numbers 𝒚and 𝒛(other than 𝒚 = 𝟎and 𝒛 = 𝟎) such that
𝑨 [
𝒚
𝒛
] = 𝟎
=A =b

Sol.
[
1 2
2 4
] [
𝑦
𝑧
] = 0
[
𝑦 + 2𝑧
2𝑦 + 4𝑧
] = 0
On comparing LHS and RHS
𝑦 = −2 𝑎𝑛𝑑 𝑧 = 1
c) If 𝑨 [
𝒚
𝒛
] = 𝟎, then explain why we must have
[
𝒚
𝒛
] = 𝜷 [
−𝟐
𝟏
]
for some number 𝜷.
Sol. From part (b) we get
[
𝒚
𝒛
] = [
−𝟐
𝟏
]
Hence for any number 𝛽, [
𝒚
𝒛
] = 𝜷 [
−𝟐
𝟏
] 𝑖𝑠 𝑡𝑟𝑢𝑒.
d) Take the numbers 𝒚and 𝒛from part (a). Without any calculation, explain why
𝑨 [
𝒚 + 𝟖
𝒛 − 𝟒
] = 𝒃
Sol.
𝐴 [
𝑦 + 8
𝑧 − 4
] = 𝑏
[1 2
2 4] [
𝑦 + 8
𝑧 − 4
] = [
1
2]
[1 2
2 4] [ 9
−4] = [
1
2]
Hence it is clear that LHS = RHS

Ques 3. a) Compute the 3 by 3 matrices 𝑴𝟏, 𝑴𝟐 𝑴𝟑where 𝑴𝟏 =
[
𝟏
𝟏
𝟏]
[𝟐 −𝟏 𝟑],
𝑴𝟐 =
[
𝟎
𝟏
−𝟑]
[𝟎 𝟐 −𝟏] and 𝑴𝟑 =
[
𝟎
𝟎
𝟏]
[𝟎 𝟎 𝟒].
𝑀1 = [
2 ∗ 1 1 ∗ (−1) 1 ∗ 3
1 ∗ 2 1 ∗ (−1) 1 ∗ 3
1 ∗ 2 1 ∗ (−1) 1 ∗ 3
]
𝑀1 = [
2 −1 3
2 −1 3
2 −1 3
]
𝑀2 = [
0 ∗ 0 0 ∗ 2 0 ∗ (−1)
1 ∗ 0 1 ∗ 2 1 ∗ (−1)
(−3) ∗ 0 (−3) ∗ 2 (−3) ∗ (−1)
]
𝑀2 = [
0 0 0
0 2 −1
0 −6 3
]
𝑀3 = [
0 ∗ 0 0 ∗ 0 0 ∗ 4
0 ∗ 0 0 ∗ 0 0 ∗ 4
1 ∗ 0 1 ∗ 0 1 ∗ 4
]
𝑀3 = [
0 0 0
0 0 0
0 0 4
]
b) Use Gaussian elimination and back substitution to solve 𝑨𝒙 = 𝒃, where
𝑨 = 𝑴𝟏 + 𝑴𝟐 + 𝑴𝟑and 𝒃 =
[
𝟏
𝟓
𝟏]
.
Sol.
𝐴 = 𝑀1 + 𝑀2 + 𝑀3
𝐴 = [
2 −1 3
2 −1 3
2 −1 3
] + [
0 0 0
0 2 −1
0 −6 3
] + [
0 0 0
0 0 0
0 0 4
]
𝐴 = [
2 −1 3
2 1 2
2 −7 10
]

𝑏 = [
1
5
1
]
𝐴𝑥 = 𝑏
[
2 −1 3
2 1 2
2 −7 10
] 𝑥 = [
1
5
1
]
𝑥 = 𝑏[𝐴]−1
[𝐴]−1 = 1
|𝐴| 𝐴𝑑𝑗[𝐴]
𝐴𝑑𝑗[𝐴] = (𝑀𝑎𝑡𝑟𝑖𝑥 𝑜𝑓 𝑐𝑜𝑓𝑎𝑐𝑡𝑜𝑟𝑠[𝐴])𝑇
𝐴𝑑𝑗[𝐴] = [
24 −16 −16
−11 14 16
−5 2 4
]
𝑇
𝐴𝑑𝑗[𝐴] = [
24 −11 −5
−16 14 2
−16 16 4
]
|𝐴| = 2 ∗(24) + 2 ∗(11) + 2 ∗ (−5)
|𝐴| = 48 + 22 − 10
|𝐴| = 60
[𝐴]−1 = 1
60[
24 −11 −5
−16 14 2
−16 16 4
]
𝑥 = [
1
5
1
] . 1
60[
24 −11 −5
−16 14 2
−16 16 4
]
𝑥 = 1
12[
1
1
1
] [
24 −11 −5
−16 14 2
−16 16 4
]
𝑥 = 1
12[
24 −11 −5
−16 14 2
−16 16 4
]
𝑥 =
[
2 −11
12
−5
12
−4
3
7
6
1
6
−4
3
4
3
1
3 ]