A Detailed Analysis of Macroeconomic Models and Solutions

This assignment provides a detailed analysis of macroeconomic models, focusing on Keynesian economics and related concepts. The solution explores utility functions, consumer problems, and constrained optimization, including the use of Lagrange multipliers. It examines the impact of governm...Read More

Solution 1
a) Let U =f ( x1 , x2) be utility function
Let V =f (x1 , x2) = F(U )
Where F’(U ) > 0 ( V is a monotonic transformation of U)
(i) We know that
MRS (x ¿¿ 1 , x2)¿ = −M U1
M U2
=−M V 1
M V 2
Hence ,
V1/V2 = U1/U2
(ii) i = 1and j = 2
V12/V21 = U12/U21
No, It is not necessary Vij and Uij always have the same sign .Because it will vary as per
constraint and utility function .
b ) Considering a standard Constrained utility maximization problem (i.e consumer’s problem) or cost
minimization problem (i.e firm’s problem)
(i) Optimization problem and corresponding Lagrangian :
Considering a consumer problem
max
x1 ,x2
u( x1 ¿, x2)¿
s.t p1 x1 + p2 x2=I
(ii) Writing a first order conditions

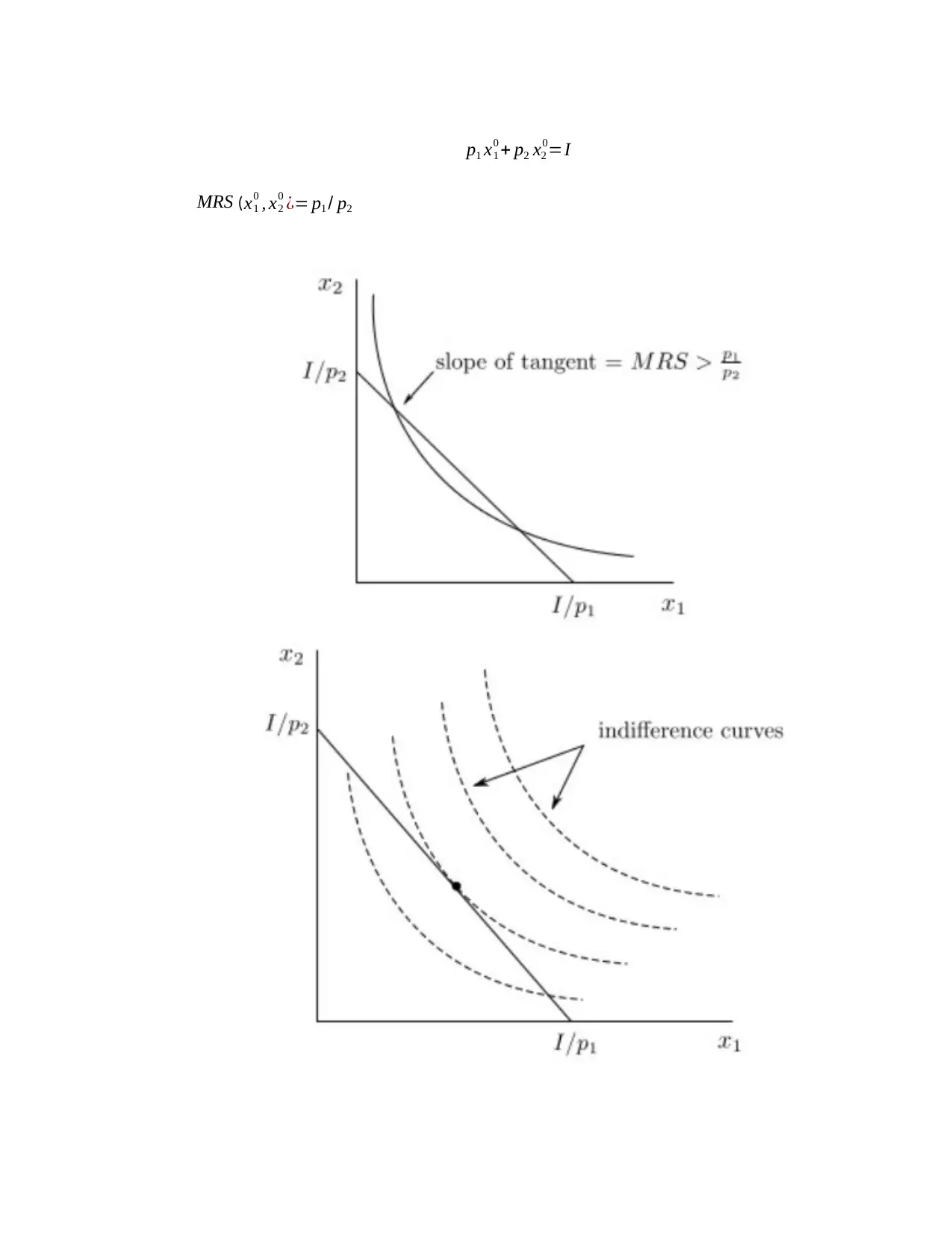
p1 x1
0 + p2 x2
0=I
MRS ( x1
0 , x2
0 ¿= p1 / p2

(iii) Lagrange Multiplier : It is basically applied to identify optimum situation and sometime
used to find out the maximum and minimum coordinates of the tangent points between
objective function .
(iv) Envelope theorem : This theorem says the direct effect of changes in exogenous variable
which is based considered , even though the exogenous variable might enter the maximum
value function indirectly as the part of solution to the endogenous choice variables.
The problem then becomes Maximize
U = f( x , y , α)
Subject to g( x , y , α)=0
The Lagrangian for this problem is Z = f(x, y, α) + λg(x, y, α)
Solution 4
According to Keynesian Macroeconomic model ,
Y =C + I+ G
C=200−0.8 Y
I =1000−2000r
G and r  exogenous variable
Y, C and I endogenous variable
(a) Set up of model in matrix form :
Y −C + I =G
−0.8 Y +C=200
I =1000−2000r
Thus ,
AX = B

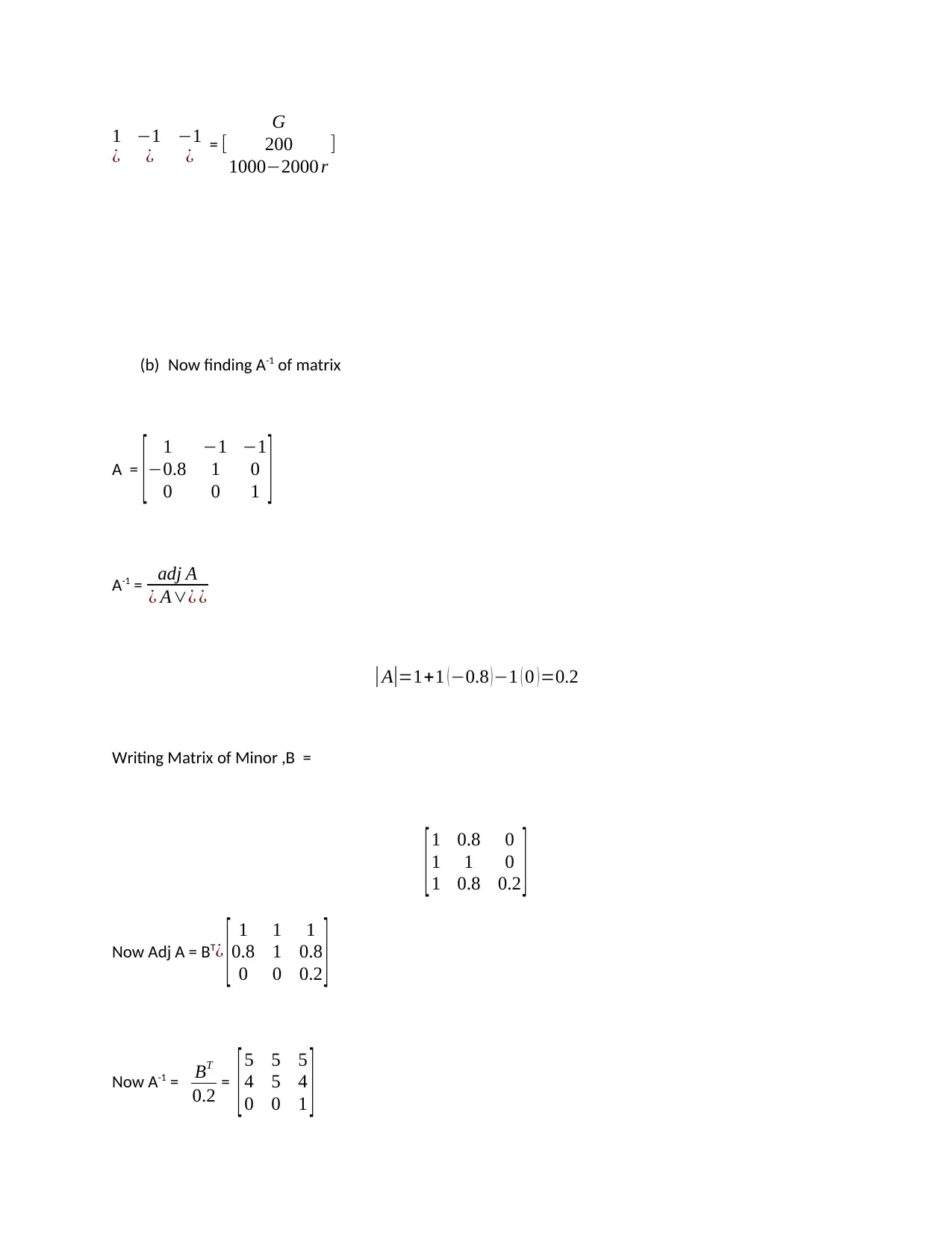
1 −1 −1
¿ ¿ ¿ = [
G
200
1000−2000 r
]
(b) Now finding A-1 of matrix
A = [ 1 −1 −1
−0.8 1 0
0 0 1 ]
A-1 = adj A
¿ A∨¿ ¿
| A|=1+1 ( −0.8 ) −1 ( 0 ) =0.2
Writing Matrix of Minor ,B =
[1 0.8 0
1 1 0
1 0.8 0.2 ]
Now Adj A = BT¿ [ 1 1 1
0.8 1 0.8
0 0 0.2 ]
Now A-1 = BT
0.2 = [ 5 5 5
4 5 4
0 0 1 ]

I = -2000r + 1000
C = 4G – 8000r + 5000
Y = 5G – 10000r + 6000
(c) Now G is decreasing by 50$ billon effect on Y
Multipliers = 1
1−MPC = 1
0.2 =5
So dY
dG =Multiplier=5
Thus dY = 5 dG
dG = - 50
d Y = (-50) x 5 = -250
Hence income is decreased by 250 $ Billon .
(d) Now considering the consumption depends upon the interest rate as well income.
Replacing the second equation :
Y −C + I =G
−0.8 Y +C−1000 r=200
Or
−0.8 Y +C=200+1000 r
I =1000−2000r
Formation of new matrix :

AX = B
1 −1 −1
¿ ¿ ¿ = [
G
200+1000 r
1000−2000 r
]
Applying the cramer’s rule ,
We got ,
Y =5 G−15,000 r+ 6000
(e) On comparing The income we got in b part and d part and indicated that economy will have to pay
more interest for borrowing in part C . Y will be less in Part C as compare to part b if interest is same .