Advanced Solid Mechanics: Stress Relaxation, Creep, Factors Affecting Creep, Fatigue Life Methods, Stress Concentration, Miner's Rule
VerifiedAdded on 2023/05/30
|19
|2327
|493
AI Summary
This article covers topics such as stress relaxation, creep, factors affecting creep, fatigue life methods, stress concentration, and Miner's rule in advanced solid mechanics.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Advanced Solid Mechanics 1
ADVANCE SOLID MECHANICS
By Name
Course
Instructor
Institution
Location
Date
ADVANCE SOLID MECHANICS
By Name
Course
Instructor
Institution
Location
Date
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Advanced Solid Mechanics 2
QUESTION ONE
Part 1 Stress relaxation
This is reduction in stress which can be observed with accordance to the quantity of deflection
produced in the structure. It is usually true since maintaining a material in a deformed position
for some period of time leads to plastic deformation1. Creep is an increased plastic deformation
in a constant force applied.
Fig 1 illustrate stress-strain graph for material under stress relaxation.
Fig 2 illustrates a rubber band model, the rubber is made to hang on a pin at step A as shown.
The band is then made to stretch in pin 2 as illustrated in fig 2. Pin 2 is abruptly withdrawn and
1 W. Bottega, Engineering Vibrations. Florida: CRC Press, 2013,p.123.
A. Culp, Principles of Energy Conversion. Hull: McGraw-Hill, 2011, p.541.
QUESTION ONE
Part 1 Stress relaxation
This is reduction in stress which can be observed with accordance to the quantity of deflection
produced in the structure. It is usually true since maintaining a material in a deformed position
for some period of time leads to plastic deformation1. Creep is an increased plastic deformation
in a constant force applied.
Fig 1 illustrate stress-strain graph for material under stress relaxation.
Fig 2 illustrates a rubber band model, the rubber is made to hang on a pin at step A as shown.
The band is then made to stretch in pin 2 as illustrated in fig 2. Pin 2 is abruptly withdrawn and
1 W. Bottega, Engineering Vibrations. Florida: CRC Press, 2013,p.123.
A. Culp, Principles of Energy Conversion. Hull: McGraw-Hill, 2011, p.541.

Advanced Solid Mechanics 3
the band gets its initial shape again as in C.
Fig b showing a rubber band example
Figure 3 illustrates the band after exposure. There is no conspicuous difference between step 3
and step 2 in the second diagram. But, the pin is withdrawn at step 4, and it becomes stable and
observable.
2. Creep rupture strength
This is the force needed to produce a creep fracture experiment in a given time span. It is as well
referred to as the stress which at a specified temperature can make a structure to rapture in a
specified time.
3. Creep life
Blends of materials employed in applications such as blocks experience a bigger
temperature range and stress for fluctuating time during, start up, shut down creep strain, landing
and take-off accrue at these stages and design could presume a worst case scenario, however, this
the band gets its initial shape again as in C.
Fig b showing a rubber band example
Figure 3 illustrates the band after exposure. There is no conspicuous difference between step 3
and step 2 in the second diagram. But, the pin is withdrawn at step 4, and it becomes stable and
observable.
2. Creep rupture strength
This is the force needed to produce a creep fracture experiment in a given time span. It is as well
referred to as the stress which at a specified temperature can make a structure to rapture in a
specified time.
3. Creep life
Blends of materials employed in applications such as blocks experience a bigger
temperature range and stress for fluctuating time during, start up, shut down creep strain, landing
and take-off accrue at these stages and design could presume a worst case scenario, however, this

Advanced Solid Mechanics 4
could result in a weight penalty2. This can also be illustrated by the fraction law which states that
the fraction of rupture equals to unity as shown in the following diagram;
∑ ¿¿+ t 2
tR 2 + t 3
tR 3 ….) = ∑ i ti
tR 1 = ∫ 1
tR dt = 1
4. Factors affecting creep
The below are main factors which affect creep;
i. Load applied
ii. Exposure temperature
iii. Property of material
iv. Time of exposure
2 L. Steinbeisser, Vibration Problems in Structures: Practical Guidelines. London: Birkhäuser, 2012,p.451.
F. Taylor, The Internal-combustion Engine in Theory and Practice: Thermodynamics, fluid flow,
performance. Stoke: MIT Press, 2014,p.431.
could result in a weight penalty2. This can also be illustrated by the fraction law which states that
the fraction of rupture equals to unity as shown in the following diagram;
∑ ¿¿+ t 2
tR 2 + t 3
tR 3 ….) = ∑ i ti
tR 1 = ∫ 1
tR dt = 1
4. Factors affecting creep
The below are main factors which affect creep;
i. Load applied
ii. Exposure temperature
iii. Property of material
iv. Time of exposure
2 L. Steinbeisser, Vibration Problems in Structures: Practical Guidelines. London: Birkhäuser, 2012,p.451.
F. Taylor, The Internal-combustion Engine in Theory and Practice: Thermodynamics, fluid flow,
performance. Stoke: MIT Press, 2014,p.431.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Advanced Solid Mechanics 5
QUESTION 2
QUESTION THREE
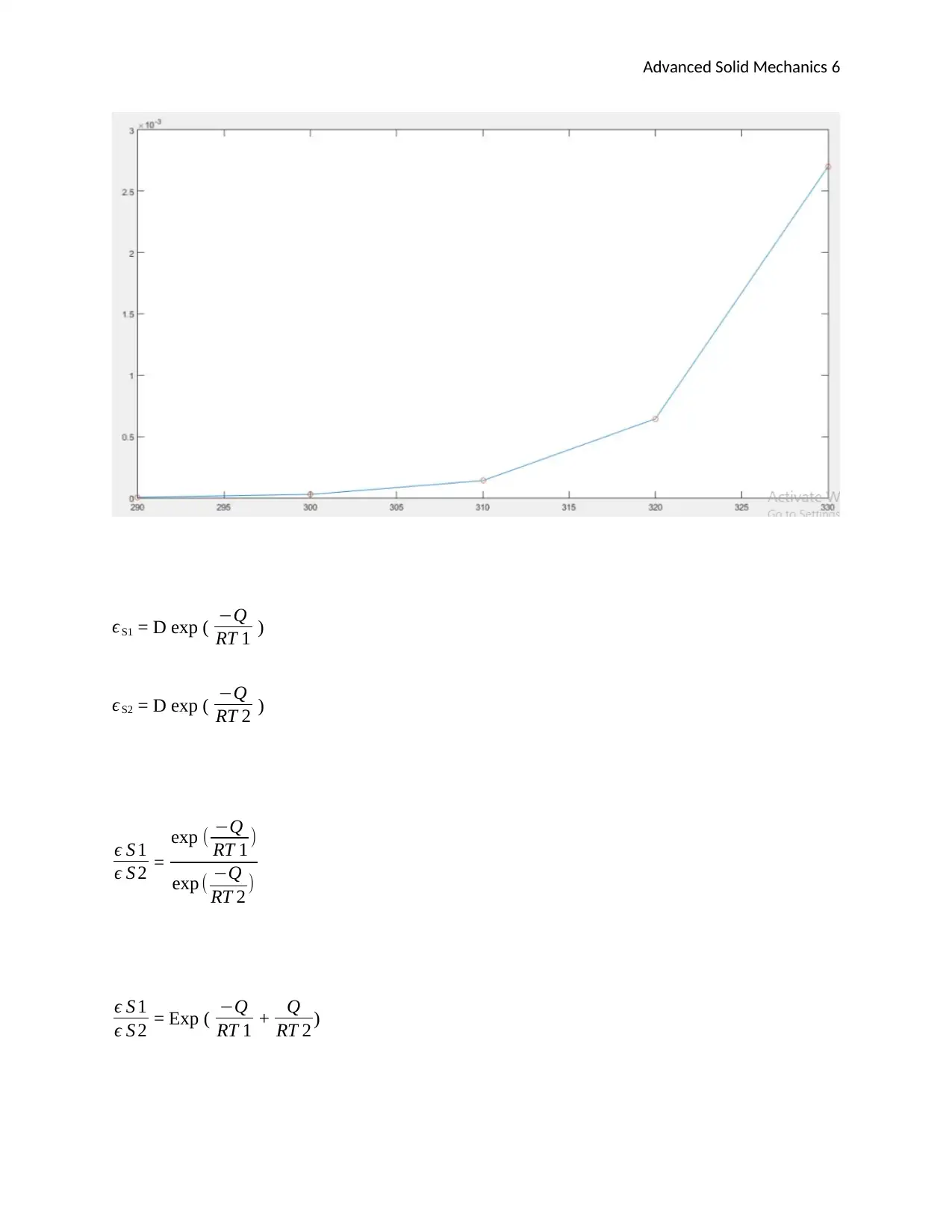
x=290:10:330
y=[4.8e-6 2.74e-5 1.4e-4 6.44e-4 2.7e-3]
p=polyfit(x,y,4)
f=polyval(p,x)
plot(x,y,x,f,'o')
QUESTION 2
QUESTION THREE
x=290:10:330
y=[4.8e-6 2.74e-5 1.4e-4 6.44e-4 2.7e-3]
p=polyfit(x,y,4)
f=polyval(p,x)
plot(x,y,x,f,'o')
Advanced Solid Mechanics 6
ϵ S1 = D exp ( −Q
RT 1 )
ϵ S2 = D exp ( −Q
RT 2 )
ϵ S 1
ϵ S 2 =
exp ( −Q
RT 1 )
exp ( −Q
RT 2 )
ϵ S 1
ϵ S 2 = Exp ( −Q
RT 1 + Q
RT 2 )
ϵ S1 = D exp ( −Q
RT 1 )
ϵ S2 = D exp ( −Q
RT 2 )
ϵ S 1
ϵ S 2 =
exp ( −Q
RT 1 )
exp ( −Q
RT 2 )
ϵ S 1
ϵ S 2 = Exp ( −Q
RT 1 + Q
RT 2 )

Advanced Solid Mechanics 7
Therefore Q ( 1
RT 2 - 1
RT 1 ) = ln ( ϵ S 1
ϵ S 2 )
Q= ln¿
Putting the values from the table given as T1=290 and T2= 300
Q= -35.142 kj/mole
4.8×10-6 = D exp (- Q
RT 1 ¿ ¿)
D=
4.8× 1 0−6
exp( −Q
RT 1 )
D= 2.245×10-12
QUESTION FOUR
Vessel Pressure (p) = 1.5 Mpa
D:a of the cover plate (D) = 450 mm
R= 225mm
Therefore Q ( 1
RT 2 - 1
RT 1 ) = ln ( ϵ S 1
ϵ S 2 )
Q= ln¿
Putting the values from the table given as T1=290 and T2= 300
Q= -35.142 kj/mole
4.8×10-6 = D exp (- Q
RT 1 ¿ ¿)
D=
4.8× 1 0−6
exp( −Q
RT 1 )
D= 2.245×10-12
QUESTION FOUR
Vessel Pressure (p) = 1.5 Mpa
D:a of the cover plate (D) = 450 mm
R= 225mm
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Advanced Solid Mechanics 8
Amount of bolts = 30
D:a of bolt (d) = 20mm
Elasticity E = 210×103 Mpa
Area A = π
4 (D2)
A = π
4 (450×450)
A= 159043.12 mm2
Secondary creep rate = 32.2 ×10-17 σ−4h-1
Part 1
Original tightening stress in the bolt n can be obtained by
σ = P D2
nd = 1.5× 450 × 450
30 ×20
σ = 303750
600
σ = 506.25Mpa
Part 2
Amount of bolts = 30
D:a of bolt (d) = 20mm
Elasticity E = 210×103 Mpa
Area A = π
4 (D2)
A = π
4 (450×450)
A= 159043.12 mm2
Secondary creep rate = 32.2 ×10-17 σ−4h-1
Part 1
Original tightening stress in the bolt n can be obtained by
σ = P D2
nd = 1.5× 450 × 450
30 ×20
σ = 303750
600
σ = 506.25Mpa
Part 2

Advanced Solid Mechanics 9
Time for tightening
R= Pr
t = 1.5× 225
t
506 = 1.5× 225
t
t= 337.5
506.25
t= 0.66 seconds
QUESTION FIVE
Part 1
i. Nominal stress-life (S-N) method
This employs nominal stress and it is linked to the local fatigue strength for unnotched and the
notched members.
Advantage of the method
This method has an advantage since it is grounded on the actual/real figures , the figures are published
in different international standards and they have been employed in all kinds of welded structures.
Limitations of the methods
i. The standard is workable to wrought steel working below the creep temperature of the
material.
ii. For this method the material will need low cycle methods since it doesn’t depend on the
steel grade employed in construction
iii. This method has less yield strength of just 102 ksi
Time for tightening
R= Pr
t = 1.5× 225
t
506 = 1.5× 225
t
t= 337.5
506.25
t= 0.66 seconds
QUESTION FIVE
Part 1
i. Nominal stress-life (S-N) method
This employs nominal stress and it is linked to the local fatigue strength for unnotched and the
notched members.
Advantage of the method
This method has an advantage since it is grounded on the actual/real figures , the figures are published
in different international standards and they have been employed in all kinds of welded structures.
Limitations of the methods
i. The standard is workable to wrought steel working below the creep temperature of the
material.
ii. For this method the material will need low cycle methods since it doesn’t depend on the
steel grade employed in construction
iii. This method has less yield strength of just 102 ksi

Advanced Solid Mechanics 10
ii. Local strain-life ( ε-N) method
This methods was formulated first around the year 1960, this method is a deformation at a notch
and is linked to a smooth deformation-managed behaviour fatigue.
Advantages
This method of fatigue life can be employed to obtain the local strain from nominal or global or
deformations.
Limitation
This method lacks real figures unlike the nominal stress-life (S-N) method
iii. Fatigue crack growth (da/dN-∆K) method
This fatigue life method was developed in the year 1960s . It was employed fracture mechanics
and structures of materials. It is viewed as a total fatigue life if it is employed in conjunction with
existing original size of crack following manufacture.
Advantages o of this methods
This method can be employed to get the total number of cycle to develop in crack from a specific
length to another length and fracture.
Limitations
This methods works best for only existing original crack size given just by the manufacturer.
ii. Local strain-life ( ε-N) method
This methods was formulated first around the year 1960, this method is a deformation at a notch
and is linked to a smooth deformation-managed behaviour fatigue.
Advantages
This method of fatigue life can be employed to obtain the local strain from nominal or global or
deformations.
Limitation
This method lacks real figures unlike the nominal stress-life (S-N) method
iii. Fatigue crack growth (da/dN-∆K) method
This fatigue life method was developed in the year 1960s . It was employed fracture mechanics
and structures of materials. It is viewed as a total fatigue life if it is employed in conjunction with
existing original size of crack following manufacture.
Advantages o of this methods
This method can be employed to get the total number of cycle to develop in crack from a specific
length to another length and fracture.
Limitations
This methods works best for only existing original crack size given just by the manufacturer.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Advanced Solid Mechanics 11
Part 2
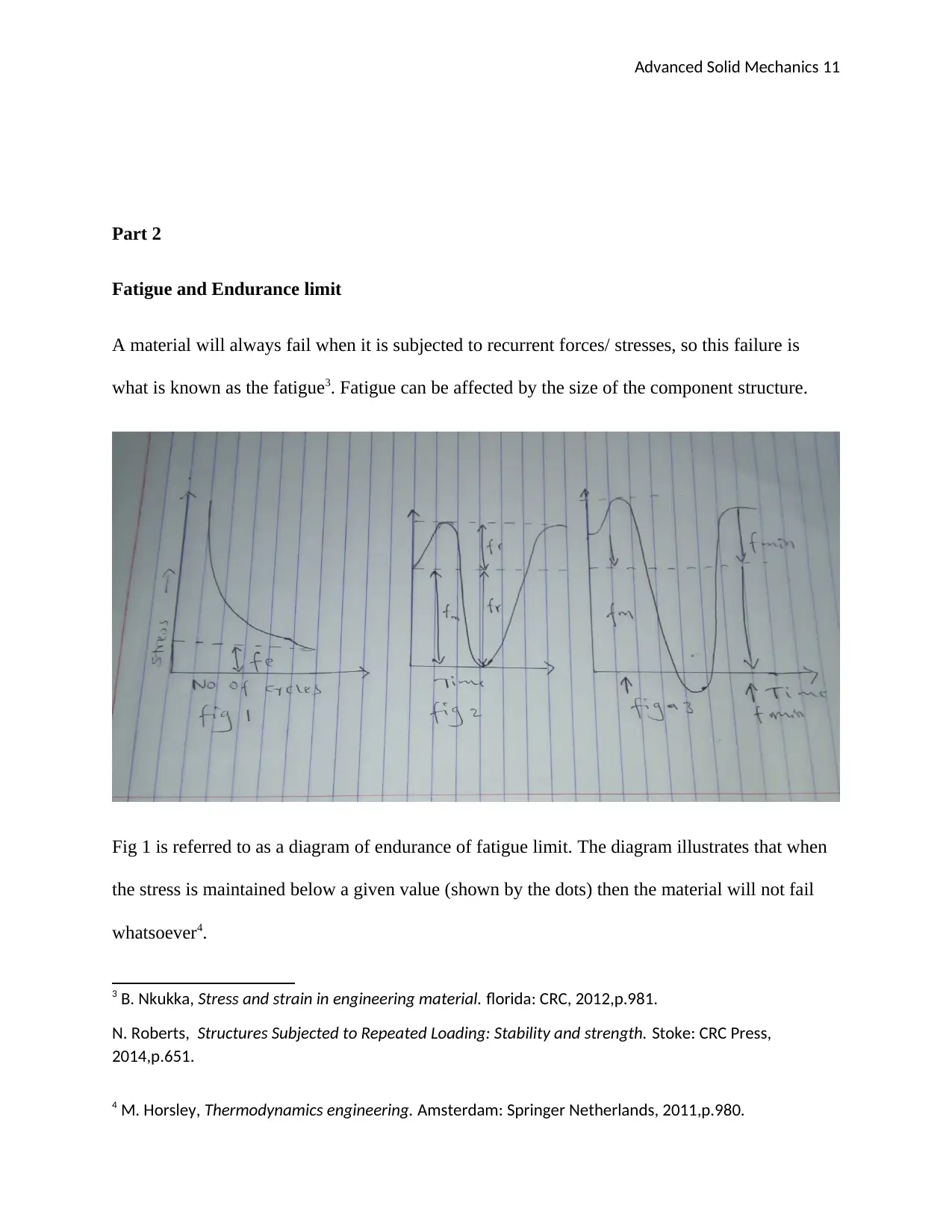
Fatigue and Endurance limit
A material will always fail when it is subjected to recurrent forces/ stresses, so this failure is
what is known as the fatigue3. Fatigue can be affected by the size of the component structure.
Fig 1 is referred to as a diagram of endurance of fatigue limit. The diagram illustrates that when
the stress is maintained below a given value (shown by the dots) then the material will not fail
whatsoever4.
3 B. Nkukka, Stress and strain in engineering material. florida: CRC, 2012,p.981.
N. Roberts, Structures Subjected to Repeated Loading: Stability and strength. Stoke: CRC Press,
2014,p.651.
4 M. Horsley, Thermodynamics engineering. Amsterdam: Springer Netherlands, 2011,p.980.
Part 2
Fatigue and Endurance limit
A material will always fail when it is subjected to recurrent forces/ stresses, so this failure is
what is known as the fatigue3. Fatigue can be affected by the size of the component structure.
Fig 1 is referred to as a diagram of endurance of fatigue limit. The diagram illustrates that when
the stress is maintained below a given value (shown by the dots) then the material will not fail
whatsoever4.
3 B. Nkukka, Stress and strain in engineering material. florida: CRC, 2012,p.981.
N. Roberts, Structures Subjected to Repeated Loading: Stability and strength. Stoke: CRC Press,
2014,p.651.
4 M. Horsley, Thermodynamics engineering. Amsterdam: Springer Netherlands, 2011,p.980.

Advanced Solid Mechanics 12
Fig 2 represents a diagram of time-stress for repeated stress.
Fig 3 Shows a diagram of the time-stress diagram for shifting stress with values fmax and fmin .
Mean stress=fm= fmax +fmin
2
Reversed stress=fv= fmax−fmin
2
For repeat loading stress values from max to zero
Fm=fv= fmax
2
Stress ratio R= fmax
fmin
R=-1 for fully reversed stress
R=0 for recurrent stress
`R` can´t be bigger than unity
The connection between endurance limits and stress ratio
Fe= 3 fe
Z−R
The factor of safety = Endurance limit stress
design of working stress = fe
fd
A. Kobayashi, Aeronautical fatigue: Key to safety and structural integrity; proceedings of the 16th
Symposium of the International Committee on Aeronautical Fatigue. London: Ryoin, 2011,p.67.
Fig 2 represents a diagram of time-stress for repeated stress.
Fig 3 Shows a diagram of the time-stress diagram for shifting stress with values fmax and fmin .
Mean stress=fm= fmax +fmin
2
Reversed stress=fv= fmax−fmin
2
For repeat loading stress values from max to zero
Fm=fv= fmax
2
Stress ratio R= fmax
fmin
R=-1 for fully reversed stress
R=0 for recurrent stress
`R` can´t be bigger than unity
The connection between endurance limits and stress ratio
Fe= 3 fe
Z−R
The factor of safety = Endurance limit stress
design of working stress = fe
fd
A. Kobayashi, Aeronautical fatigue: Key to safety and structural integrity; proceedings of the 16th
Symposium of the International Committee on Aeronautical Fatigue. London: Ryoin, 2011,p.67.

Advanced Solid Mechanics 13
Part 3
Stress concentration
The distribution of stress will not hold good and the neighbourhood of the disjointedness is not
the same. This difference in stress distribution produced by the abrupt change of form is known
as the concentration of stress. If this happens for all types of stress available in notches, fillets
and holes5.
A small consideration will illustrate that the nominal stress in the left and right-hand side shall be
equal in all positions where the area will be changing, this is illustrated in the below diagram;
Stress concentration
Theoretical stress concentration factors, K= Maximum stress
Norminal stress
And fatigue stress concentration is given by the below equation;
Fatigue stress concentration factor = Endurance limit without Stress Concentration
Endurance limit with Stress Concentration
5 J. Krul, Fatigue in most engineering materials. Leicester: Springer, 2011, p.561
Part 3
Stress concentration
The distribution of stress will not hold good and the neighbourhood of the disjointedness is not
the same. This difference in stress distribution produced by the abrupt change of form is known
as the concentration of stress. If this happens for all types of stress available in notches, fillets
and holes5.
A small consideration will illustrate that the nominal stress in the left and right-hand side shall be
equal in all positions where the area will be changing, this is illustrated in the below diagram;
Stress concentration
Theoretical stress concentration factors, K= Maximum stress
Norminal stress
And fatigue stress concentration is given by the below equation;
Fatigue stress concentration factor = Endurance limit without Stress Concentration
Endurance limit with Stress Concentration
5 J. Krul, Fatigue in most engineering materials. Leicester: Springer, 2011, p.561
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Advanced Solid Mechanics 14
Notch sensitivity
In loading (cyclic), the impact of fillet´s notch is always smaller than the projected by the use of
the hypothetical factors as seen before6. The notch sensitivity is employed to this behaviour. This
can be given as a degree by which the hypothetical consequence on the concentration basically is
dependent on the diameter of the notch, size of the material (grain size) and the hole of the fillet.
QUESTION SIX
δ 1 σ 1 σ 2 σ 3
Mpa ±130 Mpa ±120 Mpa ±80 Mpa
NF1 8000 10000 400000
Part 1
From Miner’s rule, failure occurs only when the below equation is true
n 1
N 1 + n 2
N 2 + ……………………..+ ¿
¿ = 1 . . . . . . . . . . . . . . . . . . . . . . . . . . . i
6 A. Culp, Principles of Energy Conversion. Hull: McGraw-Hill, 2011,p.651.
H.GUPTA, FUNDAMENTALS OF INTERNAL COMBUSTION ENGINES. Leicester: PHI Learning Pvt. Ltd,
2012,p.54.
Notch sensitivity
In loading (cyclic), the impact of fillet´s notch is always smaller than the projected by the use of
the hypothetical factors as seen before6. The notch sensitivity is employed to this behaviour. This
can be given as a degree by which the hypothetical consequence on the concentration basically is
dependent on the diameter of the notch, size of the material (grain size) and the hole of the fillet.
QUESTION SIX
δ 1 σ 1 σ 2 σ 3
Mpa ±130 Mpa ±120 Mpa ±80 Mpa
NF1 8000 10000 400000
Part 1
From Miner’s rule, failure occurs only when the below equation is true
n 1
N 1 + n 2
N 2 + ……………………..+ ¿
¿ = 1 . . . . . . . . . . . . . . . . . . . . . . . . . . . i
6 A. Culp, Principles of Energy Conversion. Hull: McGraw-Hill, 2011,p.651.
H.GUPTA, FUNDAMENTALS OF INTERNAL COMBUSTION ENGINES. Leicester: PHI Learning Pvt. Ltd,
2012,p.54.

Advanced Solid Mechanics 15
ni is the no of cycles at ith stress table. Ni is the no of failure corresponds to ith stress relevant.
¿
¿ = ratio of damage
Stages
1. 250 cycles at σ 1
2. 250 cycles at σ 2
3. 250 cycle at σ 3
250
8 ×1 03 + 250
8 ×1 04 + 250
8 ×1 05 = C damage . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2
C = 0.056875 (which is the ratio damage) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3
When C = 1, life is entirely entrusted
X= 10.056875) = 1(repetitions required)
X= 17.5824 repetition needed
Therefore according to Miner’s rule
Number of days = 17.5824 days
Part 2
Life time left after all stages
At 130 MPa, days = 8 ×1 03 × 0.943125
120
ni is the no of cycles at ith stress table. Ni is the no of failure corresponds to ith stress relevant.
¿
¿ = ratio of damage
Stages
1. 250 cycles at σ 1
2. 250 cycles at σ 2
3. 250 cycle at σ 3
250
8 ×1 03 + 250
8 ×1 04 + 250
8 ×1 05 = C damage . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 2
C = 0.056875 (which is the ratio damage) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 3
When C = 1, life is entirely entrusted
X= 10.056875) = 1(repetitions required)
X= 17.5824 repetition needed
Therefore according to Miner’s rule
Number of days = 17.5824 days
Part 2
Life time left after all stages
At 130 MPa, days = 8 ×1 03 × 0.943125
120

Advanced Solid Mechanics 16
= 62.85 days
At 120 Mpa , days = 103 × 0.943125
120
= 78.59 days
At 80Mpa, days = 4 × 105 × 0.943125
120
= 3143.75 days
QUESTION SEVEN
The reverse steel fatigue limit
= ± 270 Mpa = σ max
σ max= +270 Mpa
σ min = -270 Mpa
Endurance limit Se= 0.5 , Sut = 300 Mpa
Sut = 600 Mpa , Sut = 360 Mpa
σ a= σ max+ σ min
2
= 62.85 days
At 120 Mpa , days = 103 × 0.943125
120
= 78.59 days
At 80Mpa, days = 4 × 105 × 0.943125
120
= 3143.75 days
QUESTION SEVEN
The reverse steel fatigue limit
= ± 270 Mpa = σ max
σ max= +270 Mpa
σ min = -270 Mpa
Endurance limit Se= 0.5 , Sut = 300 Mpa
Sut = 600 Mpa , Sut = 360 Mpa
σ a= σ max+ σ min
2
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Advanced Solid Mechanics 17
σ a= 270± 270
2 = 0Mpa
σ m= 0 Mpa
1). The safe range of stress
According to Gerbers creteria
Se= 0.5 , sut = 0.5 ×600 = 300 mpa
hσ
se +( nσm
sut )2 = 1 = σa
se = 1 (σ m =0)
Factor of safety n = se
σa = 300
270
n= 1.11111
safe range is 300-270 = 30
2)
The peak stress and safe range of the stress
From goodmans criteria
σa
se + σm
sut = 1
m n= se
σa (σ m =0)
σ a= 270± 270
2 = 0Mpa
σ m= 0 Mpa
1). The safe range of stress
According to Gerbers creteria
Se= 0.5 , sut = 0.5 ×600 = 300 mpa
hσ
se +( nσm
sut )2 = 1 = σa
se = 1 (σ m =0)
Factor of safety n = se
σa = 300
270
n= 1.11111
safe range is 300-270 = 30
2)
The peak stress and safe range of the stress
From goodmans criteria
σa
se + σm
sut = 1
m n= se
σa (σ m =0)

Advanced Solid Mechanics 18
n= 300
270 = 1.11111111
Based on Soderberg prediction
σa
se + σm
sut = 1
m
σa
300 + 185
260 = 1
m
σ a= σmax−σmin
2 = 86.53
= σ max-σ min = 173.07
σm = σmax+ σmin= 370
σmax= 271.5Mpa
σ min = 98.46 Mpa
n= 300
270 = 1.11111111
Based on Soderberg prediction
σa
se + σm
sut = 1
m
σa
300 + 185
260 = 1
m
σ a= σmax−σmin
2 = 86.53
= σ max-σ min = 173.07
σm = σmax+ σmin= 370
σmax= 271.5Mpa
σ min = 98.46 Mpa

Advanced Solid Mechanics 19
Bibliography
Bottega, W, Engineering Vibrations. Florida: CRC Press, 2013.
Culp, A., Principles of Energy Conversion. Hull: McGraw-Hill, 2011.
GUPTA, H., FUNDAMENTALS OF INTERNAL COMBUSTION ENGINES. Leicester: PHI Learning Pvt. Ltd,
2012.
Horsley, M., Thermodynamics engineering. Amsterdam: Springer Netherlands, 2011.
Kobayashi, A., Aeronautical fatigue: Key to safety and structural integrity; proceedings of the 16th
Symposium of the International Committee on Aeronautical Fatigue. London: Ryoin, 2011.
Krul, J.,Fatigue in most engineering materials. Leicester: Springer, 2011.
Nkukka, B., Stress and strain in engineering material. florida: CRC, 2012.
Roberts, N., Structures Subjected to Repeated Loading: Stability and strength. Stoke: CRC Press, 2014.
Steinbeisser, L., Vibration Problems in Structures: Practical Guidelines. London: Birkhäuser, 2012..
Taylor, F., The Internal-combustion Engine in Theory and Practice: Thermodynamics, fluid flow,
performance. Stoke: MIT Press, 2014.
Bibliography
Bottega, W, Engineering Vibrations. Florida: CRC Press, 2013.
Culp, A., Principles of Energy Conversion. Hull: McGraw-Hill, 2011.
GUPTA, H., FUNDAMENTALS OF INTERNAL COMBUSTION ENGINES. Leicester: PHI Learning Pvt. Ltd,
2012.
Horsley, M., Thermodynamics engineering. Amsterdam: Springer Netherlands, 2011.
Kobayashi, A., Aeronautical fatigue: Key to safety and structural integrity; proceedings of the 16th
Symposium of the International Committee on Aeronautical Fatigue. London: Ryoin, 2011.
Krul, J.,Fatigue in most engineering materials. Leicester: Springer, 2011.
Nkukka, B., Stress and strain in engineering material. florida: CRC, 2012.
Roberts, N., Structures Subjected to Repeated Loading: Stability and strength. Stoke: CRC Press, 2014.
Steinbeisser, L., Vibration Problems in Structures: Practical Guidelines. London: Birkhäuser, 2012..
Taylor, F., The Internal-combustion Engine in Theory and Practice: Thermodynamics, fluid flow,
performance. Stoke: MIT Press, 2014.
1 out of 19
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.
