AIM. The aim of this experiment is to determine the cha
Added on -2019-09-21
| 14 pages
| 2226 words
| 660 views
Trusted by 2+ million users,
1000+ happy students everyday
Showing pages 1 to 4 of 14 pages
AIMThe aim of this experiment is to determine the characteristics of fluid flow of a various tube andplate in a Gunt flow system.Equipment1.Orifice plate2.Nozzle plate3.Venturi tube4.Iris diaphragm valve5.Pitot tube6.PVC pipes7.Hoses8.Flexible hoses 9.16 manometers panel10.anemometer11.electrical air blowerEXPERIMENTALFirstly, a long pipe with 2 sections was unscrewed and pushed away slightly. Between the spaces created, an orifice plate was installed and screwed between them. Not to forget to mention, one of the pipe was attached to a fan. Then, hoses were used and attached to the inlets on the manometer panel. Before doing anything, the potentiometer was set into zero. As the fan was turned on afterwards, air velocities was recorded for each potentiometer for the experiment, which is 5, starting from 2. The difference of water level was measured as well. Steps taken was repeated for the other plate, which is nozzle.Another thing that were used in this experiment is the pilot tube. Using panel 19 and 20, those panels were attached to the hoses. Specifically, the hoses that were used were I and J as it weresmaller in diameter. The experiment carried out in five different potentiometer settings with five different vertical distances from the centre of the pipe.Alternatively, a venture tube was also used and measured in this experiment. Varying diameters on the tube, the panel inlets that were used for the hoses were number 21 until 26. It was recorded with five different potentiometer settings starting from 4 to 9, and also the airflow for each settings.Lastly, the venture tube was replaced with diaphragm valve, using panel inlets number 17 and 18 attached to the hoses. The potentiometer settings that were used are 2 until 6.1
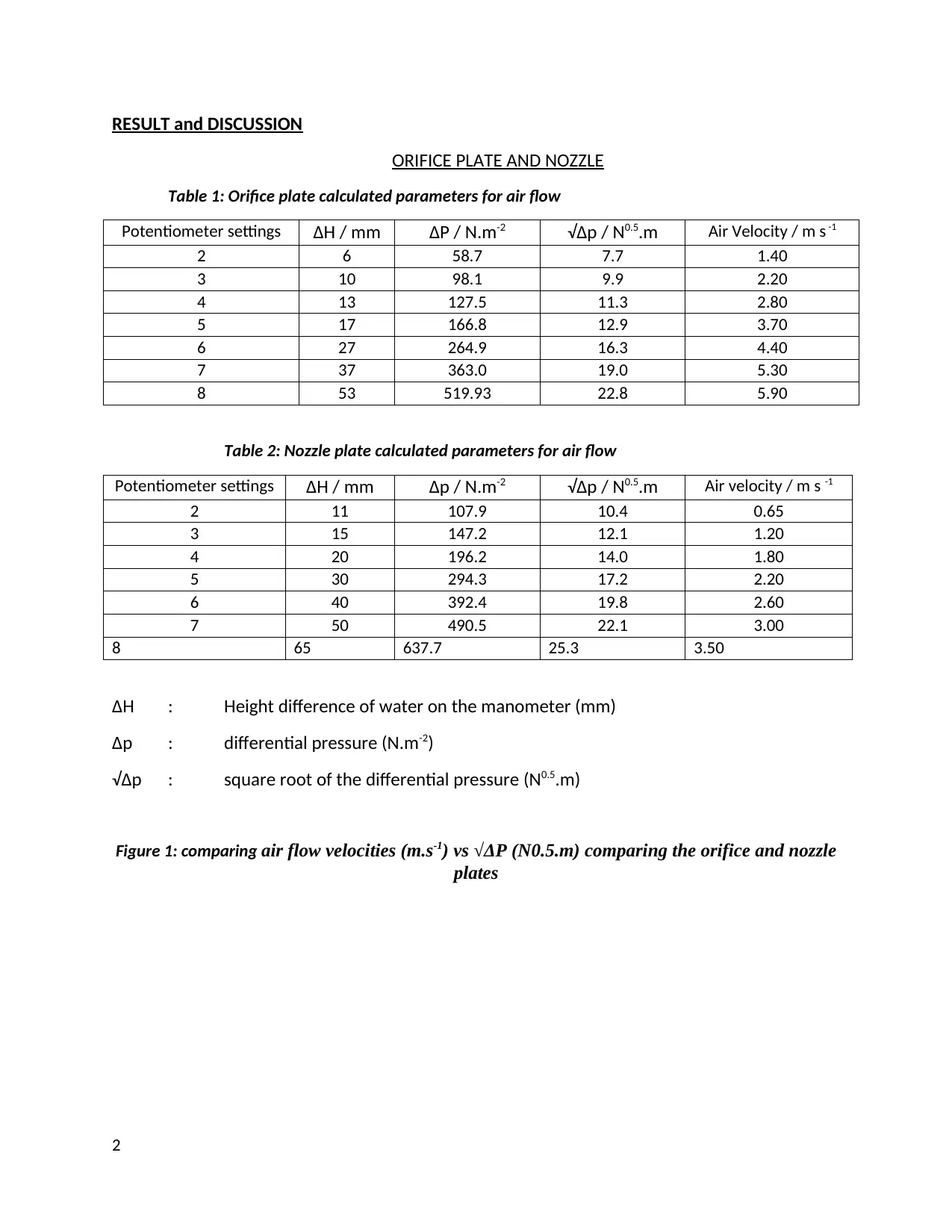
RESULT and DISCUSSIONORIFICE PLATE AND NOZZLETable 1: Orifice plate calculated parameters for air flowPotentiometer settings∆H / mm∆P / N.m-2√∆p / N0.5.mAir Velocity / m s -12658.77.71.4031098.19.92.20413127.511.32.80517166.812.93.70627264.916.34.40737363.019.05.30853519.9322.85.90Table 2: Nozzle plate calculated parameters for air flowPotentiometer settings∆H / mm∆p / N.m-2√∆p / N0.5.mAir velocity / m s -1211107.910.40.65315147.212.11.20420196.214.01.80530294.317.22.20640392.419.82.60750490.522.13.00865637.725.33.50∆H: Height difference of water on the manometer (mm)∆p: differential pressure (N.m-2)√∆p: square root of the differential pressure (N0.5.m)Figure 1: comparing air flow velocities (m.s-1) vs √ΔP (N0.5.m) comparing the orifice and nozzleplates25101520253001234567Orifice PlateLinear (Orifice Plate)Nozzle PlateLinear (Nozzle Plate)√∆p / N0.5.mAirvelocity/ms-1
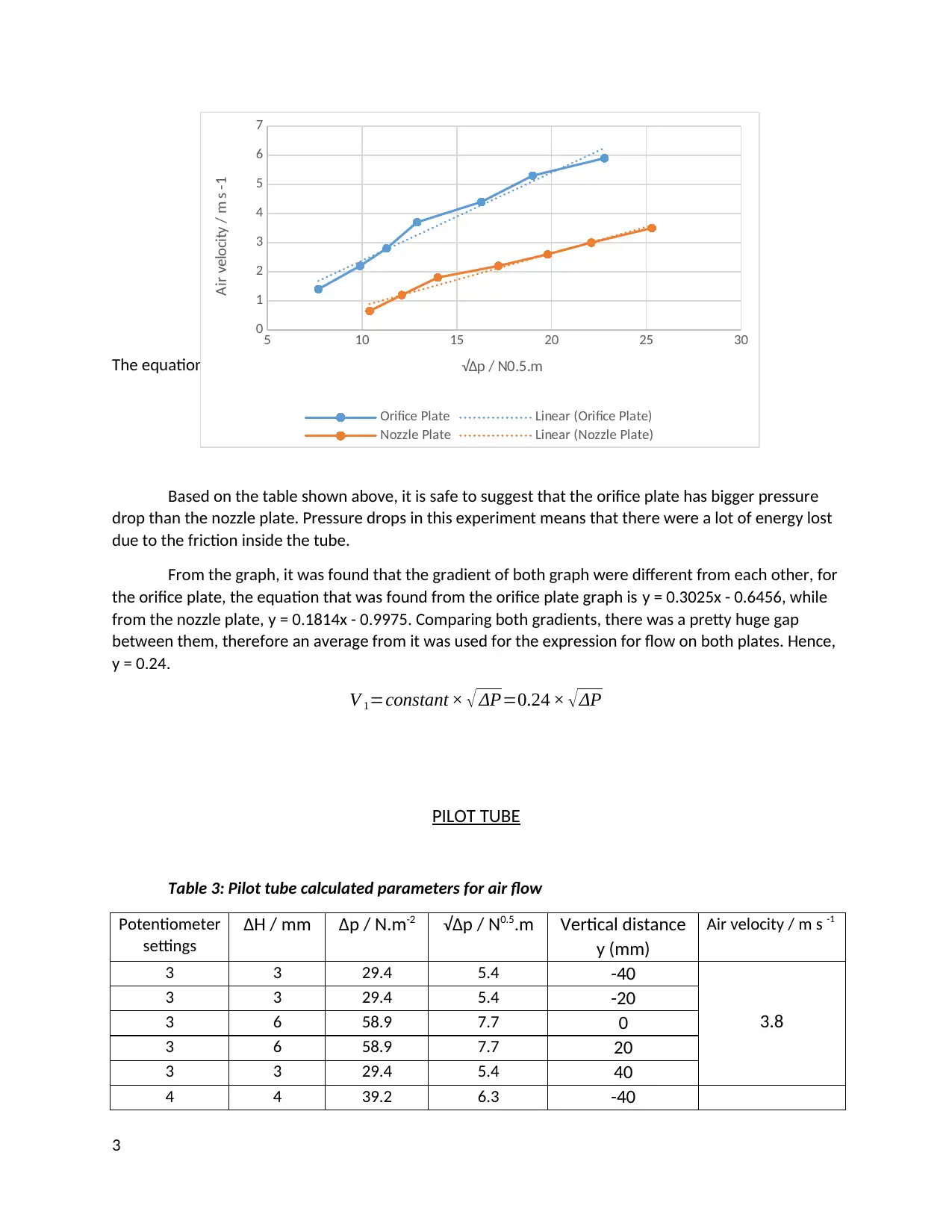
The equation used to find the differential pressure on the table above:∆p=ρw×g×Δhw=1000×9.81×Δhw(basedonthetablegivenabove)Based on the table shown above, it is safe to suggest that the orifice plate has bigger pressure drop than the nozzle plate. Pressure drops in this experiment means that there were a lot of energy lost due to the friction inside the tube.From the graph, it was found that the gradient of both graph were different from each other, forthe orifice plate, the equation that was found from the orifice plate graph is y = 0.3025x - 0.6456, while from the nozzle plate, y = 0.1814x - 0.9975. Comparing both gradients, there was a pretty huge gap between them, therefore an average from it was used for the expression for flow on both plates. Hence,y = 0.24.V1=constant×√ΔP=0.24×√ΔPPILOT TUBETable 3: Pilot tube calculated parameters for air flowPotentiometersettings∆H / mm∆p / N.m-2√∆p / N0.5.mVertical distancey (mm)Air velocity / m s -13329.45.4-403.83329.45.4-203658.97.703658.97.7203329.45.4404439.26.3-405.24988.29.4-2041098.19.9041098.19.9204329.45.4405549.07.0-406.2512117.610.8-20514137.211.70513127.411.3205658.97.7403
61098.19.9-407.8617166.812.9-20622215.814.70618176.413.320611107.910.440720196.014.0-409.4730294.017.1-20733323.7318.00730294.017.120715147.012.140Table 4: Pressure affecting volumetric flowratePotentiometersettings∆p / N.m-2Area of pipe / m2Volumetric flow rate / m3 s-1329.45.62 × 10-3676.1439.25.62 × 10-3925.3549.05.62 × 10-31103.2698.15.62 × 10-31387.97196.05.62 × 10-31672.6Figure 2: comparing vertical distances vs √∆p / N0.5.m in each potentiometers settings4468101214161820-50-40-30-20-1001020304050Setting 3Setting 4Setting 5Setting 6Setting 7√∆p / N0.5.mVerticaldistance(mm)
Found this document preview useful?
You are reading a preview
Upload your documents to download
or
Become a Desklib member to get accesss
Paid Plans
Free Plan
Single Unlock
Monthly Plan
Yearly Plan