Algebraic Methods for Engineers

This assignment covers algebraic methods relevant to engineering applications. Students need to transpose formulas, solve equations, and apply algebraic principles to problems involving physics quantities like velocity, time, and gravity. There are also questions related to geometric progressions and workforce expansion.

MODULE TITLE : ANALYTICAL METHODS FOR ENGINEERS
TOPIC TITLE : ALGEBRAIC METHODS
TUTOR MARKED ASSIGNMENT 1 (v2.1)
NAM
E........................................................................................................................................
ADDRESS.................................................................................................................................
................................................................................................................................................
...................................................... HOME TELEPHONE ..................................................
EMPLOYE
R..............................................................................................................................
...................................................................................................................................................
...................................................... WORK TELEPHONE......................................................
Student declaration:
I declare that all the work submitted is my own work and that no part of it has been copied from
any other source without full acknowledgement and complies with the University's guiding
principles as stated in the Regulations Relating To Academic Misconduct*.
Student signature:.....................................................................................................
Date: ....................................................................................................
Student code: ....................................................................................................
Email: ....................................................................................................
* http://www.tees.ac.uk/docs/index.cfm?folder=Student%20Regulations&name=Academic%20Regulations
Teesside University Open Learning © Teesside University 2011
( Engineering )

1. (a) Transpose the following formula to make v the subject
f = uv
u+v
Solution
f = uv
( u+v )
f ( u+ v )=uv
fu+ fv=uv
fu=uv−fv
fu=v ( u−f )
v= fu
u−f
Teesside University Open Learning © Teesside University 2011
( Engineering )

(b) Solve the following equation to find the value of x:
(3.4)2x +3 = 8.5
solution
3.4 × 3.4 x+3=14.56 x
14.56 x=8.5
x=1.713
–Rt
(c) In the formula θ = Ve L , the value of θ = 58, V = 255, R = 0.1
and L = 0.5. Find the corresponding value of t.
solution
k =ve ×− Rt
l
58=255 e ×− 0.1t
0.5
−0.1
0.5 =0.2 t
255 e ×−0.2t=−51 et
Teesside University Open Learning © Teesside University 2011
( Engineering )

58=−51 et
et=58 — 51
tlog=log (58−−51
elog )
t= 2.037
elog
(d) ω = 1h ln⎛⎜⎝ LL0 – 1⎞ ⎟ ⎠
Find L if ω = –2.6, L0 = 16 and h = 1.5.
solution
d−2.6=ln ( l
16 −1)
taking on both sides we get ,
d e−3.9= l
16 −1
d 1.02024=l/16
dl=16.32384
Teesside University Open Learning © Teesside University 2011
( Engineering )

2. (a) Use polynomial long division to determine the quotient when
3x3 – 5x2 + 10x + 4 is divided by 3x + 1
solution
divide the didvidend by the divisor
1 st term of the quotient : x 2
multiply ×the divisor ¿ get 3 x 3+x 2
subtract ¿ the dividend ¿ get −6 x 2+10 x+4
divide that dividend by the divisor
2 nd term of the quotient −2 x
multiply ×the divisor ¿ get−6 x 2−2 x
subtract ¿ the dividend ¿ get 12 x + 4
divide the dividend by the divisor ¿ get 3 rd term of the quotient 4
multiply ×the divisor ¿ get 12 x+ 4
subtract ¿ the dividend ¿ get 0
¿ x 2−2 x + 4
(b) Show, by polynomial long division that
x3 – 3x2 + 12x – 5 = (x2 – x + 10) + 15 x – 2
x – 2 solution
−x2+ 12 x−5
−x2+ 2 x
10 x−5
10 x−20
now since dividend=quotient∗divisor +remainder
d x3−3 x2 +12 x−5= ( x −2 )∗( x2−x +10 ) +15
¿ x3 −3 x2 +5
x−2 =¿
Teesside University Open Learning © Teesside University 2011
( Engineering )

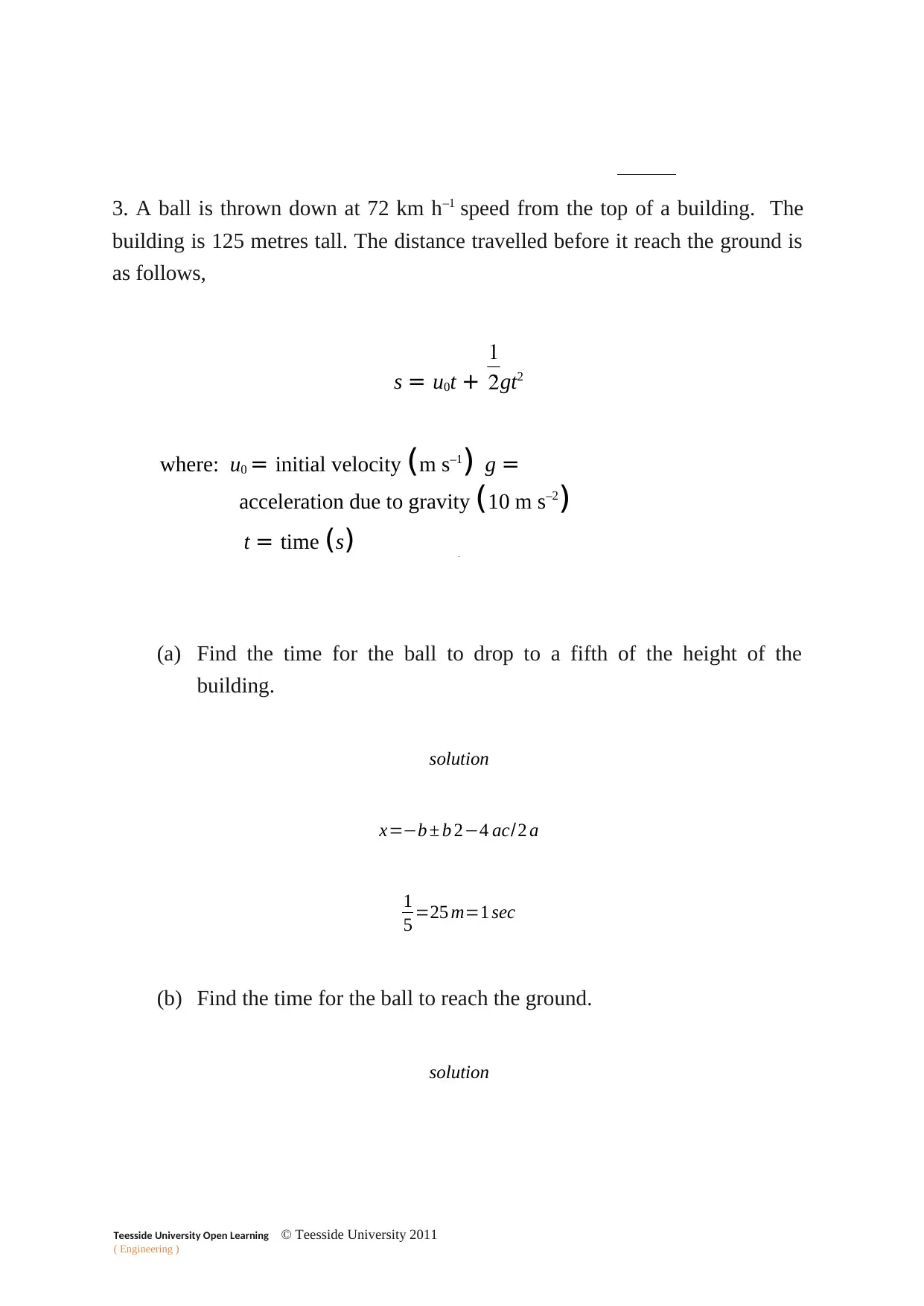
3. A ball is thrown down at 72 km h–1 speed from the top of a building. The
building is 125 metres tall. The distance travelled before it reach the ground is
as follows,
s = u0t + gt2
where: u0 = initial velocity (m s–1) g =
acceleration due to gravity (10 m s–2)
t = time (s)
(a) Find the time for the ball to drop to a fifth of the height of the
building.
solution
x=−b ± b 2−4 ac/2 a
1
5 =25 m=1 sec
(b) Find the time for the ball to reach the ground.
solution
Teesside University Open Learning © Teesside University 2011
( Engineering )

125=20t +( 1
2 ) ( 9.8 ) t 2
4.9 t 2+ 20t−125=0
t=−20 ±53.3854
9.8
t=3.4067 s
4. Water fills a tank at a rate of 150 litres during the first hour, 350 litres
during the second hour, 550 litres during the third hour and so on. Find
the number of hours necessary to fill a rectangular tank 16 m × 9 m × 9
m.
solution
sn=n2 ¿
¿ n 2 ¿
100 n 2+50 n−1296000=0
n=113.59 hours
5. (a) A firm starts work with 110 employees for the 1st week. The number
of the employees rises by 6% per week. How many persons will be
employed in the 20th week if the present rate of expansion continues.
solution
Teesside University Open Learning © Teesside University 2011
( Engineering )

the multiplier is 1.06
total=110 (1.0620 )
¿ 353 employees
(b) A contractor hires out machinery. In the first year of hiring out one
piece of equipment the profit is £6000, but this diminishes by 5% in
successive years. Show that the annual profits form a geometric
progression and find the total of all the profit for the first 5 years.
solution
Profit in 1st year (p) = 60,000
Rate of decrease = 5% = 0.05 (r)
Total profit at the end of 5 years
= p + p(1-r) + p(1-r)2 + p(1-r)3 + p(1-r)4
= p [1 + (1-r) + (1-r)2 + (1-r)3 + (1-r)4 ]
Thus, it forms a geometric series with common ratio = 1-r
= p [1-(1-r)5]/[1-(1-r)]
= 60,000 * (1-0.955) / 0.95
= 14287.5 (approx)
ANS: The total of all the profit for the first 5 years is £14287.5
Teesside University Open Learning © Teesside University 2011
( Engineering )