Retail Markup and Pricing Strategies
VerifiedAdded on 2020/04/07
|9
|1006
|358
AI Summary
This assignment focuses on understanding and applying retail markup and pricing concepts. It presents several scenarios involving businesses like Homeward Hardware and appliance stores. Students must calculate selling prices, markups based on cost and selling price, overhead expenses, profit margins, break-even points, and operating profits or losses under different sale conditions.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Applied Mathematics
Name
University
21st September 2017
1
Name
University
21st September 2017
1
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Page 30:
Q16: Silvio’s gross earnings for last week were $328.54. His remuneration consists of a base
salary of $280 plus a commission of 6% on net sales exceeding his weekly quota of $5000. What
were Silvio’s gross sales for the week if sales returns and allowances were $136?
Solution
Let gross sales be s;
280+0.06 (s−136−5000)=$ 328.54280+0.06 s−308.16=328.54 0.06 s−28.16=328.54 ,
S=$ 5945=Gross sales .
Q18: Ramona’s Dry Cleaning shows sales revenue of $76,000 for the year. Romano’s GST-
taxable expenses were $14,960. How much should she remit to the government at the end of the
year?
Solution
Tax on GST =0.05∗14 ,960=$ 748
Tax on Sales revenue=0.08∗76,000=$ 6,080
Total amount ¿ remit =$ 6,080+$ 748=$ 6,828
Q20: A store located in Kelowna, B.C., sells a computer for $1868 plus GST and PST. If the
same model is sold at the same price in a store in Kenora, Ontario, what is the difference in the
prices paid by customers in the two stores?
Solution
In Kelowna BC, total tax rate = PST + GST = 7% + 5% = 12%
Similarly
In Kenora Ontario, total tax rate = HST + GST = 8% + 5% = 13%
Therefore in Kelowna BC,
Consumer would pay $1868 + 12% of $1868 = 1868 + 224.16 = $2092.16
Also in Kenora
Consumer would pay $1868 + 13% of $1868 = 1868 + 242.84 = $2110.84
Difference in price = $2110.84 - $2092.16 = $18.68
Page 116-117:
2
Q16: Silvio’s gross earnings for last week were $328.54. His remuneration consists of a base
salary of $280 plus a commission of 6% on net sales exceeding his weekly quota of $5000. What
were Silvio’s gross sales for the week if sales returns and allowances were $136?
Solution
Let gross sales be s;
280+0.06 (s−136−5000)=$ 328.54280+0.06 s−308.16=328.54 0.06 s−28.16=328.54 ,
S=$ 5945=Gross sales .
Q18: Ramona’s Dry Cleaning shows sales revenue of $76,000 for the year. Romano’s GST-
taxable expenses were $14,960. How much should she remit to the government at the end of the
year?
Solution
Tax on GST =0.05∗14 ,960=$ 748
Tax on Sales revenue=0.08∗76,000=$ 6,080
Total amount ¿ remit =$ 6,080+$ 748=$ 6,828
Q20: A store located in Kelowna, B.C., sells a computer for $1868 plus GST and PST. If the
same model is sold at the same price in a store in Kenora, Ontario, what is the difference in the
prices paid by customers in the two stores?
Solution
In Kelowna BC, total tax rate = PST + GST = 7% + 5% = 12%
Similarly
In Kenora Ontario, total tax rate = HST + GST = 8% + 5% = 13%
Therefore in Kelowna BC,
Consumer would pay $1868 + 12% of $1868 = 1868 + 224.16 = $2092.16
Also in Kenora
Consumer would pay $1868 + 13% of $1868 = 1868 + 242.84 = $2110.84
Difference in price = $2110.84 - $2092.16 = $18.68
Page 116-117:
2
Q2: The following information is available from the accounting records of Eva Corporation:
Fixed costs per period are $4800. Sales volume for the last period was $19,360, and variable
costs were $13,552. Capacity per period is a sales volume of $32,000.
(a) Compute
(i) The contribution margin;
Contribution margin=Revenue – Variable cost
Contribution margin=$ 19,360 – $ 13,552
Contribution margin=$ 5,808
(ii) The contribution rate
Solution
Contribution rate=Contribution margin
Revenue = $ 5808
$ 19360 =30 %
(b) Compute the break-even point
(i) In sales dollars;
Solution
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 4800
0.3 =$ 16 , 000
(ii) As a percent of capacity.
Solution
Break−even point as a percentage of capacity= Break−even point ∈units
C apacity for the period = $ 16 000
$ 32000 ∗100 %=50
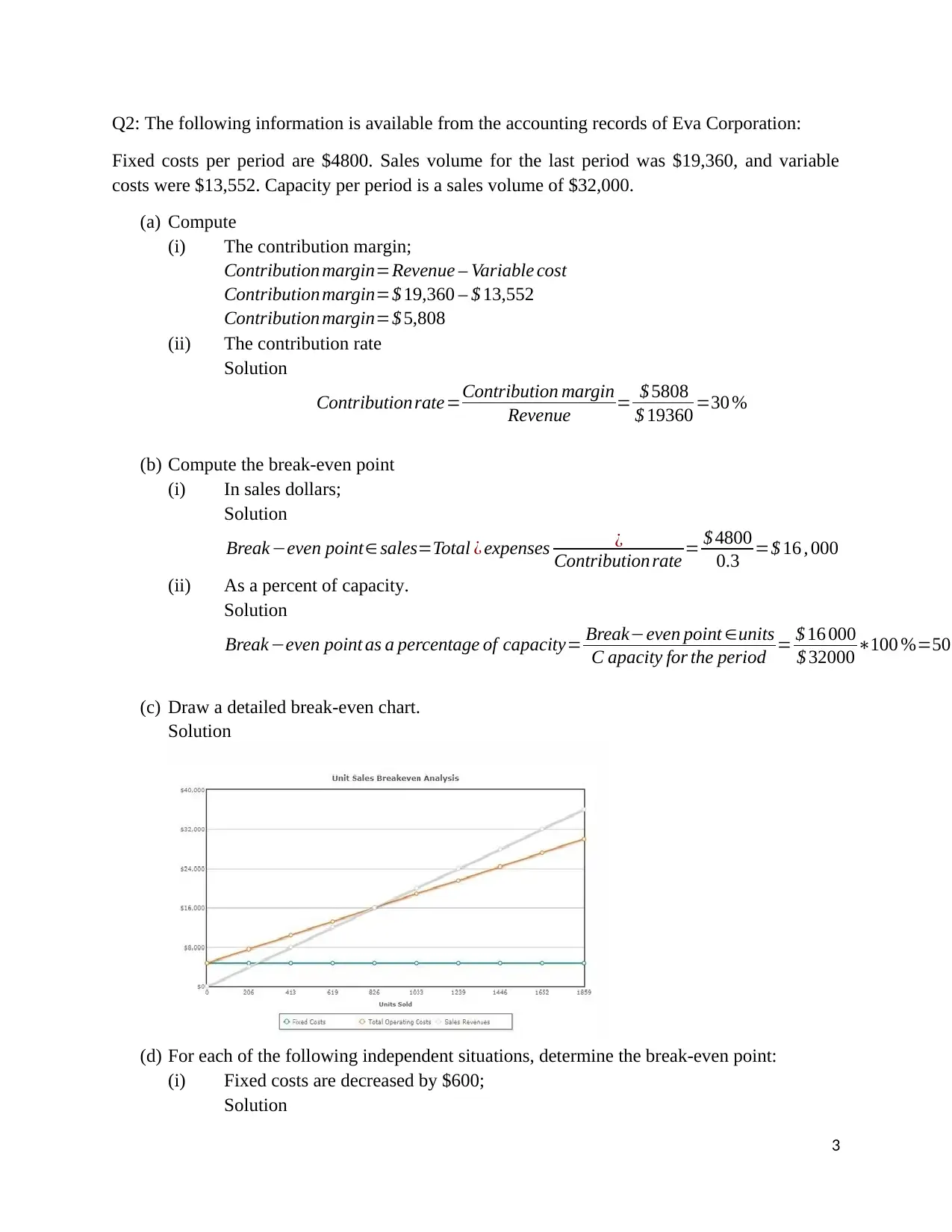
(c) Draw a detailed break-even chart.
Solution
(d) For each of the following independent situations, determine the break-even point:
(i) Fixed costs are decreased by $600;
Solution
3
Fixed costs per period are $4800. Sales volume for the last period was $19,360, and variable
costs were $13,552. Capacity per period is a sales volume of $32,000.
(a) Compute
(i) The contribution margin;
Contribution margin=Revenue – Variable cost
Contribution margin=$ 19,360 – $ 13,552
Contribution margin=$ 5,808
(ii) The contribution rate
Solution
Contribution rate=Contribution margin
Revenue = $ 5808
$ 19360 =30 %
(b) Compute the break-even point
(i) In sales dollars;
Solution
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 4800
0.3 =$ 16 , 000
(ii) As a percent of capacity.
Solution
Break−even point as a percentage of capacity= Break−even point ∈units
C apacity for the period = $ 16 000
$ 32000 ∗100 %=50
(c) Draw a detailed break-even chart.
Solution
(d) For each of the following independent situations, determine the break-even point:
(i) Fixed costs are decreased by $600;
Solution
3

In Sales dollars:
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 4800+ $ 600
0.3 = $ 5400
0.3 =$ 18 ,000
As a percent of capacity
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = $ 18 000
$ 32000 ∗100 %=5 6
(ii) Fixed costs are increased to $5670 and variable costs are changed to 55% of sales.
Solution
New Fixes costs is $5670
New Variable costs is 0.55*19360 = $10,648
Contribution margin=Revenue – Variable cost
Contribution margin=$ 19,360 – $ 10648
Contribution margin=$ 8,712
C ontributionrate=Contribution margin
Revenue = $ 8712
$ 19360 =45 %
In sales dollars
Break−even point∈sales=Total ¿ expenses ¿
Contribution rate = $ 5670
0.45 =$ 12,6 00
As a percent of capacity
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = $ 12600
$ 32000∗100 %=39.
Q4: A manufacturer of major appliances provides the following information about the operations
of the refrigeration division:
Fixed costs per period are $26,880; variable costs per unit are $360; selling price per unit is
$640; and capacity is 150 units.
(a) Compute
(i) The contribution margin;
Solution
Contribution margin per unit=Revenue per unit – Variable cost per unit
Contribution margin=$ 640 – $ 360
Contribution margin=$ 280
(ii) The contribution rate
Solution
Contribution rate=Contribution margin
Revenue = $ 280
$ 640 =43.75%
(b) Compute the break-even point
4
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 4800+ $ 600
0.3 = $ 5400
0.3 =$ 18 ,000
As a percent of capacity
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = $ 18 000
$ 32000 ∗100 %=5 6
(ii) Fixed costs are increased to $5670 and variable costs are changed to 55% of sales.
Solution
New Fixes costs is $5670
New Variable costs is 0.55*19360 = $10,648
Contribution margin=Revenue – Variable cost
Contribution margin=$ 19,360 – $ 10648
Contribution margin=$ 8,712
C ontributionrate=Contribution margin
Revenue = $ 8712
$ 19360 =45 %
In sales dollars
Break−even point∈sales=Total ¿ expenses ¿
Contribution rate = $ 5670
0.45 =$ 12,6 00
As a percent of capacity
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = $ 12600
$ 32000∗100 %=39.
Q4: A manufacturer of major appliances provides the following information about the operations
of the refrigeration division:
Fixed costs per period are $26,880; variable costs per unit are $360; selling price per unit is
$640; and capacity is 150 units.
(a) Compute
(i) The contribution margin;
Solution
Contribution margin per unit=Revenue per unit – Variable cost per unit
Contribution margin=$ 640 – $ 360
Contribution margin=$ 280
(ii) The contribution rate
Solution
Contribution rate=Contribution margin
Revenue = $ 280
$ 640 =43.75%
(b) Compute the break-even point
4
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

(i) In units;
Solution
Break−even point∈units=Total ¿ expenses ¿
Contribution per unit = $ 26880
$ 280 =96
(ii) As a percent of capacity;
Solution
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = 96
15 0∗100 %=64 %
(iii) In sales dollars.
Solution
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 2688 0
0.4375 =$ 61,440
(c) Determine the break-even point in sales dollars if fixed costs are increased to $32,200
Solution
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 3220 0
0.4375 =$ 73,600
(d) Determine the break-even point as a percent of capacity of fixed costs are reduced to
$23,808, while variable costs are increased to 60% of sales.
Solution
New Fixed costs is $23808
New Variable costs is 0.6*640 = $384
Contribution margin=Revenue – Variable cost
Contribution margin=$ 640 – $ 384
Contribution margin=$ 256
ontribution rate=Contribution margin
Revenue = $ 256
$ 640 =40 %
In sales dollars
Break−even point∈units=Total ¿ expenses ¿
Contribution per unit = $ 2380 8
$ 256 =93
As a percent of capacity
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = 93
150∗100 %=6 2 %
5
Solution
Break−even point∈units=Total ¿ expenses ¿
Contribution per unit = $ 26880
$ 280 =96
(ii) As a percent of capacity;
Solution
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = 96
15 0∗100 %=64 %
(iii) In sales dollars.
Solution
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 2688 0
0.4375 =$ 61,440
(c) Determine the break-even point in sales dollars if fixed costs are increased to $32,200
Solution
Break−even point∈ sales=Total ¿ expenses ¿
Contribution rate = $ 3220 0
0.4375 =$ 73,600
(d) Determine the break-even point as a percent of capacity of fixed costs are reduced to
$23,808, while variable costs are increased to 60% of sales.
Solution
New Fixed costs is $23808
New Variable costs is 0.6*640 = $384
Contribution margin=Revenue – Variable cost
Contribution margin=$ 640 – $ 384
Contribution margin=$ 256
ontribution rate=Contribution margin
Revenue = $ 256
$ 640 =40 %
In sales dollars
Break−even point∈units=Total ¿ expenses ¿
Contribution per unit = $ 2380 8
$ 256 =93
As a percent of capacity
Break−even point as a percentage of capacity= Break−even point ∈units
Capacity for the period = 93
150∗100 %=6 2 %
5

Page 162-163:
Q12: The Peel Trading Company received an invoice dated September 20 for $16,000
less 25% and 20%, terms 5/10, 2/30, n/60. Peel made a payment on September 30 to
reduce the debt to $5000 and a payment on October 20 to reduce the debt by $3000.
(a) What amount must Peel remit to pay the balance of the debt at the end of the credit
period?
(b) What is the total amount paid by Peel?
Q14: Homeward Hardware buys cat litter for $6 less 20% per bag. The store’s overhead
is 45% of cost and the owner requires a profit of 20% of cost.
(a) For how much should the bags be sold?
Cost =0.8∗$ 6=$ 4.8
Profit=0.2∗$ 4.8=$ 0.96
Profit=Selling Price−Cost Price−Overhead costs
Selling Price=Cost Price+ Profit+Overhead cost
Selling Price=$ 4.8+ $ 0.96+ $ 2.16=$ 7.92
(b) What is the amount of markup included in the selling price?
Solution
Mark up Amount= ( Sale price−Cost price )
Mark up= ( $ 7.92−$ 4.8 ) =$ 3.18
(c) What is the rate of the markup based on selling price?
Solution
M ark up= ( Sale price−Cost price )
Sale price
Mark up= ( $ 7.92−$ 4.8 )
$ 7.92 ∗100 %= $ 3.18
$ 7.92∗100 %=39.39%
(d) What is the rate of markup based on cost?
Solution
M ark up= ( Sale price−Cost price )
Cost price
M ark up= ( $ 7.92−$ 4.8 )
$ 4.8 = $ 3.18
$ 4.8 ∗100 %=66.25 %
(e) What is the break-even price?
Solution
Break−even price=Overhead expenses/(1 – (Cost of Goods Sold/Total Sales))
6
Q12: The Peel Trading Company received an invoice dated September 20 for $16,000
less 25% and 20%, terms 5/10, 2/30, n/60. Peel made a payment on September 30 to
reduce the debt to $5000 and a payment on October 20 to reduce the debt by $3000.
(a) What amount must Peel remit to pay the balance of the debt at the end of the credit
period?
(b) What is the total amount paid by Peel?
Q14: Homeward Hardware buys cat litter for $6 less 20% per bag. The store’s overhead
is 45% of cost and the owner requires a profit of 20% of cost.
(a) For how much should the bags be sold?
Cost =0.8∗$ 6=$ 4.8
Profit=0.2∗$ 4.8=$ 0.96
Profit=Selling Price−Cost Price−Overhead costs
Selling Price=Cost Price+ Profit+Overhead cost
Selling Price=$ 4.8+ $ 0.96+ $ 2.16=$ 7.92
(b) What is the amount of markup included in the selling price?
Solution
Mark up Amount= ( Sale price−Cost price )
Mark up= ( $ 7.92−$ 4.8 ) =$ 3.18
(c) What is the rate of the markup based on selling price?
Solution
M ark up= ( Sale price−Cost price )
Sale price
Mark up= ( $ 7.92−$ 4.8 )
$ 7.92 ∗100 %= $ 3.18
$ 7.92∗100 %=39.39%
(d) What is the rate of markup based on cost?
Solution
M ark up= ( Sale price−Cost price )
Cost price
M ark up= ( $ 7.92−$ 4.8 )
$ 4.8 = $ 3.18
$ 4.8 ∗100 %=66.25 %
(e) What is the break-even price?
Solution
Break−even price=Overhead expenses/(1 – (Cost of Goods Sold/Total Sales))
6

Break−even price= $ 2.16
(1− $ 4.8
$ 7.92 )=$ 5.48
(f) What operating profit or loss is made if a bag is sold for $6?
Solution
Sale price $ 6
Total deductions=$ 4.8+$ 2.16=$ 6.96
Operating loss=$ 6.96−$ 6=$ 0.96
Q16: Using a markup of 35% of cost, a store priced a book at $8.91.
(a) What was the cost of the book?
Solution
Mark up= ( Sale price−Cost price )
Cost price
Let Cost price be x
Mark up= ( 8.91−x )
x =0.35
8.91−x =0.35 x
1.35 x=8.91
x= 8.91
1.35 =$ 6.6
Thus the cost of the book was $6.6
(b) What is the markup as a percent of selling price?
Solution
Mark up= ( Sale price−Cost price )
Sale Price
Mark up= 8.91−6.6
8.91 ∗100 %=25. 93 %
Q20: An appliance store sold GE coffeemakers for $22.95 during a promotional sale.
The store bought the coffeemakers for $36 less 40% and 15%. Overhead is 25% of
the regular selling price.
(a) If the store’s markup is 40% of the regular selling price, what was the rate of
markdown?
Solution
Cost price=0.6∗0.85∗36=$ 18.36
Mark up= ( Sale price−Cost price )
Sale Price
Let sale price be x
Mark up= ( x −18.36 )
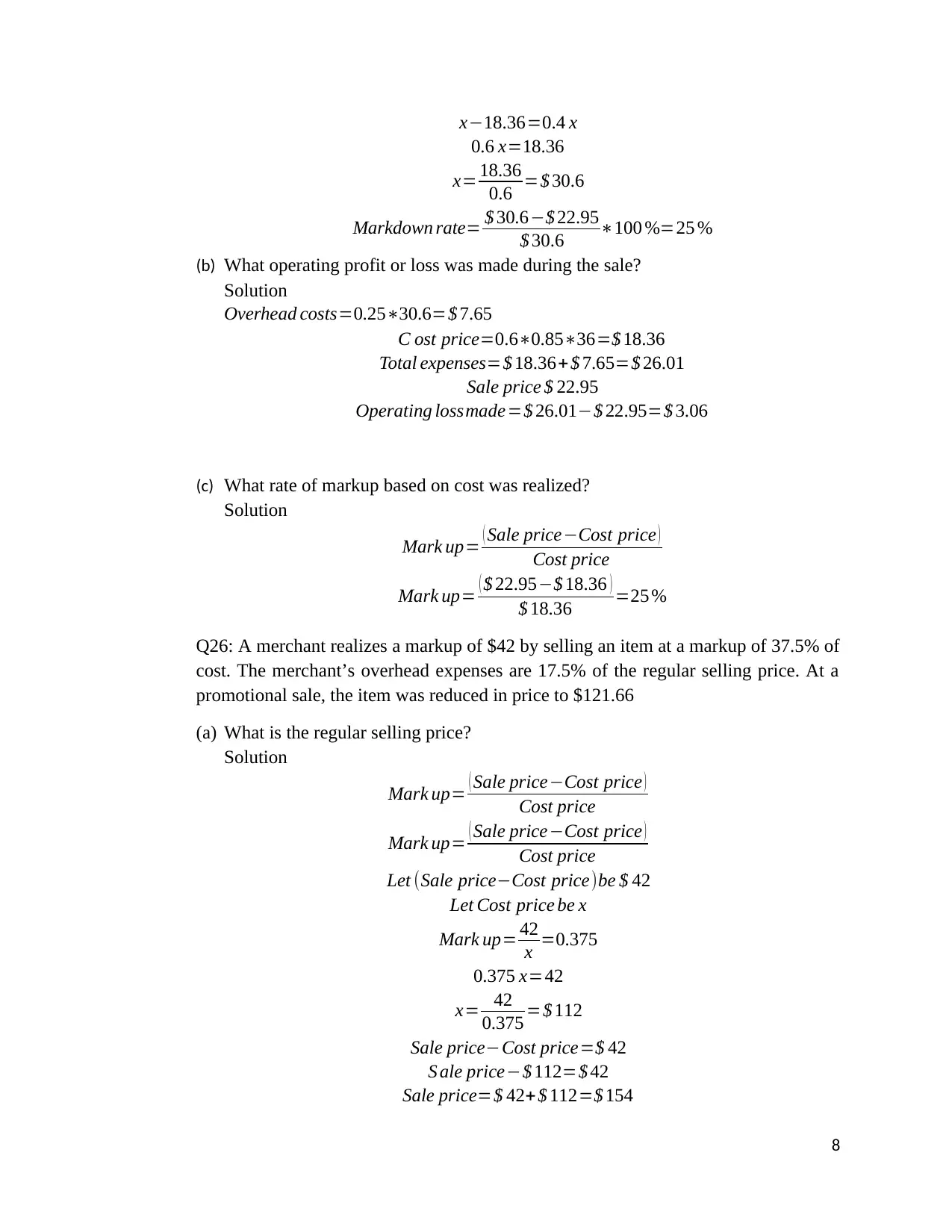
x =0.4
7
(1− $ 4.8
$ 7.92 )=$ 5.48
(f) What operating profit or loss is made if a bag is sold for $6?
Solution
Sale price $ 6
Total deductions=$ 4.8+$ 2.16=$ 6.96
Operating loss=$ 6.96−$ 6=$ 0.96
Q16: Using a markup of 35% of cost, a store priced a book at $8.91.
(a) What was the cost of the book?
Solution
Mark up= ( Sale price−Cost price )
Cost price
Let Cost price be x
Mark up= ( 8.91−x )
x =0.35
8.91−x =0.35 x
1.35 x=8.91
x= 8.91
1.35 =$ 6.6
Thus the cost of the book was $6.6
(b) What is the markup as a percent of selling price?
Solution
Mark up= ( Sale price−Cost price )
Sale Price
Mark up= 8.91−6.6
8.91 ∗100 %=25. 93 %
Q20: An appliance store sold GE coffeemakers for $22.95 during a promotional sale.
The store bought the coffeemakers for $36 less 40% and 15%. Overhead is 25% of
the regular selling price.
(a) If the store’s markup is 40% of the regular selling price, what was the rate of
markdown?
Solution
Cost price=0.6∗0.85∗36=$ 18.36
Mark up= ( Sale price−Cost price )
Sale Price
Let sale price be x
Mark up= ( x −18.36 )
x =0.4
7
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
x−18.36=0.4 x
0.6 x=18.36
x= 18.36
0.6 =$ 30.6
Markdown rate= $ 30.6−$ 22.95
$ 30.6 ∗100 %=25 %
(b) What operating profit or loss was made during the sale?
Solution
Overhead costs=0.25∗30.6=$ 7.65
C ost price=0.6∗0.85∗36=$ 18.36
Total expenses=$ 18.36+$ 7.65=$ 26.01
Sale price $ 22.95
Operating lossmade=$ 26.01−$ 22.95=$ 3.06
(c) What rate of markup based on cost was realized?
Solution
Mark up= ( Sale price−Cost price )
Cost price
Mark up= ( $ 22.95−$ 18.36 )
$ 18.36 =25 %
Q26: A merchant realizes a markup of $42 by selling an item at a markup of 37.5% of
cost. The merchant’s overhead expenses are 17.5% of the regular selling price. At a
promotional sale, the item was reduced in price to $121.66
(a) What is the regular selling price?
Solution
Mark up= ( Sale price−Cost price )
Cost price
Mark up= ( Sale price−Cost price )
Cost price
Let (Sale price−Cost price)be $ 42
Let Cost price be x
Mark up= 42
x =0.375
0.375 x=42
x= 42
0.375 =$ 112
Sale price−Cost price=$ 42
S ale price−$ 112=$ 42
Sale price=$ 42+$ 112=$ 154
8
0.6 x=18.36
x= 18.36
0.6 =$ 30.6
Markdown rate= $ 30.6−$ 22.95
$ 30.6 ∗100 %=25 %
(b) What operating profit or loss was made during the sale?
Solution
Overhead costs=0.25∗30.6=$ 7.65
C ost price=0.6∗0.85∗36=$ 18.36
Total expenses=$ 18.36+$ 7.65=$ 26.01
Sale price $ 22.95
Operating lossmade=$ 26.01−$ 22.95=$ 3.06
(c) What rate of markup based on cost was realized?
Solution
Mark up= ( Sale price−Cost price )
Cost price
Mark up= ( $ 22.95−$ 18.36 )
$ 18.36 =25 %
Q26: A merchant realizes a markup of $42 by selling an item at a markup of 37.5% of
cost. The merchant’s overhead expenses are 17.5% of the regular selling price. At a
promotional sale, the item was reduced in price to $121.66
(a) What is the regular selling price?
Solution
Mark up= ( Sale price−Cost price )
Cost price
Mark up= ( Sale price−Cost price )
Cost price
Let (Sale price−Cost price)be $ 42
Let Cost price be x
Mark up= 42
x =0.375
0.375 x=42
x= 42
0.375 =$ 112
Sale price−Cost price=$ 42
S ale price−$ 112=$ 42
Sale price=$ 42+$ 112=$ 154
8

(b) What is the rate of markup based on the regular selling price?
Solution
Mark up= ( Sale price−Cost price )
Sale price
ark up= 42
154∗100 %=27.27 %
(c) What is the rate of markdown?
Solution
Markdown rate= $ 154−$ 121.66
$ 154 ∗100 %=21 %
(d) What is the profit or loss during the promotional sale?
Solution
Overhead expenses=17.5 %∗$ 154=$ 26.95
Cost price=$ 112
Total expenses=$ 112+$ 26.95=$ 138.95
Promotional sale price=$ 121.66
Loss made=$ 138.95−$ 121.66=$ 17.29
9
Solution
Mark up= ( Sale price−Cost price )
Sale price
ark up= 42
154∗100 %=27.27 %
(c) What is the rate of markdown?
Solution
Markdown rate= $ 154−$ 121.66
$ 154 ∗100 %=21 %
(d) What is the profit or loss during the promotional sale?
Solution
Overhead expenses=17.5 %∗$ 154=$ 26.95
Cost price=$ 112
Total expenses=$ 112+$ 26.95=$ 138.95
Promotional sale price=$ 121.66
Loss made=$ 138.95−$ 121.66=$ 17.29
9
1 out of 9
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.

