Heat Equation Solutions on Disk and Sphere: Applied Mathematics

This assignment presents a comprehensive solution to the heat equation on a disk and a sphere. It begins by establishing the problem's context, including the PDE and boundary conditions. The solution employs separation of variables, deriving ODEs and identifying eigenvalues and eigenfunctions. It...Read More

Applied Mathematics
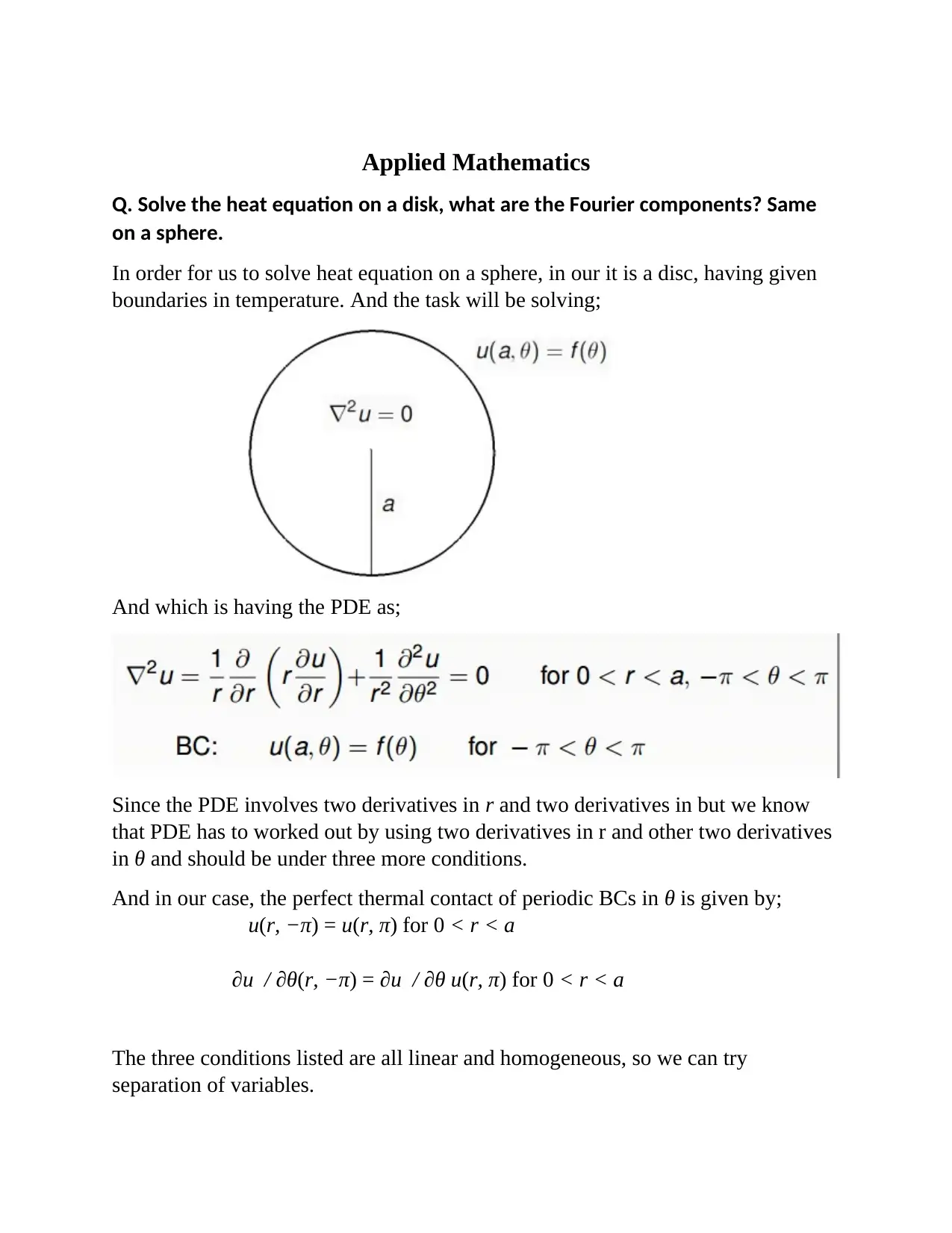
Q. Solve the heat equation on a disk, what are the Fourier components? Same
on a sphere.
In order for us to solve heat equation on a sphere, in our it is a disc, having given
boundaries in temperature. And the task will be solving;
And which is having the PDE as;
Since the PDE involves two derivatives in r and two derivatives in but we know
that PDE has to worked out by using two derivatives in r and other two derivatives
in θ and should be under three more conditions.
And in our case, the perfect thermal contact of periodic BCs in θ is given by;
u(r, −π) = u(r, π) for 0 < r < a
∂u / ∂θ(r, −π) = ∂u / ∂θ u(r, π) for 0 < r < a
The three conditions listed are all linear and homogeneous, so we can try
separation of variables.

The three conditions under which this problem can be solved should be all linear
and homogenous. And therefore the first step will be separating the variables
We firstly start separating Ansatz which is;
u(r, θ) = R(r)Θ(θ)
We then separate the PDE;
Let’s remember that a positive λ works better in this task than −λ.
Then we get the two resulting ODEs as:
Or
And

In the above equations we have the periodic boundary conditions as;
Θ(−π) = Θ(π), Θ0(−π) = Θ0(π)
And we already know our eigenvalues and Eigen functions:
λ0 = 0, λn = n^2, n = 1, 2, . . .
Θ0(θ) = 1, Θn(θ) = c1 cos nθ + c2 sin nθ, n = 1, 2, . . .
Therefore, by sing the eigenvalues above, we can get;
Before we go further let’s review the heat equations on a disk;
If;
R(r) = r p, then
R’(r) = pr^ p−1 and R’’(r) = p(p − 1)r^ p−2,
so that the CE equation;
will turn to;
And by assuming that r^p 6= 0 we then get the characteristic
equation as ;
p (p − 1) + p – n^2 = 0
⇐⇒ p^2 = n^2

which satisfies p = ±n.
but if n = 0, then the second solution R(r) = ln r is introduced.
Let’s have a look at the two cases.
Case I, n = 0:
The general solution of the dick is of the form;
R(r) = c3 + c4 ln r.
and by imposing the missing BC we get;
ln r → −∞ for r → 0.
This means that R (0) – and thus u (0, θ), and with this conditions,
the temperature at the center of the disk will pile and blow up.
And this is completely not physical and should be prevented from
happening (David W. Hahn, 2012).
We then require a bound temperature at the beginning of the
equation;
|u(0, θ)| < ∞ =
⇒ |R(0)| < ∞.
The above boundary condition will mean that c4 = 0, and
R(r) = c3 = const
For Case 2, n > 0 giving us the general solution of the form;
R(r) = c3r n + c4r −n
And once again we impose the bound temperature condition as,

|R (0) | < ∞.
Remember that;
Thus the above condition means that c4 = 0, and R(r) = c3r n, n
= 1, 2, . . .
Therefore in summary we have up to now;
So we use the boundary temperature distribution u(a, θ) = f(θ) to
determine the coefficients An, Bn:
But from the heat equation we know that the functions (Ozisik,
2013);
{1, cos θ, sin θ, cos 2θ, sin 2θ, . . .}
are orthogonal on the interval [−π, π] (just substitute L = π in our
earlier analysis).
It thus follows as;

Giving the solution;
of the circular disk problem which tells us that the temperature at
the center
of the disk is given by the equation;
Therefore the above equation obeys the rule of Laplace’s
equation which says that the temperature at the center of any
circle inside of which the temperature is harmonic is equal to the
average of the boundary temperature.
This fact is reminiscent of the mean value theorem from calculus
and is therefore called the mean value principle for Laplace’s
equation.
This rule is derived from the mean value theorem from calculus
and is known as mean value principle for Laplace’s equation.

References
David W. Hahn, M. N. (2012). Heat Conduction. New York: John Wiley & Sons.
Ozisik, M. N. (2013). Boundary Value Problems of Heat Conduction. New York:
Courier Corporation.