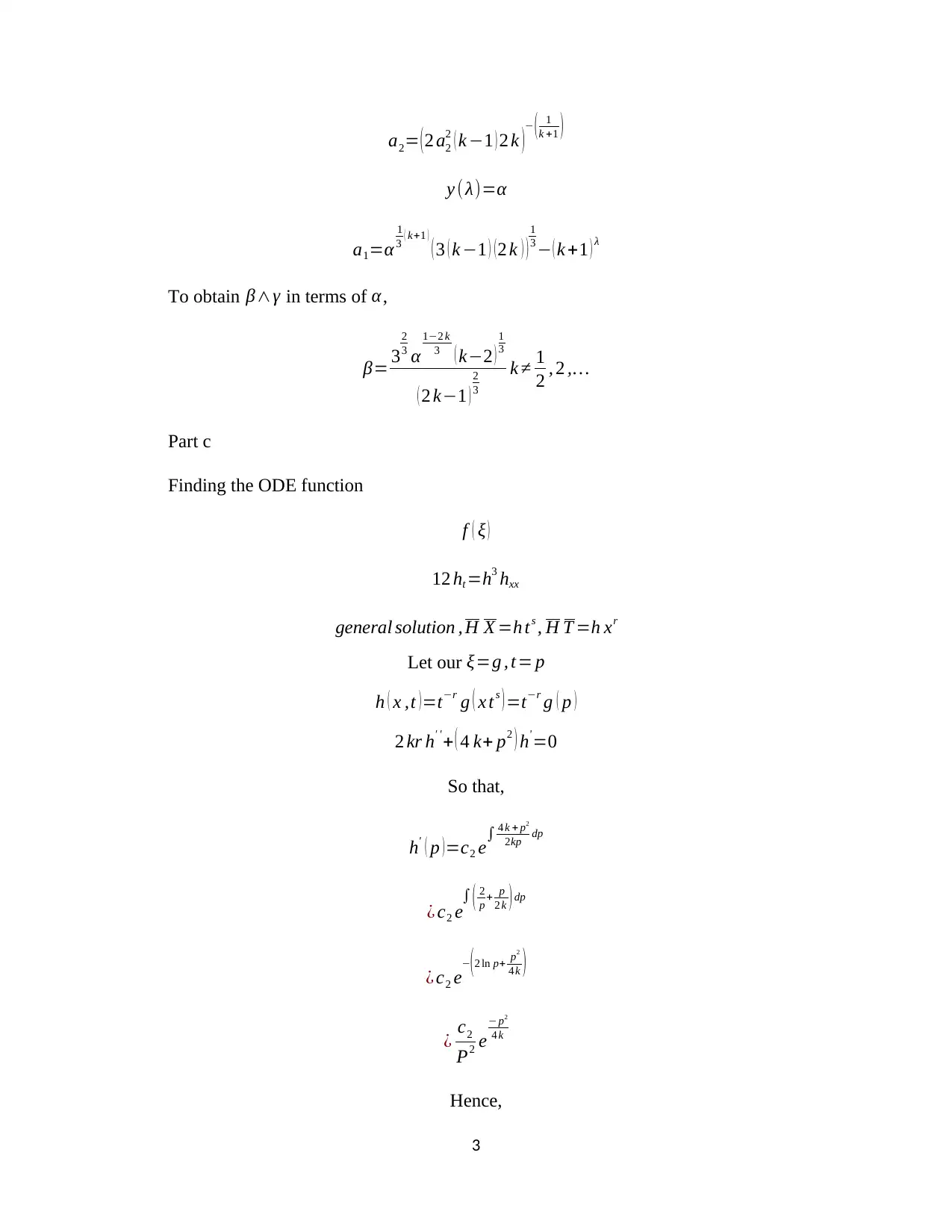
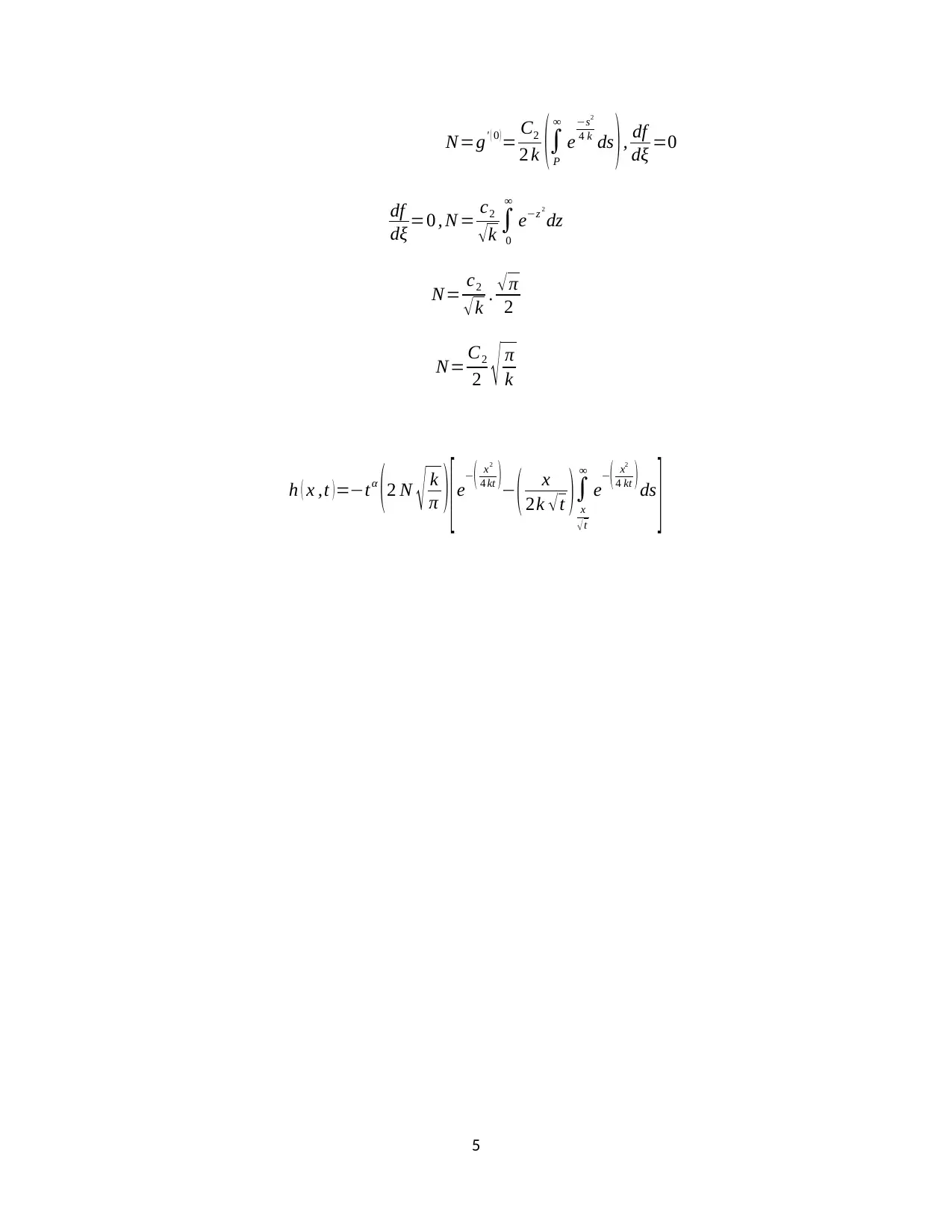
Applied Transport Theory: Deriving Similarity Solution for Spreading of Thin Film

This article explains how to derive a similarity solution for the spreading of a thin film along a horizontal surface in Applied Transport Theory. It covers the relationship between constants, conservation of mass argument, and solving differential equations. The article is relevant for students studying transport theory and related subjects.

2018
INSTITUTIONAL AFFILIATION
FACULTY OR DEPARTMENT
COURSE ID & NAME
TITLE: APPLIED TRANSPORT THEORY
STUDENT NAME
STUDENT ID NUMBER
DATE OF SUBMISSION

If we neglect the effects of surface tension, the governing equation for the spreading of a thin
film along a horizontal surface is (in dimensionless variables)
∂ h
∂ t = ∂
∂ x ( 1
3 h3 ∂ h
∂ x )
Part 1
Derive a similarity solution of the form
h=tα f ( ξ ) where ξ= x
tβ
(a) Determine the relationship between the constants α ∧β
(b) Using a conservation of mass argument, determine another relationship between the
constants α∧β, and thus solve for α ∧β
(c) Use the values of α∧β, write down the ordinary differential equations for f ( ξ )
(d) Solve the differential equation from part c subject to the boundary conditions f=0,
df
dξ =0 , ξ → ∞
SOLUTION
Part a
The similarity solution exists for a given spreading of a thin film to convert the PDE
equation to an ODE for one to obtain the roots,
∂ h
∂ t = ∂
∂ x ( 1
3 h3 ∂ h
∂ x )
Let k = 1
3 , therefore,
∂ h
∂ t = ∂
∂ x ( k h3 ∂ h
∂ x )
Simplifying further,
k
4
∂
∂ x (4 h ∂ h4
∂ x )= 1
12 . ∂2
∂ x2 ( ∂h4
∂ x )
12 ∂ h
∂t = ∂2
∂ x2 ( h4 )
Using the values of h we find,
h=tα f ( ξ ) where ξ= x
tβ
1

12 ∂
∂ t (tα f ( ξ ) )= ∂2
∂ x2 ( tα f ( ξ ) )4
But , ξ= x
t β
12 ∂
∂ t ( tα f ( x
tβ )) = ∂2
∂ x2 ( tα f ( x
tβ ) )
4
Part b
Using the conservation of mass argument,
12 ∂ h
∂t = ∂2
∂ x2 ( h4 )
12 ht =hxx
4
12 ∂ h
∂t =h3 ∂2 h
∂ x2
The operator X =ξ ( x , y ) ∂ x +η( x , y)∂ y generates a point symmetry to determine the
equation,
X |2| ( y!11− y−k ) ¿ y' '− y−k=0
Where,
ζ j ( x , y , y' , … , y j )= d ζ j−1
dx − y j dξ
dx j=1,2,3 …
ζ 2 ¿ y' ' = y−k
1 + kη y−k−1=0
η=3 a1 y
ξ=ao +a1 ( k +1 ) x
The solution yields,
y ( x ) =h ( x , t ) =a2 ( a1 ( k +1 ) x
3
k+ 1 ) k ≠−1
Where a2 is a constant of integration
2

a2= ( 2 a2
2 ( k −1 ) 2 k )
− ( 1
k +1 )
y ( λ)=α
a1=α
1
3 ( k+1 )
( 3 ( k −1 ) (2 k ) )
1
3 − ( k +1 ) λ
To obtain β∧γ in terms of α,
β= 3
2
3 α
1−2 k
3 ( k−2 )
1
3
( 2 k−1 )
2
3
k ≠ 1
2 , 2 ,…
Part c
Finding the ODE function
f ( ξ )
12 ht =h3 hxx
general solution , H X =h ts , H T =h xr
Let our ξ=g , t= p
h ( x ,t )=t−r g ( x ts ) =t−r g ( p )
2 kr h' ' + ( 4 k+ p2 ) h'=0
So that,
h' ( p )=c2 e∫ 4 k + p2
2kp dp
¿ c2 e∫ ( 2
p + p
2 k ) dp
¿ c2 e−(2 ln p+ p2
4 k )
¿ c2
P2 e
− p2
4 k
Hence,
3

h ( ∞ ) −h ( p ) =∫
p
∞ C2
s2 e
−s2
4 k ds
therefore,
h ( p )=M −c2 ( 1
p e−( p2
4 k )− 1
2 k ∫
p
∞
e
− s2
4 k ds )
g ( p )= ( M +C1 ) p−C2 (e
−p 2
4 k −( p
2 k )∫
P
∞
e
−s2
4 k ds )
Part d
Solving the differential equation.
The boundary conditions are f=0, df
dξ =0 , ξ → ∞
Using chain rule,
H X=h ts , H T =h xr
H X=aγ ut tT =aγ −β ut
When γ−β =γ−2 α … PDE under stretching transformation
β=2 α , γ is arbitrary
As a result, aγ u ( aα x ,aβ t )
g ( p )= ( M +C1 ) p−C2 (e
−p 2
4 k −( p
2 k )∫
P
∞
e
−s2
4 k ds )
M +C1 =0 , as f =0
g ( p )=−C2 (e
− p2
4 k − ( p
2k )∫
P
∞
e
−s2
4 k ds )
In addition,
4

N=g' ( 0 ) = C2
2 k (∫
P
∞
e
−s2
4 k ds ) , df
dξ =0
df
dξ =0 , N = c2
√ k ∫
0
∞
e−z 2
dz
N= c2
√k . √ π
2
N= C2
2 √ π
k
h ( x ,t )=−tα
(2 N √ k
π ) [e−( x2
4 kt )− ( x
2k √t )∫
x
√t
∞
e−( x2
4 kt ) ds
]
5