Spherical Star Flux and Surface Temperature, Angular Resolution and Mass Calculation, Eclipse of Stars

This document discusses topics such as spherical star flux and surface temperature, angular resolution and mass calculation, and eclipse of stars. It includes formulas and calculations for each topic. The subject is ASTR364 and the document type is a solved assignment.

ASTR364
[Document subtitle]
[DATE]

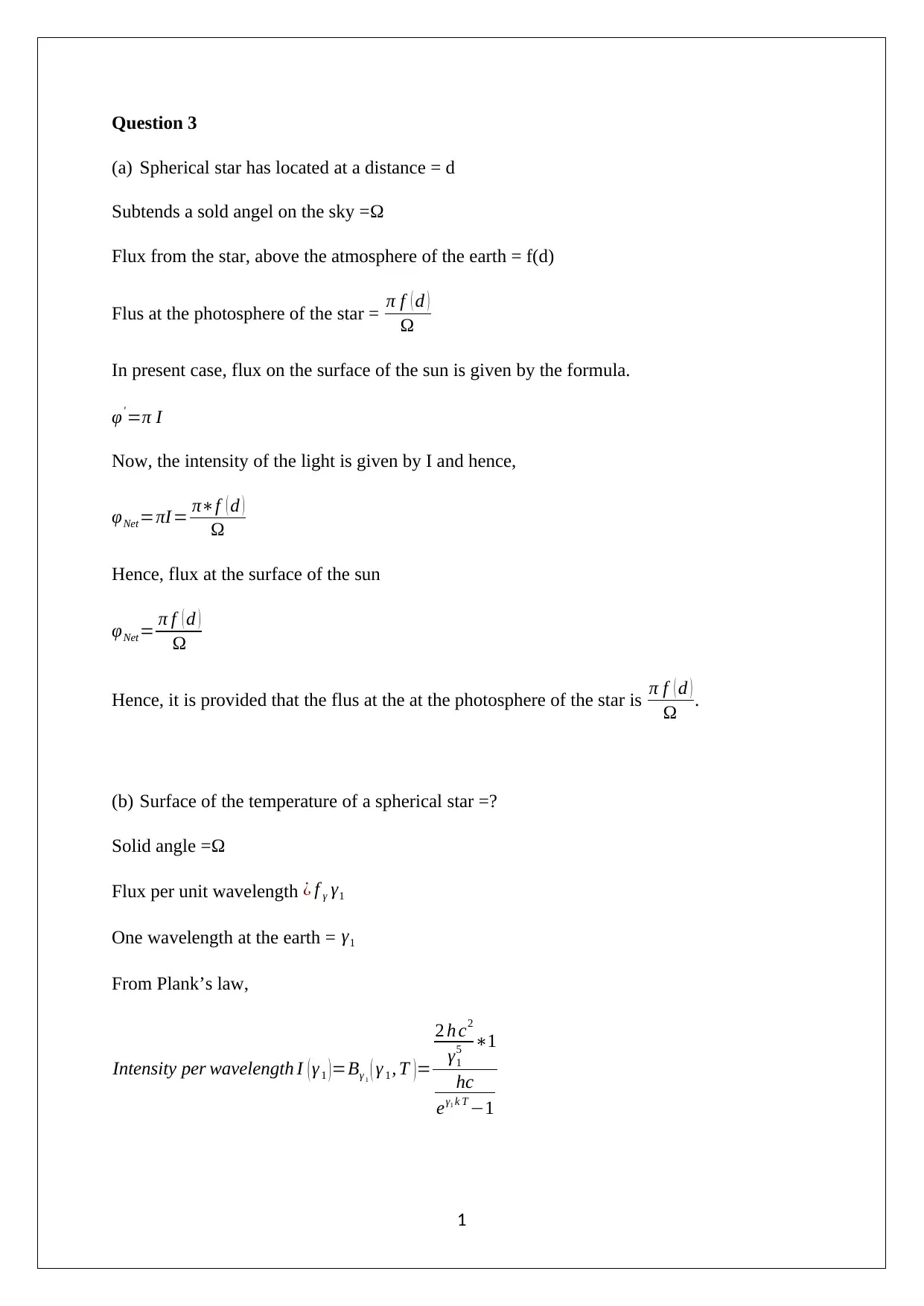
Question 3
(a) Spherical star has located at a distance = d
Subtends a sold angel on the sky =Ω
Flux from the star, above the atmosphere of the earth = f(d)
Flus at the photosphere of the star = π f ( d )
Ω
In present case, flux on the surface of the sun is given by the formula.
φ' =π I
Now, the intensity of the light is given by I and hence,
φNet=πI = π∗f ( d )
Ω
Hence, flux at the surface of the sun
φNet= π f ( d )
Ω
Hence, it is provided that the flus at the at the photosphere of the star is π f ( d )
Ω .
(b) Surface of the temperature of a spherical star =?
Solid angle =Ω
Flux per unit wavelength ¿ f γ γ1
One wavelength at the earth = γ1
From Plank’s law,
Intensity per wavelength I ( γ 1 )=Bγ 1 ( γ 1 , T )=
2 h c2
γ1
5 ∗1
hc
eγ1 k T −1
1

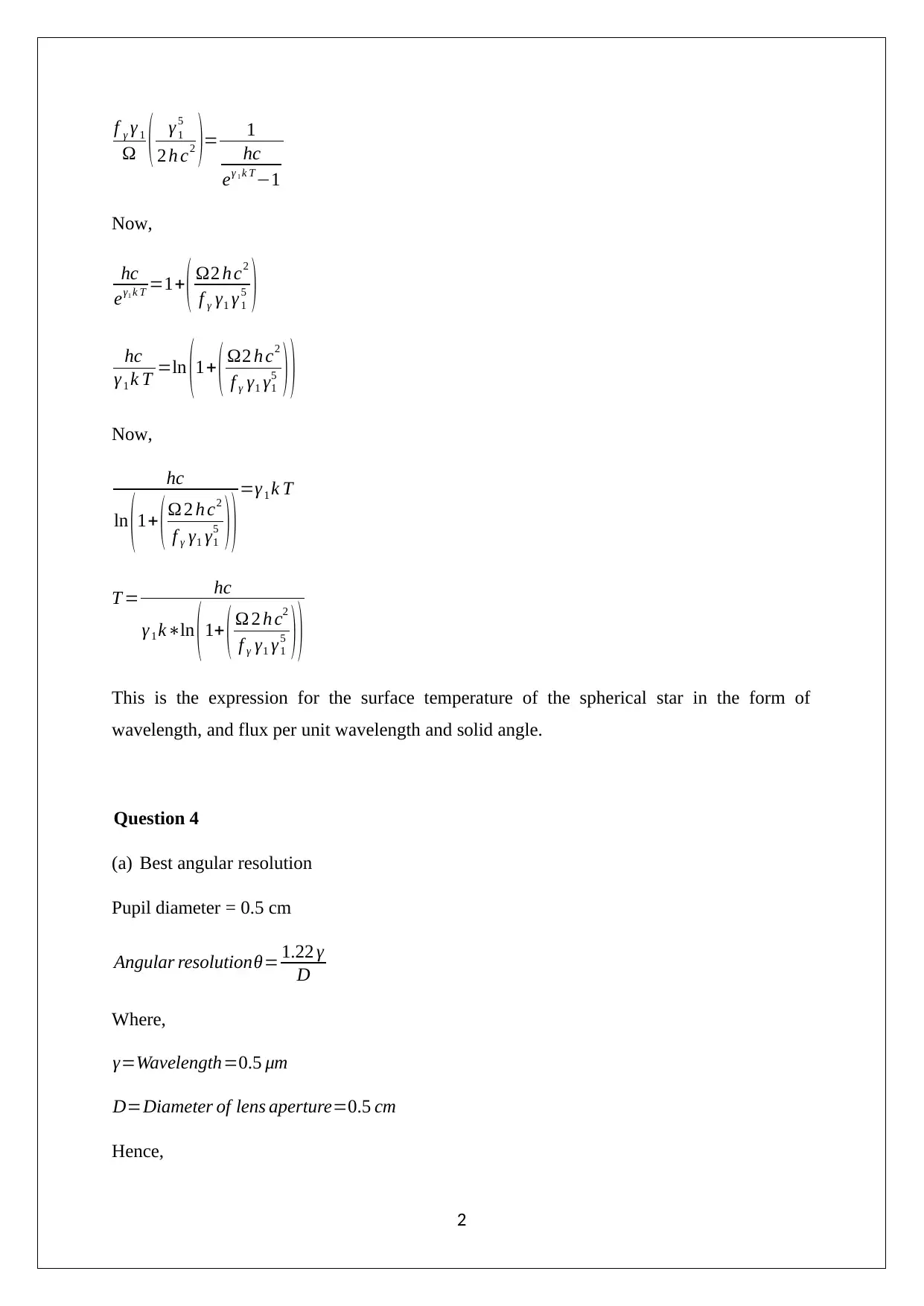
f γ γ 1
Ω ( γ 1
5
2 h c2 )= 1
hc
eγ 1 k T−1
Now,
hc
eγ1 k T =1+ ( Ω2 h c2
f γ γ1 γ 1
5 )
hc
γ 1 k T =ln ( 1+ ( Ω2 h c2
f γ γ1 γ1
5 ) )
Now,
hc
ln (1+ (Ω 2 h c2
f γ γ1 γ1
5 ) )=γ 1 k T
T = hc
γ 1 k∗ln ( 1+ ( Ω 2 h c2
f γ γ1 γ1
5 ) )
This is the expression for the surface temperature of the spherical star in the form of
wavelength, and flux per unit wavelength and solid angle.
Question 4
(a) Best angular resolution
Pupil diameter = 0.5 cm
Angular resolutionθ= 1.22 γ
D
Where,
γ=Wavelength=0.5 μm
D=Diameter of lens aperture=0.5 cm
Hence,
2

θ=1.22 γ
D =1.22∗5∗10−7
5∗10−3 =1.22∗10−4 radians∨0.42 arc minute
(b) Angular resolution of the Hubble Space Telescope
Aperture diameter = 2.4 m at 0.50μm
Angular resolutionθ= 1.22 γ
D
Where,
γ=Wavelength=0.5 μm
D=Diameter of lens aperture=2.4 m
Hence,
θ=1.22 γ
D =1.22∗5∗10−7
2.4 =8.7∗10− 4 arc minute
(c) Angular resolution as a fraction of an arcsecond of VLBI
Effective aperture diameter = 8600 km at a frequency 86 GHz
86 GHz frequency to wavelength = 0.003488 m
Angular resolutionθ= 1.22 γ
D
Where,
γ=Wavelength=0.003488 m
D=Diameter of lens aperture=8600 km
Hence,
θ=1.22 γ
D =1.22∗0.003488
8600∗103 =4.948∗10−10 degree
Question 5
3

(a) It is apparent that the orbital velocities are basically the ratio of the masses.
Here,
v 1=20 km/sec
v 2=5 km/sec
v 1
v 2 = m1
m 2 =20
5 = 4
1 … … … … .(1)
From, Kepler’s third law
m1+m2= P
4 π G ( v 1+ v 2 )3
From equation 1
5 m2= P
4 π G ( v 1+ v 2 ) 3
m 2= P
4 π G∗5 ( v 1+ v 2 )3
P=5 years=5∗365 days= ( 5∗365∗865400 ) sec
G=6.67∗10−11 NM
m 2= ( 5∗365∗865400 )
4 π 6.67∗10−11∗5 ( 20∗103 +5∗103 ) 3
m 2=1.1542∗1033 kg
Mass of sun = 1.99∗1033kg and hence,
Hence, m2 can be written in the form of Mass of sun i.e. M(sun)
m 2=0.58 M ( sun )
m1=4 m2=2.32 M ( sun)
Hence, the mass of each star is 2.32 M(Sun) and 0.58 M(sun).
4

(b) It is given that smaller star would take =1+0.3 days to cross the bigger star and hence, the
distance would be same as its diameter. Therefore, it can be concluded that the diameter
of the bigger star would be same as the formula shown below.
Diameter of bigger star = Relative velocity* Time taken
Diameter of the bigger star = 25*1.3*24*3600 = 4 r(Sun)
Hence, the radius of the bigger star would be 2 r (Sun).
Now, the time taken to reach the eclipse is 0.3 days and hence, the expression can be derived
to find the radius of the small sta.
Radius of the small star = Velocity* time = 25 *0.3 *24*3600 = 0.46 r(Sun)
Hence, the radius of small star is 0.46 r (Sun) and radius of bigger star is 2 r (Sun).
5

6