Biostatistics Assignment 3: Paired t-test, Independent t-test and LOS Analysis

This assignment covers paired t-test for Reiki treatment impact, independent t-test for Cholesterol levels and LOS analysis for different living conditions. Average LOS for home alone patients, for patients staying at home with spouse, and for nursing home stay were evaluated and tabled from EXCEL output. The LOS for three living levels were plotted in a side-by-side box plot. Get solved assignments, essays, dissertation and more at Desklib.

1
Introduction to Biostatistics (Assignment 3)
Introduction to Biostatistics
Assignment 3

2
Introduction to Biostatistics (Assignment 3)
Question 1
a) Paired t-test was performed to investigate the effect of Reiki treatment impact on the VAS scores
as follows.
For the VAS scale population, μVA and μVB were taken as the average of visual analogue scale
for pre and post conditions of Reiki treatment. The test was conducted in EXCEL and the output
has been used for hypothesis testing
The hypotheses for the paired t-test were constructed as below.
Null hypothesis: H0: ( μVB−μVA ) =0
Alternate hypothesis: HA: ( μVB−μVA ) ≠0
Level of significance for the test was considered at 5% ( α=0 . 05 ).
The t-statistic was calculated as
t= estimated difference−hypothesized difference
s tan dard error = 1. 95−0
0 . 4 =4 .87
for 19 degrees of freedom.
The p-value was found to be P (|t|> 4 . 87 ) =0. 000 (from EXCEL output)
The p-value implied that at 5% level of significance the null hypothesis could be rejected against
the alternate hypothesis. It could be concluded that the differences in scores were significant (t =
4.87, p < 0.05) at 5% level and average VAS score was better after the Reiki treatment.
The confidence interval for the difference in VAS scores was evaluated as
Xd
−
±t∗s tan dard error ( s
√ n ) =[ 1. 11 ,2 .79 ]
(from EXCEL output). With 95% confidence, it was
estimated that the population mean difference would be within the limits of the confidence
interval. But, the hypothesized mean difference of VAS scores was outside the interval. Hence,
decision of rejecting the null hypothesis was justified.

3
Introduction to Biostatistics (Assignment 3)
For the Likert scale population, μLA and μLB were taken as the average of visual analogue
scale for pre and post conditions of Reiki treatment. The test was conducted in EXCEL and the
output has been used for hypothesis testing (Sausen et al., 2015)
The hypotheses for the paired t-test were constructed as below.
Null hypothesis: H0: ( μLB−μLA ) =0
Alternate hypothesis: HA: ( μLB−μLA ) ≠0
Level of significance for the test was considered at 5% ( α=0 . 05 ).
The t-statistic was calculated as
t= estimated difference−hypothesized difference
s tan dard error = 1. 18−0
0. 18 =6 .29
for ( n−1 ) =20−1= 19 degrees of freedom, where n was the number of observations.
The p-value was found to be P (|t|>6 . 29 ) =0. 000 (from EXCEL output)
The p-value implied that at 5% level of significance the null hypothesis could be rejected against
the alternate hypothesis. It could be concluded that the differences in scores were significant (t =
6.29, p < 0.05) at 5% level and average Likert score was better after the Reiki treatment.
The confidence interval for the difference in Likert scores was evaluated as
Xd
−
±t∗s tan dard error =[ 0. 801 , 1. 549 ] (from EXCEL output). With 95% confidence, it was
estimated that the population mean difference would be within the limits of the confidence
interval. But, the hypothesized mean difference of Likert scores was outside the interval. Hence,
decision of rejecting the null hypothesis was justified.

4
Introduction to Biostatistics (Assignment 3)
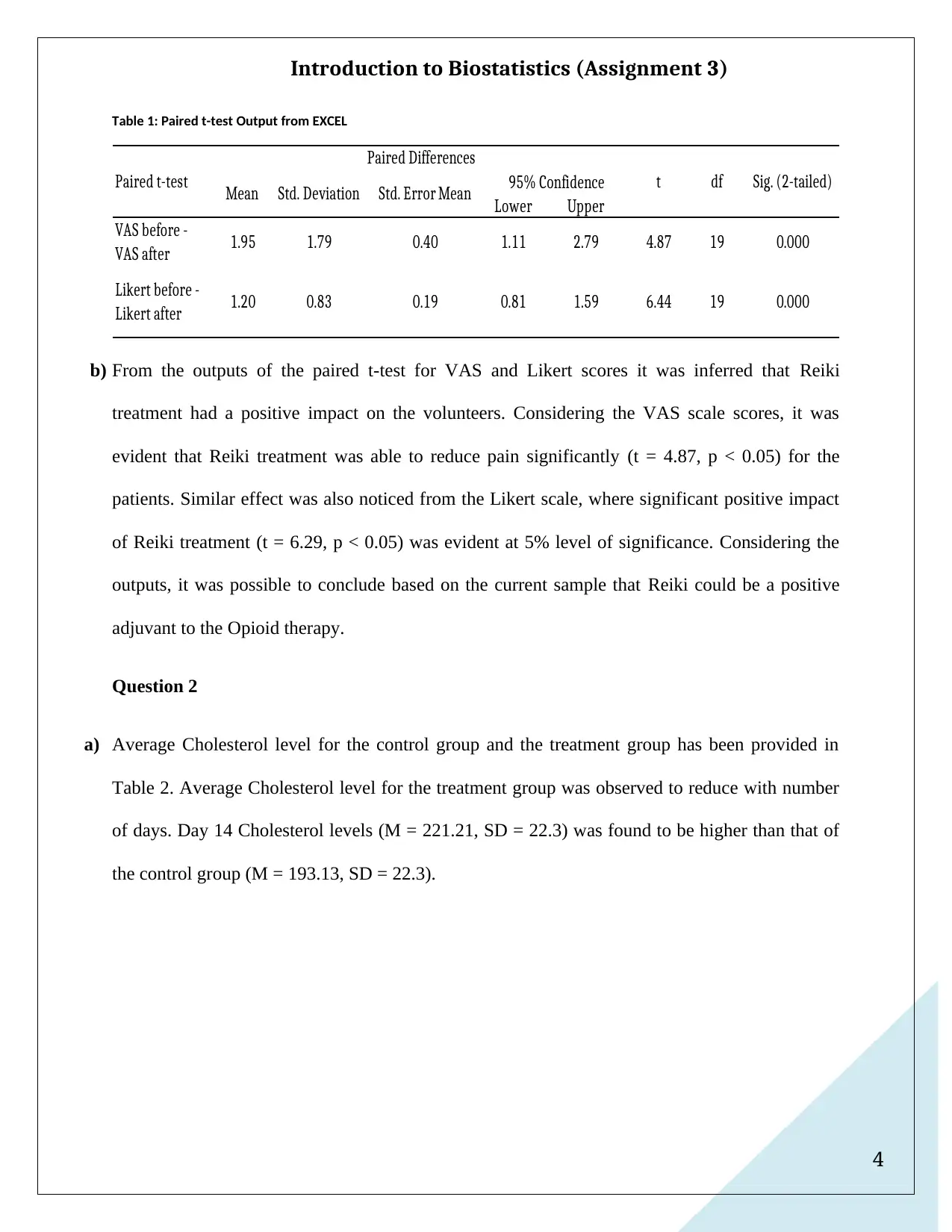
Table 1: Paired t-test Output from EXCEL
Lower Upper
VAS before -
VAS after 1.95 1.79 0.40 1.11 2.79 4.87 19 0.000
Likert before -
Likert after 1.20 0.83 0.19 0.81 1.59 6.44 19 0.000
Paired t-test
Paired Differences
t df Sig. (2-tailed)
Mean Std. Deviation Std. Error Mean 95% Confidence
b) From the outputs of the paired t-test for VAS and Likert scores it was inferred that Reiki
treatment had a positive impact on the volunteers. Considering the VAS scale scores, it was
evident that Reiki treatment was able to reduce pain significantly (t = 4.87, p < 0.05) for the
patients. Similar effect was also noticed from the Likert scale, where significant positive impact
of Reiki treatment (t = 6.29, p < 0.05) was evident at 5% level of significance. Considering the
outputs, it was possible to conclude based on the current sample that Reiki could be a positive
adjuvant to the Opioid therapy.
Question 2
a) Average Cholesterol level for the control group and the treatment group has been provided in
Table 2. Average Cholesterol level for the treatment group was observed to reduce with number
of days. Day 14 Cholesterol levels (M = 221.21, SD = 22.3) was found to be higher than that of
the control group (M = 193.13, SD = 22.3).

5
Introduction to Biostatistics (Assignment 3)
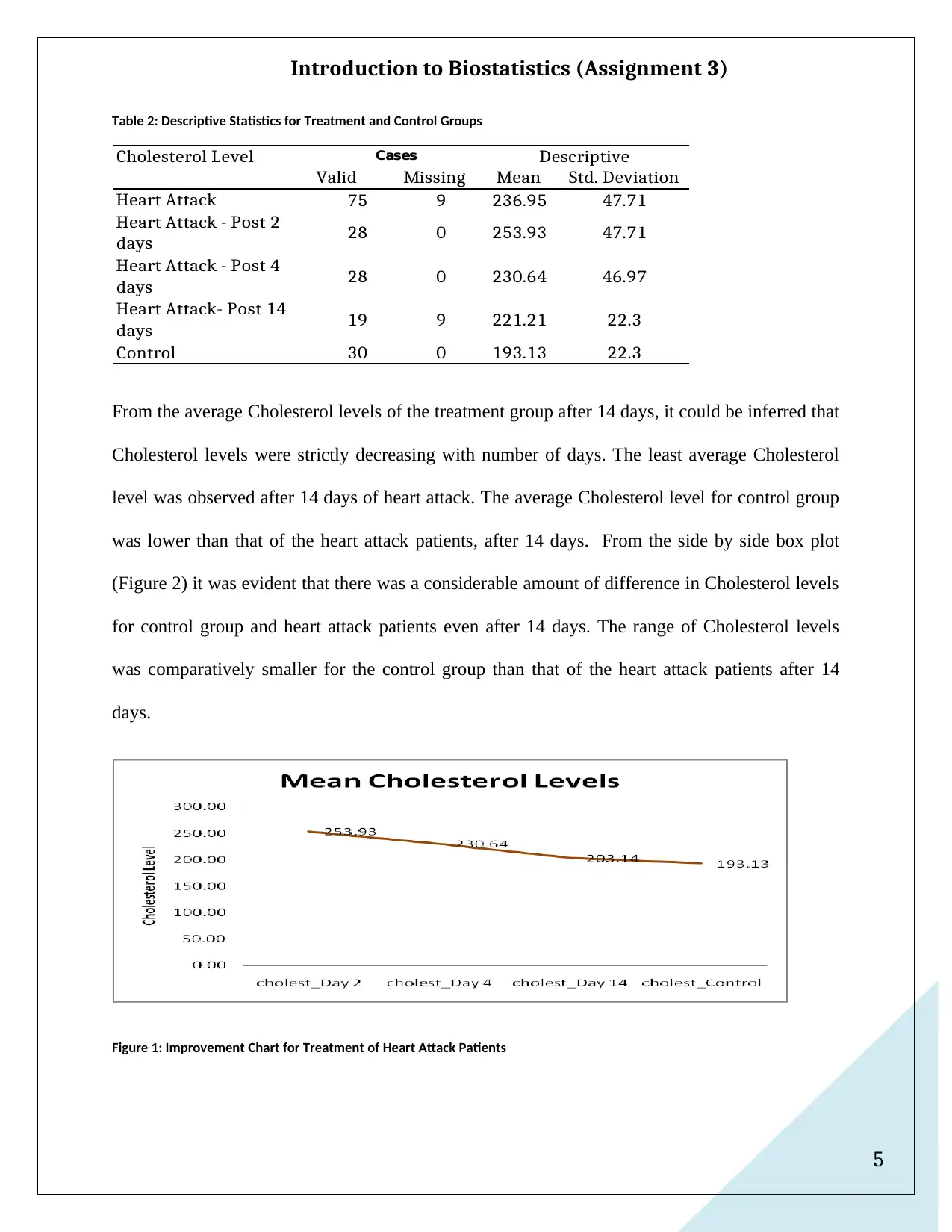
Table 2: Descriptive Statistics for Treatment and Control Groups
Valid Missing Mean Std. Deviation
Heart Attack 75 9 236.95 47.71
Heart Attack - Post 2
days 28 0 253.93 47.71
Heart Attack - Post 4
days 28 0 230.64 46.97
Heart Attack- Post 14
days 19 9 221.21 22.3
Control 30 0 193.13 22.3
Cholesterol Level Cases Descriptive
From the average Cholesterol levels of the treatment group after 14 days, it could be inferred that
Cholesterol levels were strictly decreasing with number of days. The least average Cholesterol
level was observed after 14 days of heart attack. The average Cholesterol level for control group
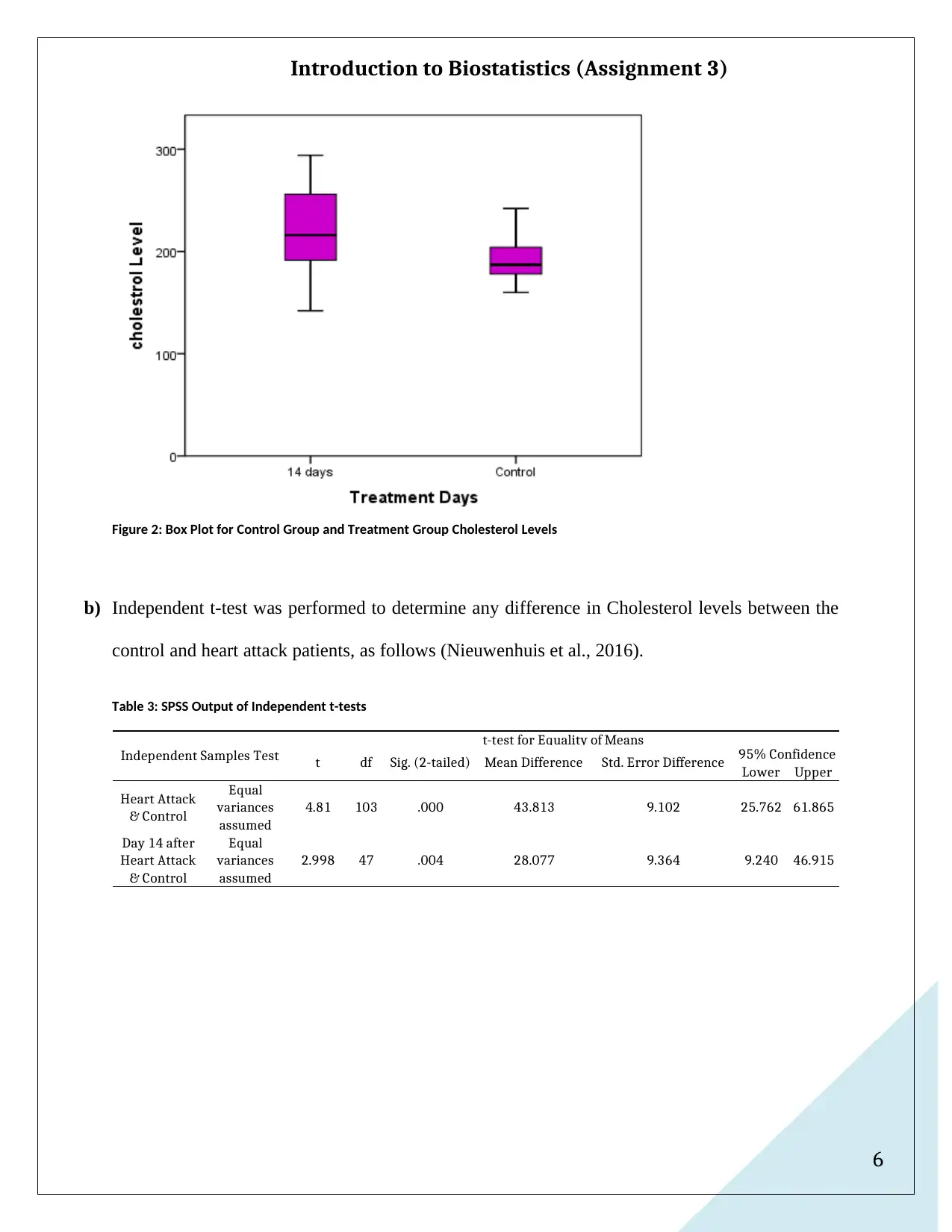
was lower than that of the heart attack patients, after 14 days. From the side by side box plot
(Figure 2) it was evident that there was a considerable amount of difference in Cholesterol levels
for control group and heart attack patients even after 14 days. The range of Cholesterol levels
was comparatively smaller for the control group than that of the heart attack patients after 14
days.
Figure 1: Improvement Chart for Treatment of Heart Attack Patients

6
Introduction to Biostatistics (Assignment 3)
Figure 2: Box Plot for Control Group and Treatment Group Cholesterol Levels
b) Independent t-test was performed to determine any difference in Cholesterol levels between the
control and heart attack patients, as follows (Nieuwenhuis et al., 2016).
Table 3: SPSS Output of Independent t-tests
Lower Upper
Heart Attack
& Control
Equal
variances
assumed
4.81 103 .000 43.813 9.102 25.762 61.865
Day 14 after
Heart Attack
& Control
Equal
variances
assumed
2.998 47 .004 28.077 9.364 9.240 46.915
Std. Error Difference 95% ConfidenceIndependent Samples Test
t-test for Equality of Means
t df Sig. (2-tailed) Mean Difference

7
Introduction to Biostatistics (Assignment 3)
For the Cholesterol levels, μH and μC were taken as the average of Cholesterol levels for heart
attack patients and control group. The test was conducted in SPSS and the output has been used
for hypothesis testing
The hypotheses for the paired t-test were constructed as below.
Null hypothesis: H0: μH - μC = 0
Alternate hypothesis: HA: μH - μC≠0
Level of significance for the test was considered at 5% ( α=0 . 05 ).
The t-statistic was calculated as
t= estimated difference−hypothesized difference
s tandard error =43. 81−0
9 .1 =4 . 81 for 103 degrees of freedom.
The p-value was found to be P (|t|> 4 . 81 ) =0 . 000 (from SPSS output)
The p-value implied that at 5% level of significance the null hypothesis could be rejected against
the alternate hypothesis. It could be concluded that the differences in scores were significant (t =
4.81, p < 0.05) at 5% level and average Cholesterol levels was lower in control patients
compared to heart attack patients.
The confidence interval for the difference in Cholesterol levels was evaluated as
Xd
−
±t∗s tan dard error =[ 25. 76 , 61. 86 ] (from SPSS output). With 95% confidence, it was
estimated that the population mean difference would be within the limits of the confidence
interval. But, the hypothesized zero mean difference of Cholesterol levels, was outside the
interval. Hence, decision of rejecting the null hypothesis was justified, and Cholesterol levels
were lower in control group compared to Cholesterol levels of heart attack patients.

8
Introduction to Biostatistics (Assignment 3)
Now, especially for the Cholesterol levels in heart attack patients after 14 days, μ14 and μC
were taken as the average of Cholesterol levels for heart attack patients and control group. The
test was conducted in SPSS and the output has been used for hypothesis testing
The hypotheses for the paired t-test were constructed as below.
Null hypothesis: H0: μ14 - μC = 0
Alternate hypothesis: HA: μ14 - μC≠0
Level of significance for the test was considered at 5% ( α=0 . 05 ).
The t-statistic was calculated as
t= estimated difference−hypothesized difference
s tandard error =28 . 07−0
9 .36 =2 . 998 for 103 degrees of freedom.
The p-value was found to be P (|t|>2 . 998 ) =0 . 004 (from SPSS output)
The p-value implied that at 5% level of significance the null hypothesis could be rejected against
the alternate hypothesis. It could be concluded that the differences in scores were significant (t =
2.99, p < 0.05) at 5% level and average Cholesterol levels was lower in control patients
compared to heart attack patients after 14 days.
The confidence interval for the difference in Cholesterol levels was evaluated as
Xd
−
±t∗s tan dard error =[ 9 .24 , 46 .91 ] (from SPSS output). With 95% confidence, it was estimated
that the population mean difference would be within the limits of the confidence interval. But,
the hypothesized zero mean difference of Cholesterol levels, was outside the interval. Hence,
decision of rejecting the null hypothesis was justified, and Cholesterol levels were lower in
control group compared to Cholesterol levels of heart attack patients after 14 days

9
Introduction to Biostatistics (Assignment 3)
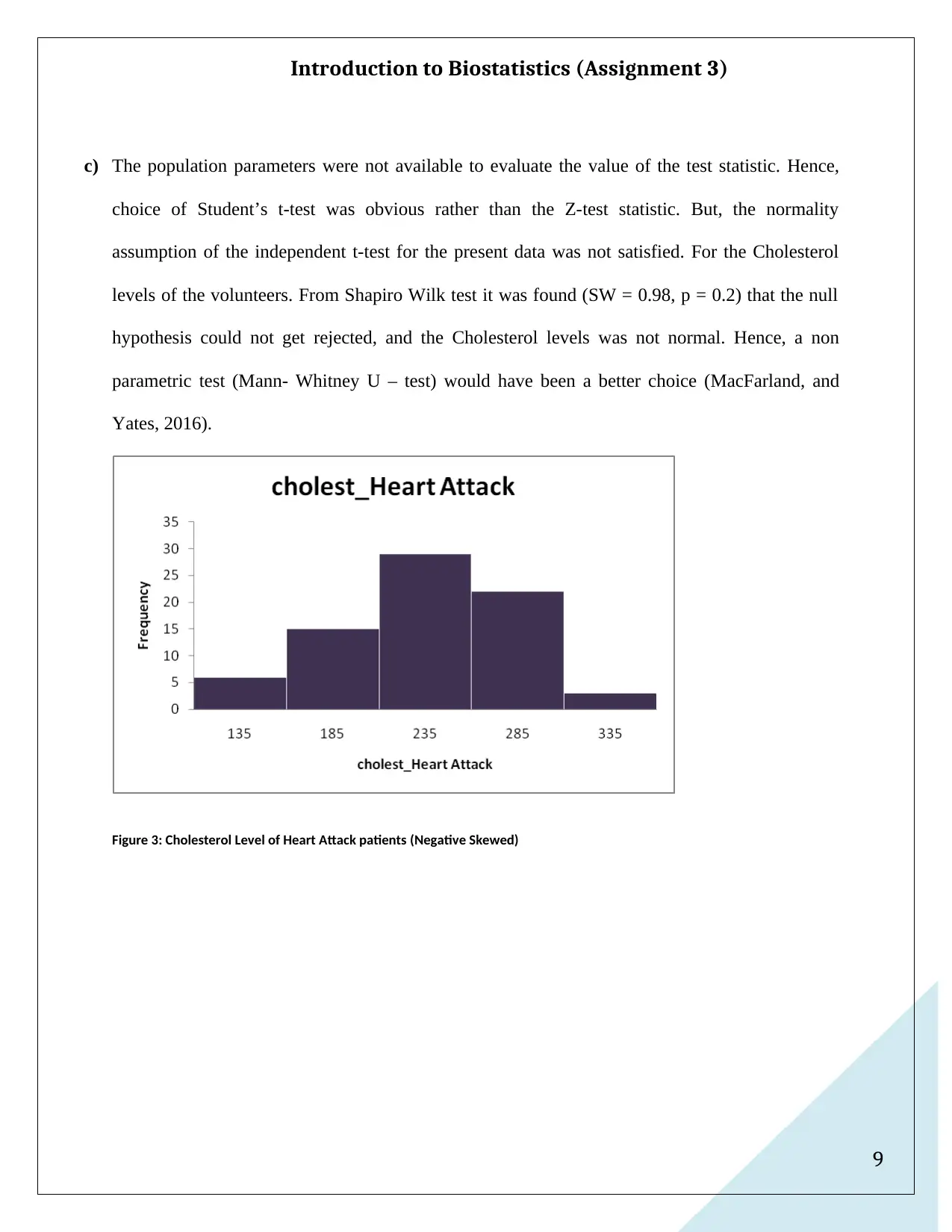
c) The population parameters were not available to evaluate the value of the test statistic. Hence,
choice of Student’s t-test was obvious rather than the Z-test statistic. But, the normality
assumption of the independent t-test for the present data was not satisfied. For the Cholesterol
levels of the volunteers. From Shapiro Wilk test it was found (SW = 0.98, p = 0.2) that the null
hypothesis could not get rejected, and the Cholesterol levels was not normal. Hence, a non
parametric test (Mann- Whitney U – test) would have been a better choice (MacFarland, and
Yates, 2016).
Figure 3: Cholesterol Level of Heart Attack patients (Negative Skewed)

10
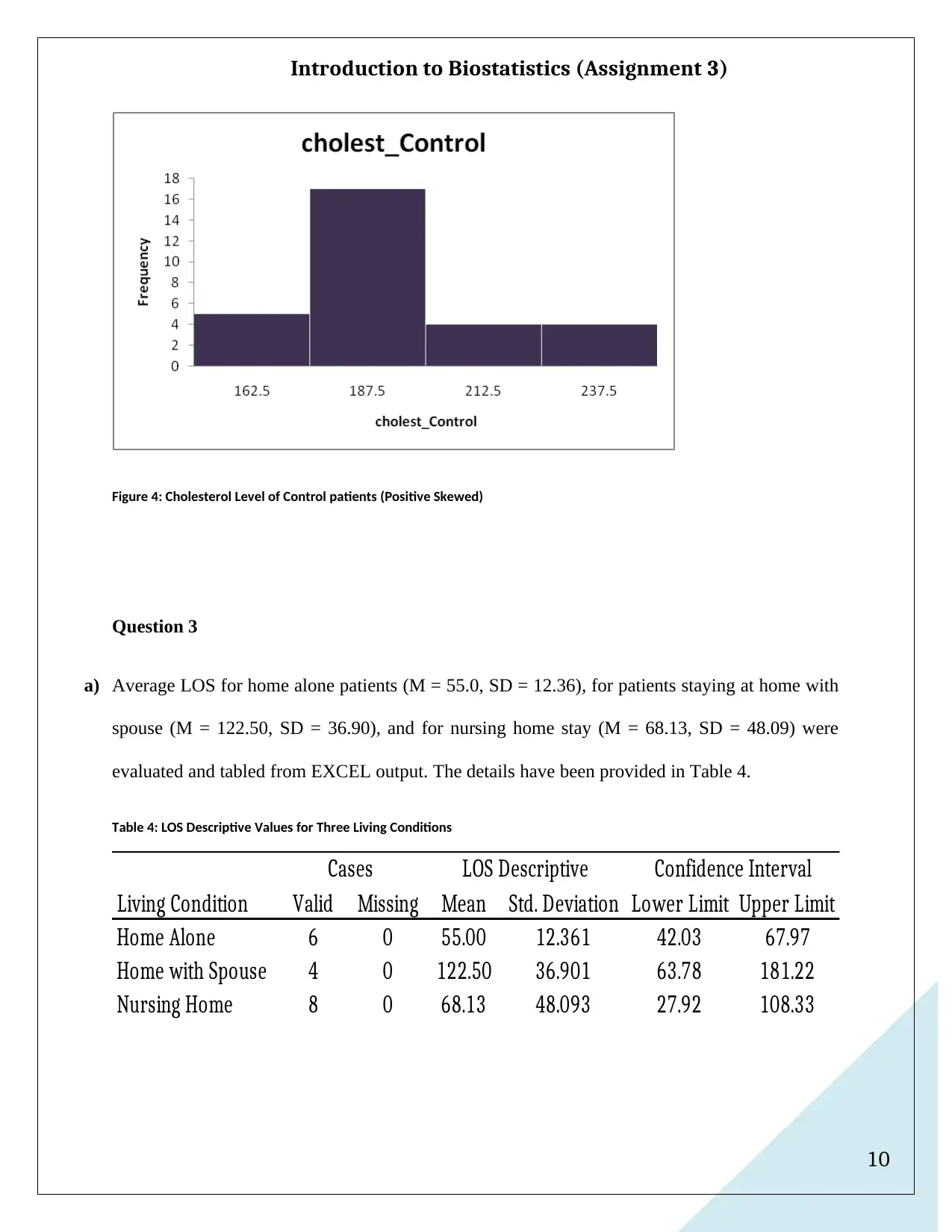
Introduction to Biostatistics (Assignment 3)
Figure 4: Cholesterol Level of Control patients (Positive Skewed)
Question 3
a) Average LOS for home alone patients (M = 55.0, SD = 12.36), for patients staying at home with
spouse (M = 122.50, SD = 36.90), and for nursing home stay (M = 68.13, SD = 48.09) were
evaluated and tabled from EXCEL output. The details have been provided in Table 4.
Table 4: LOS Descriptive Values for Three Living Conditions
Living Condition Valid Missing Mean Std. Deviation Lower Limit Upper Limit
Home Alone 6 0 55.00 12.361 42.03 67.97
Home with Spouse 4 0 122.50 36.901 63.78 181.22
Nursing Home 8 0 68.13 48.093 27.92 108.33
Cases LOS Descriptive Confidence Interval

11
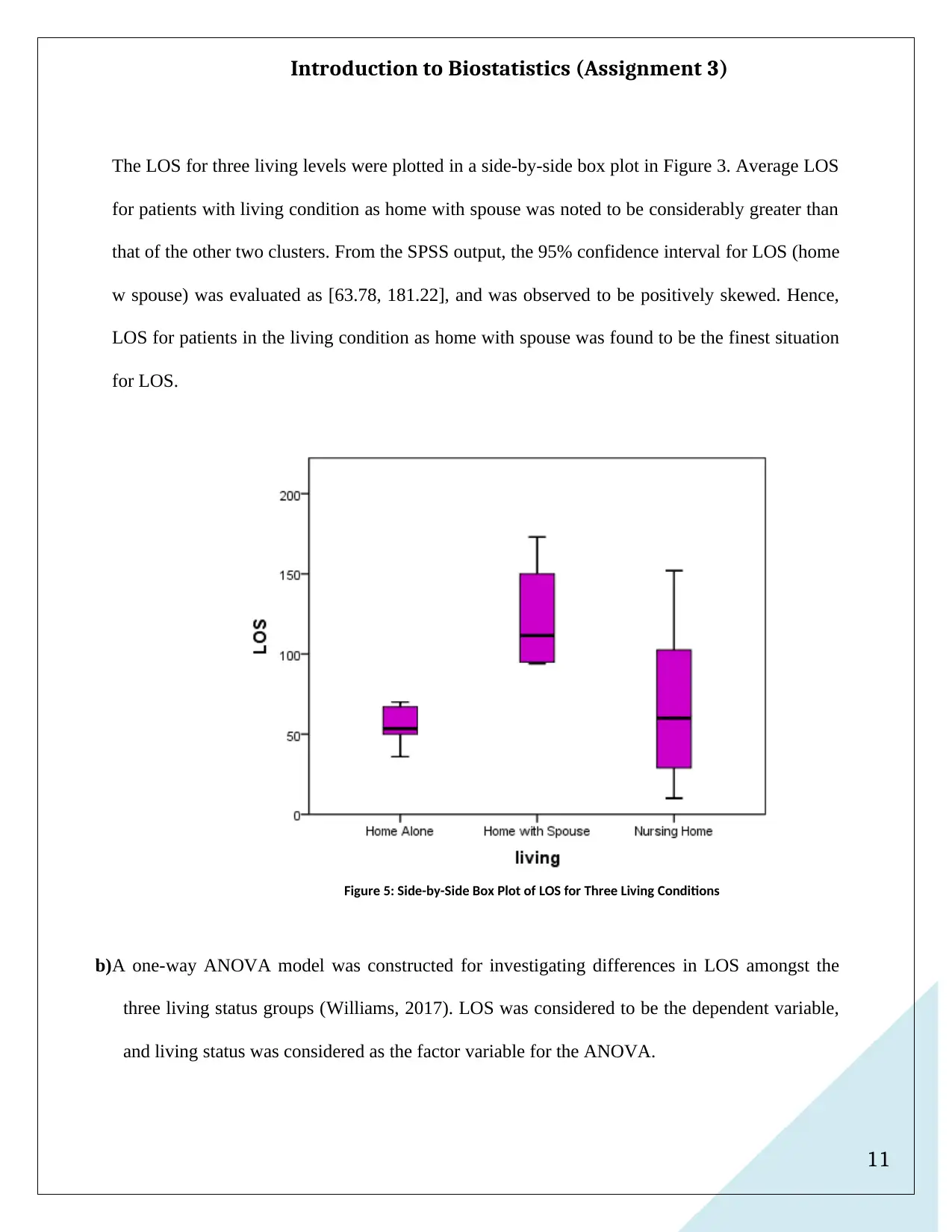
Introduction to Biostatistics (Assignment 3)
The LOS for three living levels were plotted in a side-by-side box plot in Figure 3. Average LOS
for patients with living condition as home with spouse was noted to be considerably greater than
that of the other two clusters. From the SPSS output, the 95% confidence interval for LOS (home
w spouse) was evaluated as [63.78, 181.22], and was observed to be positively skewed. Hence,
LOS for patients in the living condition as home with spouse was found to be the finest situation
for LOS.
Figure 5: Side-by-Side Box Plot of LOS for Three Living Conditions
b)A one-way ANOVA model was constructed for investigating differences in LOS amongst the
three living status groups (Williams, 2017). LOS was considered to be the dependent variable,
and living status was considered as the factor variable for the ANOVA.

12
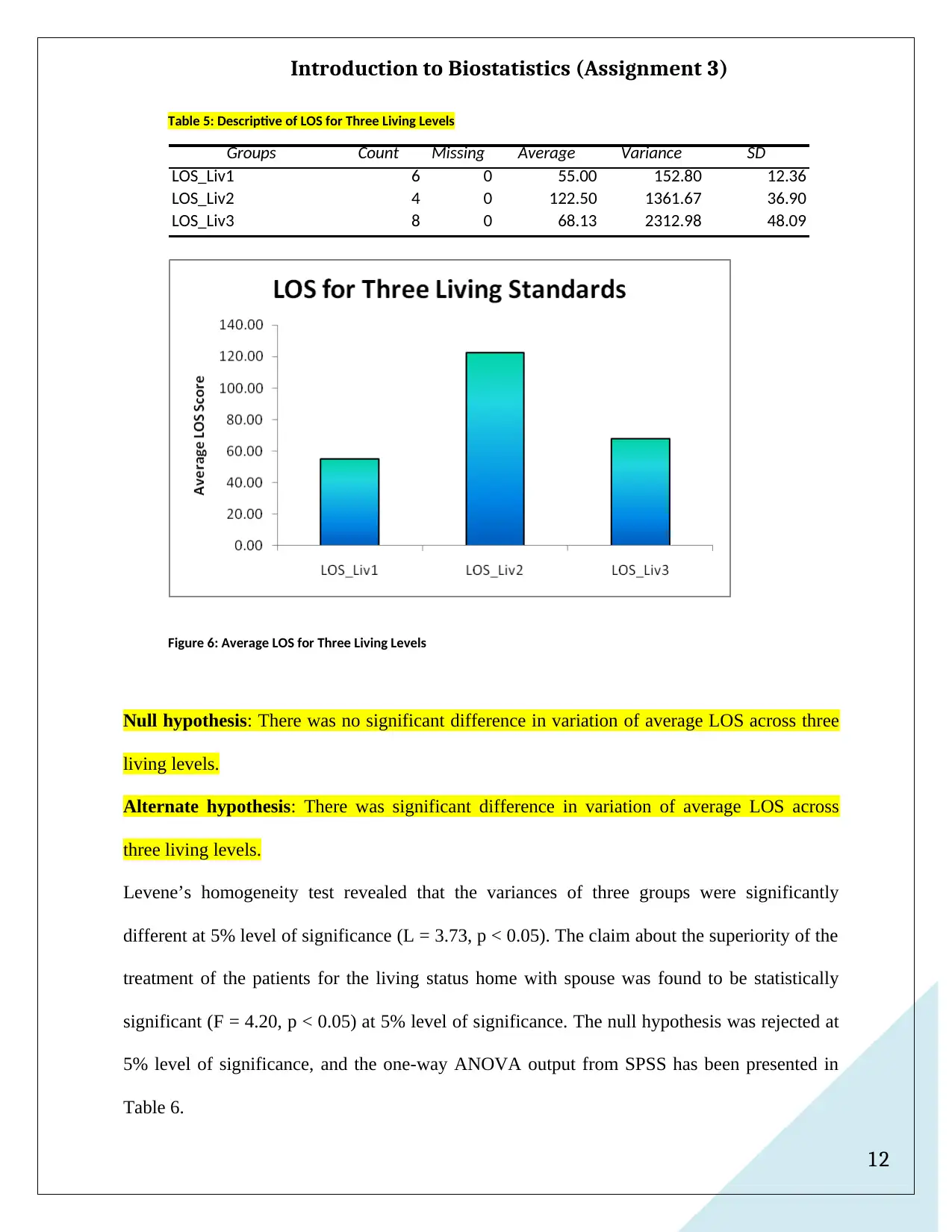
Introduction to Biostatistics (Assignment 3)
Table 5: Descriptive of LOS for Three Living Levels
Groups Count Missing Average Variance SD
LOS_Liv1 6 0 55.00 152.80 12.36
LOS_Liv2 4 0 122.50 1361.67 36.90
LOS_Liv3 8 0 68.13 2312.98 48.09
Figure 6: Average LOS for Three Living Levels
Null hypothesis: There was no significant difference in variation of average LOS across three
living levels.
Alternate hypothesis: There was significant difference in variation of average LOS across
three living levels.
Levene’s homogeneity test revealed that the variances of three groups were significantly
different at 5% level of significance (L = 3.73, p < 0.05). The claim about the superiority of the
treatment of the patients for the living status home with spouse was found to be statistically
significant (F = 4.20, p < 0.05) at 5% level of significance. The null hypothesis was rejected at
5% level of significance, and the one-way ANOVA output from SPSS has been presented in
Table 6.

13
Introduction to Biostatistics (Assignment 3)
Table 6: One-Way ANOVA for Comparing the Three Living Status
ANOVA Sum of
Squares df Mean
Square F Sig.
Between
Groups 11790.63 2 5895.31 4.20 .036
Within
Groups 21039.88 15 1402.66
Total 32830.50 17
From post hoc analysis by Tukey HSD test, it was revealed from pair wise comparison that
mean difference (MD) of LOS for home alone, and home with spouse living status was
significantly different (MD = -67.5, p < 0.05) at 5% level of significance (Julkowska et al.,
2018).
c) Kruskal-Wallis test was used as a non-parametric test to find out the existence of difference in
mean LOS for the three living status.
The test was conducted in EXCEL and SPSS and the outputs have been used for hypothesis
testing.
The hypotheses for the Kruskal-Wallis test were constructed as below.
Null hypothesis: H0: Distribution of LOS for the three living status were identical
Alternate hypothesis: HA: At least, LOS for one of the three living status would yield larger
parameter values compared to other two living status groups.
Level of significance for the test was considered at 5% ( α=0 . 05 ).

14
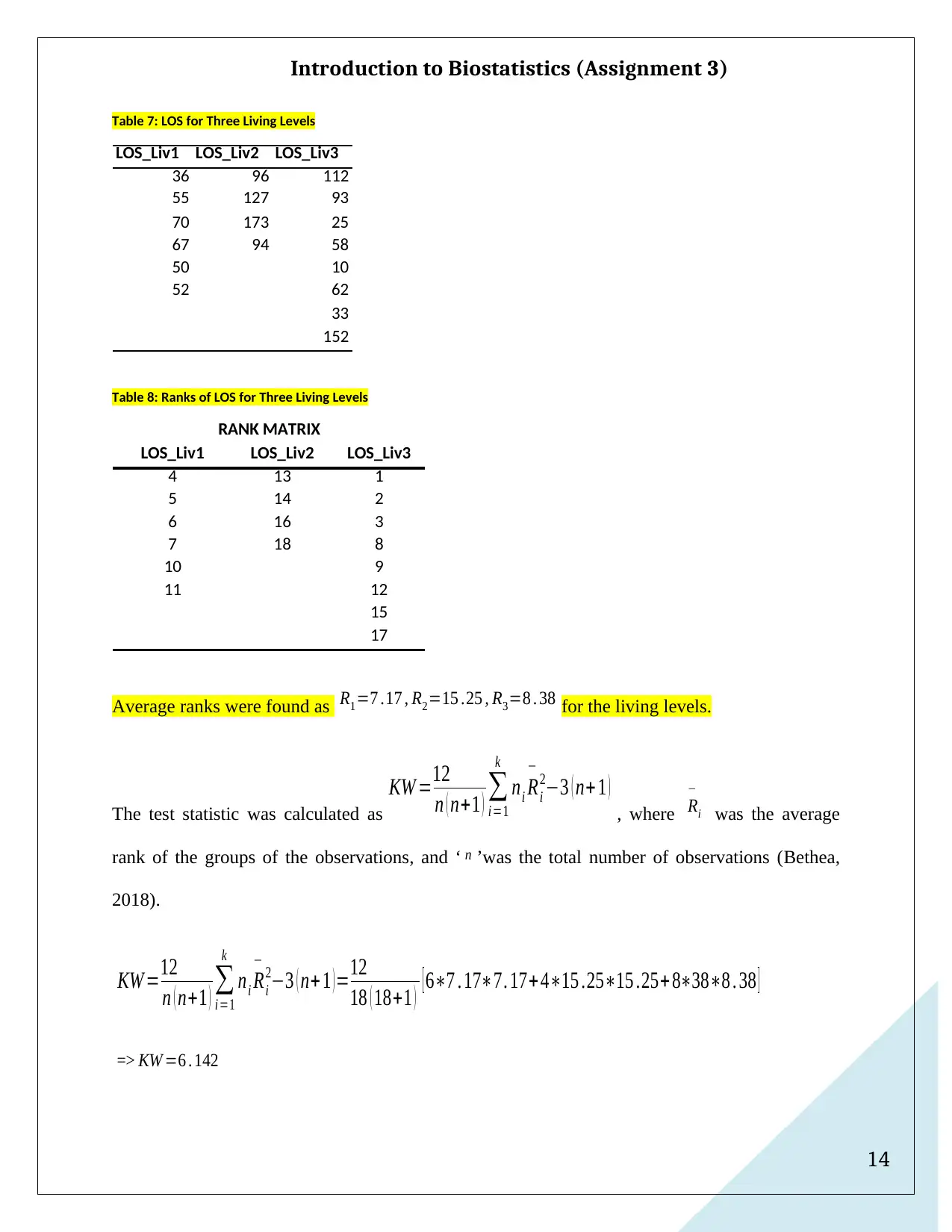
Introduction to Biostatistics (Assignment 3)
Table 7: LOS for Three Living Levels
LOS_Liv1 LOS_Liv2 LOS_Liv3
36 96 112
55 127 93
70 173 25
67 94 58
50 10
52 62
33
152
Table 8: Ranks of LOS for Three Living Levels
LOS_Liv1 LOS_Liv2 LOS_Liv3
4 13 1
5 14 2
6 16 3
7 18 8
10 9
11 12
15
17
RANK MATRIX
Average ranks were found as R1=7 .17 , R2=15 .25 , R3=8 . 38 for the living levels.
The test statistic was calculated as
KW =12
n ( n+1 ) ∑
i=1
k
ni R
−
i
2−3 ( n+ 1 )
, where R
−
i was the average
rank of the groups of the observations, and ‘ n ’was the total number of observations (Bethea,
2018).
KW =12
n ( n+1 ) ∑
i =1
k
ni R
−
i
2−3 ( n+ 1 ) =12
18 ( 18+1 ) [ 6∗7 . 17∗7. 17+4∗15 .25∗15 .25+ 8∗38∗8 . 38 ]
=> KW =6 . 142

15
Introduction to Biostatistics (Assignment 3)
Where k −1=3−1=2 degrees of freedom with p-value = 0.046 (Kherlakian et al., 2016; Saeed et
al., 2017).
The Chi-square statistics was calculated at 2 (k-1= 3 – 1 = 2) degrees of freedom as 5.991 using
Excel using CHIINV (0.05, 2).
The p-value was found to be 0.046 at 5% level of significance (from SPSS output)
The p-value implied that at 5% level of significance the null hypothesis could be rejected against
the alternate hypothesis. It could be concluded that the differences in LOSs were significant at
5% level and average LOS for patients with home with spouse living status was greater than
other two living status.
Table 9: Kruskal-Wallis SPSS Output

16
Introduction to Biostatistics (Assignment 3)
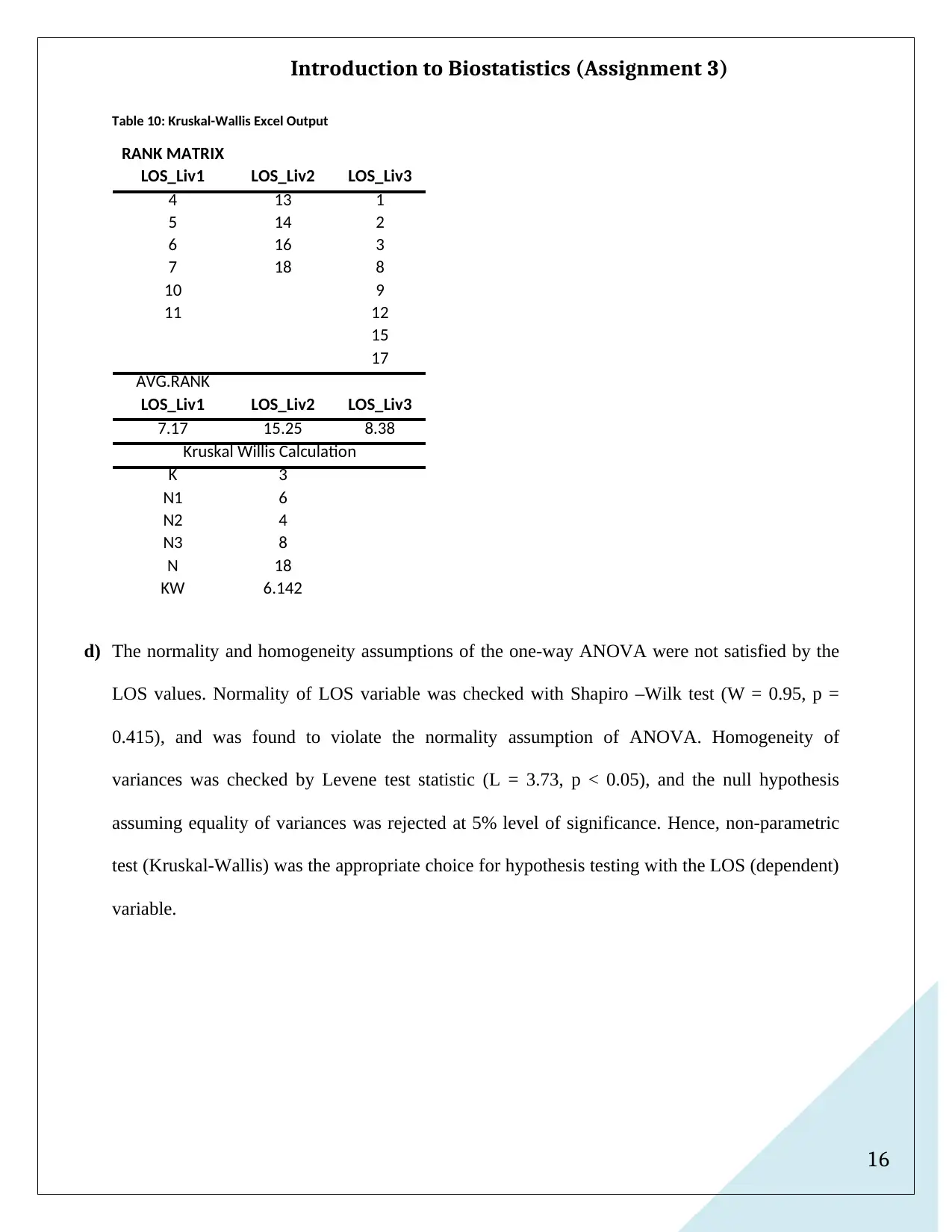
Table 10: Kruskal-Wallis Excel Output
RANK MATRIX
LOS_Liv1 LOS_Liv2 LOS_Liv3
4 13 1
5 14 2
6 16 3
7 18 8
10 9
11 12
15
17
AVG.RANK
LOS_Liv1 LOS_Liv2 LOS_Liv3
7.17 15.25 8.38
K 3
N1 6
N2 4
N3 8
N 18
KW 6.142
Kruskal Willis Calculation
d) The normality and homogeneity assumptions of the one-way ANOVA were not satisfied by the
LOS values. Normality of LOS variable was checked with Shapiro –Wilk test (W = 0.95, p =
0.415), and was found to violate the normality assumption of ANOVA. Homogeneity of
variances was checked by Levene test statistic (L = 3.73, p < 0.05), and the null hypothesis
assuming equality of variances was rejected at 5% level of significance. Hence, non-parametric
test (Kruskal-Wallis) was the appropriate choice for hypothesis testing with the LOS (dependent)
variable.

17
Introduction to Biostatistics (Assignment 3)
References
Bethea, R.M., 2018. Statistical methods for engineers and scientists.Routledge.
Julkowska, M.M., Saade, S., Agarwal, G., Gao, G., Pailles, Y., Morton, M., Awlia, M. and
Tester, M., 2018. MVAPP–MULTIVARIATE ANALYSIS APPLICATION FOR
STREAMLINED DATA ANALYSIS AND CURATION.
Kherlakian, D., Cunha, R.S., Ehrhardt, I.C., Zuolo, M.L., Kishen, A. and da Silveira Bueno,
C.E., 2016. Comparison of the incidence of postoperative pain after using 2 reciprocating
systems and a continuous rotary system: a prospective randomized clinical trial. Journal of
endodontics, 42(2), pp.171-176.
MacFarland, T.W. and Yates, J.M., 2016. Mann–whitney u test.In Introduction to nonparametric
statistics for the biological sciences using R (pp. 103-132).Springer, Cham.
Nieuwenhuis, M., Schnack, H.G., van Haren, N.E., Abramovic, L., Scheewe, T.W., Brouwer,
R.M., Pol, H.E.H. and Kahn, R.S., 2016. Can structural MRI aid in clinical classification? A
machine learning study in two independent samples of patients with schizophrenia,
bipolardisorder and healthy subjects. The studies described in this thesis were performed at the
Rudolf Magnus Institute of Neuroscience, Department of Psychiatry, University Medical Center
Utrecht, the Netherlands., 84, p.42.
Saeed, M., Koller, G., Niazi, S., Patel, S., Mannocci, F., Bruce, K. and Foschi, F., 2017. bacterial
Contamination of Endodontic Materials before and after Clinical Storage. Journal of
endodontics, 43(11), pp.1852-1856.

18
Introduction to Biostatistics (Assignment 3)
Sausen, M., Phallen, J., Adleff, V., Jones, S., Leary, R.J., Barrett, M.T., Anagnostou, V., Parpart-
Li, S., Murphy, D., Li, Q.K. and Hruban, C.A., 2015. Clinical implications of genomic
alterations in the tumour and circulation of pancreatic cancer patients. Nature communications,
6, p.7686.
Williams, B., 2017. Biostatistics: Concepts and applications for biologists. CRC Press.