HSH 746 Biostatistics Assignment: Confidence Intervals and T-Tests

This biostatistics assignment delves into the analysis of drug use proportions and dietary fat. It calculates and interprets 95% confidence intervals for differences in illicit drug use between men and women in 2001. It further examines whether targets for reducing illicit drug use in 2016 were met...Read More

Running Head: BIOSTATISTICS
Biostatistics
Name of the Student
Name of the University
Author Note

1BIOSTATISTICS
Table of Contents
ANSWER 1.....................................................................................................................................2
ANSWER 2.....................................................................................................................................3
Part a............................................................................................................................................3
Part b............................................................................................................................................3
ANSWER 3.....................................................................................................................................4
ANSWER 4.....................................................................................................................................4
ANSWER 5.....................................................................................................................................5
ANSWER 6.....................................................................................................................................6
Reference.........................................................................................................................................7

2BIOSTATISTICS
ANSWER 1
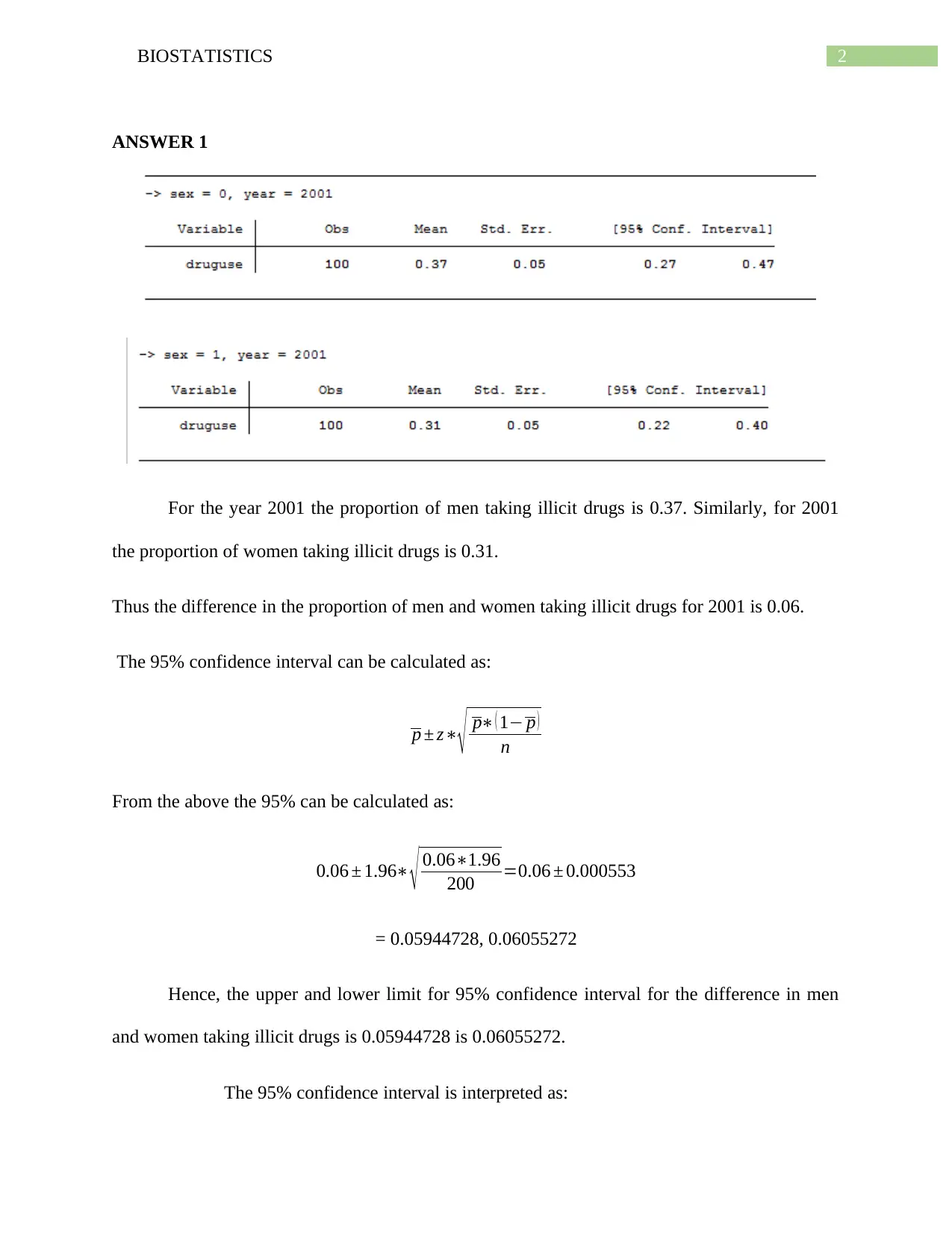
For the year 2001 the proportion of men taking illicit drugs is 0.37. Similarly, for 2001
the proportion of women taking illicit drugs is 0.31.
Thus the difference in the proportion of men and women taking illicit drugs for 2001 is 0.06.
The 95% confidence interval can be calculated as:
p ± z∗
√ p∗ ( 1− p )
n
From the above the 95% can be calculated as:
0.06 ± 1.96∗ √ 0.06∗1.96
200 =0.06 ± 0.000553
= 0.05944728, 0.06055272
Hence, the upper and lower limit for 95% confidence interval for the difference in men
and women taking illicit drugs is 0.05944728 is 0.06055272.
The 95% confidence interval is interpreted as:

3BIOSTATISTICS
There is a 95% probability that the mean of repeat sample of men and women taking illicit drugs
in the year 2001 would be between 0.05944728 is 0.06055272.
ANSWER 2
Part a
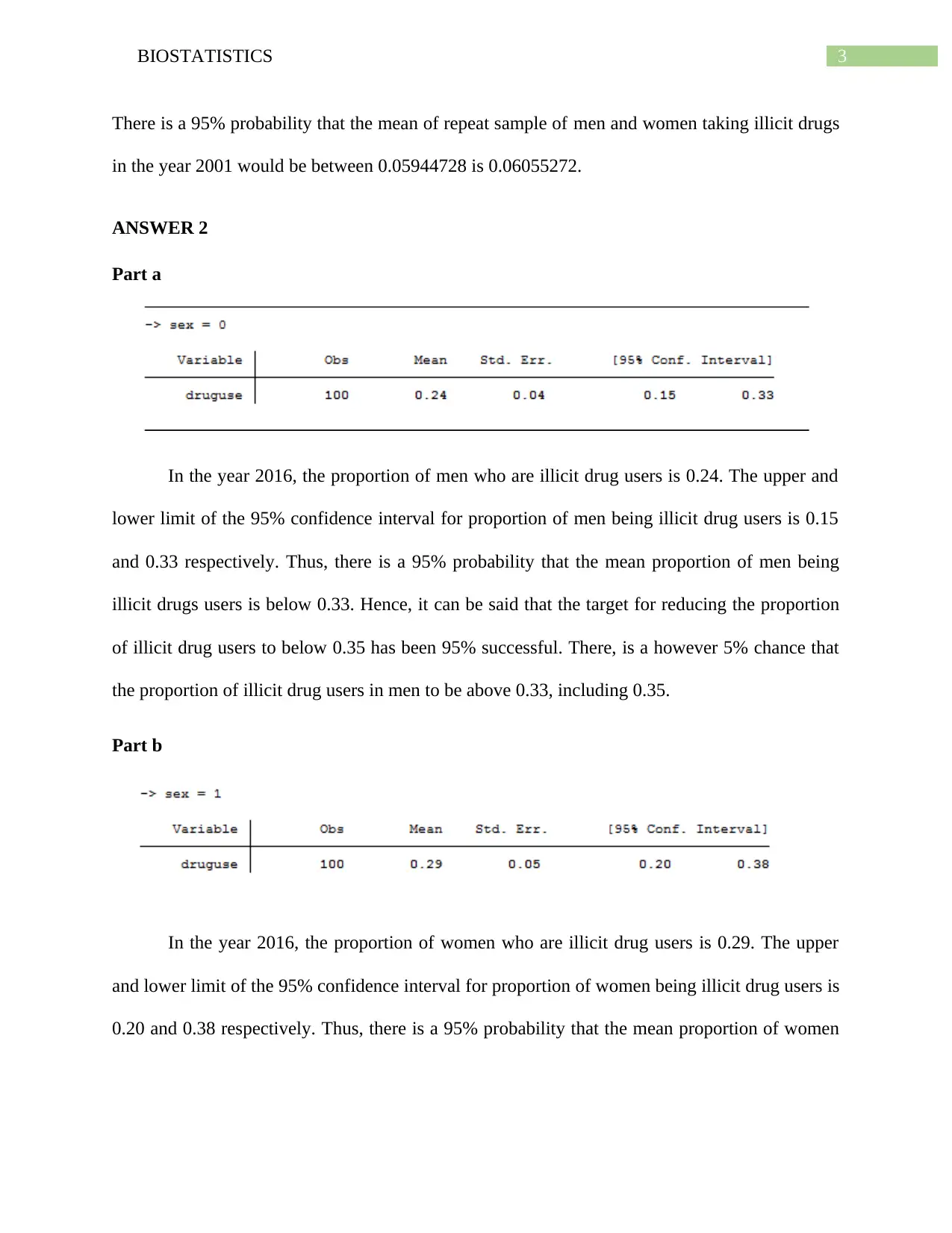
In the year 2016, the proportion of men who are illicit drug users is 0.24. The upper and
lower limit of the 95% confidence interval for proportion of men being illicit drug users is 0.15
and 0.33 respectively. Thus, there is a 95% probability that the mean proportion of men being
illicit drugs users is below 0.33. Hence, it can be said that the target for reducing the proportion
of illicit drug users to below 0.35 has been 95% successful. There, is a however 5% chance that
the proportion of illicit drug users in men to be above 0.33, including 0.35.
Part b
In the year 2016, the proportion of women who are illicit drug users is 0.29. The upper
and lower limit of the 95% confidence interval for proportion of women being illicit drug users is
0.20 and 0.38 respectively. Thus, there is a 95% probability that the mean proportion of women

4BIOSTATISTICS
being illicit drugs users is below 0.38. Thus, it can be said that the target for reducing the
proportion of illicit drug users in women to below 0.35 has not been successful.
ANSWER 3
The mean proportion of dietary fat for the intervention group is 0.202. The upper and
lower limit of 95% confidence interval for the mean score is 0.170 and 0.235 respectively.
0.170< μ<0.235
The 95% confidence interval is interpreted as:
There is a 95% probability that the mean of repeat sample of proportion of dietary fat for
the intervention group would be between 0.170 and 0.235.
In calculating the 95% confidence interval the normal probability distribution is used.
x ± z∗σ
√n
Where x is the mean of sample population, σ is the sample standard deviation and “n” is
the sample size. “z” denotes the normal probability distribution.
ANSWER 4
The above calculation shows that for the intervention group the mean of the “proportion
of calories coming from dietary” is 0.202. In addition, the upper and lower limits of the 95%
confidence interval for the mean are 0.170 and 0.235 respectively. Hence, it can be inferred that

5BIOSTATISTICS
there is a 95% probability that the mean would lie below 0.235 i.e., 23.5%. However, there is a
5% probability that the mean can lie above 23.5%. Hence, it can be assumed that for 95% of the
people the mean “proportion of calories coming from dietary” is below the recommended 35%.
ANSWER 5
The independent sample t-test is used to test for differences in mean “proportion of
calories from dietary fat,” (Waller 2016)
Null Hypothesis: The mean of the “proportion of calories from dietary fat.” For control and
intervention group are equal.
H0 : μcontrol=μintervention
Alternate Hypothesis: The mean of the “proportion of calories from dietary fat.” For control and
intervention group are not equal.
H A : μcontrol ≠ μintervention

6BIOSTATISTICS
From the results it can be inferred that there are statistically significant differences
between control and intervention group, p < 0.000 at 0.05 level of significance.
The mean of the “proportion of calories coming from dietary fat” for the control group
(0.3414815) is higher than for the intervention group (0.2024828).
ANSWER 6
The mean score of the 80 students is 35.46. The upper and lower limit of 95% confidence
interval for the mean score is 31.57 and 39.36 respectively.
31.57< μ<39.36
The 95% confidence interval is interpreted as (Anderson et al., 2016):
There is a 95% probability that the mean of repeat samples of the score of 80 students
would be between 31.57 and 39.36.

7BIOSTATISTICS
Reference
Anderson, D.R., Sweeney, D.J., Williams, T.A., Camm, J.D. and Cochran, J.J., 2016. Statistics
for business & economics. 8th Edition., Cengage Learning
Waller, D., 2016. Statistics for business. Routledge.