Business Analysis: Hypotheses Testing, ANOVA, and Chi-Square
VerifiedAdded on 2023/06/09
|19
|3280
|401
AI Summary
This article covers hypotheses testing, ANOVA, and Chi-Square in Business Analysis. It explains how to calculate test statistics, determine appropriate probabilities for committing Type I error, and interpret results. It also provides insights into data analysis techniques for exercises, blood glucose levels, and rental and homeowner vacancy rates.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Business Analysis
Student Name
Course Name
Institution Affiliation
Student Name
Course Name
Institution Affiliation
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
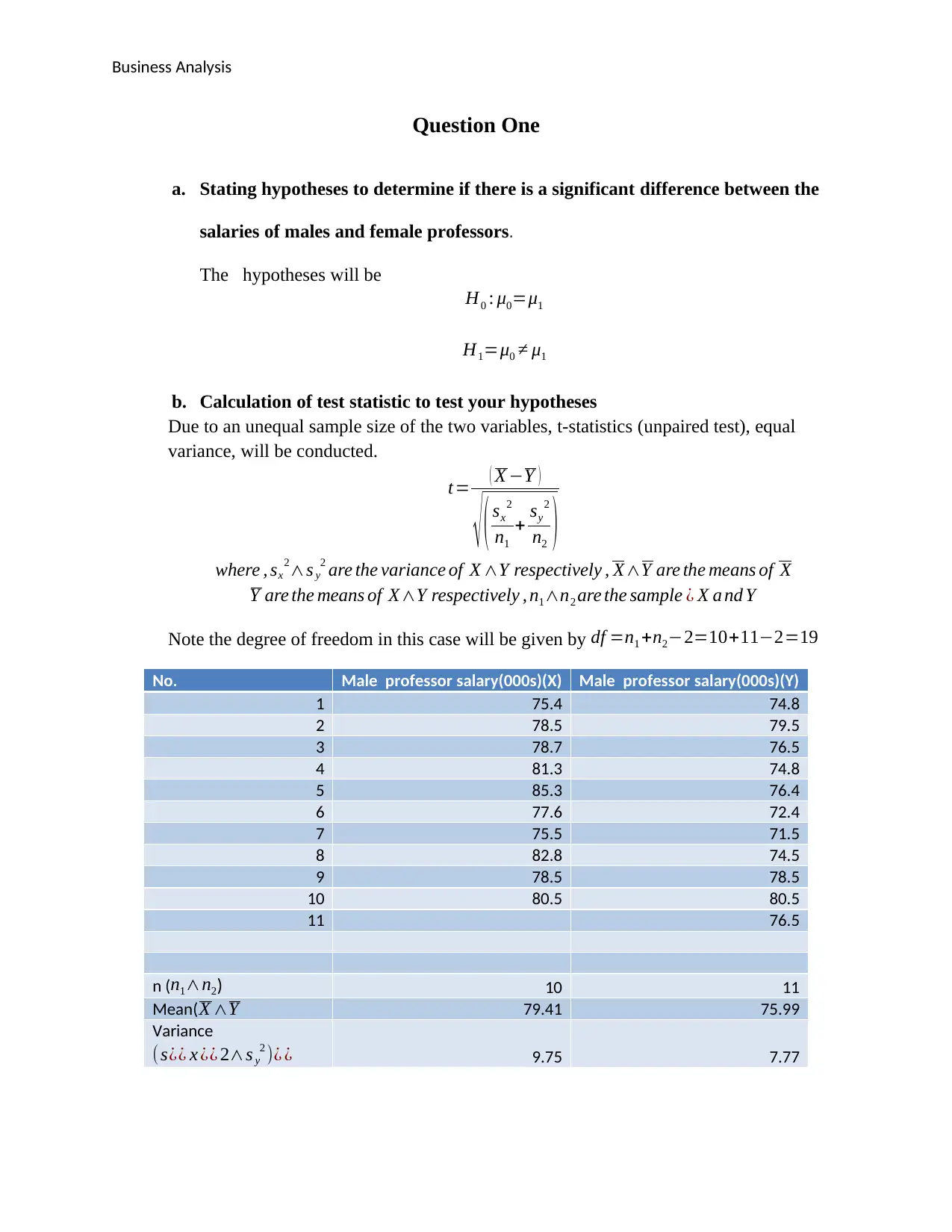
Business Analysis
Question One
a. Stating hypotheses to determine if there is a significant difference between the
salaries of males and female professors.
The hypotheses will be
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Calculation of test statistic to test your hypotheses
Due to an unequal sample size of the two variables, t-statistics (unpaired test), equal
variance, will be conducted.
t= ( X −Y )
√ ( sx
2
n1
+ sy
2
n2 )
where , sx
2∧s y
2 are the variance of X ∧Y respectively , X∧Y are the means of X
Y are the means of X∧Y respectively , n1∧n2 are the sample ¿ X a nd Y
Note the degree of freedom in this case will be given by df =n1 +n2−2=10+11−2=19
No. Male professor salary(000s)(X) Male professor salary(000s)(Y)
1 75.4 74.8
2 78.5 79.5
3 78.7 76.5
4 81.3 74.8
5 85.3 76.4
6 77.6 72.4
7 75.5 71.5
8 82.8 74.5
9 78.5 78.5
10 80.5 80.5
11 76.5
n (n1∧n2) 10 11
Mean( X ∧Y 79.41 75.99
Variance
(s¿¿ x ¿¿ 2∧s y
2 )¿ ¿ 9.75 7.77
Question One
a. Stating hypotheses to determine if there is a significant difference between the
salaries of males and female professors.
The hypotheses will be
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Calculation of test statistic to test your hypotheses
Due to an unequal sample size of the two variables, t-statistics (unpaired test), equal
variance, will be conducted.
t= ( X −Y )
√ ( sx
2
n1
+ sy
2
n2 )
where , sx
2∧s y
2 are the variance of X ∧Y respectively , X∧Y are the means of X
Y are the means of X∧Y respectively , n1∧n2 are the sample ¿ X a nd Y
Note the degree of freedom in this case will be given by df =n1 +n2−2=10+11−2=19
No. Male professor salary(000s)(X) Male professor salary(000s)(Y)
1 75.4 74.8
2 78.5 79.5
3 78.7 76.5
4 81.3 74.8
5 85.3 76.4
6 77.6 72.4
7 75.5 71.5
8 82.8 74.5
9 78.5 78.5
10 80.5 80.5
11 76.5
n (n1∧n2) 10 11
Mean( X ∧Y 79.41 75.99
Variance
(s¿¿ x ¿¿ 2∧s y
2 )¿ ¿ 9.75 7.77

Business Analysis
t= (79.41−75.99 )
√ ( 9.75
10 + 7.77
11 )= 3.42
√1.6818
¿ 3.42
1.2968 =2.636
Thus tcomputed=2.636
c. Specification and justification of an appropriate probability for committing
Type I error (α)
The probability of committing type I error will be 5%, which is the probability of
incorrectly rejecting the null hypothesis. This will be attained at the significance level
of 95%, which is the probability of rejecting or accepting the null hypothesis
accurately.
To make the decision, in this case, the critical value of need to determine from
t-tables
t0.05 ( 19 df ) ( twotailed ) =1.729
d. Reporting of the decision and clearly explain your result.
The tcomputed=2.636 ¿ tα=1.729 , therefore, null hypothesis,H0 t h e t h e : μ0=μ1 will
be rejected. This suggests that there is significant diffa erence between the salaries of
males and female professors. In this case, alternative hypothe thesis will be accepted
as it’s supported by the results of the test conducted.
In conclusion, the salary of a male professor and a female professor is not the same.
t= (79.41−75.99 )
√ ( 9.75
10 + 7.77
11 )= 3.42
√1.6818
¿ 3.42
1.2968 =2.636
Thus tcomputed=2.636
c. Specification and justification of an appropriate probability for committing
Type I error (α)
The probability of committing type I error will be 5%, which is the probability of
incorrectly rejecting the null hypothesis. This will be attained at the significance level
of 95%, which is the probability of rejecting or accepting the null hypothesis
accurately.
To make the decision, in this case, the critical value of need to determine from
t-tables
t0.05 ( 19 df ) ( twotailed ) =1.729
d. Reporting of the decision and clearly explain your result.
The tcomputed=2.636 ¿ tα=1.729 , therefore, null hypothesis,H0 t h e t h e : μ0=μ1 will
be rejected. This suggests that there is significant diffa erence between the salaries of
males and female professors. In this case, alternative hypothe thesis will be accepted
as it’s supported by the results of the test conducted.
In conclusion, the salary of a male professor and a female professor is not the same.
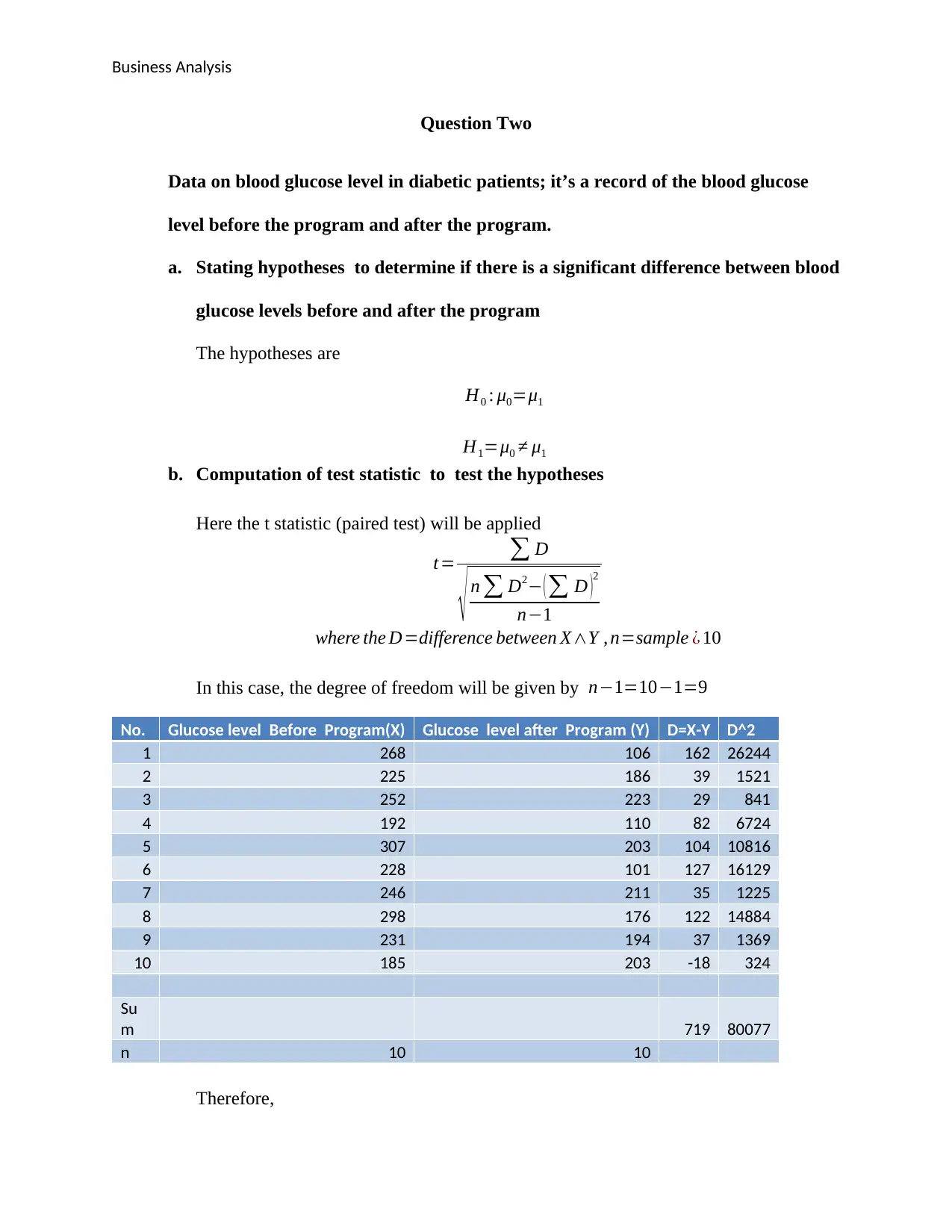
Business Analysis
Question Two
Data on blood glucose level in diabetic patients; it’s a record of the blood glucose
level before the program and after the program.
a. Stating hypotheses to determine if there is a significant difference between blood
glucose levels before and after the program
The hypotheses are
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Computation of test statistic to test the hypotheses
Here the t statistic (paired test) will be applied
t= ∑ D
√ n ∑ D2− ( ∑ D )
2
n−1
where the D=difference between X∧Y , n=sample ¿ 10
In this case, the degree of freedom will be given by n−1=10−1=9
No. Glucose level Before Program(X) Glucose level after Program (Y) D=X-Y D^2
1 268 106 162 26244
2 225 186 39 1521
3 252 223 29 841
4 192 110 82 6724
5 307 203 104 10816
6 228 101 127 16129
7 246 211 35 1225
8 298 176 122 14884
9 231 194 37 1369
10 185 203 -18 324
Su
m 719 80077
n 10 10
Therefore,
Question Two
Data on blood glucose level in diabetic patients; it’s a record of the blood glucose
level before the program and after the program.
a. Stating hypotheses to determine if there is a significant difference between blood
glucose levels before and after the program
The hypotheses are
H0 : μ0=μ1
H1=μ0 ≠ μ1
b. Computation of test statistic to test the hypotheses
Here the t statistic (paired test) will be applied
t= ∑ D
√ n ∑ D2− ( ∑ D )
2
n−1
where the D=difference between X∧Y , n=sample ¿ 10
In this case, the degree of freedom will be given by n−1=10−1=9
No. Glucose level Before Program(X) Glucose level after Program (Y) D=X-Y D^2
1 268 106 162 26244
2 225 186 39 1521
3 252 223 29 841
4 192 110 82 6724
5 307 203 104 10816
6 228 101 127 16129
7 246 211 35 1225
8 298 176 122 14884
9 231 194 37 1369
10 185 203 -18 324
Su
m 719 80077
n 10 10
Therefore,
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Business Analysis
t= 719
√ 10 ( 80077 ) − ( 719 )2
10−1
= 719
√ 283809
9
¿ 719
177.5791=4.0489
Hence, tcomputed=4.0489
c. Specification and justification of an appropriate probability for committing
Type I error (α)
The probability of committing type I error will be 5%, which is the probability of
incorrectly rejecting the null hypothesis. This will be attained at the significance level
of 95%, which is the probability of rejecting or accepting the null hypothesis
accurately.
To make the decision, in this case, the critical value of need to determine from
t-tables
t0.05 (9 df ) ( twotailed ) =1.833
d. Reporting of the decision and clearly explain your result.
The tcomputed=4.0489> ¿ tα =1.833 , therefore, null hypothesis, H0 t h e t h e : μ0=μ1 will
be rejected. This suggests that there is a significant difference between blood glucose
levels before and after the program. In this case, alternative hythe pothesis
H1=μ0 ≠ μ1 is accepted, clearly support that the blood glucose level of diabetic
t= 719
√ 10 ( 80077 ) − ( 719 )2
10−1
= 719
√ 283809
9
¿ 719
177.5791=4.0489
Hence, tcomputed=4.0489
c. Specification and justification of an appropriate probability for committing
Type I error (α)
The probability of committing type I error will be 5%, which is the probability of
incorrectly rejecting the null hypothesis. This will be attained at the significance level
of 95%, which is the probability of rejecting or accepting the null hypothesis
accurately.
To make the decision, in this case, the critical value of need to determine from
t-tables
t0.05 (9 df ) ( twotailed ) =1.833
d. Reporting of the decision and clearly explain your result.
The tcomputed=4.0489> ¿ tα =1.833 , therefore, null hypothesis, H0 t h e t h e : μ0=μ1 will
be rejected. This suggests that there is a significant difference between blood glucose
levels before and after the program. In this case, alternative hythe pothesis
H1=μ0 ≠ μ1 is accepted, clearly support that the blood glucose level of diabetic
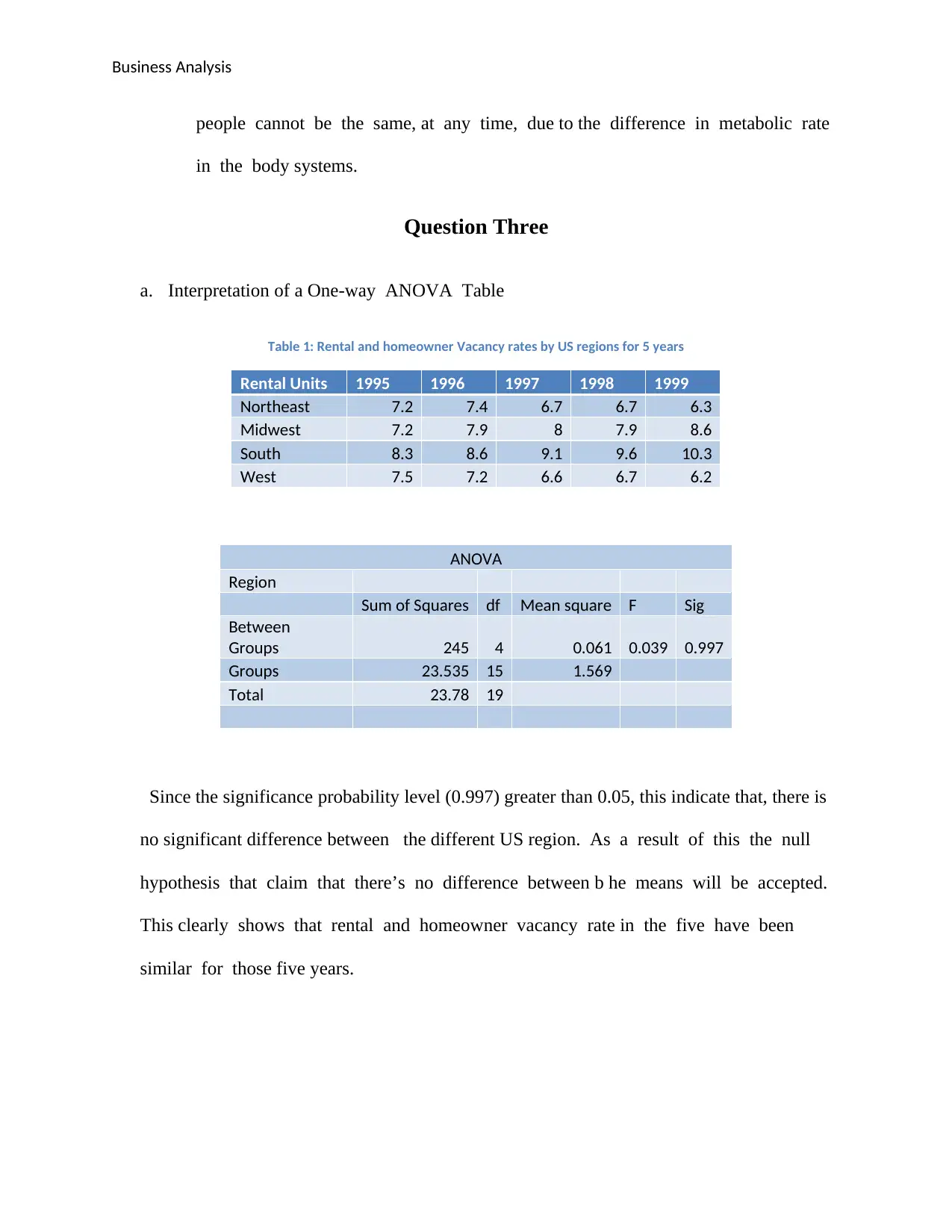
Business Analysis
people cannot be the same, at any time, due to the difference in metabolic rate
in the body systems.
Question Three
a. Interpretation of a One-way ANOVA Table
Table 1: Rental and homeowner Vacancy rates by US regions for 5 years
Rental Units 1995 1996 1997 1998 1999
Northeast 7.2 7.4 6.7 6.7 6.3
Midwest 7.2 7.9 8 7.9 8.6
South 8.3 8.6 9.1 9.6 10.3
West 7.5 7.2 6.6 6.7 6.2
ANOVA
Region
Sum of Squares df Mean square F Sig
Between
Groups 245 4 0.061 0.039 0.997
Groups 23.535 15 1.569
Total 23.78 19
Since the significance probability level (0.997) greater than 0.05, this indicate that, there is
no significant difference between the different US region. As a result of this the null
hypothesis that claim that there’s no difference between b he means will be accepted.
This clearly shows that rental and homeowner vacancy rate in the five have been
similar for those five years.
people cannot be the same, at any time, due to the difference in metabolic rate
in the body systems.
Question Three
a. Interpretation of a One-way ANOVA Table
Table 1: Rental and homeowner Vacancy rates by US regions for 5 years
Rental Units 1995 1996 1997 1998 1999
Northeast 7.2 7.4 6.7 6.7 6.3
Midwest 7.2 7.9 8 7.9 8.6
South 8.3 8.6 9.1 9.6 10.3
West 7.5 7.2 6.6 6.7 6.2
ANOVA
Region
Sum of Squares df Mean square F Sig
Between
Groups 245 4 0.061 0.039 0.997
Groups 23.535 15 1.569
Total 23.78 19
Since the significance probability level (0.997) greater than 0.05, this indicate that, there is
no significant difference between the different US region. As a result of this the null
hypothesis that claim that there’s no difference between b he means will be accepted.
This clearly shows that rental and homeowner vacancy rate in the five have been
similar for those five years.
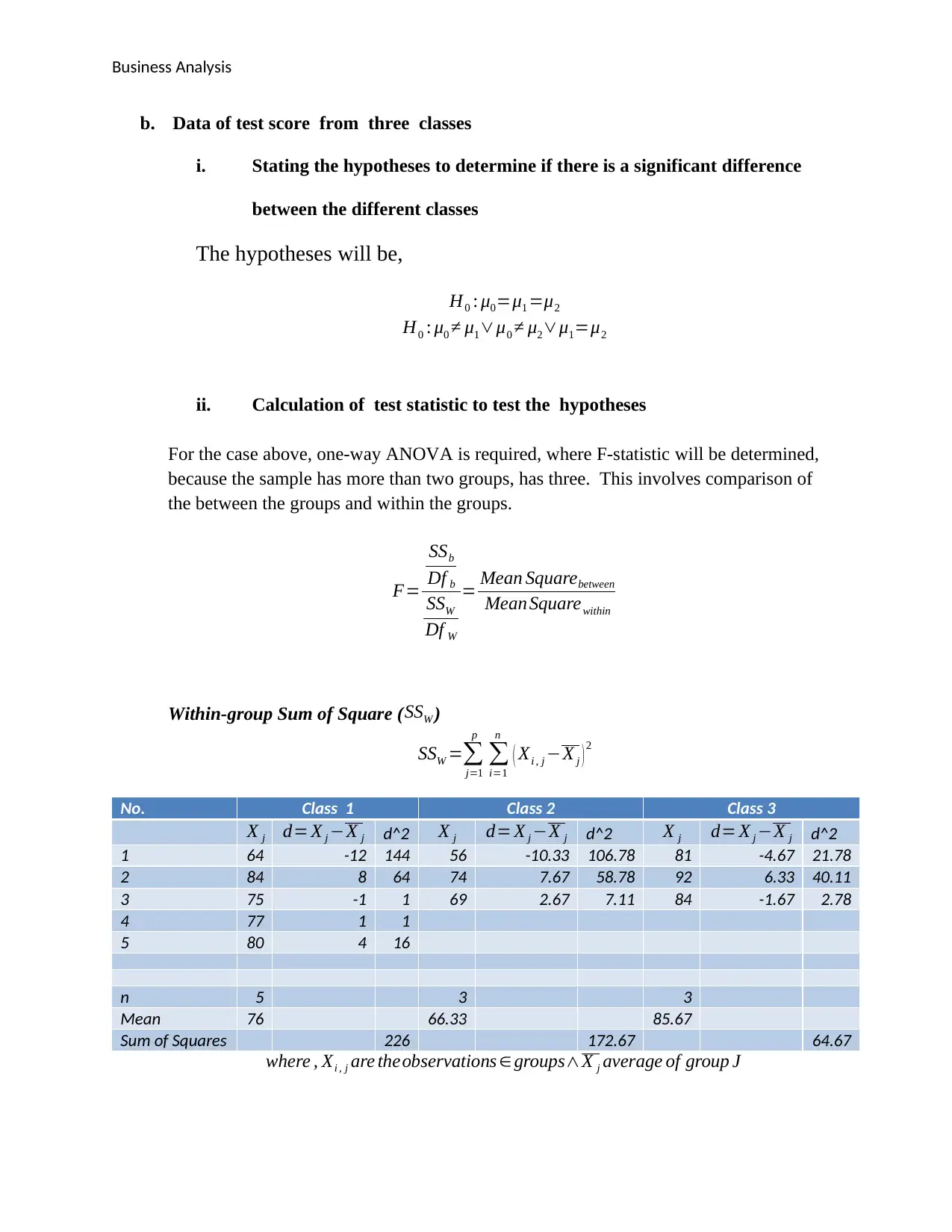
Business Analysis
b. Data of test score from three classes
i. Stating the hypotheses to determine if there is a significant difference
between the different classes
The hypotheses will be,
H0 : μ0=μ1 =μ2
H0 : μ0 ≠ μ1∨μ0 ≠ μ2∨μ1=μ2
ii. Calculation of test statistic to test the hypotheses
For the case above, one-way ANOVA is required, where F-statistic will be determined,
because the sample has more than two groups, has three. This involves comparison of
the between the groups and within the groups.
F=
SSb
Df b
SSW
Df W
= Mean Squarebetween
Mean Squarewithin
Within-group Sum of Square ( SSW )
SSW =∑
j=1
p
∑
i=1
n
( Xi , j −X j ) 2
No. Class 1 Class 2 Class 3
X j d= X j −X j d^2 X j d= X j −X j d^2 X j d= X j −X j d^2
1 64 -12 144 56 -10.33 106.78 81 -4.67 21.78
2 84 8 64 74 7.67 58.78 92 6.33 40.11
3 75 -1 1 69 2.67 7.11 84 -1.67 2.78
4 77 1 1
5 80 4 16
n 5 3 3
Mean 76 66.33 85.67
Sum of Squares 226 172.67 64.67
where , Xi , j are theobservations ∈groups∧X j average of group J
b. Data of test score from three classes
i. Stating the hypotheses to determine if there is a significant difference
between the different classes
The hypotheses will be,
H0 : μ0=μ1 =μ2
H0 : μ0 ≠ μ1∨μ0 ≠ μ2∨μ1=μ2
ii. Calculation of test statistic to test the hypotheses
For the case above, one-way ANOVA is required, where F-statistic will be determined,
because the sample has more than two groups, has three. This involves comparison of
the between the groups and within the groups.
F=
SSb
Df b
SSW
Df W
= Mean Squarebetween
Mean Squarewithin
Within-group Sum of Square ( SSW )
SSW =∑
j=1
p
∑
i=1
n
( Xi , j −X j ) 2
No. Class 1 Class 2 Class 3
X j d= X j −X j d^2 X j d= X j −X j d^2 X j d= X j −X j d^2
1 64 -12 144 56 -10.33 106.78 81 -4.67 21.78
2 84 8 64 74 7.67 58.78 92 6.33 40.11
3 75 -1 1 69 2.67 7.11 84 -1.67 2.78
4 77 1 1
5 80 4 16
n 5 3 3
Mean 76 66.33 85.67
Sum of Squares 226 172.67 64.67
where , Xi , j are theobservations ∈groups∧X j average of group J
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Business Analysis
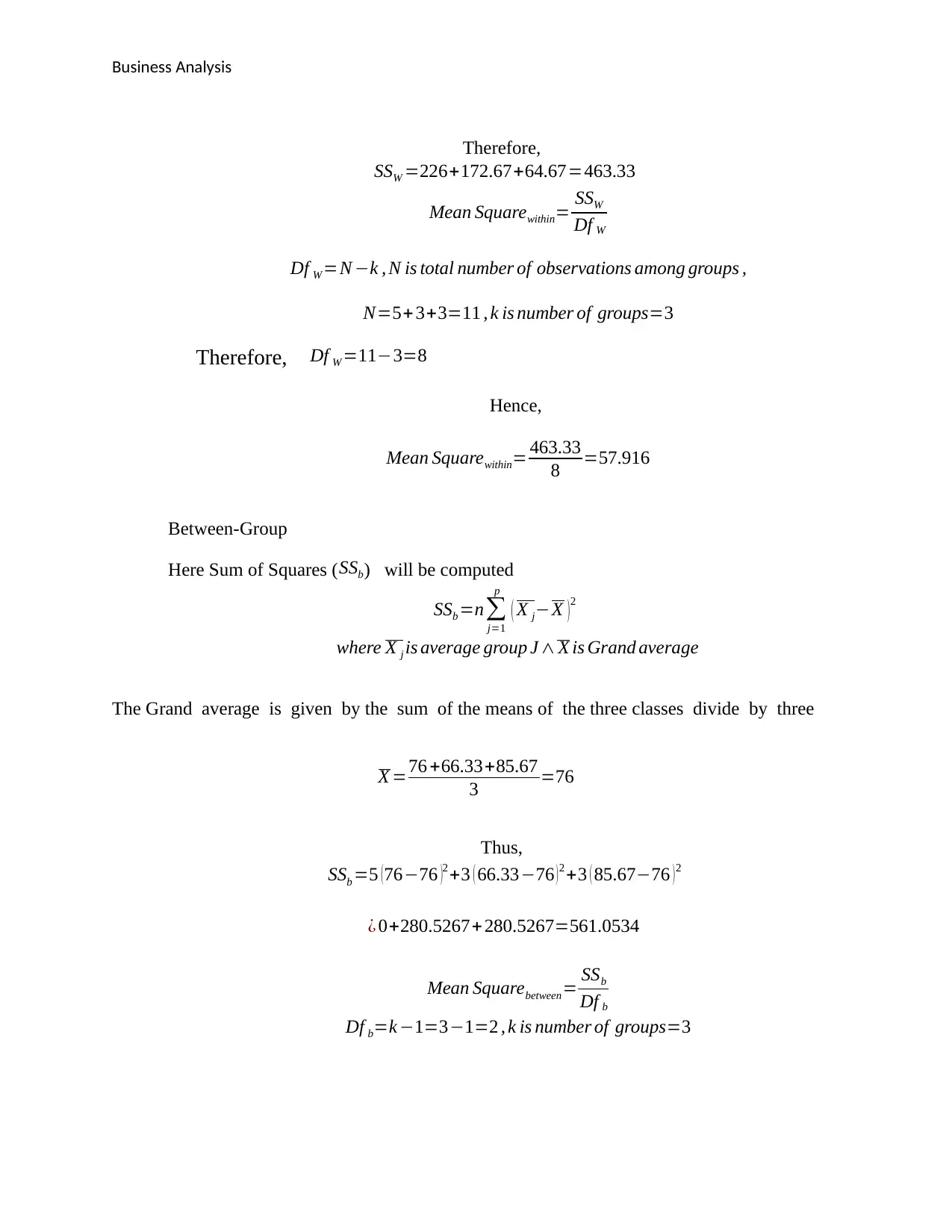
Therefore,
SSW =226+172.67+64.67=463.33
Mean Squarewithin= SSW
Df W
Df W =N −k , N is total number of observations among groups ,
N=5+ 3+3=11 , k is number of groups=3
Therefore, Df W =11−3=8
Hence,
Mean Squarewithin= 463.33
8 =57.916
Between-Group
Here Sum of Squares ( SSb) will be computed
SSb =n∑
j=1
p
( X j−X )2
where X j is average group J ∧ X is Grand average
The Grand average is given by the sum of the means of the three classes divide by three
X =76 +66.33+85.67
3 =76
Thus,
SSb =5 (76−76 )2 +3 ( 66.33−76 )2 +3 ( 85.67−76 )2
¿ 0+280.5267+ 280.5267=561.0534
Mean Squarebetween= SSb
Df b
Df b=k −1=3−1=2 , k is number of groups=3
Therefore,
SSW =226+172.67+64.67=463.33
Mean Squarewithin= SSW
Df W
Df W =N −k , N is total number of observations among groups ,
N=5+ 3+3=11 , k is number of groups=3
Therefore, Df W =11−3=8
Hence,
Mean Squarewithin= 463.33
8 =57.916
Between-Group
Here Sum of Squares ( SSb) will be computed
SSb =n∑
j=1
p
( X j−X )2
where X j is average group J ∧ X is Grand average
The Grand average is given by the sum of the means of the three classes divide by three
X =76 +66.33+85.67
3 =76
Thus,
SSb =5 (76−76 )2 +3 ( 66.33−76 )2 +3 ( 85.67−76 )2
¿ 0+280.5267+ 280.5267=561.0534
Mean Squarebetween= SSb
Df b
Df b=k −1=3−1=2 , k is number of groups=3
Business Analysis
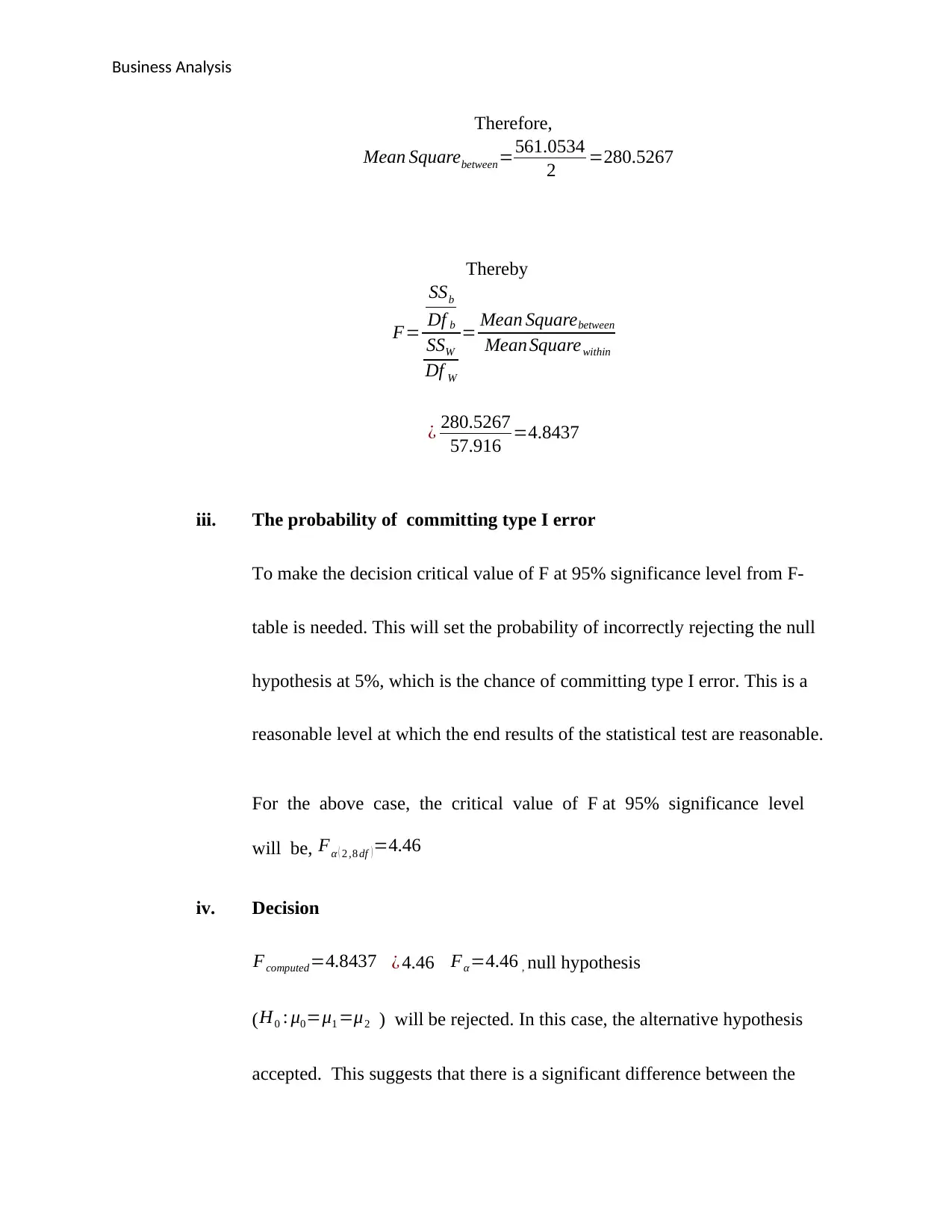
Therefore,
Mean Squarebetween=561.0534
2 =280.5267
Thereby
F=
SSb
Df b
SSW
Df W
= Mean Squarebetween
Mean Squarewithin
¿ 280.5267
57.916 =4.8437
iii. The probability of committing type I error
To make the decision critical value of F at 95% significance level from F-
table is needed. This will set the probability of incorrectly rejecting the null
hypothesis at 5%, which is the chance of committing type I error. This is a
reasonable level at which the end results of the statistical test are reasonable.
For the above case, the critical value of F at 95% significance level
will be, Fα ( 2 ,8 df )=4.46
iv. Decision
Fcomputed=4.8437 ¿ 4.46 Fα =4.46 , null hypothesis
( H0 : μ0=μ1 =μ2 ) will be rejected. In this case, the alternative hypothesis
accepted. This suggests that there is a significant difference between the
Therefore,
Mean Squarebetween=561.0534
2 =280.5267
Thereby
F=
SSb
Df b
SSW
Df W
= Mean Squarebetween
Mean Squarewithin
¿ 280.5267
57.916 =4.8437
iii. The probability of committing type I error
To make the decision critical value of F at 95% significance level from F-
table is needed. This will set the probability of incorrectly rejecting the null
hypothesis at 5%, which is the chance of committing type I error. This is a
reasonable level at which the end results of the statistical test are reasonable.
For the above case, the critical value of F at 95% significance level
will be, Fα ( 2 ,8 df )=4.46
iv. Decision
Fcomputed=4.8437 ¿ 4.46 Fα =4.46 , null hypothesis
( H0 : μ0=μ1 =μ2 ) will be rejected. In this case, the alternative hypothesis
accepted. This suggests that there is a significant difference between the

Business Analysis
different Classes. This means that student’s performance is not going to be
similar, some student will do better than the other student.
In conclusion the performance of the student will be dependent on the Class
she/ he is in and the instructor of that class. This revealed by the test statistic
conducted on the data, which clearly show the existence of the difference in
the performance from different classes
Question Four
a. Data collected on exercise question from a survey completed by 470 graduates.
i. Stating the hypotheses to test whether there is evidence of shift in
distribution of responses to the exercise question.
H0: There’s no difference
H1: There’s difference
ii. Computation of the test statistic to measure the discrepancies between
the observed and the expected result
Here, Chi-square statistic will be adopted,
χ2=∑ ( Observed−Expected )2
Expected
different Classes. This means that student’s performance is not going to be
similar, some student will do better than the other student.
In conclusion the performance of the student will be dependent on the Class
she/ he is in and the instructor of that class. This revealed by the test statistic
conducted on the data, which clearly show the existence of the difference in
the performance from different classes
Question Four
a. Data collected on exercise question from a survey completed by 470 graduates.
i. Stating the hypotheses to test whether there is evidence of shift in
distribution of responses to the exercise question.
H0: There’s no difference
H1: There’s difference
ii. Computation of the test statistic to measure the discrepancies between
the observed and the expected result
Here, Chi-square statistic will be adopted,
χ2=∑ ( Observed−Expected )2
Expected
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Business Analysis
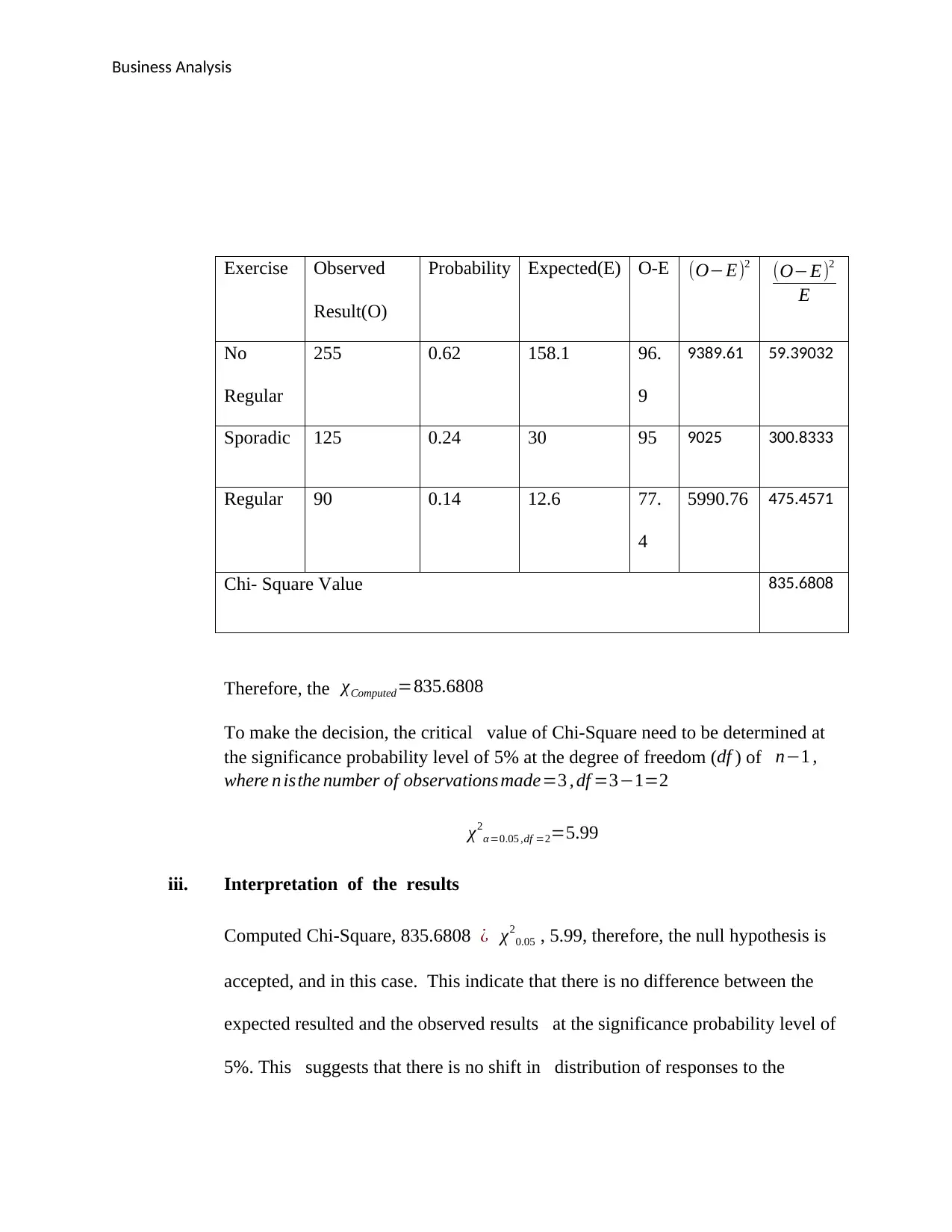
Exercise Observed
Result(O)
Probability Expected(E) O-E (O−E)2 (O−E)2
E
No
Regular
255 0.62 158.1 96.
9
9389.61 59.39032
Sporadic 125 0.24 30 95 9025 300.8333
Regular 90 0.14 12.6 77.
4
5990.76 475.4571
Chi- Square Value 835.6808
Therefore, the χComputed=835.6808
To make the decision, the critical value of Chi-Square need to be determined at
the significance probability level of 5% at the degree of freedom (df ) of n−1 ,
where n isthe number of observations made=3 , df =3−1=2
χ2
α =0.05 ,df =2=5.99
iii. Interpretation of the results
Computed Chi-Square, 835.6808 ¿ χ2
0.05 , 5.99, therefore, the null hypothesis is
accepted, and in this case. This indicate that there is no difference between the
expected resulted and the observed results at the significance probability level of
5%. This suggests that there is no shift in distribution of responses to the
Exercise Observed
Result(O)
Probability Expected(E) O-E (O−E)2 (O−E)2
E
No
Regular
255 0.62 158.1 96.
9
9389.61 59.39032
Sporadic 125 0.24 30 95 9025 300.8333
Regular 90 0.14 12.6 77.
4
5990.76 475.4571
Chi- Square Value 835.6808
Therefore, the χComputed=835.6808
To make the decision, the critical value of Chi-Square need to be determined at
the significance probability level of 5% at the degree of freedom (df ) of n−1 ,
where n isthe number of observations made=3 , df =3−1=2
χ2
α =0.05 ,df =2=5.99
iii. Interpretation of the results
Computed Chi-Square, 835.6808 ¿ χ2
0.05 , 5.99, therefore, the null hypothesis is
accepted, and in this case. This indicate that there is no difference between the
expected resulted and the observed results at the significance probability level of
5%. This suggests that there is no shift in distribution of responses to the
Business Analysis
exercise question following the implementation of the health promotion campaign
on campus.
b. Data from the Road Authority Of Ireland, shows the number of deaths on Irish roads
from 2000 to 2011
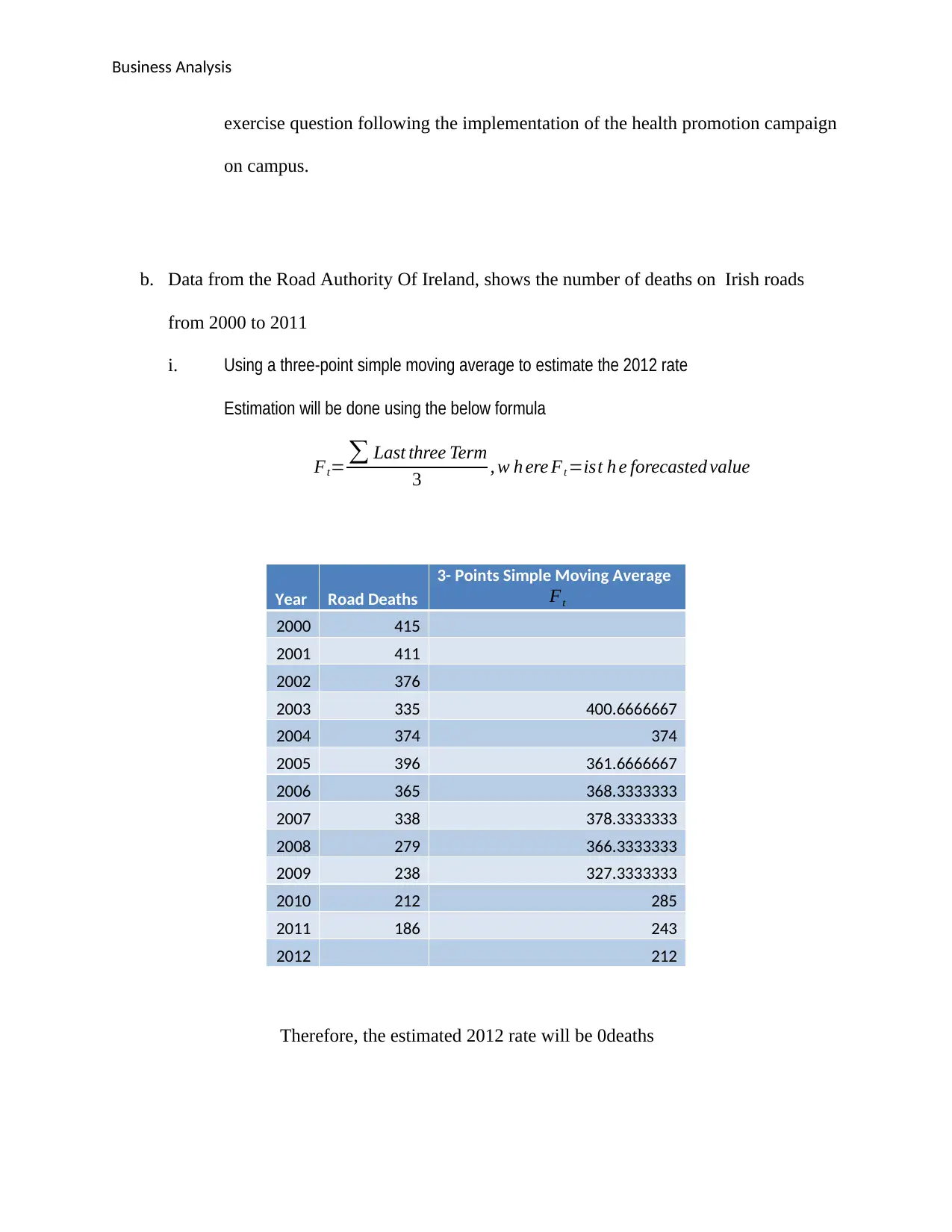
i. Using a three-point simple moving average to estimate the 2012 rate
Estimation will be done using the below formula
Ft= ∑ Last three Term
3 , w h ere Ft =ist h e forecasted value
Year Road Deaths
3- Points Simple Moving Average
Ft
2000 415
2001 411
2002 376
2003 335 400.6666667
2004 374 374
2005 396 361.6666667
2006 365 368.3333333
2007 338 378.3333333
2008 279 366.3333333
2009 238 327.3333333
2010 212 285
2011 186 243
2012 212
Therefore, the estimated 2012 rate will be 0deaths
exercise question following the implementation of the health promotion campaign
on campus.
b. Data from the Road Authority Of Ireland, shows the number of deaths on Irish roads
from 2000 to 2011
i. Using a three-point simple moving average to estimate the 2012 rate
Estimation will be done using the below formula
Ft= ∑ Last three Term
3 , w h ere Ft =ist h e forecasted value
Year Road Deaths
3- Points Simple Moving Average
Ft
2000 415
2001 411
2002 376
2003 335 400.6666667
2004 374 374
2005 396 361.6666667
2006 365 368.3333333
2007 338 378.3333333
2008 279 366.3333333
2009 238 327.3333333
2010 212 285
2011 186 243
2012 212
Therefore, the estimated 2012 rate will be 0deaths
Business Analysis
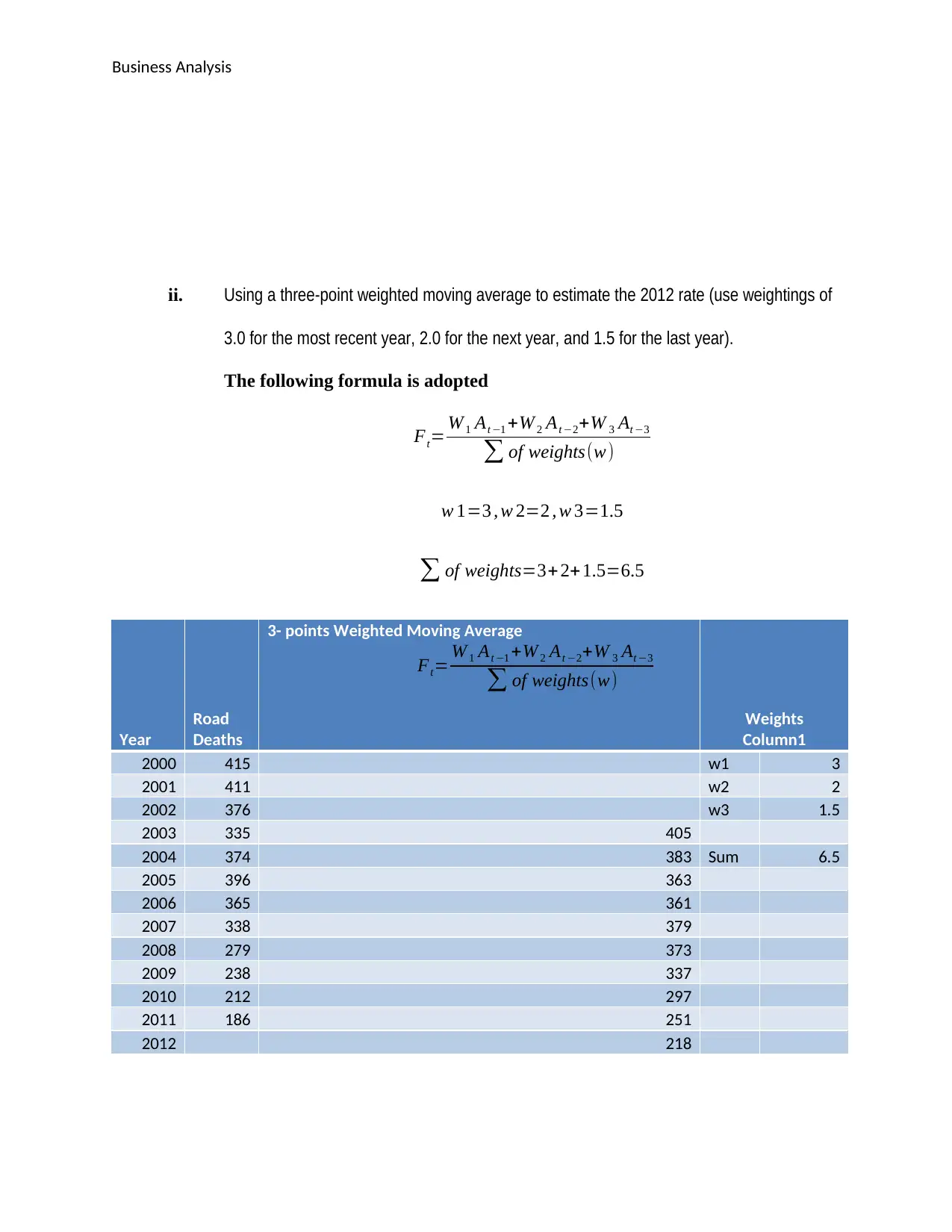
ii. Using a three-point weighted moving average to estimate the 2012 rate (use weightings of
3.0 for the most recent year, 2.0 for the next year, and 1.5 for the last year).
The following formula is adopted
Ft= W 1 At −1 +W 2 At −2+W 3 At −3
∑ of weights(w)
w 1=3 , w 2=2 , w 3=1.5
∑ of weights=3+ 2+ 1.5=6.5
Year
Road
Deaths
3- points Weighted Moving Average
Ft= W 1 At −1 +W 2 At −2+W 3 At −3
∑ of weights(w)
Weights
Column1
2000 415 w1 3
2001 411 w2 2
2002 376 w3 1.5
2003 335 405
2004 374 383 Sum 6.5
2005 396 363
2006 365 361
2007 338 379
2008 279 373
2009 238 337
2010 212 297
2011 186 251
2012 218
ii. Using a three-point weighted moving average to estimate the 2012 rate (use weightings of
3.0 for the most recent year, 2.0 for the next year, and 1.5 for the last year).
The following formula is adopted
Ft= W 1 At −1 +W 2 At −2+W 3 At −3
∑ of weights(w)
w 1=3 , w 2=2 , w 3=1.5
∑ of weights=3+ 2+ 1.5=6.5
Year
Road
Deaths
3- points Weighted Moving Average
Ft= W 1 At −1 +W 2 At −2+W 3 At −3
∑ of weights(w)
Weights
Column1
2000 415 w1 3
2001 411 w2 2
2002 376 w3 1.5
2003 335 405
2004 374 383 Sum 6.5
2005 396 363
2006 365 361
2007 338 379
2008 279 373
2009 238 337
2010 212 297
2011 186 251
2012 218
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Business Analysis
Hence the rate of death of 2012 will be 218
iii. Use Exponential Smoothing to estimate the 2012 rate. Use a smoothing constant
(α) of 0.4, assume a forecasted value of 400 for the year 2000
In this method, the next Forecasted value ( Ft+ 1) is given by
Ft+ 1=α At + ( 1−α ) Ft , w h ere At ist h e actual value , Ft ist h e forecasted value
α =0.4 ,∧1−α =0.6 , F1=$ 400
Thus, Ft+ 1=0.4 At +0.6 Ft
Year Actual Road Deaths(At) Forecasted value(Ft)
2000 415 400
2001 411 406
2002 376 408
2003 335 395
2004 374 371
2005 396 372
2006 365 382
2007 338 375
2008 279 360
2009 238 328
2010 212 292
2011 186 260
2012 230
The rate of death in 2012 will be 230
iv. Interpretation of the results
The rate of deaths predicted using the 3 methods are:
212, 218 and 230. This gives a range within which the number of deaths forecasted
for 2012 can lie, 212 ≤ X ≤230
Hence the rate of death of 2012 will be 218
iii. Use Exponential Smoothing to estimate the 2012 rate. Use a smoothing constant
(α) of 0.4, assume a forecasted value of 400 for the year 2000
In this method, the next Forecasted value ( Ft+ 1) is given by
Ft+ 1=α At + ( 1−α ) Ft , w h ere At ist h e actual value , Ft ist h e forecasted value
α =0.4 ,∧1−α =0.6 , F1=$ 400
Thus, Ft+ 1=0.4 At +0.6 Ft
Year Actual Road Deaths(At) Forecasted value(Ft)
2000 415 400
2001 411 406
2002 376 408
2003 335 395
2004 374 371
2005 396 372
2006 365 382
2007 338 375
2008 279 360
2009 238 328
2010 212 292
2011 186 260
2012 230
The rate of death in 2012 will be 230
iv. Interpretation of the results
The rate of deaths predicted using the 3 methods are:
212, 218 and 230. This gives a range within which the number of deaths forecasted
for 2012 can lie, 212 ≤ X ≤230
Business Analysis
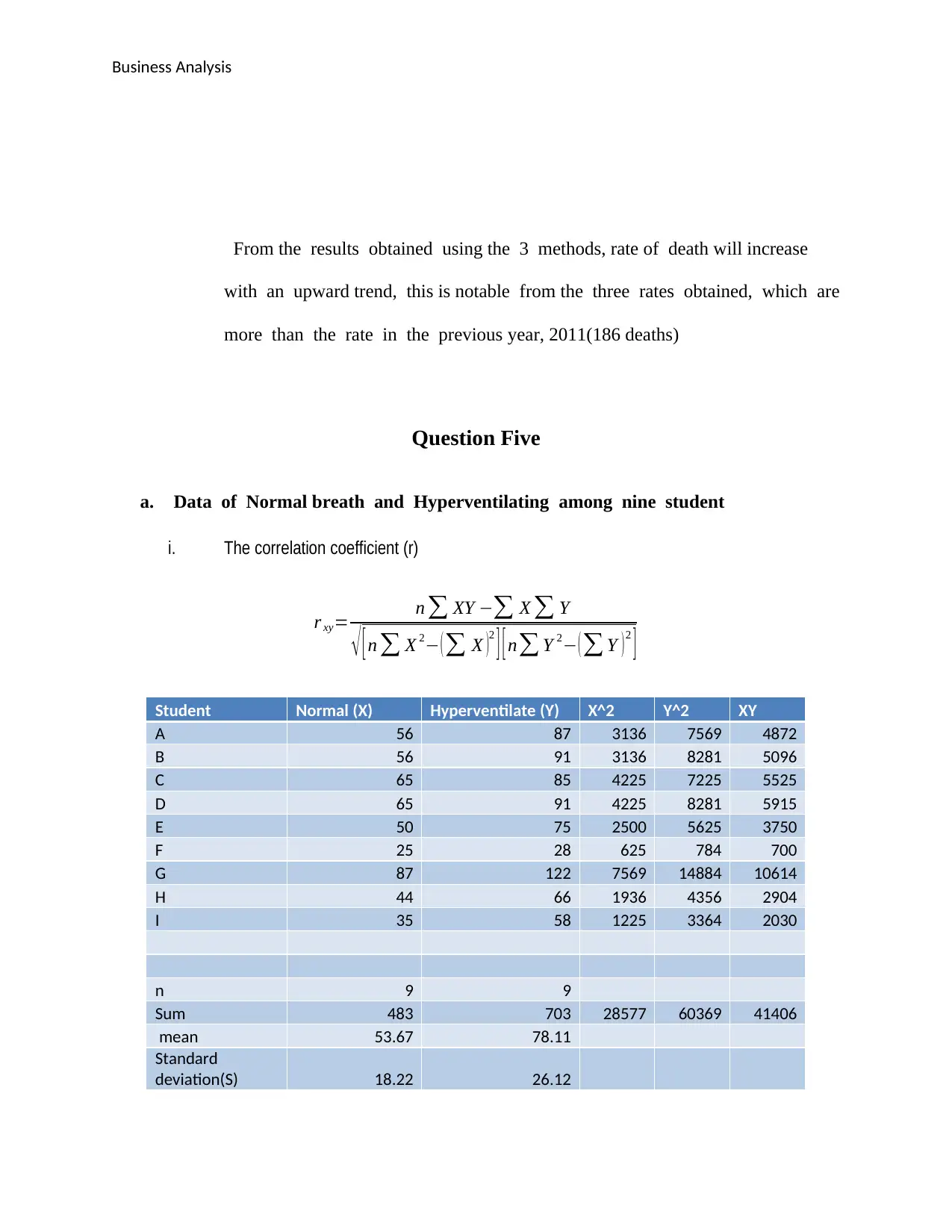
From the results obtained using the 3 methods, rate of death will increase
with an upward trend, this is notable from the three rates obtained, which are
more than the rate in the previous year, 2011(186 deaths)
Question Five
a. Data of Normal breath and Hyperventilating among nine student
i. The correlation coefficient (r)
r xy= n ∑ XY −∑ X ∑ Y
√ [ n ∑ X 2− (∑ X )2
] [ n∑ Y 2− (∑ Y )2
]
Student Normal (X) Hyperventilate (Y) X^2 Y^2 XY
A 56 87 3136 7569 4872
B 56 91 3136 8281 5096
C 65 85 4225 7225 5525
D 65 91 4225 8281 5915
E 50 75 2500 5625 3750
F 25 28 625 784 700
G 87 122 7569 14884 10614
H 44 66 1936 4356 2904
I 35 58 1225 3364 2030
n 9 9
Sum 483 703 28577 60369 41406
mean 53.67 78.11
Standard
deviation(S) 18.22 26.12
From the results obtained using the 3 methods, rate of death will increase
with an upward trend, this is notable from the three rates obtained, which are
more than the rate in the previous year, 2011(186 deaths)
Question Five
a. Data of Normal breath and Hyperventilating among nine student
i. The correlation coefficient (r)
r xy= n ∑ XY −∑ X ∑ Y
√ [ n ∑ X 2− (∑ X )2
] [ n∑ Y 2− (∑ Y )2
]
Student Normal (X) Hyperventilate (Y) X^2 Y^2 XY
A 56 87 3136 7569 4872
B 56 91 3136 8281 5096
C 65 85 4225 7225 5525
D 65 91 4225 8281 5915
E 50 75 2500 5625 3750
F 25 28 625 784 700
G 87 122 7569 14884 10614
H 44 66 1936 4356 2904
I 35 58 1225 3364 2030
n 9 9
Sum 483 703 28577 60369 41406
mean 53.67 78.11
Standard
deviation(S) 18.22 26.12

Business Analysis
r = 9 ( 41406 ) −483 ( 703 )
√ [ 9 ( 28577 ) − ( 483 )
2 ] [ 9 ( 60369 ) − ( 703 ) 2 ]
¿ 33105
√ ( 23904 ) ( 49112 ) = 33105
34263.29 =0.966
Hence the correlation coefficient (r) is 0.966
ii. The slope of the line of best fit
Is given by the b=r s y
sx
, r is correlthe ation coefficient while sx∧s y are the
standard deviations of X and Y respectively, which are already determined in the table in
(i) above, sx=18.22∧sy =26.12
b=r s y
sx
=0.966∗( 26.12
18.22 )
¿ 1.385
iii. The Y-intercept
From the equation of a straight line ( y=a+bx ) it’s given by
a=Y −b X
Y =78.11∧X =53.67, from the table in (i) above
Therefore,
a=Y −b X
¿ 78.11−1.385 ( 53.67 ) =3.787
iv. The value of Y given a value of X = 55 seconds.
Can be obtained by first writing he equation of the line of best fit, which is given
buy the general formula y=a+bx by substing the value of a∧b in this formula
y=3.787 +1.385 x
r = 9 ( 41406 ) −483 ( 703 )
√ [ 9 ( 28577 ) − ( 483 )
2 ] [ 9 ( 60369 ) − ( 703 ) 2 ]
¿ 33105
√ ( 23904 ) ( 49112 ) = 33105
34263.29 =0.966
Hence the correlation coefficient (r) is 0.966
ii. The slope of the line of best fit
Is given by the b=r s y
sx
, r is correlthe ation coefficient while sx∧s y are the
standard deviations of X and Y respectively, which are already determined in the table in
(i) above, sx=18.22∧sy =26.12
b=r s y
sx
=0.966∗( 26.12
18.22 )
¿ 1.385
iii. The Y-intercept
From the equation of a straight line ( y=a+bx ) it’s given by
a=Y −b X
Y =78.11∧X =53.67, from the table in (i) above
Therefore,
a=Y −b X
¿ 78.11−1.385 ( 53.67 ) =3.787
iv. The value of Y given a value of X = 55 seconds.
Can be obtained by first writing he equation of the line of best fit, which is given
buy the general formula y=a+bx by substing the value of a∧b in this formula
y=3.787 +1.385 x
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Business Analysis
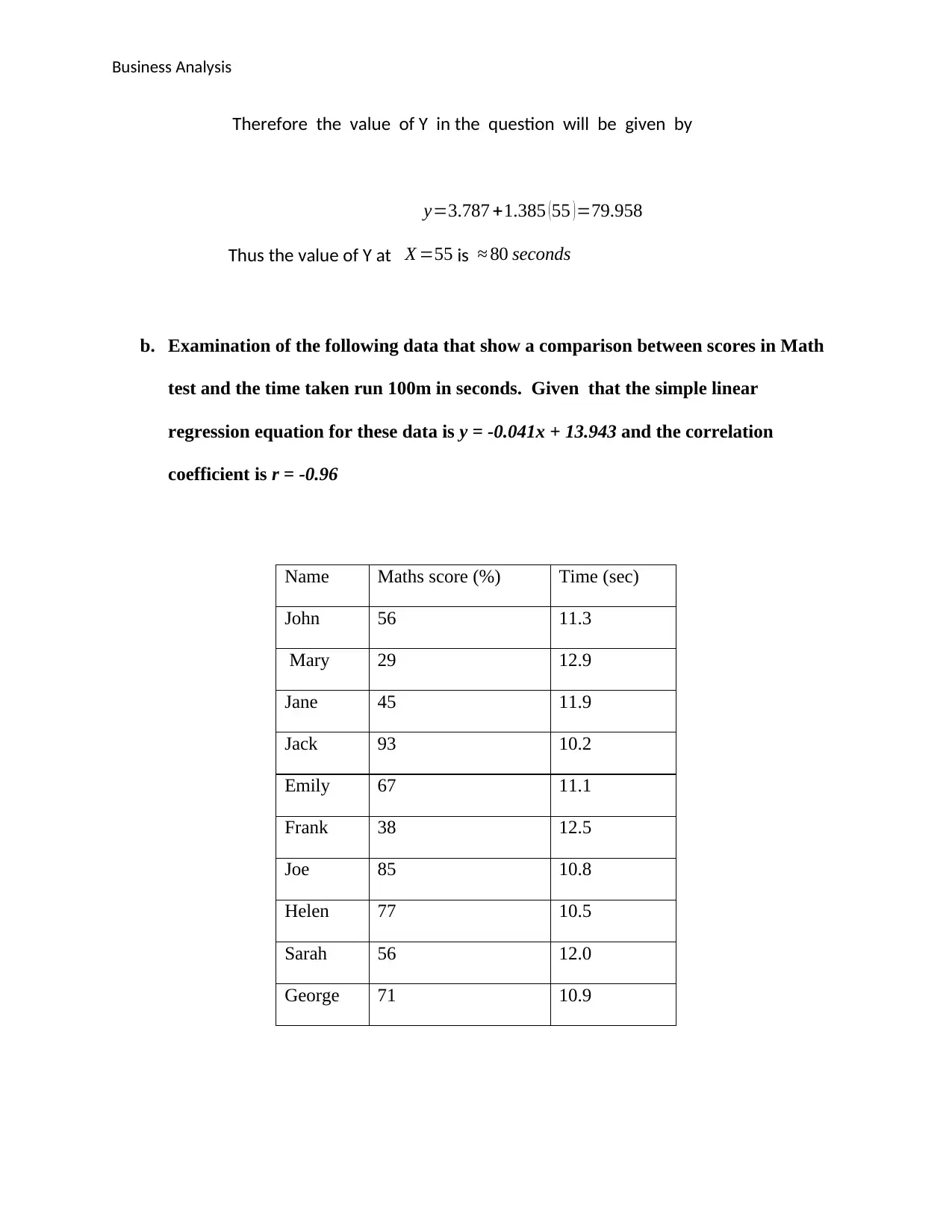
Therefore the value of Y in the question will be given by
y=3.787 +1.385 ( 55 ) =79.958
Thus the value of Y at X =55 is ≈ 80 seconds
b. Examination of the following data that show a comparison between scores in Math
test and the time taken run 100m in seconds. Given that the simple linear
regression equation for these data is y = -0.041x + 13.943 and the correlation
coefficient is r = -0.96
Name Maths score (%) Time (sec)
John 56 11.3
Mary 29 12.9
Jane 45 11.9
Jack 93 10.2
Emily 67 11.1
Frank 38 12.5
Joe 85 10.8
Helen 77 10.5
Sarah 56 12.0
George 71 10.9
Therefore the value of Y in the question will be given by
y=3.787 +1.385 ( 55 ) =79.958
Thus the value of Y at X =55 is ≈ 80 seconds
b. Examination of the following data that show a comparison between scores in Math
test and the time taken run 100m in seconds. Given that the simple linear
regression equation for these data is y = -0.041x + 13.943 and the correlation
coefficient is r = -0.96
Name Maths score (%) Time (sec)
John 56 11.3
Mary 29 12.9
Jane 45 11.9
Jack 93 10.2
Emily 67 11.1
Frank 38 12.5
Joe 85 10.8
Helen 77 10.5
Sarah 56 12.0
George 71 10.9

Business Analysis
Description of the relation between the two variables
The two variables are linearly related since the correlation of coefficient (-0.96) is
greater than 0. At the same time, since the correlation coefficient is closer to -1 than to
0, two variables will be highly associated. Moreover, since the correlation coefficient
and the gradient of the linear regression (-0.041) are negative, the two variables are said
to have a negative linear relationship. Finally, the negative gradient (-0.041) indicates
that the two variables have a negative slope, thus left-right downward slope on the chart.
In conclusion, the two variables, Math scores and time is taken to run 100m, have a high
negative linear relationship due to their negative correlation coefficient(-0.96), which
is close to one, and negative slope(-0.041)
References
Description of the relation between the two variables
The two variables are linearly related since the correlation of coefficient (-0.96) is
greater than 0. At the same time, since the correlation coefficient is closer to -1 than to
0, two variables will be highly associated. Moreover, since the correlation coefficient
and the gradient of the linear regression (-0.041) are negative, the two variables are said
to have a negative linear relationship. Finally, the negative gradient (-0.041) indicates
that the two variables have a negative slope, thus left-right downward slope on the chart.
In conclusion, the two variables, Math scores and time is taken to run 100m, have a high
negative linear relationship due to their negative correlation coefficient(-0.96), which
is close to one, and negative slope(-0.041)
References

Business Analysis
1. Berenson, M., Levine, D., Szabat, K.A. and Krehbiel, T.C., 2012. Basic business
statistics: Concepts and applications. Pearson higher education AU
2. Francis, A., 2004. Business mathematics and statistics. Cengage Learning EMEA.
3. Hassett, M.J. and Stewart, D., 2006. Probability for risk management. Actex
Publications.
4. Montgomery, D.C. and Runger, G.C., 2010. Applied statistics and probability for
engineers. John Wiley & Sons.
5. Tallarida, R.J., and Murray, R.B., 1987. Chi-square test. In Manual of Pharmacologic
Calculations (pp. 140-142). Springer, New York, NY.
6. Wald, A. and Wolfowitz, J., 1940. On a test whether two samples are from the same
population. The Annals of Mathematical Statistics, 11(2), pp.147-162.
7. Weir, B.S. and Cockerham, C.C., 1984. Estimating F‐statistics for the analysis of
population structure. Evolution, 38(6), pp.1358-1370.
8. Yamane, T., 1973. Statistics: An introductory analysis.
1.
1. Berenson, M., Levine, D., Szabat, K.A. and Krehbiel, T.C., 2012. Basic business
statistics: Concepts and applications. Pearson higher education AU
2. Francis, A., 2004. Business mathematics and statistics. Cengage Learning EMEA.
3. Hassett, M.J. and Stewart, D., 2006. Probability for risk management. Actex
Publications.
4. Montgomery, D.C. and Runger, G.C., 2010. Applied statistics and probability for
engineers. John Wiley & Sons.
5. Tallarida, R.J., and Murray, R.B., 1987. Chi-square test. In Manual of Pharmacologic
Calculations (pp. 140-142). Springer, New York, NY.
6. Wald, A. and Wolfowitz, J., 1940. On a test whether two samples are from the same
population. The Annals of Mathematical Statistics, 11(2), pp.147-162.
7. Weir, B.S. and Cockerham, C.C., 1984. Estimating F‐statistics for the analysis of
population structure. Evolution, 38(6), pp.1358-1370.
8. Yamane, T., 1973. Statistics: An introductory analysis.
1.
1 out of 19
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.

