Correlation Analysis in Business Analytics
VerifiedAdded on 2022/12/27
|5
|893
|85
AI Summary
This document discusses the concept of correlation analysis in business analytics. It explains how to calculate correlation coefficients and interpret their values. The document also provides examples and explanations of correlation analysis in different scenarios.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

BUSINESS ANALYTICS
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Question: 1
a. regression equation = y = bx + a
Y = height coefficient*x +intercept
Y = 0.182*x+12.49.
b. If for example, in the above equation, if we substitute x as 28 inches for height, then the
resulting head circumference would be as: y= 0.182*28+12.49= 17.586 inches. Here b is slope of
the regression line and a is the intercept, where the x is the independent and y is the dependent
variable.
c. In the data given as regression statistics, where the coefficient of determination has been given
as R-square and the corresponding value of this is 0.8300.
R-square measures the overall strength and not the extent of association between dependent and
independent variable. The strength of association between variables facilitates the identification
of the proportion of variance in dependent variable that can be resulted from independent
variable. Here the value of R-square is given as 0.83 which can be interpreted as, 83% of the
variance in dependent variable (head circumference) can be anticipated through independent
variable (height).
Also, the same can be calculated as: R2= 1 – RSS / TSS, where RSS is the sum of squares of
residuals and the TSS is the total of sum of squares. So the RSS is given as 0.0818 and the TSS
is given as 0.4818. The resulting value of R2 is 1 - .0818 / .4818 = .83 or 83%.
d. coefficient of correlation can be calculated as follows:
SQRT of coefficient of determination =√R2 = √0.83 = 0.911.
r = 0.911.
e. Head circumference (y) =?
Height (x) = 25.25 inches
b = 0.182, a = 12.49
y = bx + c = 0.182*25.25 + 12.49 = 17.085(head circumference).
Question: 2
1. a. P(0<Z<1.5) = 0.4332.
b. P(1<Z<2)
= P(Z=2) = 0.4772 and P(Z=1) = 0.3413,
P(1<Z<2) = 0.4772 – 0.3413 = 0.1359.
c. P (-1<Z<3) = 0.8399
a. regression equation = y = bx + a
Y = height coefficient*x +intercept
Y = 0.182*x+12.49.
b. If for example, in the above equation, if we substitute x as 28 inches for height, then the
resulting head circumference would be as: y= 0.182*28+12.49= 17.586 inches. Here b is slope of
the regression line and a is the intercept, where the x is the independent and y is the dependent
variable.
c. In the data given as regression statistics, where the coefficient of determination has been given
as R-square and the corresponding value of this is 0.8300.
R-square measures the overall strength and not the extent of association between dependent and
independent variable. The strength of association between variables facilitates the identification
of the proportion of variance in dependent variable that can be resulted from independent
variable. Here the value of R-square is given as 0.83 which can be interpreted as, 83% of the
variance in dependent variable (head circumference) can be anticipated through independent
variable (height).
Also, the same can be calculated as: R2= 1 – RSS / TSS, where RSS is the sum of squares of
residuals and the TSS is the total of sum of squares. So the RSS is given as 0.0818 and the TSS
is given as 0.4818. The resulting value of R2 is 1 - .0818 / .4818 = .83 or 83%.
d. coefficient of correlation can be calculated as follows:
SQRT of coefficient of determination =√R2 = √0.83 = 0.911.
r = 0.911.
e. Head circumference (y) =?
Height (x) = 25.25 inches
b = 0.182, a = 12.49
y = bx + c = 0.182*25.25 + 12.49 = 17.085(head circumference).
Question: 2
1. a. P(0<Z<1.5) = 0.4332.
b. P(1<Z<2)
= P(Z=2) = 0.4772 and P(Z=1) = 0.3413,
P(1<Z<2) = 0.4772 – 0.3413 = 0.1359.
c. P (-1<Z<3) = 0.8399
d. P (Z>1) = 1- 0.8413 = 0.1587.
e. P (Z<2.5) = 0.9937.
2. a.
X = 75, mean = 48.4 and standard deviation = 13.7
Z = x- mean / s.d = 75- 48.4 / 13.7 = 1.94 = 0.9738, 1-0.9738 = 0.026 or 2.62.
No of student obtaining 75 above are 2.62% of 35000 = 917.
b. x = 30
30 -48.4 / 13.7 = -1.34 = 0.090 = 35000 * 9% = 3150.
c. 4% of 35000 = 1400.
Question: 3
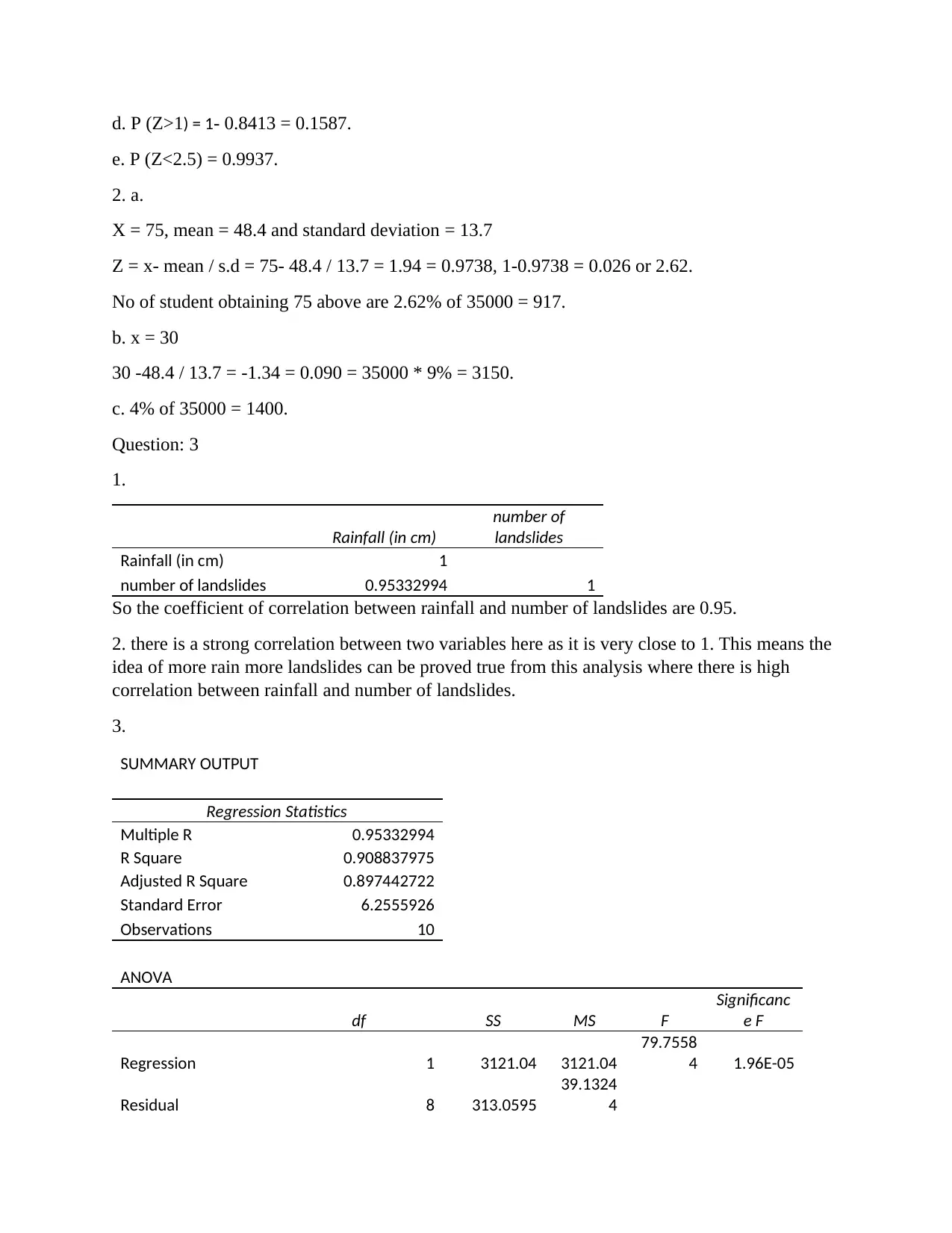
1.
Rainfall (in cm)
number of
landslides
Rainfall (in cm) 1
number of landslides 0.95332994 1
So the coefficient of correlation between rainfall and number of landslides are 0.95.
2. there is a strong correlation between two variables here as it is very close to 1. This means the
idea of more rain more landslides can be proved true from this analysis where there is high
correlation between rainfall and number of landslides.
3.
SUMMARY OUTPUT
Regression Statistics
Multiple R 0.95332994
R Square 0.908837975
Adjusted R Square 0.897442722
Standard Error 6.2555926
Observations 10
ANOVA
df SS MS F
Significanc
e F
Regression 1 3121.04 3121.04
79.7558
4 1.96E-05
Residual 8 313.0595
39.1324
4
e. P (Z<2.5) = 0.9937.
2. a.
X = 75, mean = 48.4 and standard deviation = 13.7
Z = x- mean / s.d = 75- 48.4 / 13.7 = 1.94 = 0.9738, 1-0.9738 = 0.026 or 2.62.
No of student obtaining 75 above are 2.62% of 35000 = 917.
b. x = 30
30 -48.4 / 13.7 = -1.34 = 0.090 = 35000 * 9% = 3150.
c. 4% of 35000 = 1400.
Question: 3
1.
Rainfall (in cm)
number of
landslides
Rainfall (in cm) 1
number of landslides 0.95332994 1
So the coefficient of correlation between rainfall and number of landslides are 0.95.
2. there is a strong correlation between two variables here as it is very close to 1. This means the
idea of more rain more landslides can be proved true from this analysis where there is high
correlation between rainfall and number of landslides.
3.
SUMMARY OUTPUT
Regression Statistics
Multiple R 0.95332994
R Square 0.908837975
Adjusted R Square 0.897442722
Standard Error 6.2555926
Observations 10
ANOVA
df SS MS F
Significanc
e F
Regression 1 3121.04 3121.04
79.7558
4 1.96E-05
Residual 8 313.0595
39.1324
4
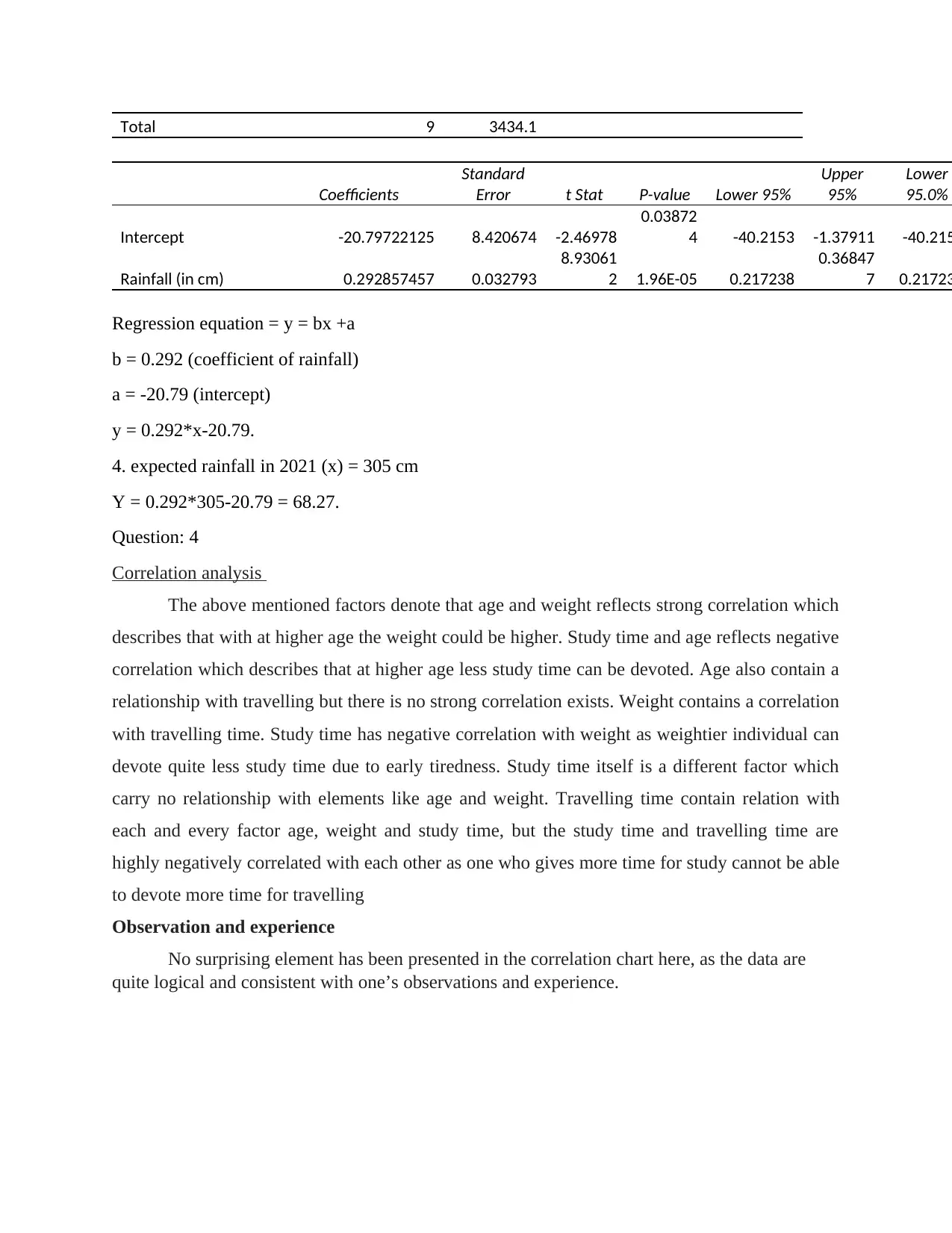
Total 9 3434.1
Coefficients
Standard
Error t Stat P-value Lower 95%
Upper
95%
Lower
95.0%
Intercept -20.79722125 8.420674 -2.46978
0.03872
4 -40.2153 -1.37911 -40.215
Rainfall (in cm) 0.292857457 0.032793
8.93061
2 1.96E-05 0.217238
0.36847
7 0.21723
Regression equation = y = bx +a
b = 0.292 (coefficient of rainfall)
a = -20.79 (intercept)
y = 0.292*x-20.79.
4. expected rainfall in 2021 (x) = 305 cm
Y = 0.292*305-20.79 = 68.27.
Question: 4
Correlation analysis
The above mentioned factors denote that age and weight reflects strong correlation which
describes that with at higher age the weight could be higher. Study time and age reflects negative
correlation which describes that at higher age less study time can be devoted. Age also contain a
relationship with travelling but there is no strong correlation exists. Weight contains a correlation
with travelling time. Study time has negative correlation with weight as weightier individual can
devote quite less study time due to early tiredness. Study time itself is a different factor which
carry no relationship with elements like age and weight. Travelling time contain relation with
each and every factor age, weight and study time, but the study time and travelling time are
highly negatively correlated with each other as one who gives more time for study cannot be able
to devote more time for travelling
Observation and experience
No surprising element has been presented in the correlation chart here, as the data are
quite logical and consistent with one’s observations and experience.
Coefficients
Standard
Error t Stat P-value Lower 95%
Upper
95%
Lower
95.0%
Intercept -20.79722125 8.420674 -2.46978
0.03872
4 -40.2153 -1.37911 -40.215
Rainfall (in cm) 0.292857457 0.032793
8.93061
2 1.96E-05 0.217238
0.36847
7 0.21723
Regression equation = y = bx +a
b = 0.292 (coefficient of rainfall)
a = -20.79 (intercept)
y = 0.292*x-20.79.
4. expected rainfall in 2021 (x) = 305 cm
Y = 0.292*305-20.79 = 68.27.
Question: 4
Correlation analysis
The above mentioned factors denote that age and weight reflects strong correlation which
describes that with at higher age the weight could be higher. Study time and age reflects negative
correlation which describes that at higher age less study time can be devoted. Age also contain a
relationship with travelling but there is no strong correlation exists. Weight contains a correlation
with travelling time. Study time has negative correlation with weight as weightier individual can
devote quite less study time due to early tiredness. Study time itself is a different factor which
carry no relationship with elements like age and weight. Travelling time contain relation with
each and every factor age, weight and study time, but the study time and travelling time are
highly negatively correlated with each other as one who gives more time for study cannot be able
to devote more time for travelling
Observation and experience
No surprising element has been presented in the correlation chart here, as the data are
quite logical and consistent with one’s observations and experience.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

1 out of 5
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.