Mechanical Engineering: Wind Turbine Power Calculation Report
VerifiedAdded on 2022/08/20
|6
|885
|14
Report
AI Summary
This report presents a comprehensive analysis of wind turbine power generation, addressing a scenario where the goal is to determine the necessary wind speed to sufficiently power 500 houses based on given parameters. The report begins by calculating the total power consumption of the 500 hou...

Calculation of the speed of wind required to sufficiently power 500 houses
through wind driven turbines
Table of contents
Contents
Executive summary...................................................................................................1
Introduction...............................................................................................................1
Scenario of the question............................................................................................2
Solution I (Power consumption for 500 houses)....................................................3
Solution II (Required speed of wind).....................................................................3
Solution III (safe speed of blade tips)....................................................................3
Speed of blade tips..............................................................................................4
Conclusion.................................................................................................................5
References.................................................................................................................6
Executive summary
This report presents a detailed analysis and answers to questions in a scenario of
power consumption in households and wind turbines being the source of energy.
Given some turbine parameters such as the number of blades and their length,
other important factors such as speed of wind required is determined by calculation
in order to meet the energy consumption demand for 500 households.
Introduction
through wind driven turbines
Table of contents
Contents
Executive summary...................................................................................................1
Introduction...............................................................................................................1
Scenario of the question............................................................................................2
Solution I (Power consumption for 500 houses)....................................................3
Solution II (Required speed of wind).....................................................................3
Solution III (safe speed of blade tips)....................................................................3
Speed of blade tips..............................................................................................4
Conclusion.................................................................................................................5
References.................................................................................................................6
Executive summary
This report presents a detailed analysis and answers to questions in a scenario of
power consumption in households and wind turbines being the source of energy.
Given some turbine parameters such as the number of blades and their length,
other important factors such as speed of wind required is determined by calculation
in order to meet the energy consumption demand for 500 households.
Introduction
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Power given out (output power) from wind turbines is dependent on factors such as
speed of wind, density of air, size of the wind turbines, the length and radiusof the
turbine rotor blades and efficiency factor.
Regarding speed of wind, reference is made to the speed at one point in time and
not the avearge speed. The density of air depends on humidity, temperature and air
pressure. Efficiency factor is a measure of the amount or part of the wind blowing
through the turbine blades is converted to electricity. The formula therefore used to
work out power output from wind driven turbines is given as; (Casini, 2015)
P= π
2 × r2 × v3 × ρ ×η
Where r denotes the radius of turbine blade, given in meters.
V denotes speed of wind, measured as meters per second (m/s).
Air density ρ in kg /m3
η denotes the efficiency factor.
And P is the output power calculated in Kilowatts.
1 watt= 1kg m2
s3
In order to achieve a high output power, it entirely depends on the speed of wind as
the most important factor.This is evident from the fact that the speed of the wind
taken into consideration goes into the formula as a power of three. The length of
the rotor blade is taken into the calculation squared since it caters for the area
through which the wind blows.
speed of wind, density of air, size of the wind turbines, the length and radiusof the
turbine rotor blades and efficiency factor.
Regarding speed of wind, reference is made to the speed at one point in time and
not the avearge speed. The density of air depends on humidity, temperature and air
pressure. Efficiency factor is a measure of the amount or part of the wind blowing
through the turbine blades is converted to electricity. The formula therefore used to
work out power output from wind driven turbines is given as; (Casini, 2015)
P= π
2 × r2 × v3 × ρ ×η
Where r denotes the radius of turbine blade, given in meters.
V denotes speed of wind, measured as meters per second (m/s).
Air density ρ in kg /m3
η denotes the efficiency factor.
And P is the output power calculated in Kilowatts.
1 watt= 1kg m2
s3
In order to achieve a high output power, it entirely depends on the speed of wind as
the most important factor.This is evident from the fact that the speed of the wind
taken into consideration goes into the formula as a power of three. The length of
the rotor blade is taken into the calculation squared since it caters for the area
through which the wind blows.

Scenario of the question.
In accordance to statistics from British gas company, consumption of electricity in
households on average was established to be 720 kWh on average, over a three-
month period. By making an assumption that the rate of consumption is annually
consistent, the task is to make an estimation of the output power from a wind
turbine that can sufficiently power 500 houses.
Solution I (Power consumption for 500 houses)
1 household consumes at the rate of 720 kWh, therefore for 500 houses, the power
consumption will be;
720 ×500=360,000 kWh
Expected output power from turbine ¿ 360,000 kilowatts .
Solution II (Required speed of wind).
The required speed of wind sufficient to supply power for 500 houses;
P= π
2 × r2 × v3 × ρ ×η
Parameters of the wind driven turbine are:
Efficiency of the turbine (C) is 0.4.
Air density is 1.2 kg/m3
Length of blade is determined by the last digit of student ID + 30 m. (34 m)
Substituting parameters in the power equation we get;
Taking r =34 m
In accordance to statistics from British gas company, consumption of electricity in
households on average was established to be 720 kWh on average, over a three-
month period. By making an assumption that the rate of consumption is annually
consistent, the task is to make an estimation of the output power from a wind
turbine that can sufficiently power 500 houses.
Solution I (Power consumption for 500 houses)
1 household consumes at the rate of 720 kWh, therefore for 500 houses, the power
consumption will be;
720 ×500=360,000 kWh
Expected output power from turbine ¿ 360,000 kilowatts .
Solution II (Required speed of wind).
The required speed of wind sufficient to supply power for 500 houses;
P= π
2 × r2 × v3 × ρ ×η
Parameters of the wind driven turbine are:
Efficiency of the turbine (C) is 0.4.
Air density is 1.2 kg/m3
Length of blade is determined by the last digit of student ID + 30 m. (34 m)
Substituting parameters in the power equation we get;
Taking r =34 m
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide

360,000,000 watts= π
2 × 342 × v3 ×1.2 ×0.4
v3 = 360,000,000× 2
1156 × 3.142×1.2 × 0.4 =412,978.3114
v=74.46 m/ s
Solution III (safe speed of blade tips)
Calculation the safe range of speeds for the blade tips as well as the corresponding
angular velocities in revolutions per minute. The main assumption made is that the
turbine taken into consideration contains 3 blades. The solution also entails a short
explanation of the importance of the speed of blade tips.
Speed of blade tips
The ratio of blade tip speed denotes a ratio between the speed of the tip of rotor
blades and speed of wind. This factor highly affects efficiency of the wind
turbines. For higher tip speeds, it results in high noise levels and thus requiring
strong blades owing to centrifugal forces. (M, 2018)
Basing our approximation on the assumption that the wind turbine has 3 blades,
then the optimum tip speed ration can be estimated to be 5-6.
tip speed ratio ( λ )= blade tip speed
wind speed
5= ts
74.46 , tip speed becomes 372m/ s
For tip speed ratio of 6, then the blade tip speed is;
6= ts
74.46 , tip speed becomes 446.76 m/ s
To find the speed in revolutions per minute (rpm)
2 × 342 × v3 ×1.2 ×0.4
v3 = 360,000,000× 2
1156 × 3.142×1.2 × 0.4 =412,978.3114
v=74.46 m/ s
Solution III (safe speed of blade tips)
Calculation the safe range of speeds for the blade tips as well as the corresponding
angular velocities in revolutions per minute. The main assumption made is that the
turbine taken into consideration contains 3 blades. The solution also entails a short
explanation of the importance of the speed of blade tips.
Speed of blade tips
The ratio of blade tip speed denotes a ratio between the speed of the tip of rotor
blades and speed of wind. This factor highly affects efficiency of the wind
turbines. For higher tip speeds, it results in high noise levels and thus requiring
strong blades owing to centrifugal forces. (M, 2018)
Basing our approximation on the assumption that the wind turbine has 3 blades,
then the optimum tip speed ration can be estimated to be 5-6.
tip speed ratio ( λ )= blade tip speed
wind speed
5= ts
74.46 , tip speed becomes 372m/ s
For tip speed ratio of 6, then the blade tip speed is;
6= ts
74.46 , tip speed becomes 446.76 m/ s
To find the speed in revolutions per minute (rpm)
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Circumference ( πD )= 22
7 ×34 × 2=213.7142 ms
Therefore 1 revolution = 213.7142 m
tip speed becomes 446.76 m
s is equivalent ¿ 26,805.6 meters/minute
rpm ( revolutions per minute )= 26,805.6 m/min
213.7142 m =125.42 rpm
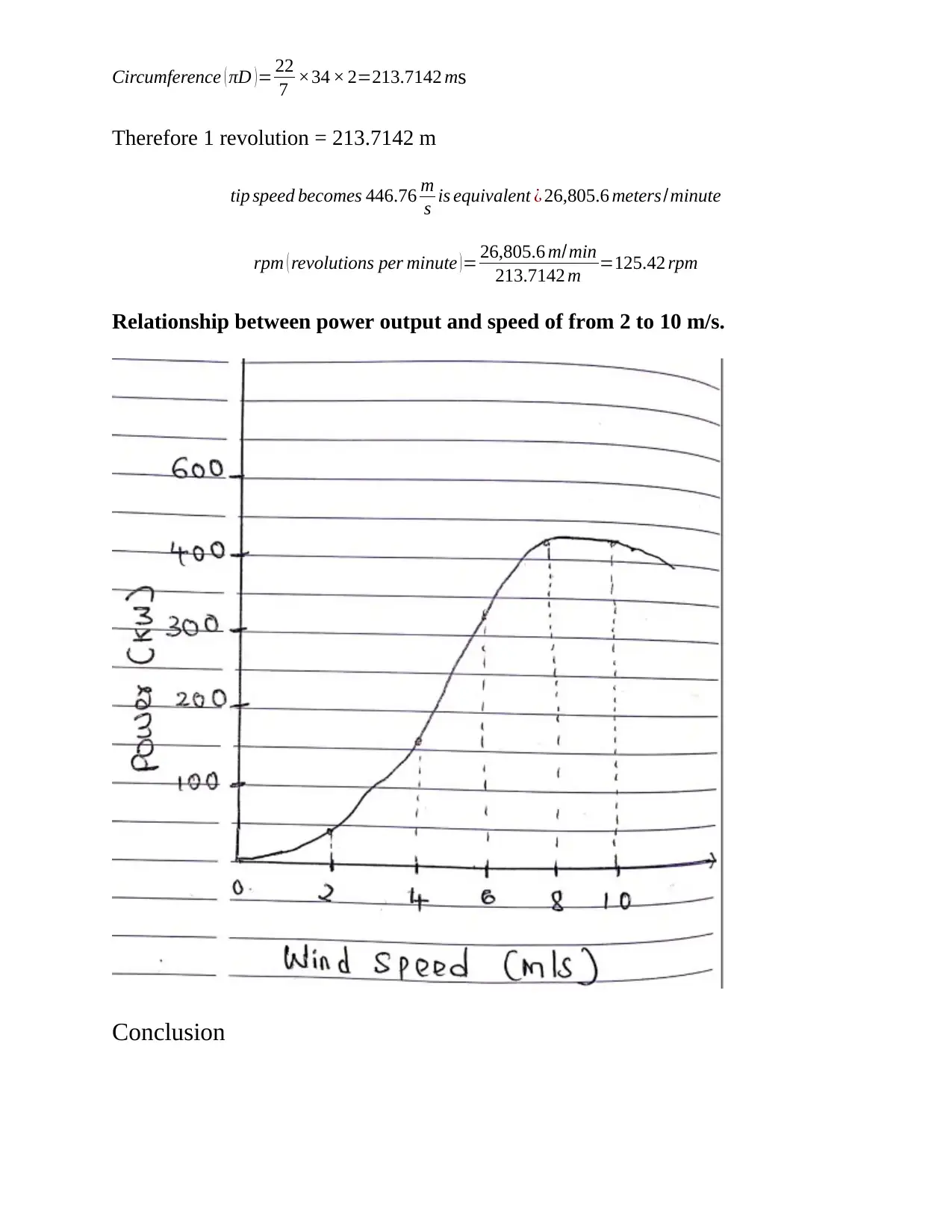
Relationship between power output and speed of from 2 to 10 m/s.
Conclusion
7 ×34 × 2=213.7142 ms
Therefore 1 revolution = 213.7142 m
tip speed becomes 446.76 m
s is equivalent ¿ 26,805.6 meters/minute
rpm ( revolutions per minute )= 26,805.6 m/min
213.7142 m =125.42 rpm
Relationship between power output and speed of from 2 to 10 m/s.
Conclusion

From the analysis, the required speed of wind that will drive the turbines into
powering 500 homes sufficiently was determined to be 74.46 m/s. An important
observation from the analysis is that the blade tips speed also determined the
threshold for maximum power generation speed without noise (losses). Beyond the
threshold speed, the efficiency of power generation reduces.
References
Casini, M., 2015. Small Vertical Axis Wind Turbines for Energy Efficiency of
Buildings. Journal of Clean Energy Technologies, 4(1), pp. 56-65.
M, X., 2018. Wind turbines output power smoothing using embedded energy
storage systems. Energypo systems, 4(3), pp. 58-75.
powering 500 homes sufficiently was determined to be 74.46 m/s. An important
observation from the analysis is that the blade tips speed also determined the
threshold for maximum power generation speed without noise (losses). Beyond the
threshold speed, the efficiency of power generation reduces.
References
Casini, M., 2015. Small Vertical Axis Wind Turbines for Energy Efficiency of
Buildings. Journal of Clean Energy Technologies, 4(1), pp. 56-65.
M, X., 2018. Wind turbines output power smoothing using embedded energy
storage systems. Energypo systems, 4(3), pp. 58-75.
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
1 out of 6
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.
