Differentiation of Functions

This document explains how to differentiate functions and find their derivatives using the given graphs. It provides step-by-step instructions and examples for estimating the derivatives of h(x) at x=1 and x=3.

Calculus
Name:
Institution:
15th March 2019

Question 1:
(a) State two formulas for the derivative
Solution
 The first formula is the power Rule of Derivatives. According to this formula we
have;
d
dx [ xn ]=n xn−1
 The second formula of the derivative is by the limit. According to this formula we
have;
f ' ( x ) = d
dx [ f (x) ] lim
h → 0
f ( x +h ) −f (x )
h
(b) For f ( x )= 3 x
2−x find f ' ( x ) using the limit definition of a derivative
Solution
The limit definition of the derivative states that for a function f(x) its derivative equals
f ' ( x )=lim
h →0
f ( x+h )−f (x)
h
f ' ( x ) =lim
h →0
1
h ( 3 (x+h)
2−x +h − 3 x
2−x )
¿ lim
h→ 0
1
h ( ( 3 x+3 h ) ( 2−x ) −3 x(2−x+ h)
( 2−x+h)(2−x) )
¿ lim
h→ 0
1
h ( ( 6 x +6 h−3 x2−3 xh ) −(6 x −3 x2 +3 xh)
(2−x+h)(2−x ) )
¿ lim
h→ 0
1
h ( 6 h−6 xh
(2−x+h)(2−x) )
¿ lim
h→ 0
6 ( 1−x
(2−x+ h)(2−x) )
¿ 6
(x−2)( x−2)
¿ 6
( x−2)2

Question 2:
Differentiate the following:
(a) y=7 t 3 +15 et
Solution
y' = dy
dx = dy
dx [ 7 t3 +15 et ]
¿ 7. dy
dt [ t3 ] +15. dy
dt [ et ] =7∗3 t2 +15 et
¿ 21 t2 +15 et
(b) f ( x ) = ( ln ( 3 ) ) x
Solution
f ' ( x )= d
dx [ ( ln (3 ) )x
]
¿ d
dx ¿
¿ e¿¿ ¿
¿∈¿
¿ 1∈¿
¿∈¿
(c) g ( x ) =e9 + xe
Solution
g' ( x)= d
dx [ e9 + xe ]
¿ d
dx [ e9 ]+ d
dx [ xe ]
¿ 0+ d
dx [ xe ]
¿ d
dx [ xe ]
¿ e xe−1

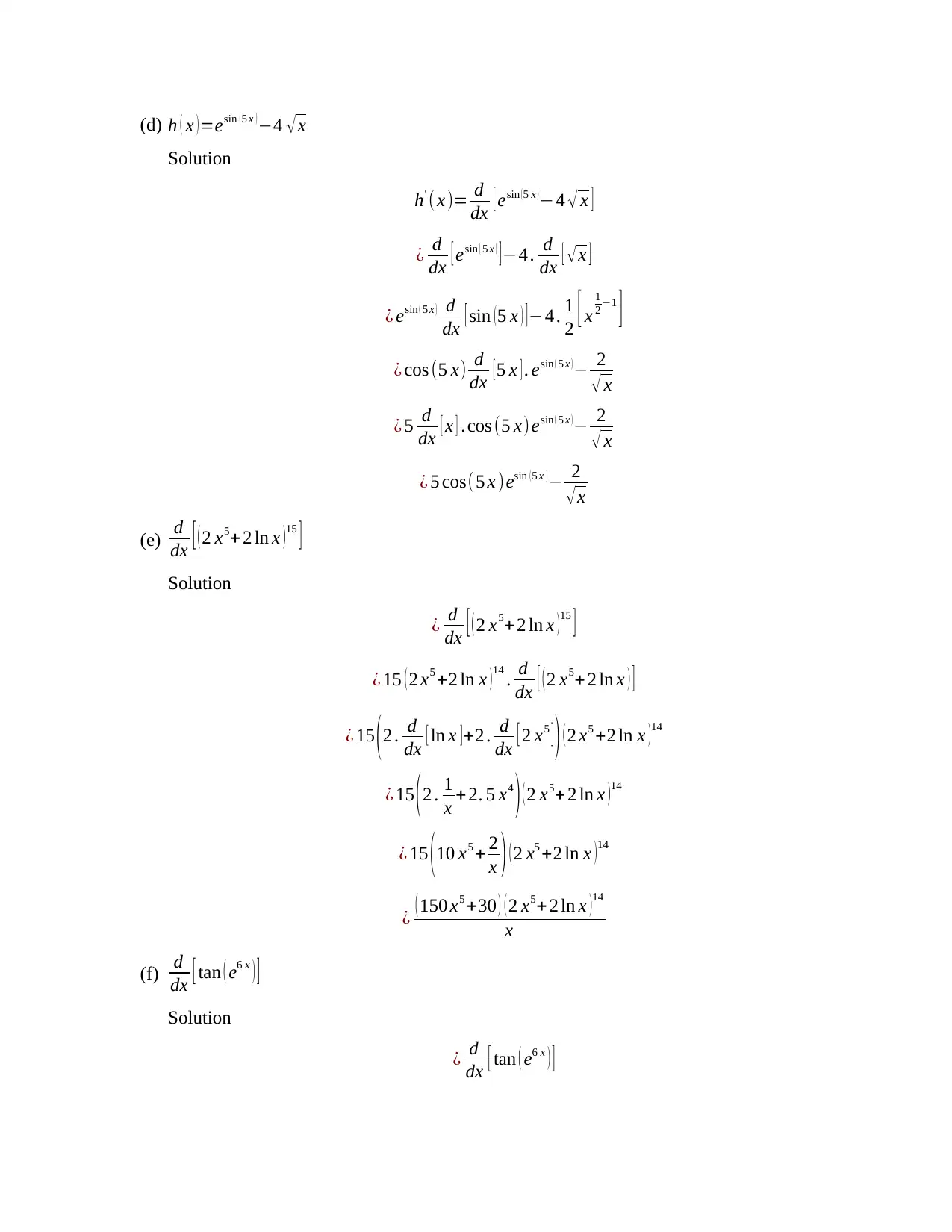
(d) h ( x ) =esin ( 5 x ) −4 √ x
Solution
h' ( x )= d
dx [ esin (5 x )−4 √ x ]
¿ d
dx [ esin ( 5 x ) ]−4 . d
dx [ √ x ]
¿ esin ( 5 x ) d
dx [ sin (5 x ) ]−4 . 1
2 [ x
1
2 −1
]
¿ cos (5 x) d
dx [5 x ] . esin ( 5 x )− 2
√ x
¿ 5 d
dx [ x ] .cos (5 x)esin ( 5 x )− 2
√ x
¿ 5 cos(5 x )esin ( 5 x ) − 2
√ x
(e) d
dx [ ( 2 x5+ 2 ln x )
15
]
Solution
¿ d
dx [ ( 2 x5+2 ln x )15
]
¿ 15 ( 2 x5 +2 ln x ) 14
. d
dx [ ( 2 x5+ 2 ln x ) ]
¿ 15 ( 2 . d
dx [ ln x ] +2 . d
dx [ 2 x5 ] ) ( 2 x5 +2 ln x )
14
¿ 15 ( 2 . 1
x + 2. 5 x4
) ( 2 x5+ 2 ln x )
14
¿ 15 (10 x5 + 2
x ) ( 2 x5 +2 ln x )14
¿ ( 150 x5 +30 ) ( 2 x5+ 2 ln x )
14
x
(f) d
dx [ tan ( e6 x ) ]
Solution
¿ d
dx [ tan ( e6 x ) ]

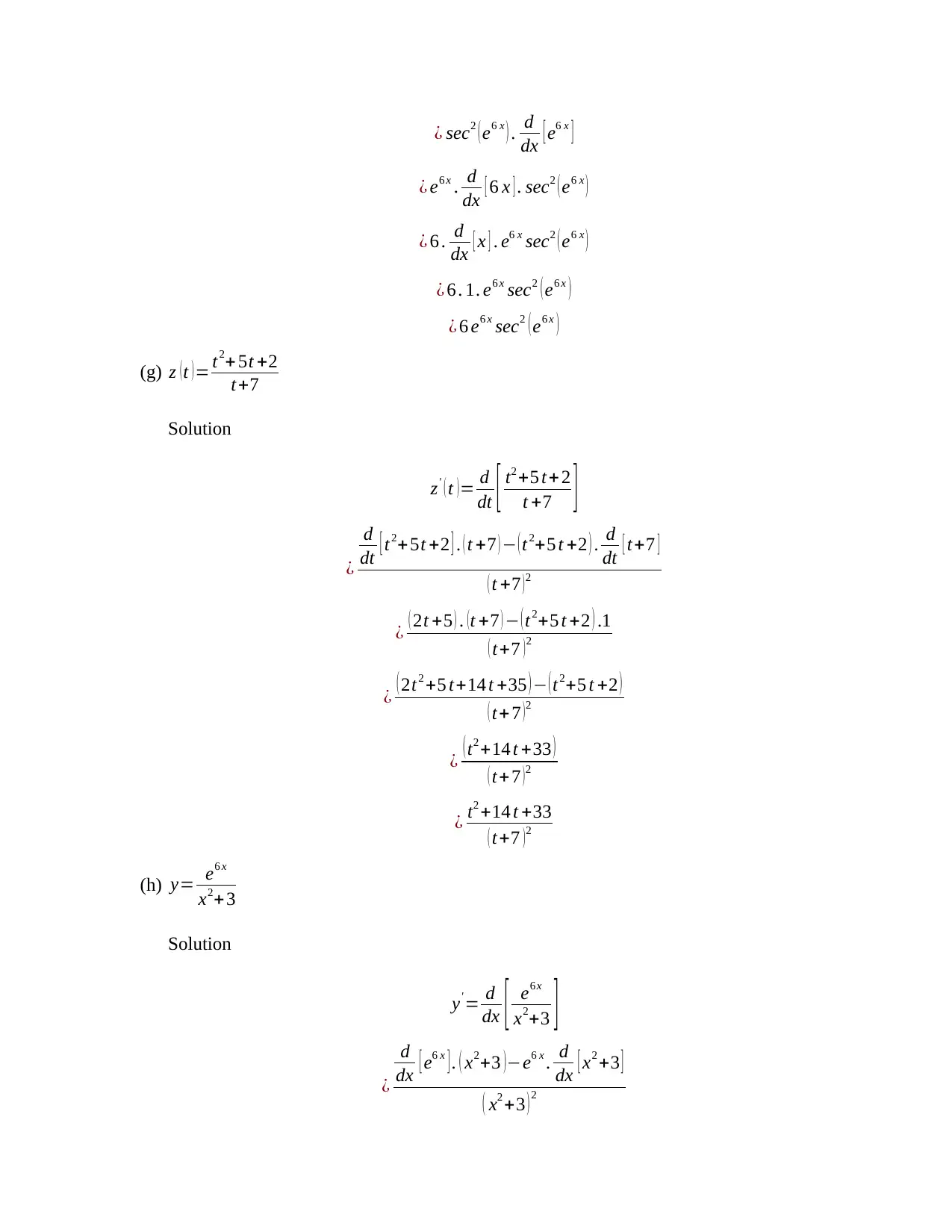
¿ sec2 ( e6 x ) . d
dx [ e6 x ]
¿ e6 x . d
dx [ 6 x ] . sec2 ( e6 x )
¿ 6 . d
dx [ x ] . e6 x sec2 ( e6 x )
¿ 6 . 1. e6 x sec2 ( e6 x )
¿ 6 e6 x sec2 ( e6 x )
(g) z (t )= t2+ 5t +2
t+7
Solution
z' ( t )= d
dt [ t2 +5 t+ 2
t +7 ]
¿
d
dt [ t2+ 5t +2 ] . ( t +7 ) − ( t2+5 t +2 ) . d
dt [ t+7 ]
( t +7 ) 2
¿ ( 2t +5 ) . ( t +7 ) − ( t2+5 t +2 ) .1
( t+7 ) 2
¿ ( 2t2 +5 t+14 t +35 ) − (t2+5 t +2 )
( t+ 7 )2
¿ ( t2 +14 t +33 )
( t+ 7 ) 2
¿ t2 +14 t +33
( t+7 ) 2
(h) y= e6 x
x2+3
Solution
y' = d
dx [ e6 x
x2+3 ]
¿
d
dx [ e6 x ] . ( x2+3 )−e6 x . d
dx [ x2 +3 ]
( x2 +3 ) 2

¿
6 . d
dx [ x ] . ( x2+ 3 ) e6 x− ( 2 x+0 ) e6 x
( x2 +3 )
2
¿ 6 ( x2+3 ) e6 x−2 x e6 x
( x2+3 )2
(i) r ( θ ) =e6 cos θ
Solution
r' ( θ )= d
dθ [ e6 cosθ ]
¿ e6 cos θ . d
dθ [6 cos θ ]
¿ 6 . d
dθ [ cos θ ] e6 cos θ
¿ 6 . ¿
¿−6 e6 cos θ sin θ
Question 3:
Consider function f ( x )=e−x2
(a) Find f ' ( x )
Solution
f ' ( x )= d
dx [ e− x2
]
¿ e− x2
. d
dx [−x2 ]
¿ (−d
dx [ x2 ] )e−x2
¿ (−2 x ) e− x2
¿−2 x e−x2
(b) Find f ' ' ( x )
Solution

f ' ' ( x ) = d
dx [ −2 x e−x2
]
¿−2 . d
dx [ x e−x2
]
¿−2 ( d
dx [ x ] . e−x2
+ x . d
dx [ e−x2
] )
¿−2 ( e− x2
+ e−x2
. d
dx [ −x2 ] . x )
¿−2 ( −2 x2 e−x2
+e− x2
)
¿−2 ( e−x2
−2 x2 e− x2
)
Question 4:
Find the equation of the tangent line to the graph at the point z = 2 for
g ( z )= 3 z2
5 z2 +2 z
Solution
g' ( z ) = d
dz [ 3 z2
5 z2 +2 z ]
¿ 3 . d
dz [ z2
5 z2+ 2 z ]
¿ 3 .
d
dz [ z2 ] . ( 5 z2+2 z )−z2 . d
dz [ 5 z2 +2 z ]
( 5 z2 +2 z )
2
¿ 3 . 2 z . ( 5 z2+ 2 z )−z2 . ( 10 z +2 )
( 5 z2+ 2 z )2
¿ 6 z . ( 5 z2+2 z )−3 z2 . ( 10 z +2 )
( 5 z2 +2 z ) 2

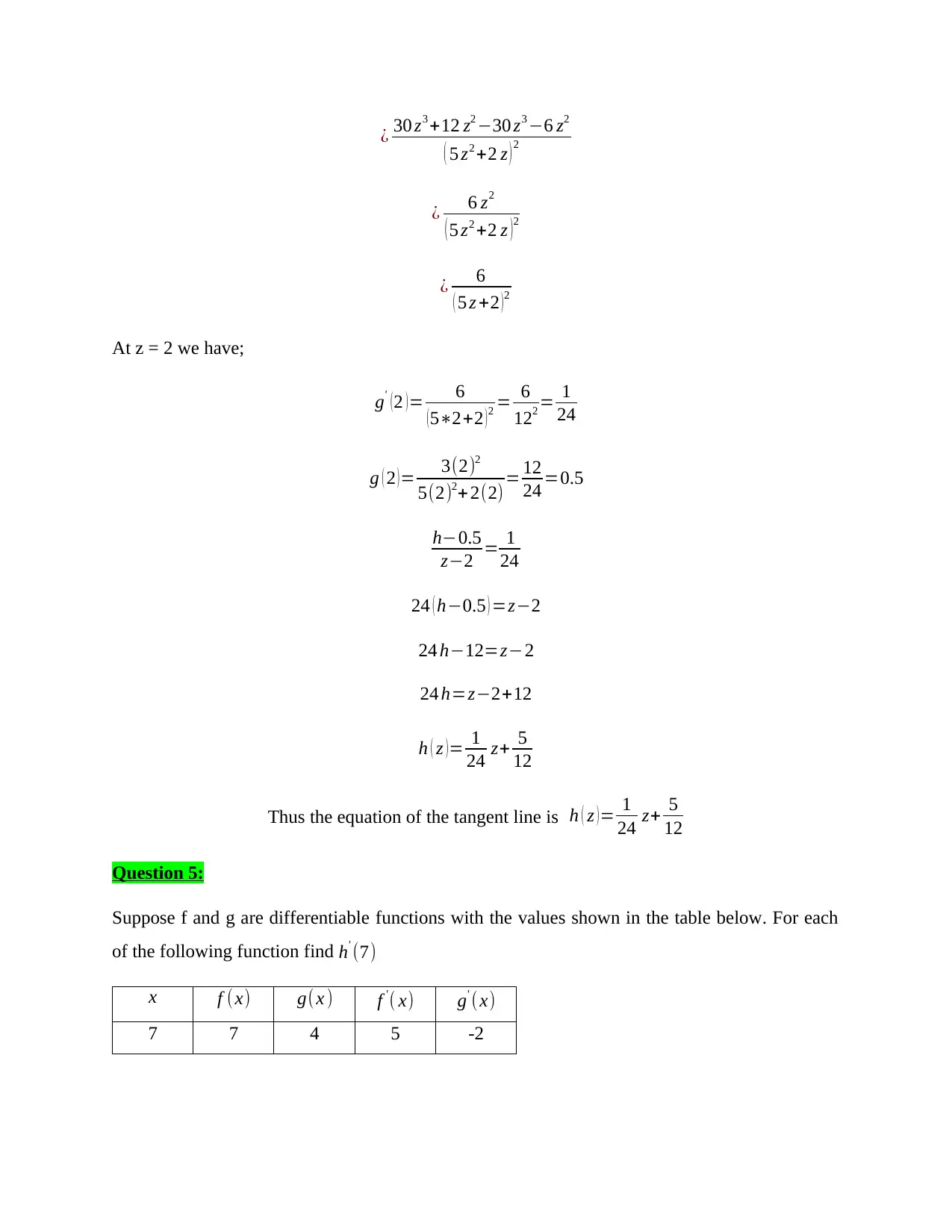
¿ 30 z3 +12 z2 −30 z3 −6 z2
( 5 z2 +2 z ) 2
¿ 6 z2
( 5 z2 +2 z )2
¿ 6
( 5 z +2 )2
At z = 2 we have;
g' ( 2 ) = 6
( 5∗2+2 ) 2 = 6
122 = 1
24
g ( 2 ) = 3(2)2
5(2)2+ 2(2)= 12
24 =0.5
h−0.5
z−2 = 1
24
24 ( h−0.5 ) =z−2
24 h−12=z−2
24 h=z−2+12
h ( z ) = 1
24 z+ 5
12
Thus the equation of the tangent line is h ( z ) = 1
24 z+ 5
12
Question 5:
Suppose f and g are differentiable functions with the values shown in the table below. For each
of the following function find h' (7)
x f (x) g(x ) f ' ( x) g' (x)
7 7 4 5 -2

(a) h ( x ) =3 f ( x ) −2 g(x )
Solution
h' ( x ) =3 f ' ( x ) −2 g'( x )
h' ( 7 ) =3 ( 5 ) −2 ( −2 ) =15+4=19
(b) h ( x )=f ( x ) g( x)
Solution
h' ( x )=f ' ( x ) g' ( x )
h' ( 7 )=5∗(−2 ) =−10
(c) h ( x )= f ( x )
g ( x )
Solution
h' ( 7 )= f ' ( 7 )
g' (7 ) = 5
−2=−2.5
(d) h ( x )= 3+f ( x )
1−g ( x )
Solution
h' ( 7 ) = 3+ f ' ( 7 )
1−g' ( 7 ) = 3+5
1−(−2)= 8
3
Question 6:
Let h ( x ) =f ( x ) g( x), and graphs of f ( x ) and g ( x ) are provided below. Using the graphs
estimate the following derivatives.
(a) h' ( 1 )
Solution
If h ( x )=f ( x ) g(x), then h' ( x )=f ' ( x ) g ( x )+ f ( x ) g' ( x )
h' ( 1 )=f ' ( 1 ) g ( 1 ) + f ( 1 ) g' (1)

The values of the functions must be
f ( 1 ) =2∧g ( 1 )=3
f ' ( 1 )= ∆ y
∆ x = 2
1 =2
g' ( 1 ) = ∆ y
∆ x =−1
1 =−1
h' ( 1 ) =2∗3+2∗( −1 ) =6−2=4
(b) h' ( 3 )
Solution
If h ( x ) =f ( x ) g(x), then h' ( x )=f ' ( x ) g ( x )+ f ( x ) g' ( x )
h' ( 3 )=f ' ( 3 ) g ( 3 ) + f ( 3 ) g' (3)
The values of the functions must be
f ( 3 )=2∧g ( 3 ) =1
f ' ( 3 ) = ∆ y
∆ x = 2
−3 =−2
3
g' (3 )= ∆ y
∆ x =−1
3 =−1
3
h' ( 3 )=−2
3 ∗1+2∗(−1
3 )=−2
3 − 2
3 =−4
3
(c) For which value of x on the interval (0, 4) derivative of h(x) does not exist? Why?
Solution
For the value of x = 0, the derivative of h(x) does not exist because the tangent at x = 0 is
vertical and therefore its slope which the value of the derivative at x =0 is undefined.
Question 7:
Suppose f ( 3 )=4 and f ' ( 3 ) =9. Find the derivative of the following functions.
(a) g ( x ) = √ f ( x )
Solution
g ( x )= √f (x )=f ( x)1 /2

g' ( x ) =1
2 f (x )−1/ 2 ( f ( x )1/ 2 )'
Substituting x = 3 into the above we obtain;
g' (3 )= 1
2 f (3)−1/ 2 ( f (3)1 /2 )'
= 1
2∗4
−1
2 ∗9
1
2 =
1
2∗1
2 ∗3= 3
4
(b) g ( x ) = 1
4 f (x)
Solution
g ( x )= 1
4 f ( x)
g' ( x ) = f ' (x )
4 ( f (x) ) 2
Substituting x = 3 into the above we obtain;
g' (3 )= f ' (3)
4 ( f (3) )2 = 9
4∗42 = 9
64