CHANGE OF VARIABLE IN MULTIPLE INTEGRALS.

CHANGE OF VARIABLE IN MULTIPLE INTEGRALS
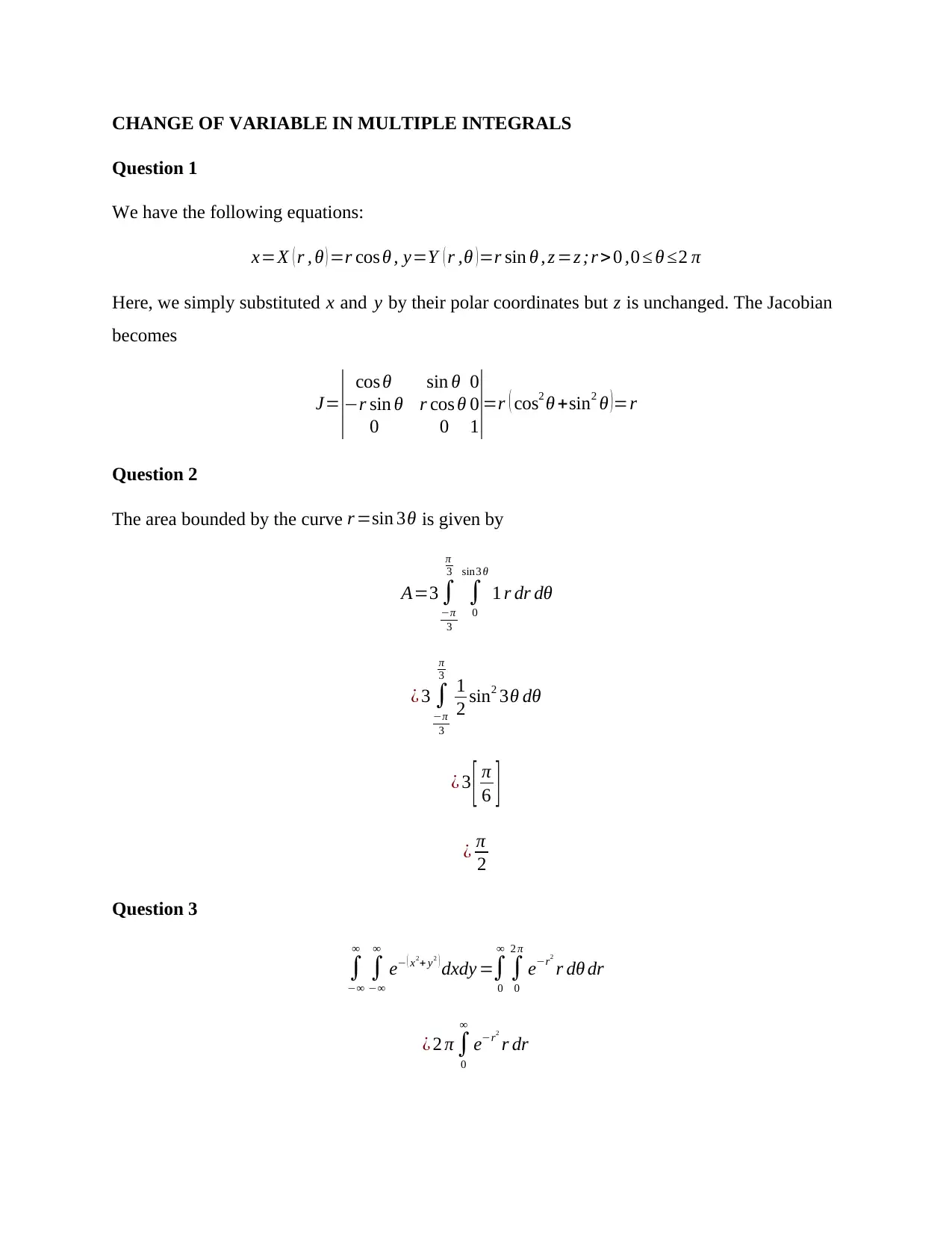
Question 1
We have the following equations:
x=X ( r , θ ) =r cos θ , y=Y ( r ,θ ) =r sin θ , z =z ;r > 0 ,0 ≤ θ ≤2 π
Here, we simply substituted x and y by their polar coordinates but z is unchanged. The Jacobian
becomes
J=
| cos θ sin θ
−r sin θ
0
r cos θ
0
0
0
1|=r ( cos2 θ +sin2 θ )=r
Question 2
The area bounded by the curve r =sin 3θ is given by
A=3 ∫
−π
3
π
3
∫
0
sin3 θ
1 r dr dθ
¿ 3 ∫
−π
3
π
3
1
2 sin2 3θ dθ
¿ 3 [ π
6 ]
¿ π
2
Question 3
∫
−∞
∞
∫
−∞
∞
e− ( x2+ y2 ) dxdy =∫
0
∞
∫
0
2 π
e−r2
r dθ dr
¿ 2 π ∫
0
∞
e−r2
r dr

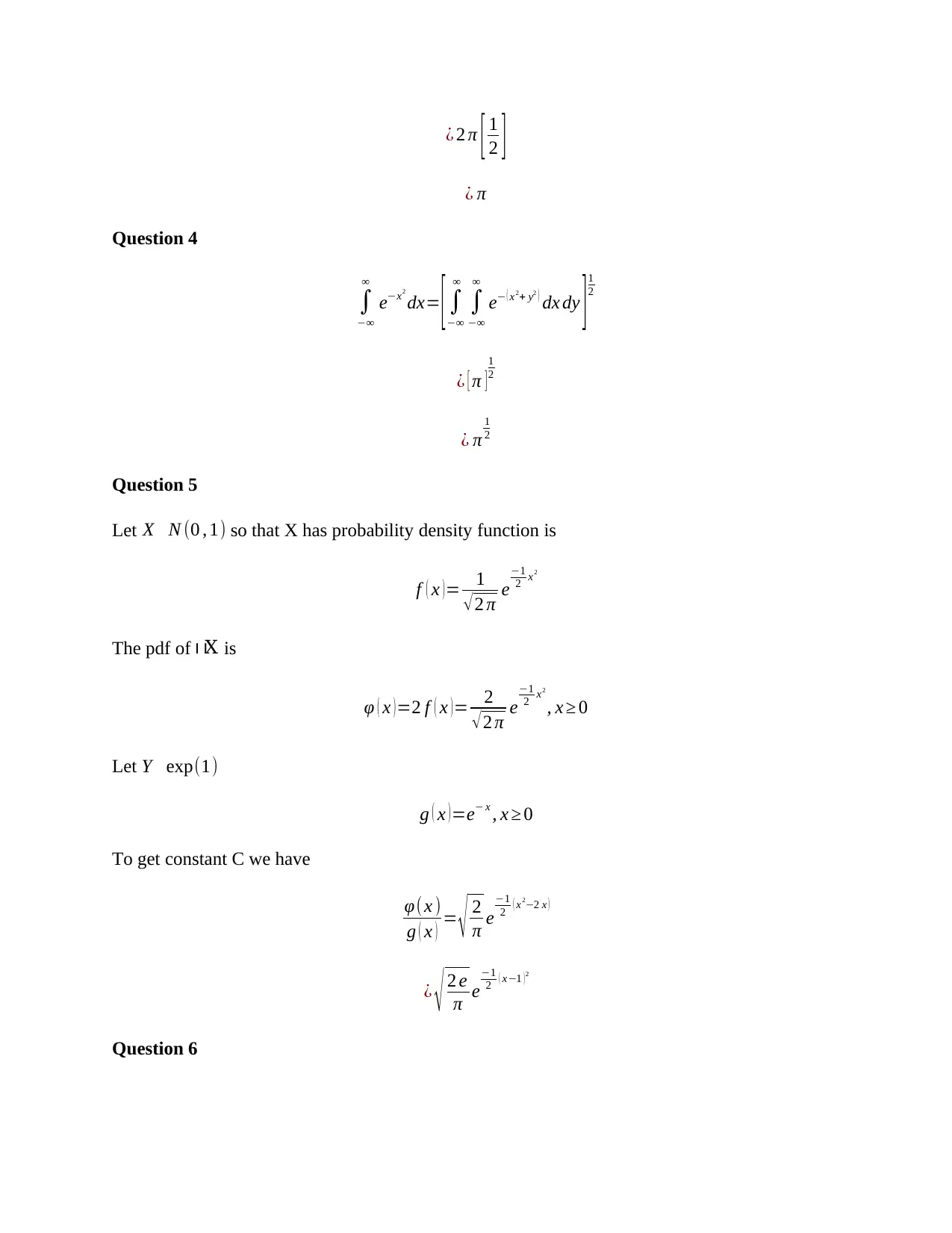
¿ 2 π [ 1
2 ]
¿ π
Question 4
∫
−∞
∞
e−x2
dx= [∫
−∞
∞
∫
−∞
∞
e− ( x2+ y2 ) dx dy ]1
2
¿ [ π ]
1
2
¿ π
1
2
Question 5
Let X N (0 , 1) so that X has probability density function is
f ( x )= 1
√2 π e
−1
2 x2
The pdf of ⃓ X⃓ is
φ ( x ) =2 f ( x ) = 2
√ 2 π e
−1
2 x2
, x ≥ 0
Let Y exp(1)
g ( x )=e− x , x ≥ 0
To get constant C we have
φ(x )
g ( x ) = √ 2
π e
−1
2 ( x2−2 x )
¿ √ 2 e
π e
−1
2 ( x−1 ) 2
Question 6

∫
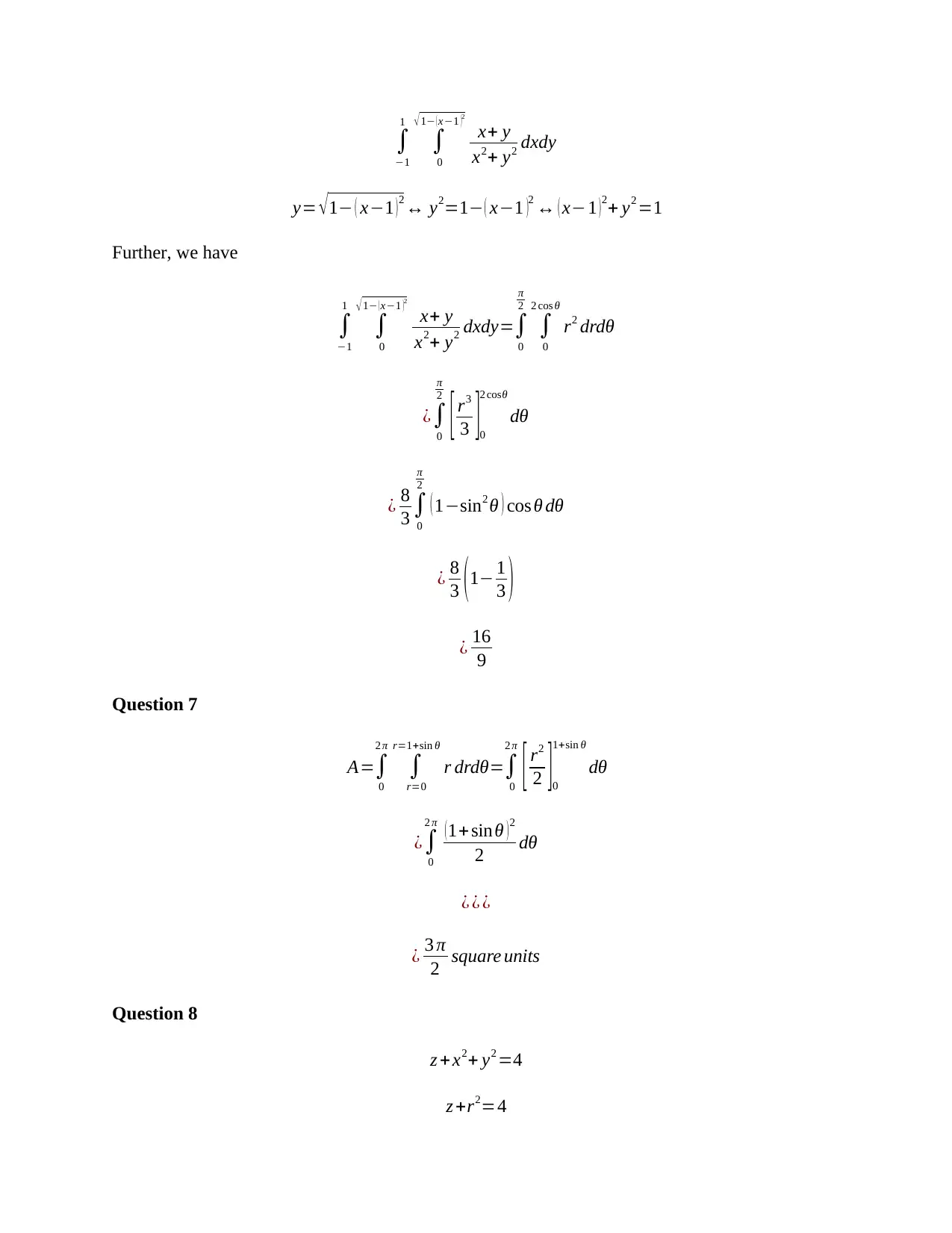
−1
1
∫
0
√ 1− ( x−1 )
2
x+ y
x2+ y2 dxdy
y= √1− ( x−1 )2 ↔ y2=1− ( x−1 )2 ↔ ( x−1 )2+ y2 =1
Further, we have
∫
−1
1
∫
0
√ 1− ( x−1 )2
x+ y
x2+ y2 dxdy=∫
0
π
2
∫
0
2 cos θ
r2 drdθ
¿∫
0
π
2
[ r3
3 ]0
2 cosθ
dθ
¿ 8
3 ∫
0
π
2
( 1−sin2 θ ) cos θ dθ
¿ 8
3 ( 1− 1
3 )
¿ 16
9
Question 7
A=∫
0
2 π
∫
r=0
r=1+sin θ
r drdθ=∫
0
2 π
[ r2
2 ]0
1+sin θ
dθ
¿∫
0
2 π ( 1+ sin θ ) 2
2 dθ
¿ ¿ ¿
¿ 3 π
2 square units
Question 8
z +x2+ y2 =4
z +r2=4

Equation of cylinder is x2+ y2=1∨r2 =1
We have region E
E= { ( r , θ , z ) 0 ≤ z ≤ 4−r2 , 0≤ r ≤ 1, 0 ≤ θ≤ 2 π }
Integral for the volume is
V ( E ) =∫
0
2 π
∫
0
1
∫
0
4 −r2
r dzdrdθ
¿∫
0
2 π
∫
0
1
[ rz ] 0
4−r2
drdθ
¿∫
0
2 π
∫
0
1
(r ( 4−r2 ) ) drdθ
¿∫
0
2 π
[r2 −r3
3 ]0
1
dθ
¿∫
0
2 π
5
3 dθ
¿ [ 5
3 θ ]0
2 π
¿ 10 π
3 cubic units
Question 9
We find the Jacobian of the transformation ( r , θ ,∅ ) →(x , y , z) of spherical coordinates. Our
partial derivatives are;
∂ x
∂r =cos θ sin∅ , ∂ x
∂θ =−r sin θ sin ∅ , ∂ x
∂∅ =r cos θ cos ∅
∂ y
∂ r =sinθ sin∅ , ∂ y
∂ θ =r cos θ sin ∅ , ∂ y
∂ ∅ =r sin θ cos ∅

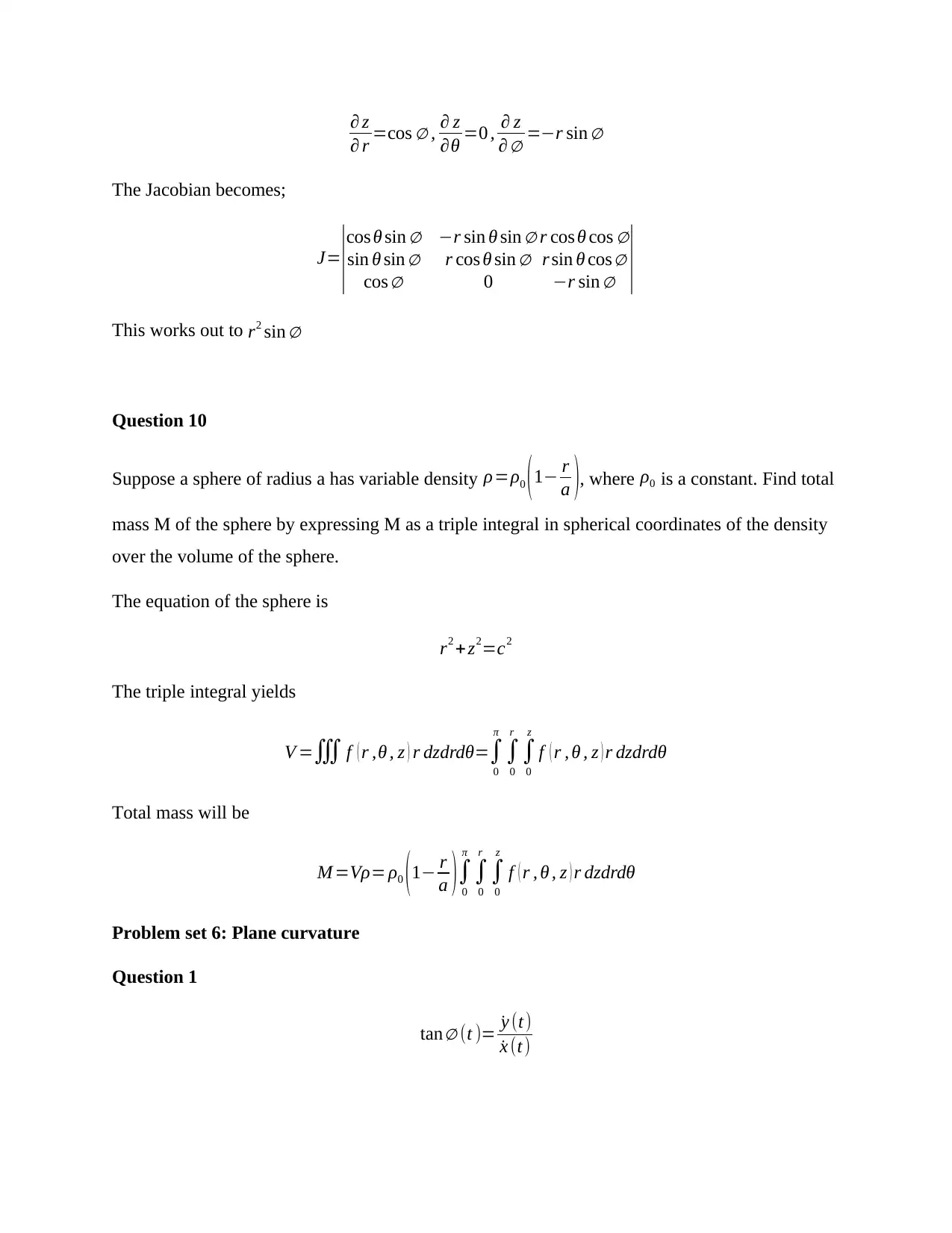
∂ z
∂ r =cos ∅ , ∂ z
∂θ =0 , ∂ z
∂ ∅ =−r sin ∅
The Jacobian becomes;
J=
|
cos θ sin ∅ −r sin θ sin ∅
sin θ sin ∅
cos ∅
r cos θ sin ∅
0
r cos θ cos ∅
r sin θ cos ∅
−r sin ∅ |
This works out to r2 sin ∅
Question 10
Suppose a sphere of radius a has variable density ρ=ρ0 (1− r
a ), where ρ0 is a constant. Find total
mass M of the sphere by expressing M as a triple integral in spherical coordinates of the density
over the volume of the sphere.
The equation of the sphere is
r2 + z2=c2
The triple integral yields
V =∭ f ( r ,θ , z ) r dzdrdθ=∫
0
π
∫
0
r
∫
0
z
f ( r , θ , z ) r dzdrdθ
Total mass will be
M =Vρ= ρ0 (1− r
a )∫
0
π
∫
0
r
∫
0
z
f ( r , θ , z ) r dzdrdθ
Problem set 6: Plane curvature
Question 1
tan∅ (t )= ˙y (t)
˙x (t)

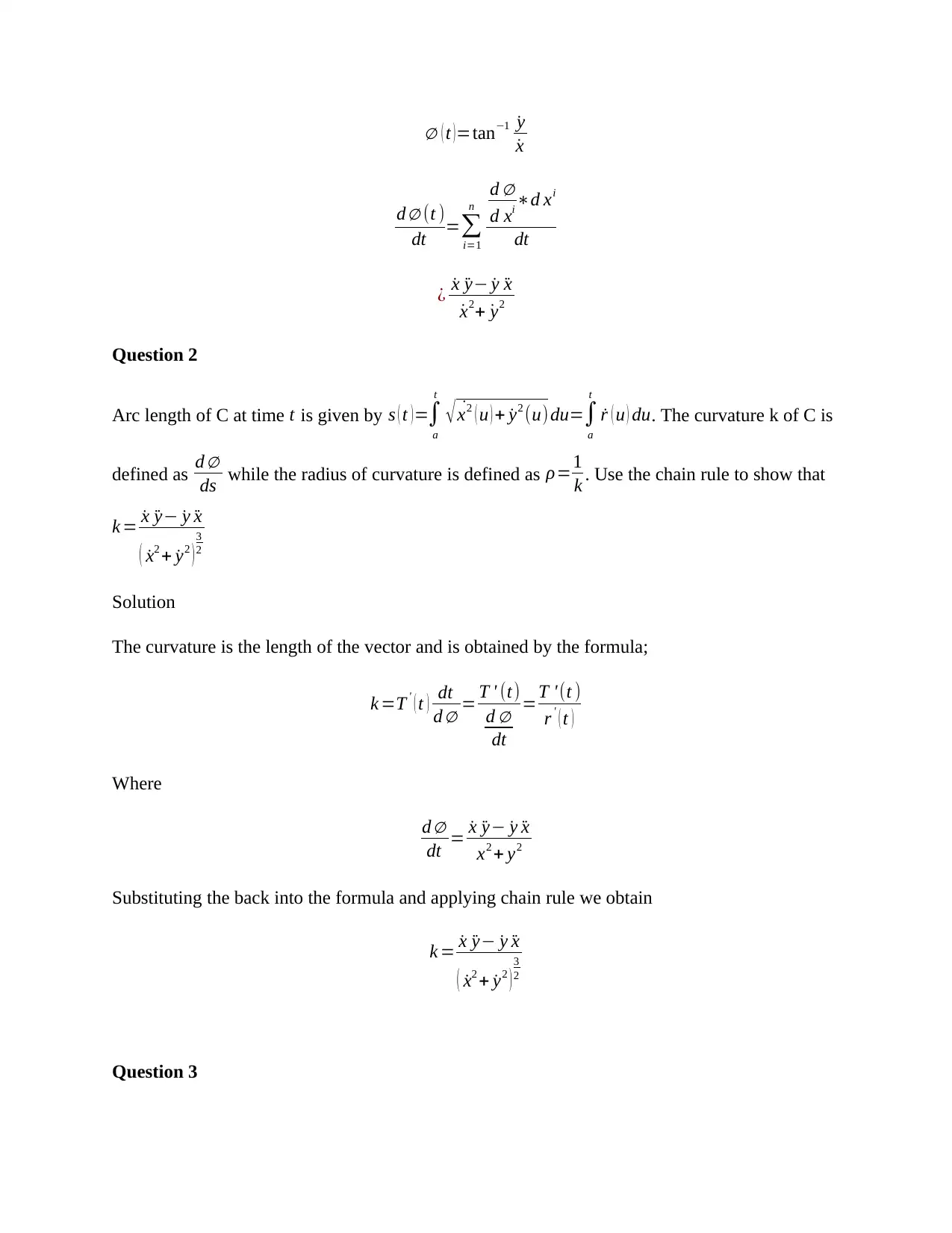
∅ ( t )=tan−1 ˙y
˙x
d ∅ (t )
dt =∑
i=1
n
d ∅
d xi ∗d xi
dt
¿ ˙x ¨y− ˙y ¨x
˙x2+ ˙y2
Question 2
Arc length of C at time t is given by s ( t ) =∫
a
t
√ ˙x2 ( u ) + ˙y2 (u)du=∫
a
t
˙r ( u ) du. The curvature k of C is
defined as d ∅
ds while the radius of curvature is defined as ρ=1
k . Use the chain rule to show that
k = ˙x ¨y− ˙y ¨x
( ˙x2 + ˙y2 )
3
2
Solution
The curvature is the length of the vector and is obtained by the formula;
k =T ' ( t ) dt
d ∅ = T ' (t)
d ∅
dt
=T '(t )
r ' ( t )
Where
d ∅
dt = ˙x ¨y− ˙y ¨x
x2 + y2
Substituting the back into the formula and applying chain rule we obtain
k = ˙x ¨y− ˙y ¨x
( ˙x2 + ˙y2 )
3
2
Question 3

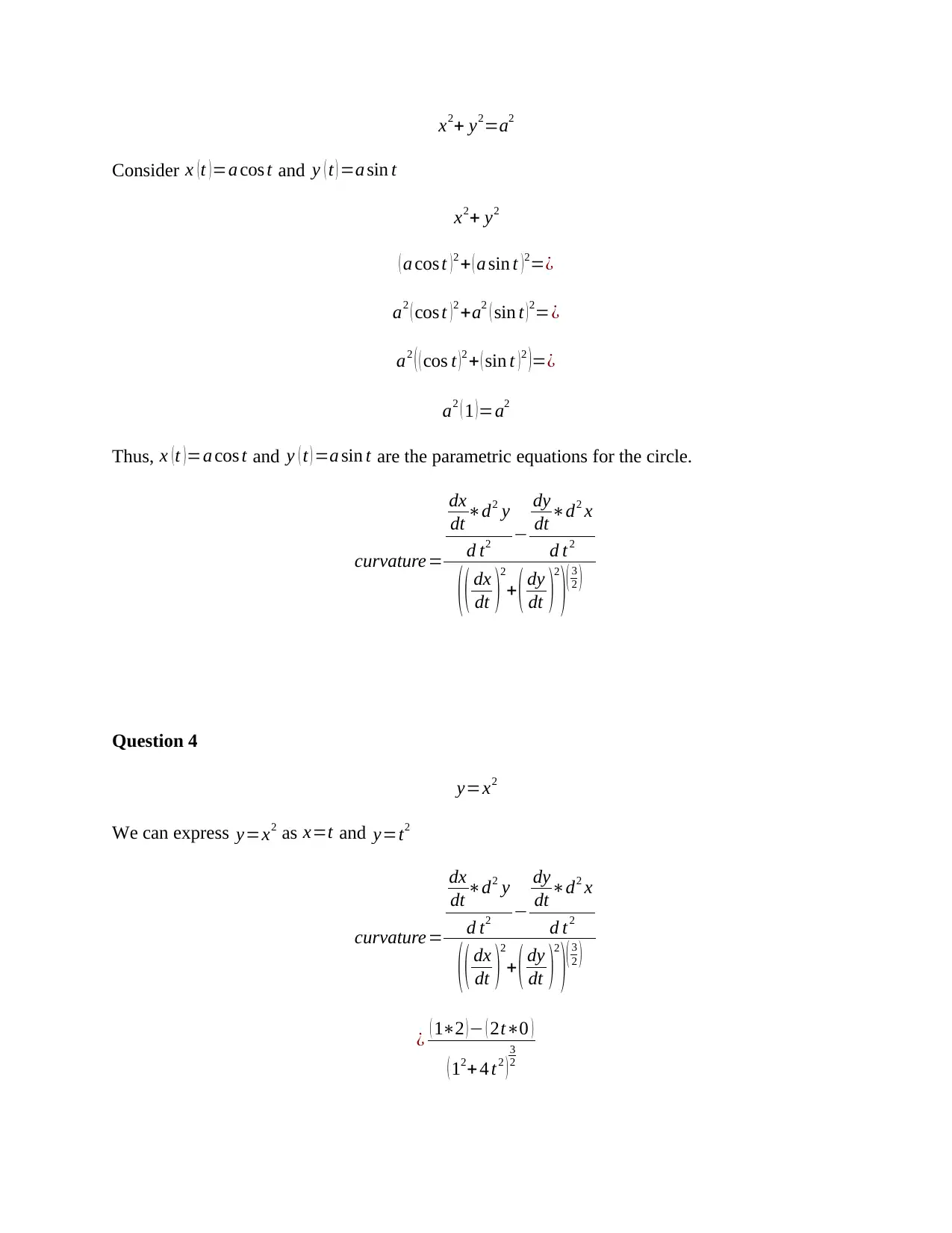
x2+ y2=a2
Consider x ( t ) =a cos t and y ( t ) =a sin t
x2+ y2
( a cos t ) 2 + ( a sin t ) 2=¿
a2 ( cos t )2 +a2 ( sin t )2=¿
a2 ( ( cos t )2 + ( sin t )2 )=¿
a2 ( 1 )=a2
Thus, x (t )=a cos t and y ( t ) =a sin t are the parametric equations for the circle.
curvature=
dx
dt ∗d2 y
d t2 −
dy
dt ∗d2 x
d t2
( ( dx
dt )2
+ ( dy
dt )2
)( 3
2 )
Question 4
y=x2
We can express y=x2 as x=t and y=t2
curvature=
dx
dt ∗d2 y
d t2 −
dy
dt ∗d2 x
d t2
( ( dx
dt )2
+ ( dy
dt )2
)( 3
2 )
¿ ( 1∗2 ) − ( 2t∗0 )
( 12+ 4 t2 )
3
2

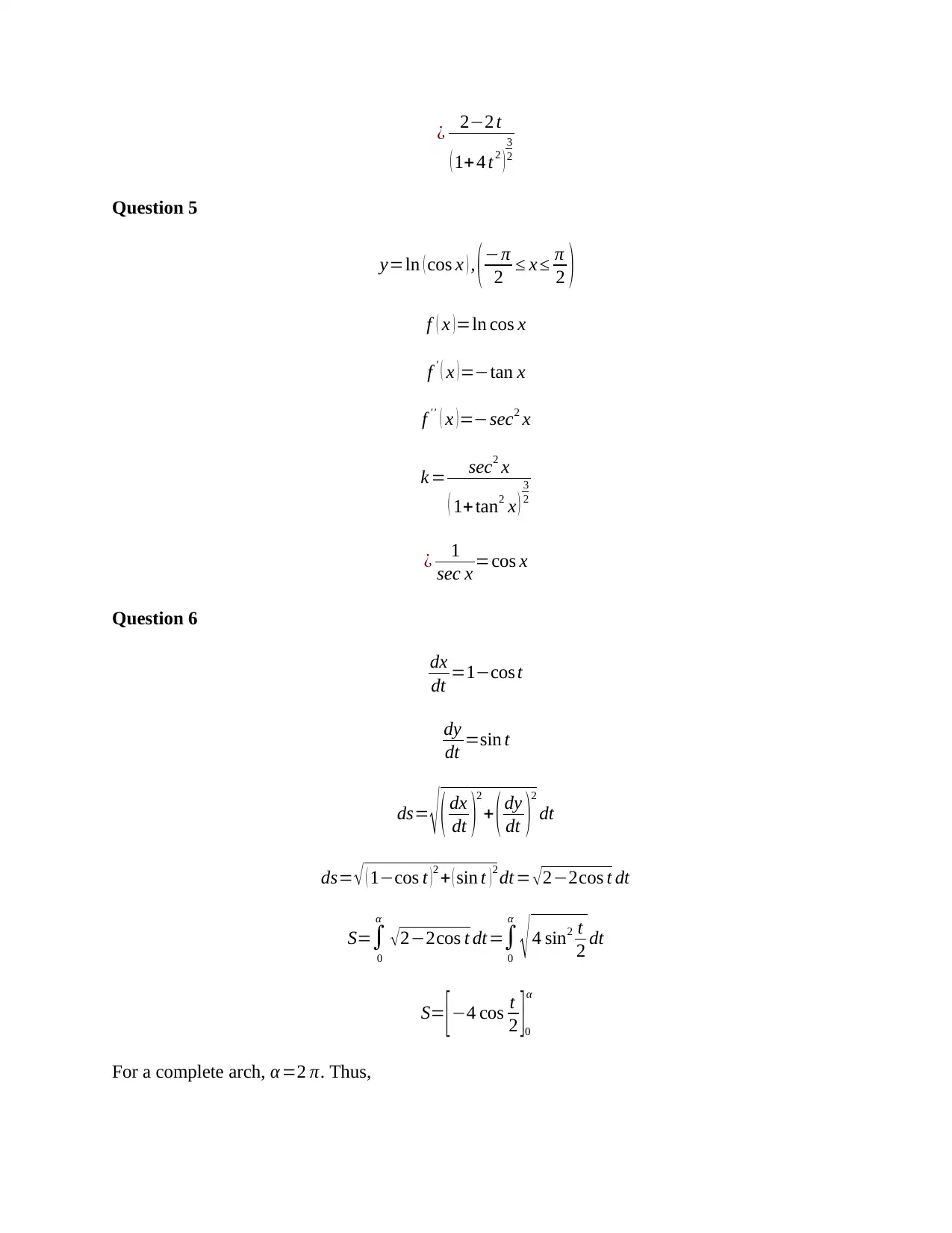
¿ 2−2 t
( 1+ 4 t2 )
3
2
Question 5
y=ln ( cos x ) , (−π
2 ≤ x ≤ π
2 )
f ( x )=ln cos x
f ' ( x )=−tan x
f ' ' ( x ) =−sec2 x
k = sec2 x
( 1+ tan2 x )
3
2
¿ 1
sec x =cos x
Question 6
dx
dt =1−cos t
dy
dt =sin t
ds= √ ( dx
dt )2
+ ( dy
dt )2
dt
ds= √ ( 1−cos t ) 2 + ( sin t ) 2 dt = √ 2−2cos t dt
S=∫
0
α
√2−2cos t dt=∫
0
α
√ 4 sin2 t
2 dt
S= [−4 cos t
2 ]0
α
For a complete arch, α =2 π. Thus,

S= [−4 cos t
2 ]0
2 π
=8
Question 7
Find the radius of curvature ρ for a point on the cycloid corresponding to a given time t=α .
The coordinates of the centre are given by x (t )=at and y ( t ) =a
Thus the radius a= √ ( at−a ( t−sin t ) )
2
+ ( a−a ( 1−cos t ) ) 2
Question 8
Find the area under one arch of the cycloid.
A=∫
0
2 πa
a ( 1−cos t ) dx
dt dt
¿∫
0
2 π
a ( 1−cos t ) [ a ( 1−cos t ) ] dt
¿ a2
∫
0
2 π
( 1−cos t )2 dt
¿ a2
[t +2 sint + t
2 + sin 2t
4 ]0
2 π
¿ 3 π a2
Question 9
Arc length
L=∫
0
t
√ [ C' ( t ) ] 2
+ [ S' ( t ) ] 2
dt
C ( t ) =∫
0
t
cos u2 du
C' ( t )=cos t2

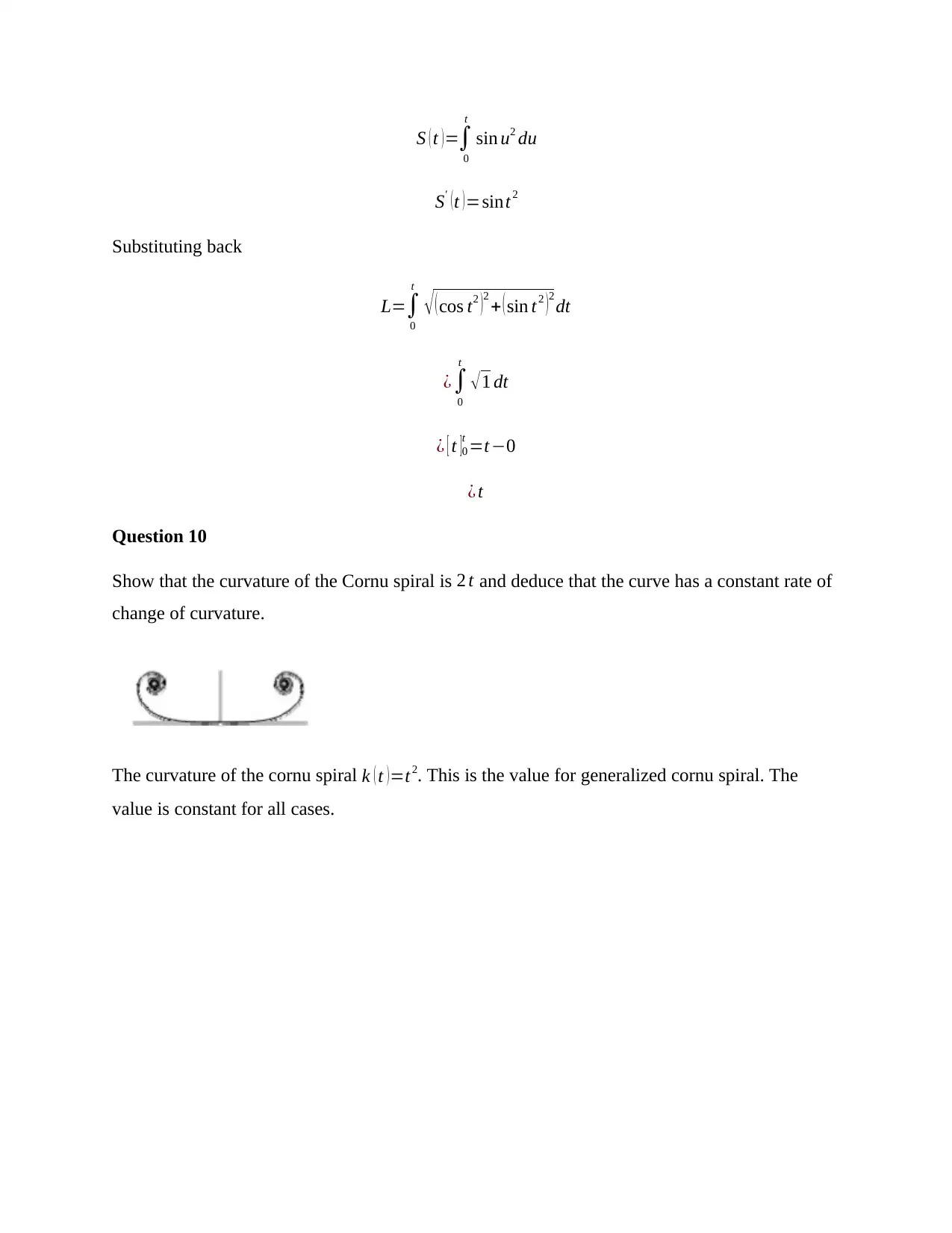
S ( t ) =∫
0
t
sin u2 du
S' (t )=sint2
Substituting back
L=∫
0
t
√ (cos t2 )2
+ ( sin t2 )2
dt
¿∫
0
t
√ 1 dt
¿ [ t ]0
t =t −0
¿ t
Question 10
Show that the curvature of the Cornu spiral is 2 t and deduce that the curve has a constant rate of
change of curvature.
The curvature of the cornu spiral k ( t )=t2. This is the value for generalized cornu spiral. The
value is constant for all cases.