Maths Assignment: Evaluating Price Changes on Revenue and Profit
VerifiedAdded on 2023/06/13
|8
|1385
|481
Homework Assignment
AI Summary
This maths assignment delves into the impact of changing prices on revenue and profit for a motorcycle manufacturing company, examining three different motorcycle types: Chrome Catalyst, Platinum Power, and Silver Streak. It defines total cost and total revenue functions for each type, calculates break-even points, and analyzes profitability based on production volume. The assignment also explores the impact of a price discount on revenue, using demand and supply equations to determine equilibrium points and revenue changes with and without the discount. Ultimately, it assesses whether implementing the discount would be beneficial for the airline, based on revenue generation.

Maths assignment
Name of the student
Name of the university
Author note
Name of the student
Name of the university
Author note
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Table of Contents
Answer to question 1: Impact of changing price upon revenue and profit................................3
Part 1......................................................................................................................................3
Part 2......................................................................................................................................4
Part 3......................................................................................................................................5
Part 4:.....................................................................................................................................5
Answer to question 2: Impact of price discount on revenue......................................................6
Bibliography...............................................................................................................................8
Answer to question 1: Impact of changing price upon revenue and profit................................3
Part 1......................................................................................................................................3
Part 2......................................................................................................................................4
Part 3......................................................................................................................................5
Part 4:.....................................................................................................................................5
Answer to question 2: Impact of price discount on revenue......................................................6
Bibliography...............................................................................................................................8
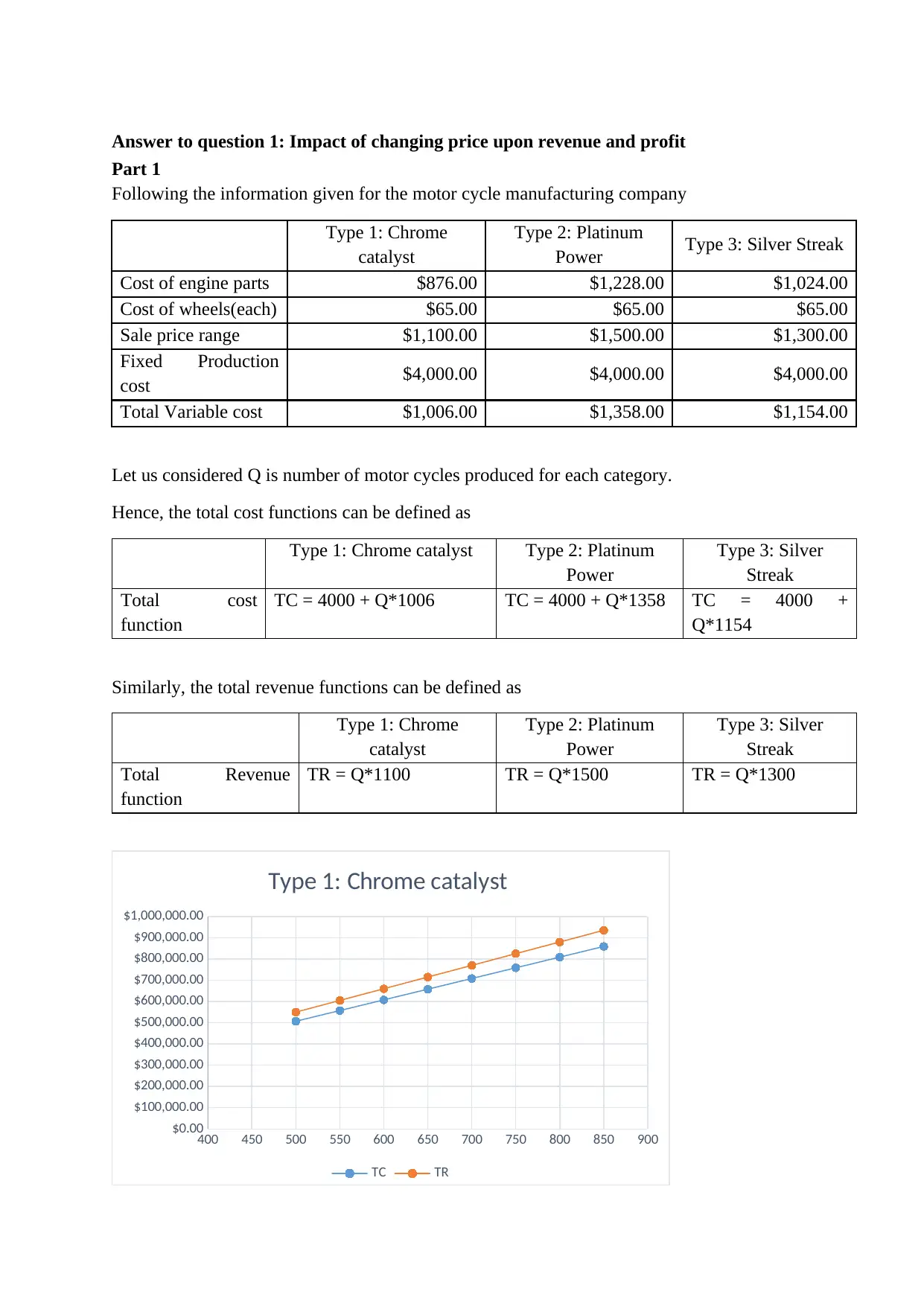
Answer to question 1: Impact of changing price upon revenue and profit
Part 1
Following the information given for the motor cycle manufacturing company
Type 1: Chrome
catalyst
Type 2: Platinum
Power Type 3: Silver Streak
Cost of engine parts $876.00 $1,228.00 $1,024.00
Cost of wheels(each) $65.00 $65.00 $65.00
Sale price range $1,100.00 $1,500.00 $1,300.00
Fixed Production
cost $4,000.00 $4,000.00 $4,000.00
Total Variable cost $1,006.00 $1,358.00 $1,154.00
Let us considered Q is number of motor cycles produced for each category.
Hence, the total cost functions can be defined as
Type 1: Chrome catalyst Type 2: Platinum
Power
Type 3: Silver
Streak
Total cost
function
TC = 4000 + Q*1006 TC = 4000 + Q*1358 TC = 4000 +
Q*1154
Similarly, the total revenue functions can be defined as
Type 1: Chrome
catalyst
Type 2: Platinum
Power
Type 3: Silver
Streak
Total Revenue
function
TR = Q*1100 TR = Q*1500 TR = Q*1300
400 450 500 550 600 650 700 750 800 850 900
$0.00
$100,000.00
$200,000.00
$300,000.00
$400,000.00
$500,000.00
$600,000.00
$700,000.00
$800,000.00
$900,000.00
$1,000,000.00
Type 1: Chrome catalyst
TC TR
Part 1
Following the information given for the motor cycle manufacturing company
Type 1: Chrome
catalyst
Type 2: Platinum
Power Type 3: Silver Streak
Cost of engine parts $876.00 $1,228.00 $1,024.00
Cost of wheels(each) $65.00 $65.00 $65.00
Sale price range $1,100.00 $1,500.00 $1,300.00
Fixed Production
cost $4,000.00 $4,000.00 $4,000.00
Total Variable cost $1,006.00 $1,358.00 $1,154.00
Let us considered Q is number of motor cycles produced for each category.
Hence, the total cost functions can be defined as
Type 1: Chrome catalyst Type 2: Platinum
Power
Type 3: Silver
Streak
Total cost
function
TC = 4000 + Q*1006 TC = 4000 + Q*1358 TC = 4000 +
Q*1154
Similarly, the total revenue functions can be defined as
Type 1: Chrome
catalyst
Type 2: Platinum
Power
Type 3: Silver
Streak
Total Revenue
function
TR = Q*1100 TR = Q*1500 TR = Q*1300
400 450 500 550 600 650 700 750 800 850 900
$0.00
$100,000.00
$200,000.00
$300,000.00
$400,000.00
$500,000.00
$600,000.00
$700,000.00
$800,000.00
$900,000.00
$1,000,000.00
Type 1: Chrome catalyst
TC TR
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
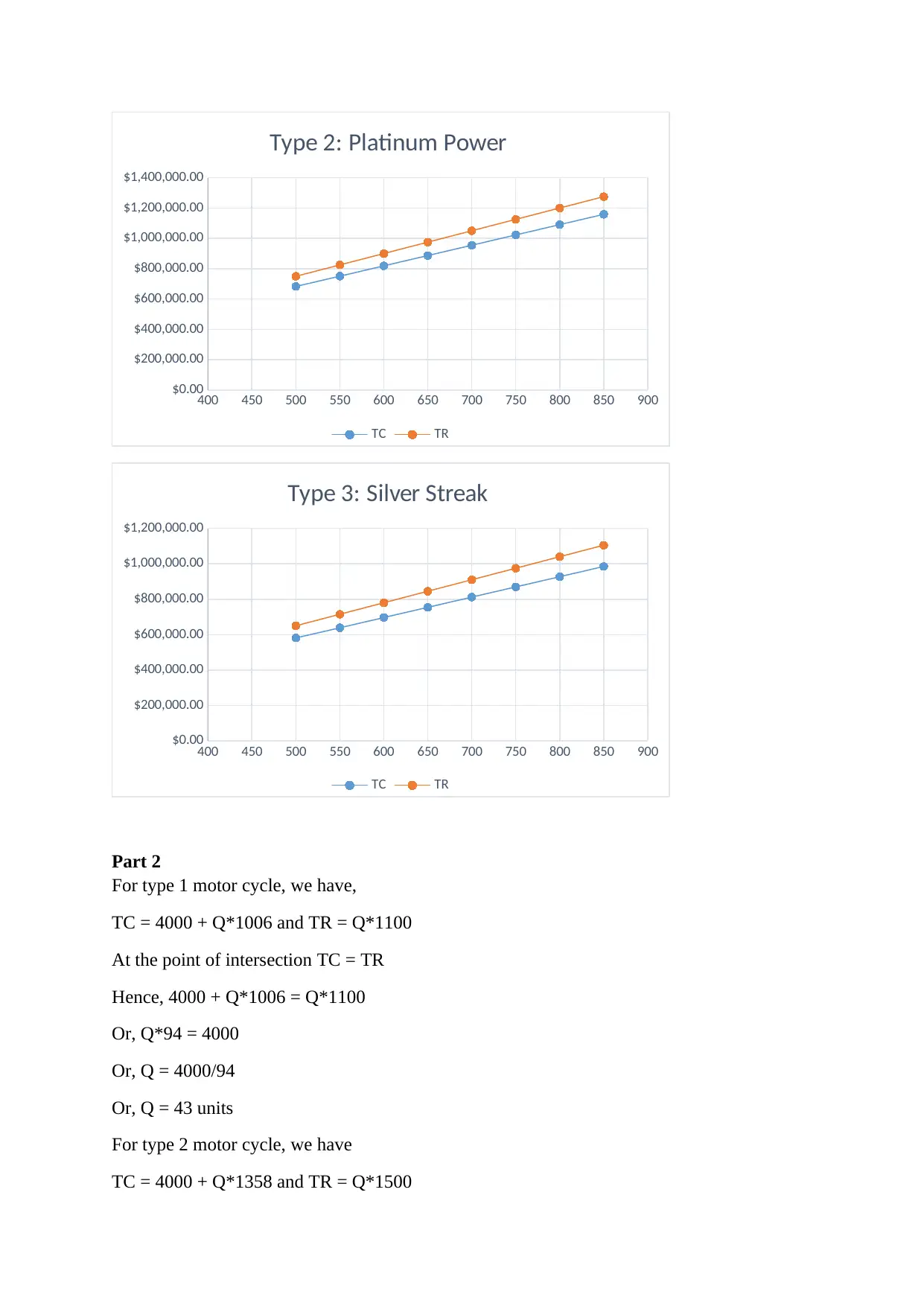
400 450 500 550 600 650 700 750 800 850 900
$0.00
$200,000.00
$400,000.00
$600,000.00
$800,000.00
$1,000,000.00
$1,200,000.00
$1,400,000.00
Type 2: Platinum Power
TC TR
400 450 500 550 600 650 700 750 800 850 900
$0.00
$200,000.00
$400,000.00
$600,000.00
$800,000.00
$1,000,000.00
$1,200,000.00
Type 3: Silver Streak
TC TR
Part 2
For type 1 motor cycle, we have,
TC = 4000 + Q*1006 and TR = Q*1100
At the point of intersection TC = TR
Hence, 4000 + Q*1006 = Q*1100
Or, Q*94 = 4000
Or, Q = 4000/94
Or, Q = 43 units
For type 2 motor cycle, we have
TC = 4000 + Q*1358 and TR = Q*1500
$0.00
$200,000.00
$400,000.00
$600,000.00
$800,000.00
$1,000,000.00
$1,200,000.00
$1,400,000.00
Type 2: Platinum Power
TC TR
400 450 500 550 600 650 700 750 800 850 900
$0.00
$200,000.00
$400,000.00
$600,000.00
$800,000.00
$1,000,000.00
$1,200,000.00
Type 3: Silver Streak
TC TR
Part 2
For type 1 motor cycle, we have,
TC = 4000 + Q*1006 and TR = Q*1100
At the point of intersection TC = TR
Hence, 4000 + Q*1006 = Q*1100
Or, Q*94 = 4000
Or, Q = 4000/94
Or, Q = 43 units
For type 2 motor cycle, we have
TC = 4000 + Q*1358 and TR = Q*1500
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
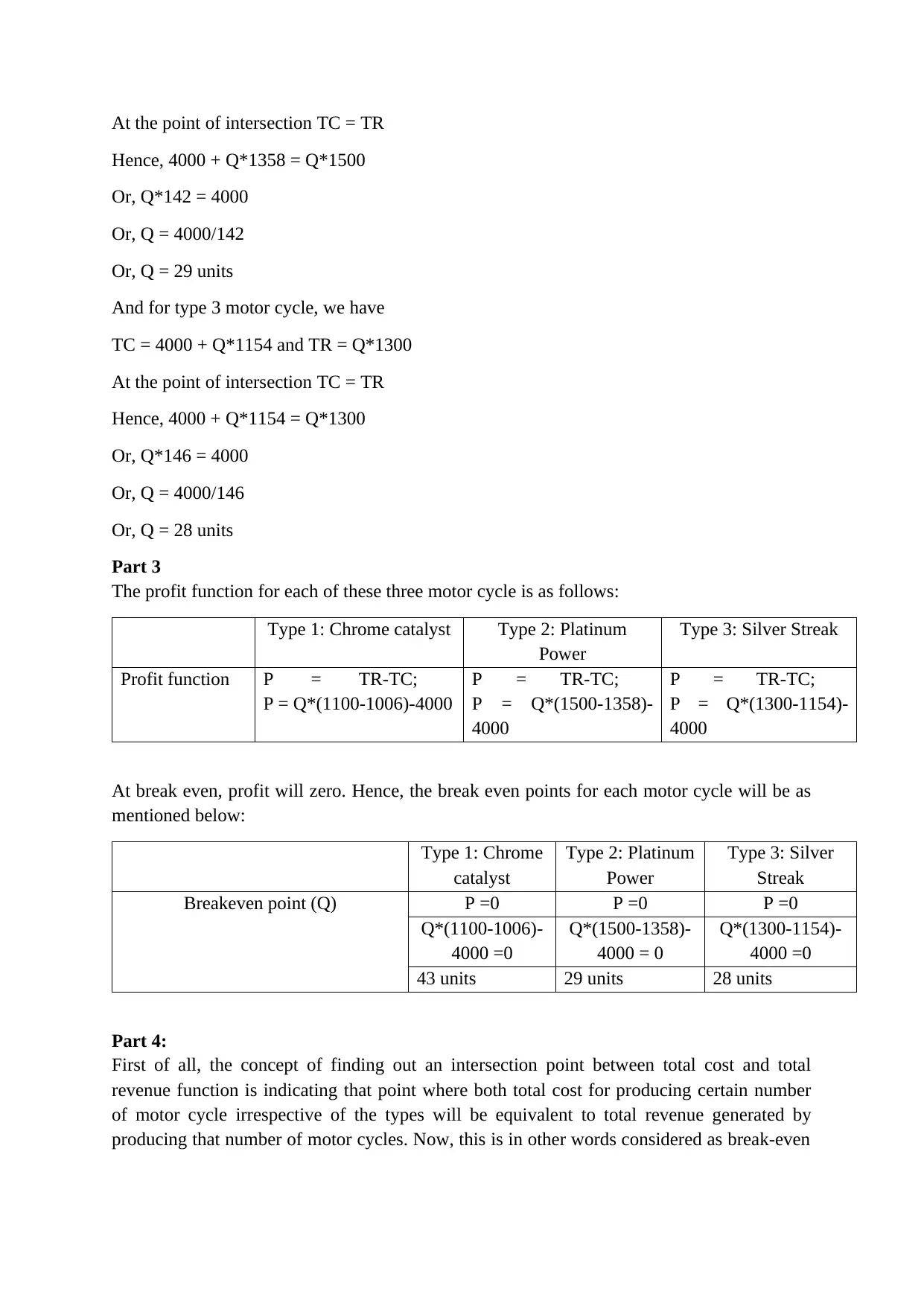
At the point of intersection TC = TR
Hence, 4000 + Q*1358 = Q*1500
Or, Q*142 = 4000
Or, Q = 4000/142
Or, Q = 29 units
And for type 3 motor cycle, we have
TC = 4000 + Q*1154 and TR = Q*1300
At the point of intersection TC = TR
Hence, 4000 + Q*1154 = Q*1300
Or, Q*146 = 4000
Or, Q = 4000/146
Or, Q = 28 units
Part 3
The profit function for each of these three motor cycle is as follows:
Type 1: Chrome catalyst Type 2: Platinum
Power
Type 3: Silver Streak
Profit function P = TR-TC;
P = Q*(1100-1006)-4000
P = TR-TC;
P = Q*(1500-1358)-
4000
P = TR-TC;
P = Q*(1300-1154)-
4000
At break even, profit will zero. Hence, the break even points for each motor cycle will be as
mentioned below:
Type 1: Chrome
catalyst
Type 2: Platinum
Power
Type 3: Silver
Streak
Breakeven point (Q) P =0 P =0 P =0
Q*(1100-1006)-
4000 =0
Q*(1500-1358)-
4000 = 0
Q*(1300-1154)-
4000 =0
43 units 29 units 28 units
Part 4:
First of all, the concept of finding out an intersection point between total cost and total
revenue function is indicating that point where both total cost for producing certain number
of motor cycle irrespective of the types will be equivalent to total revenue generated by
producing that number of motor cycles. Now, this is in other words considered as break-even
Hence, 4000 + Q*1358 = Q*1500
Or, Q*142 = 4000
Or, Q = 4000/142
Or, Q = 29 units
And for type 3 motor cycle, we have
TC = 4000 + Q*1154 and TR = Q*1300
At the point of intersection TC = TR
Hence, 4000 + Q*1154 = Q*1300
Or, Q*146 = 4000
Or, Q = 4000/146
Or, Q = 28 units
Part 3
The profit function for each of these three motor cycle is as follows:
Type 1: Chrome catalyst Type 2: Platinum
Power
Type 3: Silver Streak
Profit function P = TR-TC;
P = Q*(1100-1006)-4000
P = TR-TC;
P = Q*(1500-1358)-
4000
P = TR-TC;
P = Q*(1300-1154)-
4000
At break even, profit will zero. Hence, the break even points for each motor cycle will be as
mentioned below:
Type 1: Chrome
catalyst
Type 2: Platinum
Power
Type 3: Silver
Streak
Breakeven point (Q) P =0 P =0 P =0
Q*(1100-1006)-
4000 =0
Q*(1500-1358)-
4000 = 0
Q*(1300-1154)-
4000 =0
43 units 29 units 28 units
Part 4:
First of all, the concept of finding out an intersection point between total cost and total
revenue function is indicating that point where both total cost for producing certain number
of motor cycle irrespective of the types will be equivalent to total revenue generated by
producing that number of motor cycles. Now, this is in other words considered as break-even

point as at this point, there will be no gain and no lose situation. This is the reason, both step
2 and 3 has shown same value.
In order to understand the profitability position, let us consider 500 units production of each
three category of motor cycle on annual basis. It has seen that by producing 500 units of type
1 motor cycle, the profitability position will be 8.48%. The same will be for type 2 of 9.81%
and 11.88% respectively. Hence, it can be concluded that type 3 production will be
comparatively beneficial.
In order to calculate these figures, an average value of sales price is taken into account.
Answer to question 2: Impact of price discount on revenue
Using rand() function in excel, we have the value of a is 0.3357 and the value of b is
0.215987
Considering this two values, the demand and supply function becomes
Demand equation: p = 175 -15*0.3357q = 175 – 5.0355q
Supply equation: p = 0.00025q^2 +5*0.215987q + 35 = 0.00025q^2 + 1.079935q + 35
Considering no price reduction, the equilibrium point will be that one, where demand =
supply
Hence, 175 – 5.0355q = 0.00025q^2 + 1.079935q + 35
Or, 0.00025q^2 + 6.115435q -140 = 0
Using the formula
We have q = 23 units (approx.) [ignored the negative one as units cannot be negative]
Hence, p = 175 – 5.0355*23 = $59.18
Now, if $25.00 is considered as price discount, then the demand equation will become,
P – 25 = 175 – 5.0355q
Or, p = 200 – 5.0355q
Considering the price reduction, the equilibrium point will be that one, where demand =
supply
Hence, 200 – 5.0355q = 0.00025q^2 + 1.079935q + 35
2 and 3 has shown same value.
In order to understand the profitability position, let us consider 500 units production of each
three category of motor cycle on annual basis. It has seen that by producing 500 units of type
1 motor cycle, the profitability position will be 8.48%. The same will be for type 2 of 9.81%
and 11.88% respectively. Hence, it can be concluded that type 3 production will be
comparatively beneficial.
In order to calculate these figures, an average value of sales price is taken into account.
Answer to question 2: Impact of price discount on revenue
Using rand() function in excel, we have the value of a is 0.3357 and the value of b is
0.215987
Considering this two values, the demand and supply function becomes
Demand equation: p = 175 -15*0.3357q = 175 – 5.0355q
Supply equation: p = 0.00025q^2 +5*0.215987q + 35 = 0.00025q^2 + 1.079935q + 35
Considering no price reduction, the equilibrium point will be that one, where demand =
supply
Hence, 175 – 5.0355q = 0.00025q^2 + 1.079935q + 35
Or, 0.00025q^2 + 6.115435q -140 = 0
Using the formula
We have q = 23 units (approx.) [ignored the negative one as units cannot be negative]
Hence, p = 175 – 5.0355*23 = $59.18
Now, if $25.00 is considered as price discount, then the demand equation will become,
P – 25 = 175 – 5.0355q
Or, p = 200 – 5.0355q
Considering the price reduction, the equilibrium point will be that one, where demand =
supply
Hence, 200 – 5.0355q = 0.00025q^2 + 1.079935q + 35
⊘ This is a preview!⊘
Do you want full access?
Subscribe today to unlock all pages.

Trusted by 1+ million students worldwide
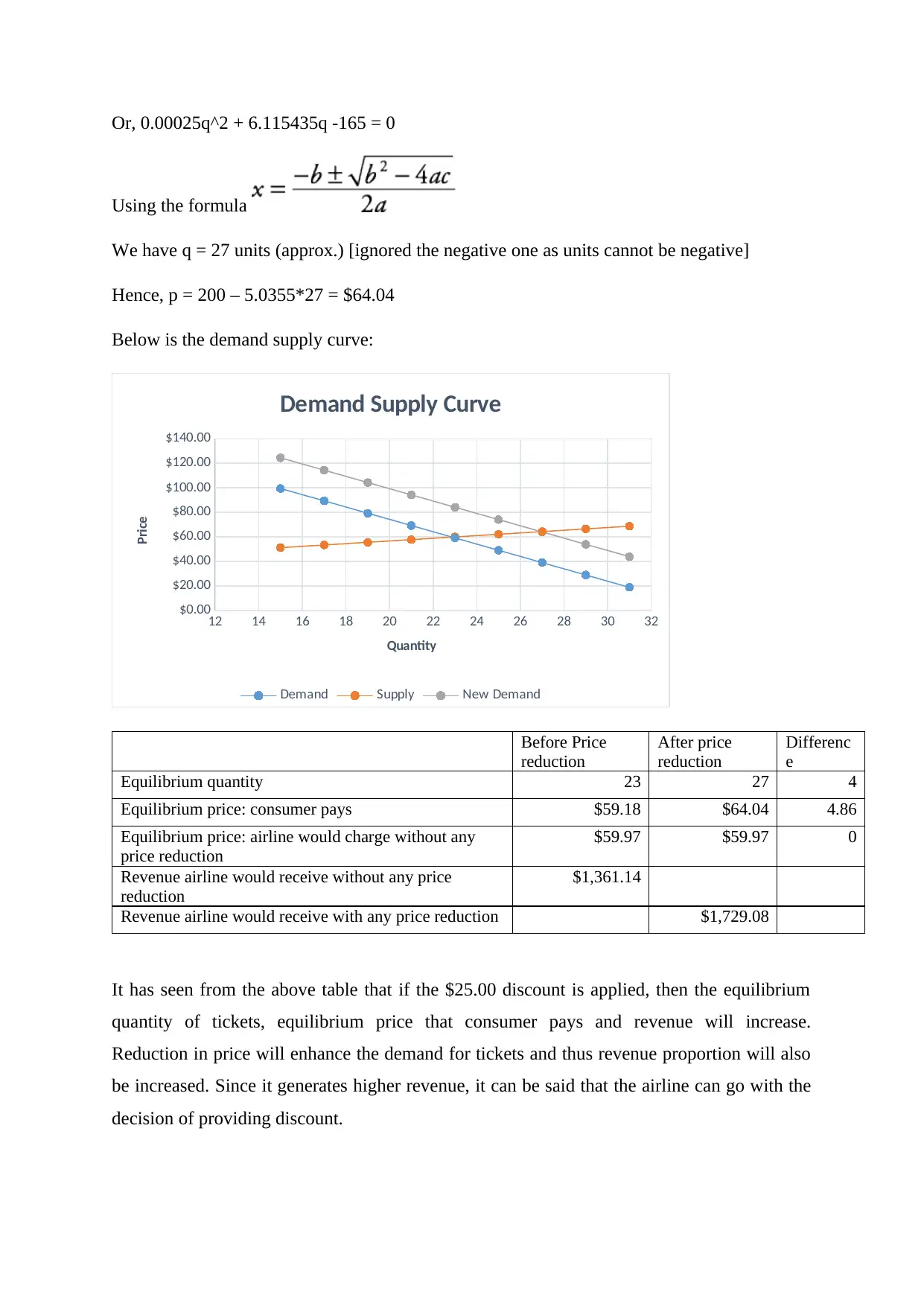
Or, 0.00025q^2 + 6.115435q -165 = 0
Using the formula
We have q = 27 units (approx.) [ignored the negative one as units cannot be negative]
Hence, p = 200 – 5.0355*27 = $64.04
Below is the demand supply curve:
12 14 16 18 20 22 24 26 28 30 32
$0.00
$20.00
$40.00
$60.00
$80.00
$100.00
$120.00
$140.00
Demand Supply Curve
Demand Supply New Demand
Quantity
Price
Before Price
reduction
After price
reduction
Differenc
e
Equilibrium quantity 23 27 4
Equilibrium price: consumer pays $59.18 $64.04 4.86
Equilibrium price: airline would charge without any
price reduction
$59.97 $59.97 0
Revenue airline would receive without any price
reduction
$1,361.14
Revenue airline would receive with any price reduction $1,729.08
It has seen from the above table that if the $25.00 discount is applied, then the equilibrium
quantity of tickets, equilibrium price that consumer pays and revenue will increase.
Reduction in price will enhance the demand for tickets and thus revenue proportion will also
be increased. Since it generates higher revenue, it can be said that the airline can go with the
decision of providing discount.
Using the formula
We have q = 27 units (approx.) [ignored the negative one as units cannot be negative]
Hence, p = 200 – 5.0355*27 = $64.04
Below is the demand supply curve:
12 14 16 18 20 22 24 26 28 30 32
$0.00
$20.00
$40.00
$60.00
$80.00
$100.00
$120.00
$140.00
Demand Supply Curve
Demand Supply New Demand
Quantity
Price
Before Price
reduction
After price
reduction
Differenc
e
Equilibrium quantity 23 27 4
Equilibrium price: consumer pays $59.18 $64.04 4.86
Equilibrium price: airline would charge without any
price reduction
$59.97 $59.97 0
Revenue airline would receive without any price
reduction
$1,361.14
Revenue airline would receive with any price reduction $1,729.08
It has seen from the above table that if the $25.00 discount is applied, then the equilibrium
quantity of tickets, equilibrium price that consumer pays and revenue will increase.
Reduction in price will enhance the demand for tickets and thus revenue proportion will also
be increased. Since it generates higher revenue, it can be said that the airline can go with the
decision of providing discount.
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Bibliography
Summers, L.H., 2014. US economic prospects: Secular stagnation, hysteresis, and the zero
lower bound. Business Economics, 49(2), pp.65-73.
Ward, D. and Begg, D., 2016. Economics for business. McGraw-Hill.
Laubach, T. and Williams, J.C., 2016. Measuring the natural rate of interest redux. Business
Economics, 51(2), pp.57-67.
Summers, L.H., 2014. US economic prospects: Secular stagnation, hysteresis, and the zero
lower bound. Business Economics, 49(2), pp.65-73.
Ward, D. and Begg, D., 2016. Economics for business. McGraw-Hill.
Laubach, T. and Williams, J.C., 2016. Measuring the natural rate of interest redux. Business
Economics, 51(2), pp.57-67.
1 out of 8