Statistical Analysis for Various Scenarios
VerifiedAdded on 2023/04/22
|13
|2220
|354
AI Summary
Get statistical analysis for various scenarios including mean, standard deviation, confidence interval, risk ratio, odds ratio, and more at Desklib. This document covers scenarios related to cholesterol level, systolic blood pressure, hypertension, pain relief, age, and AF event. Access a wide range of study material with solved assignments, essays, dissertations, and more.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
HOMEWORK # 2
1
1
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

1. ANS:
Selected sample for cholesterol level in the nine participants was as follows.
185 225 240 196 175 180 194 147 223
The average or mean total cholesterol level is calculated as,
x
¿
=∑ xi
n =185+225+240+196+175+180+ 194+147+223
9 =1765
9 =196 .11
The standard deviation is calculated as,
s= √ ∑ ( xi−x
¿
)
2
n−1 = √ ( 185−196 . 11 ) 2+ ( 225−196 .11 ) 2 +.. .+ ( 147−196. 11 ) 2+ ( 223−196 .11 )2
8
¿ √ 6728. 88
8 =29
The 95% confidence interval for the mean total cholesterol levels in children is calculated as
x
¿
±t0. 025 , 8∗ s
√ n =196 .11±2. 306∗29
√ 9 =196 . 11±22 . 29=[173 . 82, 218 . 40 ]
.
Hence with 95% confidence we can say that the average total cholesterol level in children 2 to 6
years of age will be roughly somewhere between 174 and 219.
2
Selected sample for cholesterol level in the nine participants was as follows.
185 225 240 196 175 180 194 147 223
The average or mean total cholesterol level is calculated as,
x
¿
=∑ xi
n =185+225+240+196+175+180+ 194+147+223
9 =1765
9 =196 .11
The standard deviation is calculated as,
s= √ ∑ ( xi−x
¿
)
2
n−1 = √ ( 185−196 . 11 ) 2+ ( 225−196 .11 ) 2 +.. .+ ( 147−196. 11 ) 2+ ( 223−196 .11 )2
8
¿ √ 6728. 88
8 =29
The 95% confidence interval for the mean total cholesterol levels in children is calculated as
x
¿
±t0. 025 , 8∗ s
√ n =196 .11±2. 306∗29
√ 9 =196 . 11±22 . 29=[173 . 82, 218 . 40 ]
.
Hence with 95% confidence we can say that the average total cholesterol level in children 2 to 6
years of age will be roughly somewhere between 174 and 219.
2

3. ANS:
A. Placebo patients are considered as group1 ( n1=100 ), and experimental patients are
considered as group2 ( n2=100 ).
The unbiased estimator for difference in systolic blood pressure between Experimental and
Placebo groups is estimated as x
¿
=x
¿
1−x
¿
2=131. 4−120. 2=11 .2 .
The standard deviations of the two populations are not known, so we take sample standard
deviation to estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
=18 .92
100 +15 . 42
100 =3. 5721+2. 3716=5 .9437
and the standard deviation is
calculated as s= √ 5 . 9437=2 . 438 .
Variances of the populations are not known, so we consider that the difference between the two
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom (Le, & Eberly,
2016).
3
A. Placebo patients are considered as group1 ( n1=100 ), and experimental patients are
considered as group2 ( n2=100 ).
The unbiased estimator for difference in systolic blood pressure between Experimental and
Placebo groups is estimated as x
¿
=x
¿
1−x
¿
2=131. 4−120. 2=11 .2 .
The standard deviations of the two populations are not known, so we take sample standard
deviation to estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
=18 .92
100 +15 . 42
100 =3. 5721+2. 3716=5 .9437
and the standard deviation is
calculated as s= √ 5 . 9437=2 . 438 .
Variances of the populations are not known, so we consider that the difference between the two
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom (Le, & Eberly,
2016).
3

The 95% confidence interval is evaluated as
(( x
¿
1−x
¿
2)±t1−α / 2∗
√ s1
2
n1
+ s2
2
n2 )=11 .2±1. 972∗2. 438=[ 6 .39 , 16 ].
With 95% confidence it can be stated that values of difference in mean systolic pressures
between experimental and placebo groups will be somewhere between 6.39 and 16.
B. Applying the central limit theorem (CLT) on the difference between the sample proportions,
it can be inferred that the distribution of difference between two samples will follow a normal
distribution.
The 95% confidence interval is calculated as ¿ ¿ .
According to the problem, p
^¿1=0. 22 ( placebo)
¿ and p
^¿2=0 .14 (exp erimental )
¿ .
Hence, the 95% confidence interval is calculated as
CI = ( 0 .22−0 .14 ) ±1 .96∗ √ 0 . 22∗0 . 78
100 +0 . 14∗0 .86
100 =0 . 08±1. 96∗√ 0. 001716+0. 001204
=0 . 08±1. 96∗√ 0. 00292
=0 . 08±1. 96∗0 .054
=0 . 08±0. 10584
=[−0 . 026 , 0 . 186 ]
4
(( x
¿
1−x
¿
2)±t1−α / 2∗
√ s1
2
n1
+ s2
2
n2 )=11 .2±1. 972∗2. 438=[ 6 .39 , 16 ].
With 95% confidence it can be stated that values of difference in mean systolic pressures
between experimental and placebo groups will be somewhere between 6.39 and 16.
B. Applying the central limit theorem (CLT) on the difference between the sample proportions,
it can be inferred that the distribution of difference between two samples will follow a normal
distribution.
The 95% confidence interval is calculated as ¿ ¿ .
According to the problem, p
^¿1=0. 22 ( placebo)
¿ and p
^¿2=0 .14 (exp erimental )
¿ .
Hence, the 95% confidence interval is calculated as
CI = ( 0 .22−0 .14 ) ±1 .96∗ √ 0 . 22∗0 . 78
100 +0 . 14∗0 .86
100 =0 . 08±1. 96∗√ 0. 001716+0. 001204
=0 . 08±1. 96∗√ 0. 00292
=0 . 08±1. 96∗0 .054
=0 . 08±0. 10584
=[−0 . 026 , 0 . 186 ]
4
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
With 95% confidence it is possible to conclude that the percentage difference in hypertension
between the placebo effect group and the experimental group can be anywhere between (-
0.026%) and 0.186%.
C. Risk-Ratio (RR) for the side-effect is calculated as,
RR= Risk event in exp erimental group
Risk event placebo group = se /ne
s p/n p
Now, according to the following table the value of RR is calculated.
Experimental
(n = 100)
Placebo
(n = 100)
Number of patients without side
effects 94 ( f e ) 92 ( f p )
Number of patients with side
effects 6 ( se ) 8 ( sp )
Total 100 ( ne ) 100 ( n p )
Hence, point estimate for the risk ratio is
RR= 6/100
8/100 =0 . 75
The 95% confidence interval for the relative risk (RR) is computed using a two-step procedure as
outlined below.
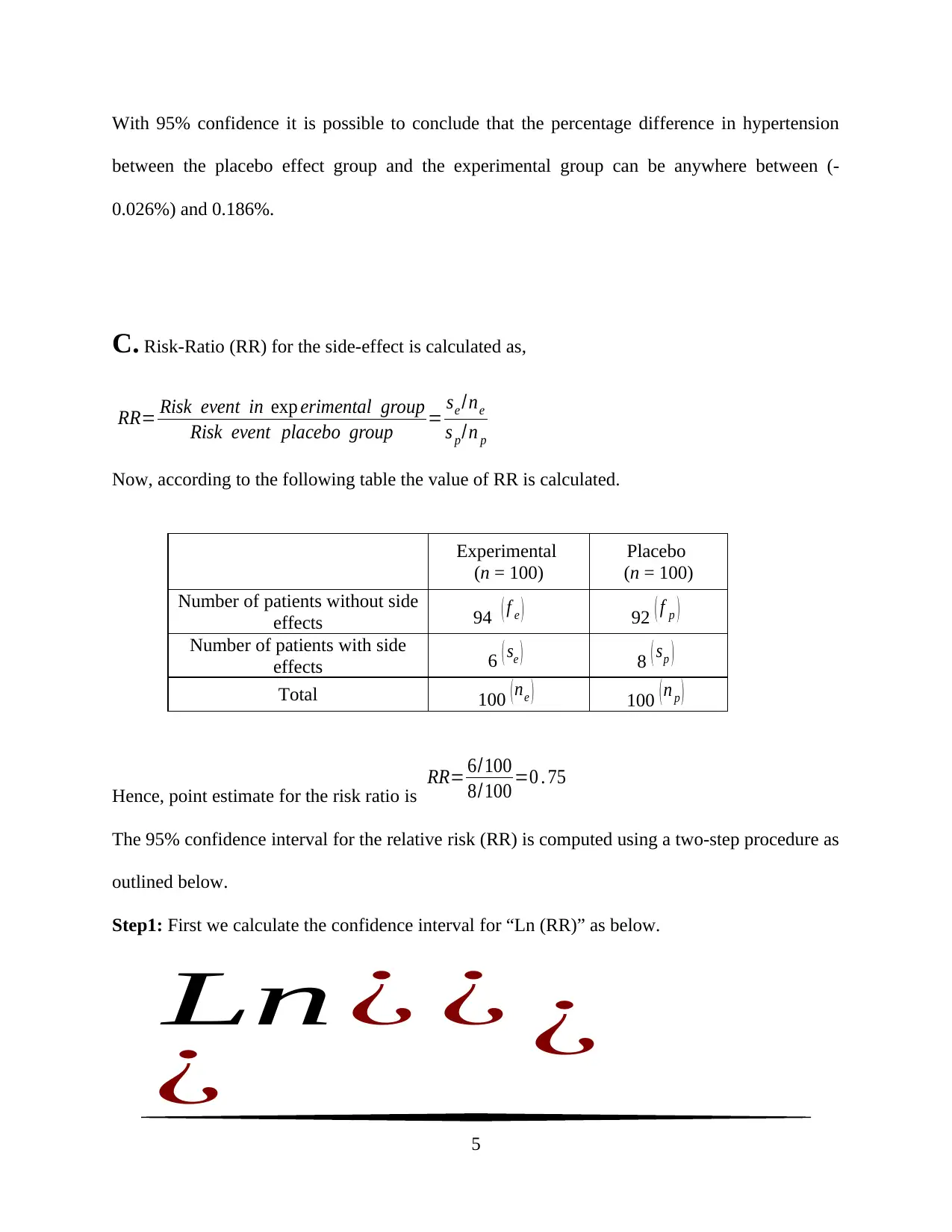
Step1: First we calculate the confidence interval for “Ln (RR)” as below.
Ln¿ ¿
¿ ¿
5
between the placebo effect group and the experimental group can be anywhere between (-
0.026%) and 0.186%.
C. Risk-Ratio (RR) for the side-effect is calculated as,
RR= Risk event in exp erimental group
Risk event placebo group = se /ne
s p/n p
Now, according to the following table the value of RR is calculated.
Experimental
(n = 100)
Placebo
(n = 100)
Number of patients without side
effects 94 ( f e ) 92 ( f p )
Number of patients with side
effects 6 ( se ) 8 ( sp )
Total 100 ( ne ) 100 ( n p )
Hence, point estimate for the risk ratio is
RR= 6/100
8/100 =0 . 75
The 95% confidence interval for the relative risk (RR) is computed using a two-step procedure as
outlined below.
Step1: First we calculate the confidence interval for “Ln (RR)” as below.
Ln¿ ¿
¿ ¿
5
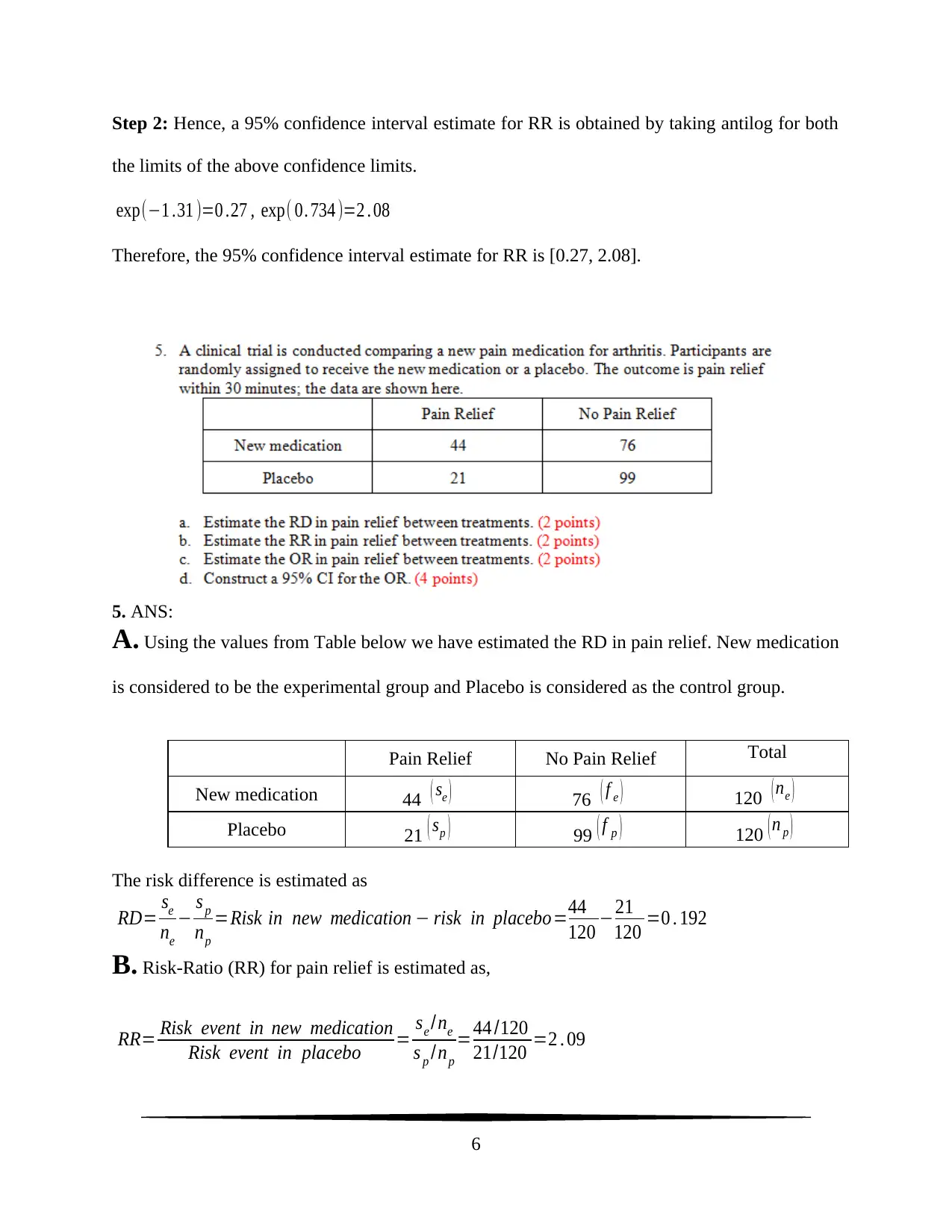
Step 2: Hence, a 95% confidence interval estimate for RR is obtained by taking antilog for both
the limits of the above confidence limits.
exp(−1 .31 )=0 .27 , exp( 0. 734 )=2 . 08
Therefore, the 95% confidence interval estimate for RR is [0.27, 2.08].
5. ANS:
A. Using the values from Table below we have estimated the RD in pain relief. New medication
is considered to be the experimental group and Placebo is considered as the control group.
Pain Relief No Pain Relief Total
New medication 44 ( se ) 76 ( f e ) 120 ( ne )
Placebo 21 ( sp ) 99 ( f p ) 120 ( n p )
The risk difference is estimated as
RD= se
ne
− s p
np
=Risk in new medication − risk in placebo=44
120 −21
120 =0 . 192
B. Risk-Ratio (RR) for pain relief is estimated as,
RR= Risk event in new medication
Risk event in placebo = se /ne
s p /np
=44 /120
21/120 =2 . 09
6
the limits of the above confidence limits.
exp(−1 .31 )=0 .27 , exp( 0. 734 )=2 . 08
Therefore, the 95% confidence interval estimate for RR is [0.27, 2.08].
5. ANS:
A. Using the values from Table below we have estimated the RD in pain relief. New medication
is considered to be the experimental group and Placebo is considered as the control group.
Pain Relief No Pain Relief Total
New medication 44 ( se ) 76 ( f e ) 120 ( ne )
Placebo 21 ( sp ) 99 ( f p ) 120 ( n p )
The risk difference is estimated as
RD= se
ne
− s p
np
=Risk in new medication − risk in placebo=44
120 −21
120 =0 . 192
B. Risk-Ratio (RR) for pain relief is estimated as,
RR= Risk event in new medication
Risk event in placebo = se /ne
s p /np
=44 /120
21/120 =2 . 09
6
C. Odds-Ratio (OR) for pain relief is estimated as,
OR= risk in new medication
risk in placebo = se f p
f e s p
=44∗99
76∗21 =2. 73
D. The 95% confidence interval for the Odds-Ratio (OR) for pain relief is computed using a
two-step procedure as outlined below.
Pain Relief No Pain Relief Total
New medication 44 ( se ) 76 ( f e ) 120 ( ne )
Placebo 21 ( sp ) 99 ( f p ) 120 ( n p )
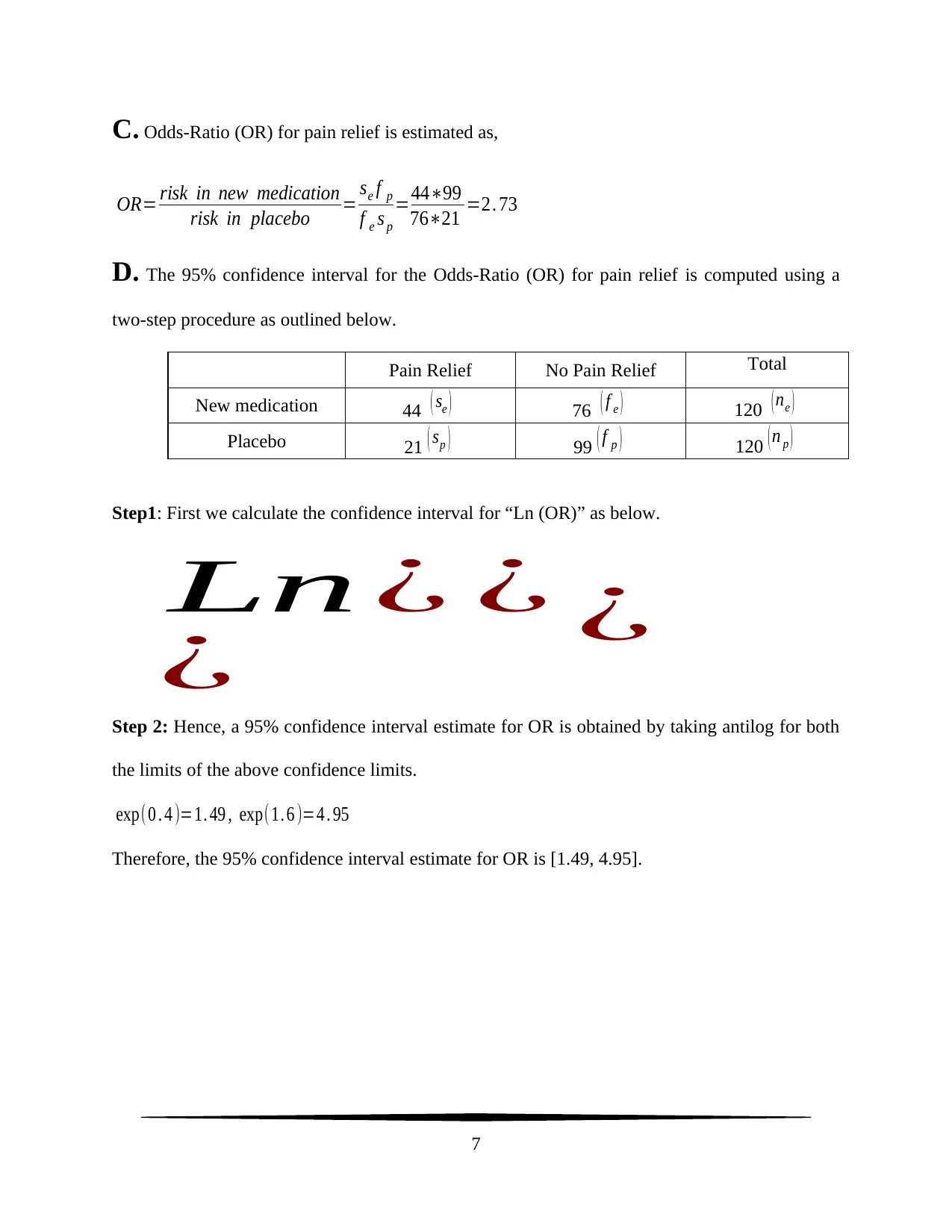
Step1: First we calculate the confidence interval for “Ln (OR)” as below.
Ln¿ ¿
¿ ¿
Step 2: Hence, a 95% confidence interval estimate for OR is obtained by taking antilog for both
the limits of the above confidence limits.
exp(0 . 4 )=1. 49 , exp(1. 6 )=4 . 95
Therefore, the 95% confidence interval estimate for OR is [1.49, 4.95].
7
OR= risk in new medication
risk in placebo = se f p
f e s p
=44∗99
76∗21 =2. 73
D. The 95% confidence interval for the Odds-Ratio (OR) for pain relief is computed using a
two-step procedure as outlined below.
Pain Relief No Pain Relief Total
New medication 44 ( se ) 76 ( f e ) 120 ( ne )
Placebo 21 ( sp ) 99 ( f p ) 120 ( n p )
Step1: First we calculate the confidence interval for “Ln (OR)” as below.
Ln¿ ¿
¿ ¿
Step 2: Hence, a 95% confidence interval estimate for OR is obtained by taking antilog for both
the limits of the above confidence limits.
exp(0 . 4 )=1. 49 , exp(1. 6 )=4 . 95
Therefore, the 95% confidence interval estimate for OR is [1.49, 4.95].
7
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
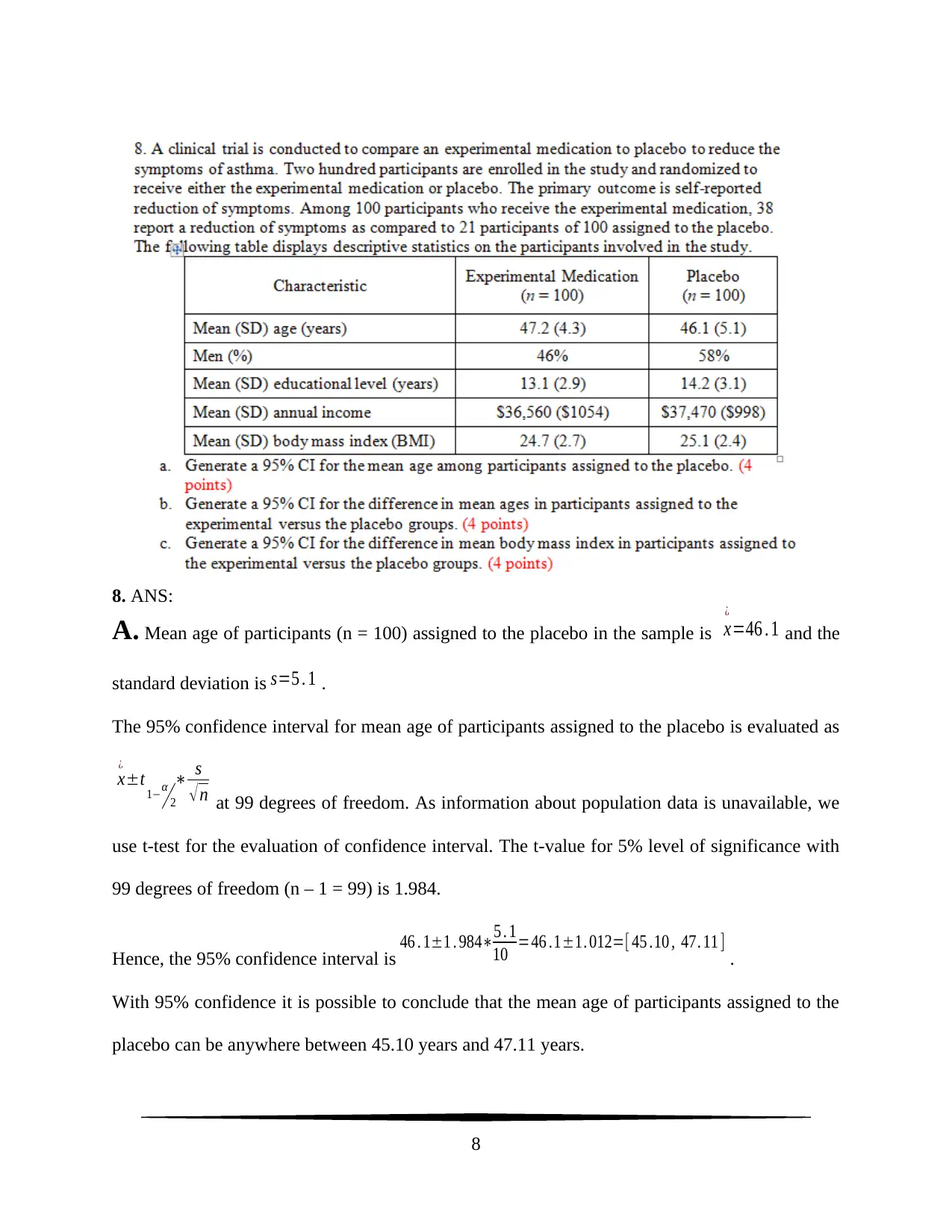
8. ANS:
A. Mean age of participants (n = 100) assigned to the placebo in the sample is x
¿
=46 . 1 and the
standard deviation is s=5 . 1 .
The 95% confidence interval for mean age of participants assigned to the placebo is evaluated as
x
¿
±t 1−α
2
∗ s
√ n at 99 degrees of freedom. As information about population data is unavailable, we
use t-test for the evaluation of confidence interval. The t-value for 5% level of significance with
99 degrees of freedom (n – 1 = 99) is 1.984.
Hence, the 95% confidence interval is 46 . 1±1 . 984∗5 . 1
10 =46 .1±1. 012=[ 45 .10 , 47. 11 ] .
With 95% confidence it is possible to conclude that the mean age of participants assigned to the
placebo can be anywhere between 45.10 years and 47.11 years.
8
A. Mean age of participants (n = 100) assigned to the placebo in the sample is x
¿
=46 . 1 and the
standard deviation is s=5 . 1 .
The 95% confidence interval for mean age of participants assigned to the placebo is evaluated as
x
¿
±t 1−α
2
∗ s
√ n at 99 degrees of freedom. As information about population data is unavailable, we
use t-test for the evaluation of confidence interval. The t-value for 5% level of significance with
99 degrees of freedom (n – 1 = 99) is 1.984.
Hence, the 95% confidence interval is 46 . 1±1 . 984∗5 . 1
10 =46 .1±1. 012=[ 45 .10 , 47. 11 ] .
With 95% confidence it is possible to conclude that the mean age of participants assigned to the
placebo can be anywhere between 45.10 years and 47.11 years.
8

B. Placebo patients are considered as group2 ( n1=100 ), and experimental medication patients
are considered as group1 ( n2=100 ). We know that the unbiased estimator for difference in
mean ages between Experimental and Placebo groups is estimated as
x
¿
=x
¿
1−x
¿
2=47 . 2−46 .1=1. 1 . The standard deviations of the two populations are not known, so
we take sample’s standard deviation to estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
= 4 . 32
100 + 5 . 12
100 =0 . 1849+0 .2601=0 . 445
and the standard deviation is calculated as
s= √ 0 . 445=0 .67 .
Variances of the populations are not known, so we consider that the difference between the two
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom. The 95%
confidence interval for the difference in mean ages is evaluated as,
(( x
¿
1−x
¿
2)±t1−α / 2∗
√ s1
2
n1
+ s2
2
n2 )=1 .1±1. 972∗0. 67=[−0 .22 , 2. 42].
C.
We know that the unbiased estimator for difference in mean body mass index between
Experimental and Placebo groups is estimated as x
¿
=x
¿
1−x
¿
2=24 . 7−25. 1=−0. 4 . The standard
deviations of the two populations are not known, so we take sample standard deviation to
estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
=2 . 72
100 + 2. 42
100 =0 . 0729+0 . 0576=0. 1305
and the standard deviation is calculated
as s= √ 0 . 1305=0 . 36 .
9
are considered as group1 ( n2=100 ). We know that the unbiased estimator for difference in
mean ages between Experimental and Placebo groups is estimated as
x
¿
=x
¿
1−x
¿
2=47 . 2−46 .1=1. 1 . The standard deviations of the two populations are not known, so
we take sample’s standard deviation to estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
= 4 . 32
100 + 5 . 12
100 =0 . 1849+0 .2601=0 . 445
and the standard deviation is calculated as
s= √ 0 . 445=0 .67 .
Variances of the populations are not known, so we consider that the difference between the two
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom. The 95%
confidence interval for the difference in mean ages is evaluated as,
(( x
¿
1−x
¿
2)±t1−α / 2∗
√ s1
2
n1
+ s2
2
n2 )=1 .1±1. 972∗0. 67=[−0 .22 , 2. 42].
C.
We know that the unbiased estimator for difference in mean body mass index between
Experimental and Placebo groups is estimated as x
¿
=x
¿
1−x
¿
2=24 . 7−25. 1=−0. 4 . The standard
deviations of the two populations are not known, so we take sample standard deviation to
estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
=2 . 72
100 + 2. 42
100 =0 . 0729+0 . 0576=0. 1305
and the standard deviation is calculated
as s= √ 0 . 1305=0 . 36 .
9

Variances of the populations are not known, so we consider that the difference between the two
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom. The 95%
confidence interval for the difference in mean body mass index is evaluated as,
(( x
¿
1−x
¿
2)±t1−α / 2∗
√ s1
2
n1
+ s2
2
n2 )=−0 . 4±1 . 972∗0 . 36=[−1 .11 , 0 . 31].
With 95% confidence it is possible to conclude that the difference in
mean body mass index
between Experimental and Placebo groups can be anywhere between -1.11 and 0.31 (Pagano, &
Gauvreau, 2018).
10
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom. The 95%
confidence interval for the difference in mean body mass index is evaluated as,
(( x
¿
1−x
¿
2)±t1−α / 2∗
√ s1
2
n1
+ s2
2
n2 )=−0 . 4±1 . 972∗0 . 36=[−1 .11 , 0 . 31].
With 95% confidence it is possible to conclude that the difference in
mean body mass index
between Experimental and Placebo groups can be anywhere between -1.11 and 0.31 (Pagano, &
Gauvreau, 2018).
10
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
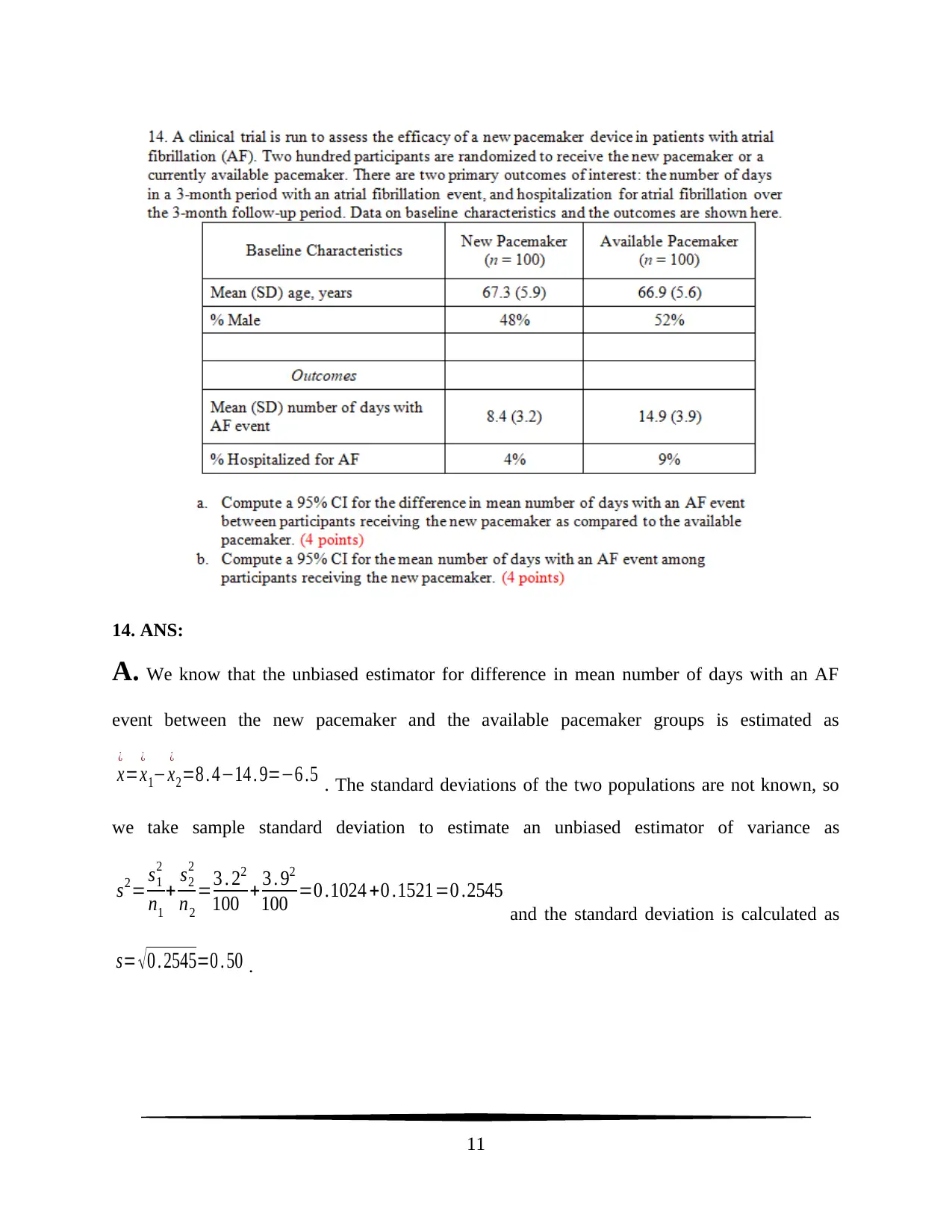
14. ANS:
A. We know that the unbiased estimator for difference in mean number of days with an AF
event between the new pacemaker and the available pacemaker groups is estimated as
x
¿
=x
¿
1−x
¿
2=8 . 4−14 . 9=−6 .5 . The standard deviations of the two populations are not known, so
we take sample standard deviation to estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
=3 . 22
100 + 3 . 92
100 =0 .1024 +0 .1521=0 .2545
and the standard deviation is calculated as
s= √ 0 . 2545=0 . 50 .
11
A. We know that the unbiased estimator for difference in mean number of days with an AF
event between the new pacemaker and the available pacemaker groups is estimated as
x
¿
=x
¿
1−x
¿
2=8 . 4−14 . 9=−6 .5 . The standard deviations of the two populations are not known, so
we take sample standard deviation to estimate an unbiased estimator of variance as
s2= s1
2
n1
+ s2
2
n2
=3 . 22
100 + 3 . 92
100 =0 .1024 +0 .1521=0 .2545
and the standard deviation is calculated as
s= √ 0 . 2545=0 . 50 .
11

Variances of the populations are not known, so we consider that the difference between the two
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom (Sullivan, 2017).
The 95% confidence interval for the difference in mean number of days with an AF event is
evaluated as,
(( x
¿
1−x
¿
2)±t1−α /2∗
√ s1
2
n1
+ s2
2
n2 )=−6 . 5±1 . 972∗0 . 50=[−7 . 49 , −5 .51 ].
B. Mean number of days with an AF event assigned to receiving the new pacemaker in the
sample x
¿
=8 . 4 and the standard deviation s=3 . 2 .
The 95% confidence interval for mean age of participants assigned to the placebo is evaluated as
x
¿
±t 1−α
2
∗ s
√ n at 99 degrees of freedom. As information about sample data is available, we use t-
test for the evaluation of confidence interval. The t-value for 5% level of significance with 99
degrees of freedom (n – 1 = 99) is 1.984 (Cross, & Daniel, 2013).
Hence, the 95% confidence interval is 8 . 4±1. 984∗3 .2
10 =8 . 4±0 . 635=[7 . 76 , 9 . 03 ] .
With 95% confidence it is possible to conclude that the mean number of days with an AF event
assigned to receiving the new pacemaker can be anywhere between 7.76 days and 9.03 days, or
roughly speaking between 8 days and 9 days.
12
groups will follow the t-distribution with n1+ n2−2=198 degrees of freedom (Sullivan, 2017).
The 95% confidence interval for the difference in mean number of days with an AF event is
evaluated as,
(( x
¿
1−x
¿
2)±t1−α /2∗
√ s1
2
n1
+ s2
2
n2 )=−6 . 5±1 . 972∗0 . 50=[−7 . 49 , −5 .51 ].
B. Mean number of days with an AF event assigned to receiving the new pacemaker in the
sample x
¿
=8 . 4 and the standard deviation s=3 . 2 .
The 95% confidence interval for mean age of participants assigned to the placebo is evaluated as
x
¿
±t 1−α
2
∗ s
√ n at 99 degrees of freedom. As information about sample data is available, we use t-
test for the evaluation of confidence interval. The t-value for 5% level of significance with 99
degrees of freedom (n – 1 = 99) is 1.984 (Cross, & Daniel, 2013).
Hence, the 95% confidence interval is 8 . 4±1. 984∗3 .2
10 =8 . 4±0 . 635=[7 . 76 , 9 . 03 ] .
With 95% confidence it is possible to conclude that the mean number of days with an AF event
assigned to receiving the new pacemaker can be anywhere between 7.76 days and 9.03 days, or
roughly speaking between 8 days and 9 days.
12

References
Cross, C. L., & Daniel, W. W. (2013). Student solutions manual to accompany Biostatistics: A
foundation for analysis in the health sciences, 10th edition Wayne W. Daniel, Ph. D.,
Chad L. Cross, Ph.D., PStat(11th ed.). Hoboken,NJ: John Wiley & Sons.
Le, C.T., & Eberly, L.E. (2016). Introductory Biostatistics(2nd ed.). Hoboken,NJ: John Wiley &
Sons.
Pagano, M., & Gauvreau, K. (2018). Principles of biostatistics(2nd ed.). New York: CRC Press.
doi:https://doi.org/10.1201/9780429489624
Sullivan, L. (2017, October 27). Confidence Intervals. Retrieved February 22, 2019, from
http://sphweb.bumc.bu.edu/otlt/MPH-Modules/BS/BS704_Confidence_Intervals/
BS704_Confidence_Intervals8.html
13
Cross, C. L., & Daniel, W. W. (2013). Student solutions manual to accompany Biostatistics: A
foundation for analysis in the health sciences, 10th edition Wayne W. Daniel, Ph. D.,
Chad L. Cross, Ph.D., PStat(11th ed.). Hoboken,NJ: John Wiley & Sons.
Le, C.T., & Eberly, L.E. (2016). Introductory Biostatistics(2nd ed.). Hoboken,NJ: John Wiley &
Sons.
Pagano, M., & Gauvreau, K. (2018). Principles of biostatistics(2nd ed.). New York: CRC Press.
doi:https://doi.org/10.1201/9780429489624
Sullivan, L. (2017, October 27). Confidence Intervals. Retrieved February 22, 2019, from
http://sphweb.bumc.bu.edu/otlt/MPH-Modules/BS/BS704_Confidence_Intervals/
BS704_Confidence_Intervals8.html
13
1 out of 13
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.
