College Algebra - Solved Assignments, Essays, Dissertation

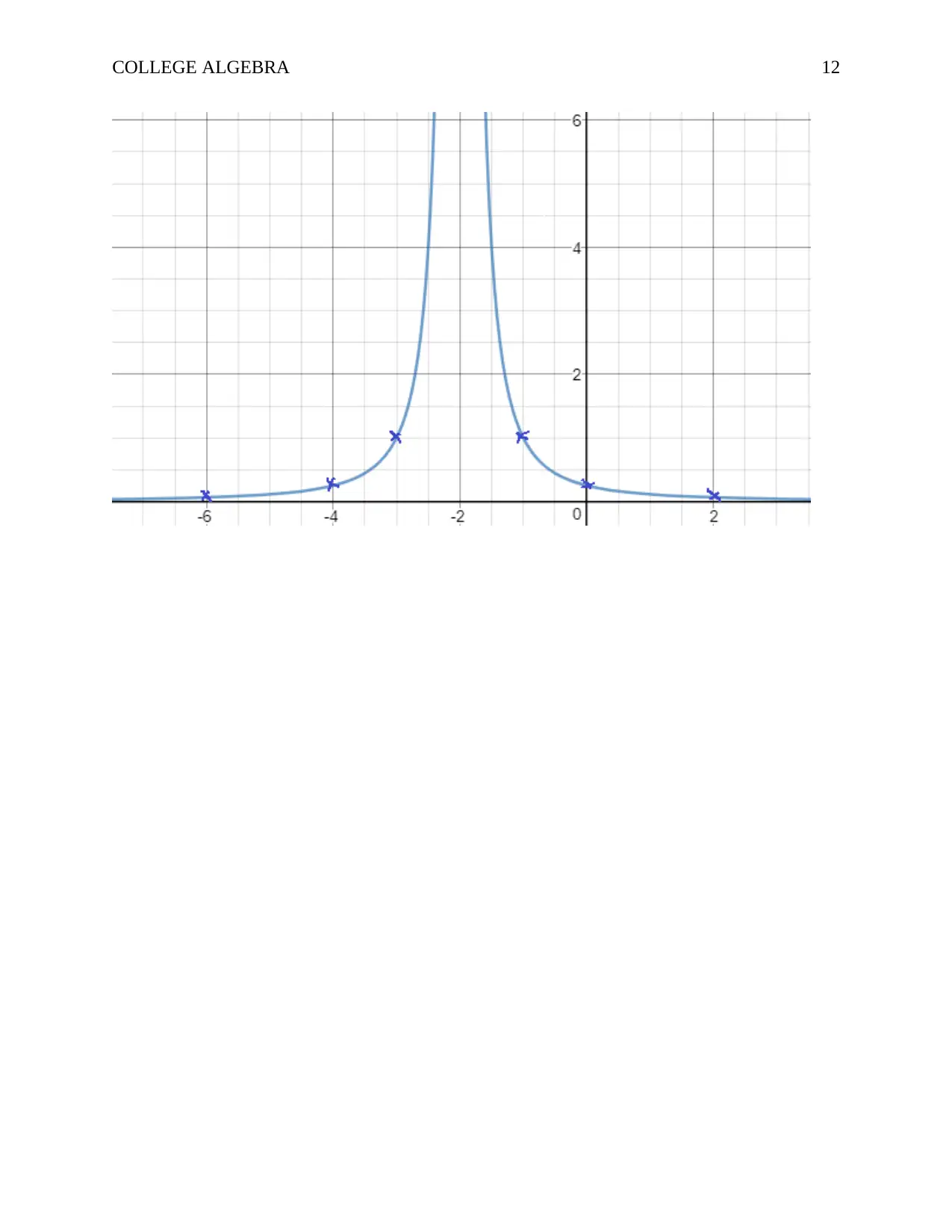
This content provides solved assignments, essays, and dissertation for College Algebra. It covers topics such as functions, parabolas, polynomials, and asymptotes. The content also includes graphs and calculations to help students understand the concepts better.

Running head: COLLEGE ALGEBRA 1
College Algebra
Name
Institution

COLLEGE ALGEBRA 2
Question 1
The equation of the function in the plot is j ( x )=−x2−1. That is, option d. The graph has a y-
intercept of j ( x )=−1. Additionally, the function decreases for all value of x denoting that
j ( x )=−x2−1 .
Question 2
The parabola f ( x )=2 x2−8 x +3
The vertex of the parabola is the stationary minimum point. We get the point by getting the
derivative of the function f ( x ) and then equating it to zero. That is,
d f ( x )
dx = d
dx ( 2 x2−8 x +3 )=4 x−8=0
4 x−8=0 , 4 x=8 , x= 8
4 =2
At x=2 , f ( x )=2(2)2−8 ( 2 ) +3=8−16+3=−5
The vertex is at x=2∧ y=−5

COLLEGE ALGEBRA 3
Question 3
Part a
f ( x )=4−(x −1)2
f ( x )=4− ( x2−2 x+1 ) =3−x2 +2 x
We have the vertex when, d f ( x )
dx =0.That is ,
d
dx (3−x2 +2 x )=−2 x+ 2=0
−2 x+2=0 , 2 x=2, x= 2
2 =1
When x=1 , f ( x )=4− (1−1 )2=4
The vertex is at the point (1 , 4)
The y-intercept is f ( 0 ) =4− ( 0−1 ) 2=4−1=3
The x-intercept is f ( x )=3−x2+ 2 x=−x2 +2 x+ 3=0
x=−2 ± √ 22 −4 (−1 ×3)¿ ¿
2(−1)=−2 ± √ 16
−2 =−2 ± 4
−2 =1±−2
x=1+ (−2 ) =−1∨x=1−−2=3
Using the obtained vertex and intercepts, we can plot the graph of the function as shown in the
figure below.

COLLEGE ALGEBRA 4
Part b
The axis of symmetry is x=1
Part c
The domain of the function from the graph is −∞ ≤ x ≤+∞ while the range is −∞ ≤ f ( x ) ≤ 4
Question 4
f ( x ) =−0.8 x2 +2.4 x +6
Part a
At maximum height, d f ( x )
dx =0= d
dx (−0.8 x2 +2.4 x +6)

COLLEGE ALGEBRA 5
d
dx (−0.8 x2+ 2.4 x+ 6 ) =−1.6 x+2.4=0
1.6 x=2.4 , x= 2.4
1.6 =1.5
When x=1.5 , f ( x )=−0.8(1.5)2+2.4 ( 1.5 )+ 6=7.8
The ball reaches a maximum height of 7.8 feet which occurs at 7.8−6=1.8 feetfrom where it
was thrown.
Part b
Given f ( x ) =−0.8 x2 +2.4 x +6 , the ball touchesthe ground when f ( x ) =0
−0.8 x2+ 2.4 x+ 6=0
x=−2.4 ± √2.42−4 (−0.8 ×6)¿ ¿
2(−0.8)=−2.4 ± √24.96
−1.6 =−2 .4 ± 4.996
−1.6 =1.5 ±−3.122
x=1 .5+ (−3.122 )=−1.622 ( negative ignored )∨x =1.5−−3.122=4.622≅ 4.6
Thus, the ball travels 4.6 feet horizontally before touching the ground.
Part c
The graph of the function f ( x )=−0.8 x2 +2.4 x +6 is shown below.

COLLEGE ALGEBRA 6
Question 5
Option b, f ( x )=7 x2−2 x5+ 1 is the polynomial function with degree 5. That is, it has the highest
power of x that is equal to 5.
Question 6
f ( x )=−5 x4 + 7 x2−x +9
The degree of the polynomial is 4 which is even denoting that its graph will have the same
behavior at each end. In the function, x4 is the leading term since it has the highest power. The
coefficient of x4 is negative 5. For a polynomial function with even degree and negative leading
coefficients, the graph falls to left and to the right. That is, option (b).
Question 7

COLLEGE ALGEBRA 7
Part a
f ( x ) =−x4 +4 x2
The degree of the polynomial is 4 which is even denoting that its graph will have the same
behavior at each end. In the function, x4 is the leading term since it has the highest power. The
coefficient of x4 is -1. For a polynomial function with an even degree and a negative leading
coefficient, the graph falls to left and to the right.
Part b
The x-intercepts f ( x ) =−x4 +4 x2 occurs when f ( x )=0
f ( x )=−x4 +4 x2=0
−x4 + 4 x2=x2(−x¿ ¿2+4)=0¿
x2=0 , x=0
¿ ¿
The x-intercepts are x=0 ,∨x=−2∨x =+ 2
The graph touches the x-axis and turns around at x=0 but crosses the x-axis when
x=−2∧x=+2.
Part c
The y-intercept is f ( x=0 ) =−04 + 4(0)2=0
Part d
The graph of f ( x ) =−x4 +4 x2 has y-axis symmetry.

COLLEGE ALGEBRA 8
Part e
Given that f ( x ) =−x4 +4 x2 ,we use the points shown in the table below to sketch its graph.
x -2 -1.5 -1 0 1 1.5 2
f ( x ) 0 3.94 3 0 3 3.94 0
The turning points of f ( x )=−x4 +4 x2 occur when df ( x )
dx = d
dx (−x¿ ¿ 4+4 x2)=0 ¿
d
dx (−x ¿¿ 4+ 4 x2)=−3 x3 +8 x=0 ¿

COLLEGE ALGEBRA 9
The highest power of df ( x )
dx is 3 implying that f ( x ) has 3 stationary points denoting that the graph
is drawn correctly.
Question 8
Question 9
❑
❑
❑
❑ =x2+x−3− 12
x2 + x−2
Therefore, the quotient is x2+ x−3 while the remainder is −12
Question 10
(x ¿¿ 2−5 x−5 x3+ x4)÷(5+ x )¿
We write the polynomial in descending order to obtain,
(x ¿¿ 2−5 x−5 x3+ x4)÷(5+ x )=x4 −5 x3 + x2−5 x ¿ ÷( x +5)

COLLEGE ALGEBRA 10
Question 11
Question 12
Question 13
g ( x )= x−3
x2−9
g ( x ) = x−3
x2−9 = x−3
( x−3)( x +3)= 1
x +3
as x →−3 , g ( −3 ) = 1
−3+3 =∞
Therefore, the function has a vertical asymptote x=−3. However, there are no holes.
Question 14
g ( x ) = 12 x2
3 x2 +1

COLLEGE ALGEBRA 11
Evidently the leading terms in the numerator and denominator have the same degree. That is,
degree 2. We get the horizontal asymptote by dividing the leading terms to obtain,
y= 12 x2
3 x2 =4
Therefore, the horizontal asymptote is y=4
Question 15
g ( x ) = 1
(x +2)2
To obtain g ( x )= 1
(x +2)2 from f ( x )= 1
x2
First, we draw f ( x )= 1
x2 followed a shift of 2 units to the left. The graph of g ( x ) is shown below.
x -6 -4 -3 -2 -1 0 2
g(x) 0.063 0.25 1 ∞ 1 0.25 0.063

COLLEGE ALGEBRA 12