Analysis of Likert Scale Data: Descriptive & Inferential Statistics

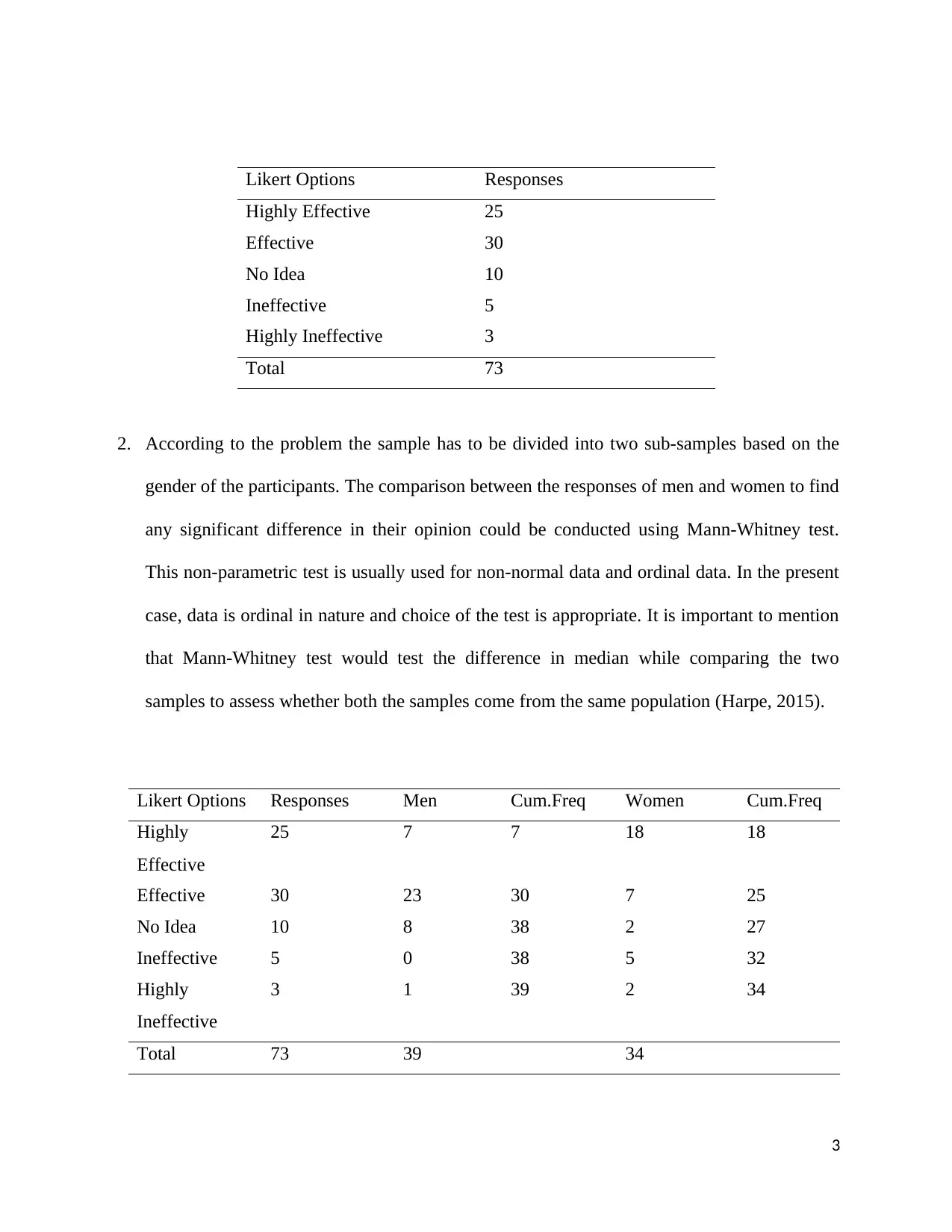
This assignment analyzes Likert scale data obtained from a needs assessment survey. The solution identifies the median, mode, and frequencies as appropriate descriptive statistics due to the ordinal nature of Likert data, explaining why these are preferred over mean and standard deviation. The assignment then addresses how to compare responses from men and women using the Mann-Whitney test, a non-parametric test suitable for ordinal data, to determine if there are significant differences between the two groups. It outlines the steps for hypothesis testing, including null and alternative hypotheses, level of significance, and the calculation of the test statistic (U) and p-value. The conclusion indicates that there is no significant difference between the two samples based on the calculated p-value.

Descriptive and Inferential Analysis of
Likert Scale
1

1. Likert data can be analyzed by comprehending the measurement scale represented by it.
Generally, any numeric figure associated with a Likert data define a “less than” or “greater
than” type of ordinal relation, however, lesser or greater amounts are not explicitly
mentioned. Therefore, the three descriptive statistics suggested for measure of central
tendency are median or mode, whereas frequencies for measure of deviation Again, Likert
scale data is an interval measurement scale evaluated from the compound score from the
Likert data by considering their average or sum. Descriptive statistics include mean for
central tendency and standard deviation for measure of dispersion. The reason behind
choosing median or mode, whereas frequencies as the three statistics for describing the
shape of the data due to the ordinal nature of Likert data. Statistics like mean and standard
deviation would give inappropriate and unclear meaning in describing the data, especially if
there is clustering of responses at a particular option in the data (Sullivan, & Artino Jr,
2013).
For example, opinions about effectiveness of an on-field industrial training are collected
on a five point Likert scale with five options ranging from “highly effective” to “highly
ineffective”. Now, if mean and standard deviation are used to understand the shape of the
data then average of the scores from “highly effective” and “effective” would provide
useless information. In this case, frequencies for each response, and the median/mode for the
distribution would help in describing the location of the peak and the variability. In case of
clustering of responses, median, mode and frequencies would efficiently help in describing
the shape of the distribution (Subedi, 2016).
2

Likert Options Responses
Highly Effective 25
Effective 30
No Idea 10
Ineffective 5
Highly Ineffective 3
Total 73
2. According to the problem the sample has to be divided into two sub-samples based on the
gender of the participants. The comparison between the responses of men and women to find
any significant difference in their opinion could be conducted using Mann-Whitney test.
This non-parametric test is usually used for non-normal data and ordinal data. In the present
case, data is ordinal in nature and choice of the test is appropriate. It is important to mention
that Mann-Whitney test would test the difference in median while comparing the two
samples to assess whether both the samples come from the same population (Harpe, 2015).
Likert Options Responses Men Cum.Freq Women Cum.Freq
Highly
Effective
25 7 7 18 18
Effective 30 23 30 7 25
No Idea 10 8 38 2 27
Ineffective 5 0 38 5 32
Highly
Ineffective
3 1 39 2 34
Total 73 39 34
3

Let, X is the variable for responses by men, and Y is the variable for responses by women.
Median for men is evaluated as 39/2 = 19.5 (effective), and 34/2 = 17 (Highly effective) for
women. Now, the shape and location of the distributions for both samples along with p-
values will decide the significance of difference. Mann-Whitney also calculates the rank of
the responses and compares them. The hypothesis testing would follow the following steps.
Null hypothesis: The two medians are same
Alternate hypothesis: Two median are significantly different (two-tailed)
Level of significance is considered to be 5%.
A two-tailed hypothesis testing would yield the test statistic U = 527.5, where Z-score =
1.49 with p-value = 0.136.
Hence, this implies there is no significant difference between the two samples.
References
Sullivan, G. M., & Artino Jr, A. R. (2013). Analyzing and interpreting data from Likert-type
scales. Journal of graduate medical education, 5(4), 541-542.
Subedi, B. P. (2016). Using Likert type data in social science research: Confusion, issues and
challenges. International journal of contemporary applied sciences, 3(2), 36-49.
Harpe, S. E. (2015). How to analyze Likert and other rating scale data. Currents in Pharmacy
Teaching and Learning, 7(6), 836-850.
4

5