Design of Shank for Biological Purpose - Analysis and Formulations
VerifiedAdded on 2023/06/15
|6
|1061
|197
AI Summary
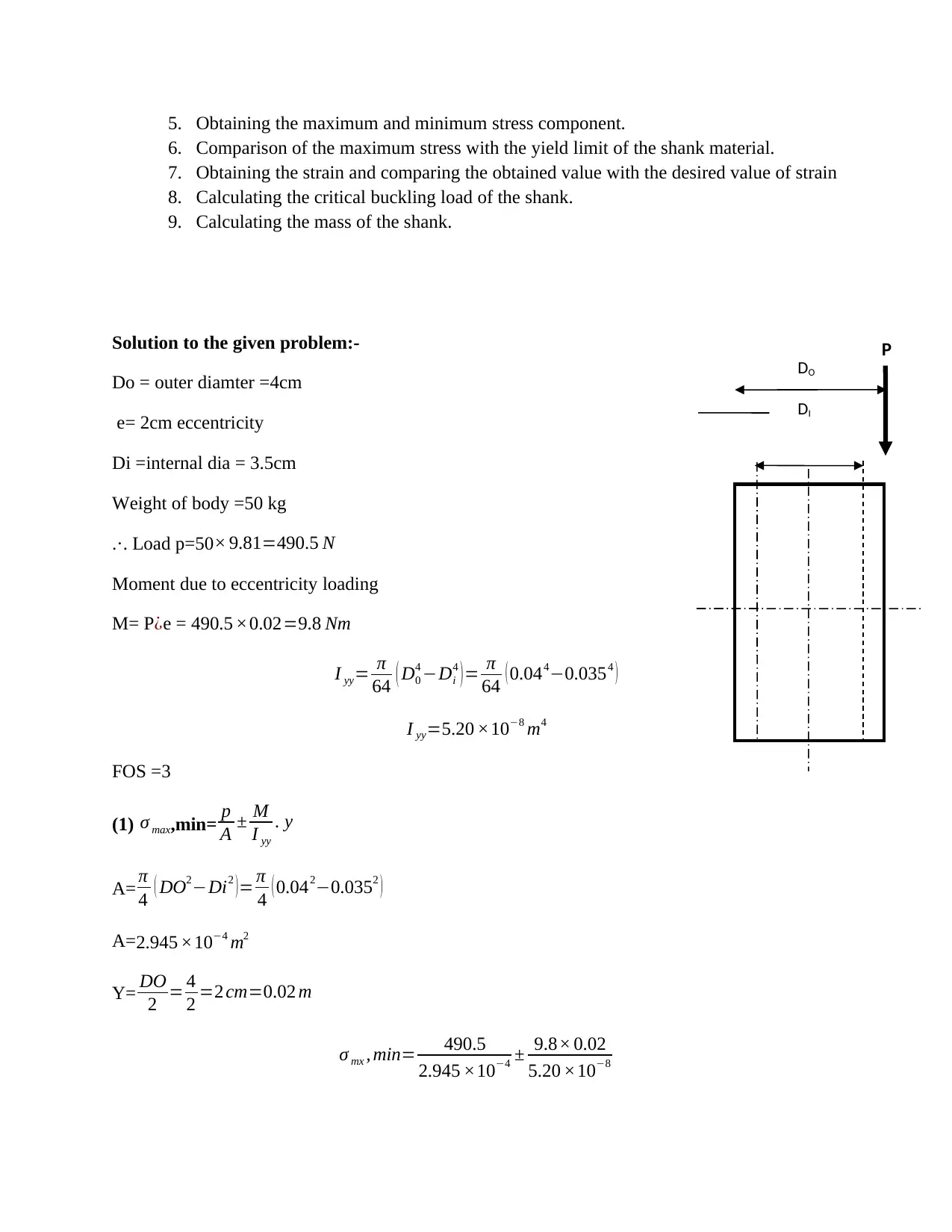
This article discusses the design of a shank for biological purpose, which is to be used as a bone to connect joints of socket and foot through a pin joint. It covers the analysis and formulations required for the design of the shank, including the assumptions made by Euler’s theory of buckling, the types of columns, and the methodology adopted. The article also provides a solution to the given problem, including the critical buckling load of the shank and the mass of the shank.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
1 out of 6







![[object Object]](/_next/static/media/star-bottom.7253800d.svg)