SEO Suggestions for Desklib - Online Library for Study Material

This article discusses various mathematical problems related to sets, limits, vectors, functions, and asymptotes. It also provides SEO suggestions for Desklib, an online library for study material with solved assignments, essays, dissertations, and more.

Question 1
a) Z : Set of Integers , k =−2 ,−1,0,1,2 C= {−4 ,−1,2,5,8 }
A ∩ B∩ C={}… an empty set
¿
( Z ∩ B ) ( A ∪C ¿= { 1,2,3,4,5 }− {[ 3,5 ] ⊔ [−4 ,−1,2,5,8 ] }={1}
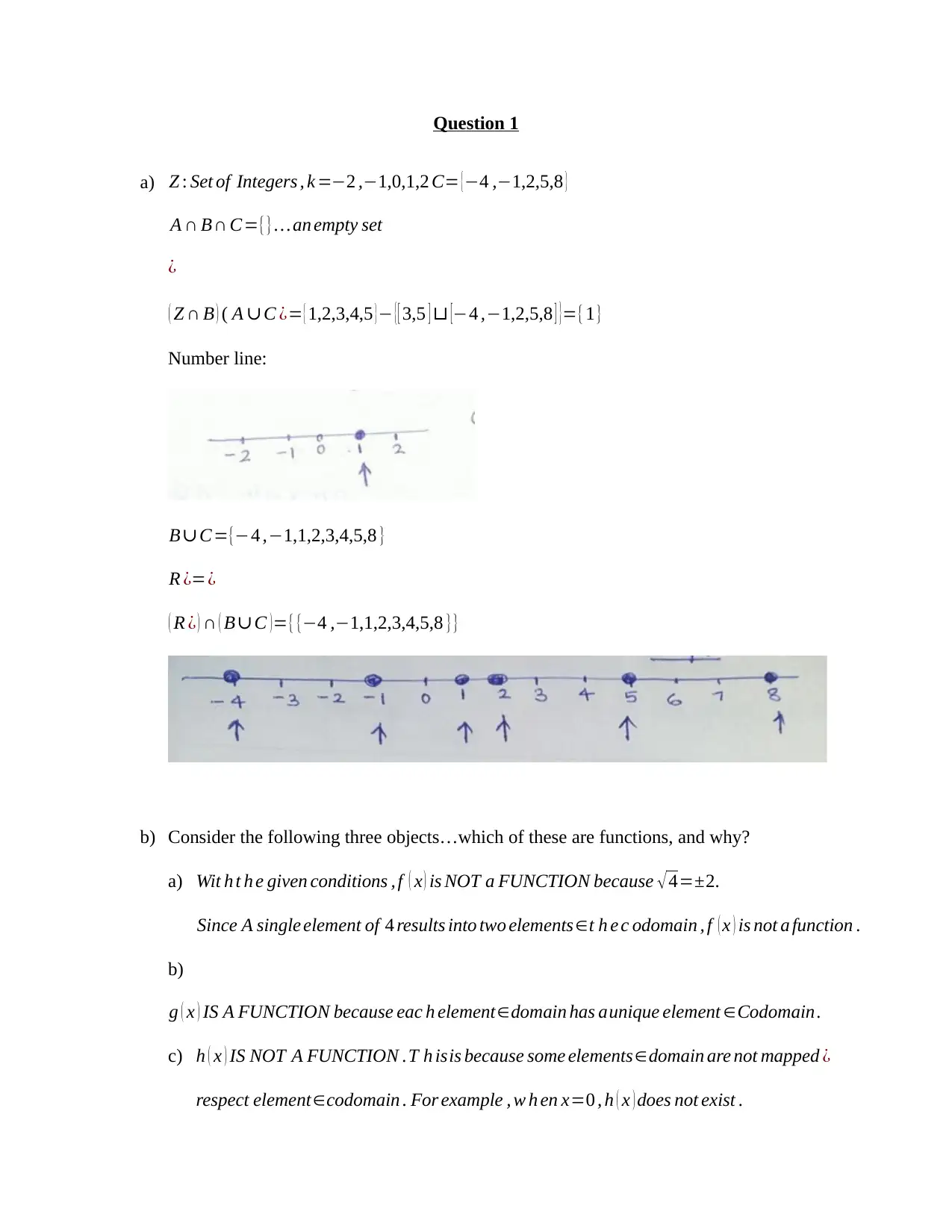
Number line:
B∪C={−4 ,−1,1,2,3,4,5,8 }
R ¿=¿
( R ¿ ) ∩ ( B∪C ) ={{−4 ,−1,1,2,3,4,5,8 }}
b) Consider the following three objects…which of these are functions, and why?
a) Wit h t h e given conditions , f ( x ) is NOT a FUNCTION because √ 4=±2.
Since A single element of 4 results into two elements∈t h e c odomain , f ( x ) is not a function .
b)
g ( x ) IS A FUNCTION because eac h element∈domain has aunique element ∈Codomain.
c) h ( x ) IS NOT A FUNCTION .T h isis because some elements∈domain are not mapped ¿
respect element∈codomain . For example , w h en x=0 , h ( x ) does not exist .

Question 2
a) lim
x →3
x2 −4 X +3
X 2−6 X +9
If lim
x → a−¿f (x)≠ lim
x→ a+ ¿f ( x)t hen t he limitdoes not exist.¿
¿¿
¿
x2 −4 x +3
x2−6 x+9 = x−1
x−3
lim
x →3
x2 −4 X +3
X 2−6 X +9 = lim
x →3 +¿ x−1
x−3 ¿
¿
x−1
x−3 = ( x−1 )∗1
x−3
lim
x→ a
[f ( x )∗g( x)]=lim
x → a
f ( x)∗lim
x → a
g(x )
lim
x→ 3+¿ x−1
x−3 = lim
x→ 3+¿ (x−1 )∗ lim
x → 3+¿ 1
x −3=2∗ ∞ ¿
¿ ¿
¿¿
¿
¿ ∞
lim
x→ 3−¿ x2−4 X +3
X2−6 X +9 =−∞ ¿
¿
∞ ≠−∞, hence t h e limit does not exist
b) lim
x→ π
2
sin2 x−2 sin x +1
sin2 x−5 sin x +4
sin2 x−2 sin x+1
sin2 x−5sin x +4 = sin x−1
sin x−4

lim
x→ π
2
sin2 x−2 sin x +1
sin2 x−5 sin x +4 =lim
x → π
2
sin x −1
sin x−4 =
sin π
2 −1
sin π
2 −4
=0
c) lim
x→ ∞
ln¿ ¿ ¿
x is positive w h en x → ∞ .T hus| x|=x
T h e Limit C h ain Rule States t h at , if lim
u→ b
f ( u ) =L,∧lim
x→ a
g ( x ) =b ,∧f (x)is
continuous at x=b , t h en lim
x →a
f (g ( x ) )=L
g ( x ) =ln ¿ ¿
lim
x→ ∞
¿ ¿
lim
x→ ∞
ln u=∞
Thus lim
x→ ∞
ln¿ ¿ ¿
d) lim
x→+∞
x2−4 x +3
x 62−6 x +9
Dividing t h e function by t h e high est denominator power , we have
1− 4
x − 3
x2
1− 6
x + 9
x2
lim
x→+∞
1− 4
x − 3
x2
1− 6
x + 9
x2
= 1−0−0
1−0−0 =1
e) lim
x→ 0−¿ x+ √x2
x ¿
¿
Multiplying by t h e conjugate of x + √ x2 , we have x+ √ x2
x =
0
x− √ x2
x

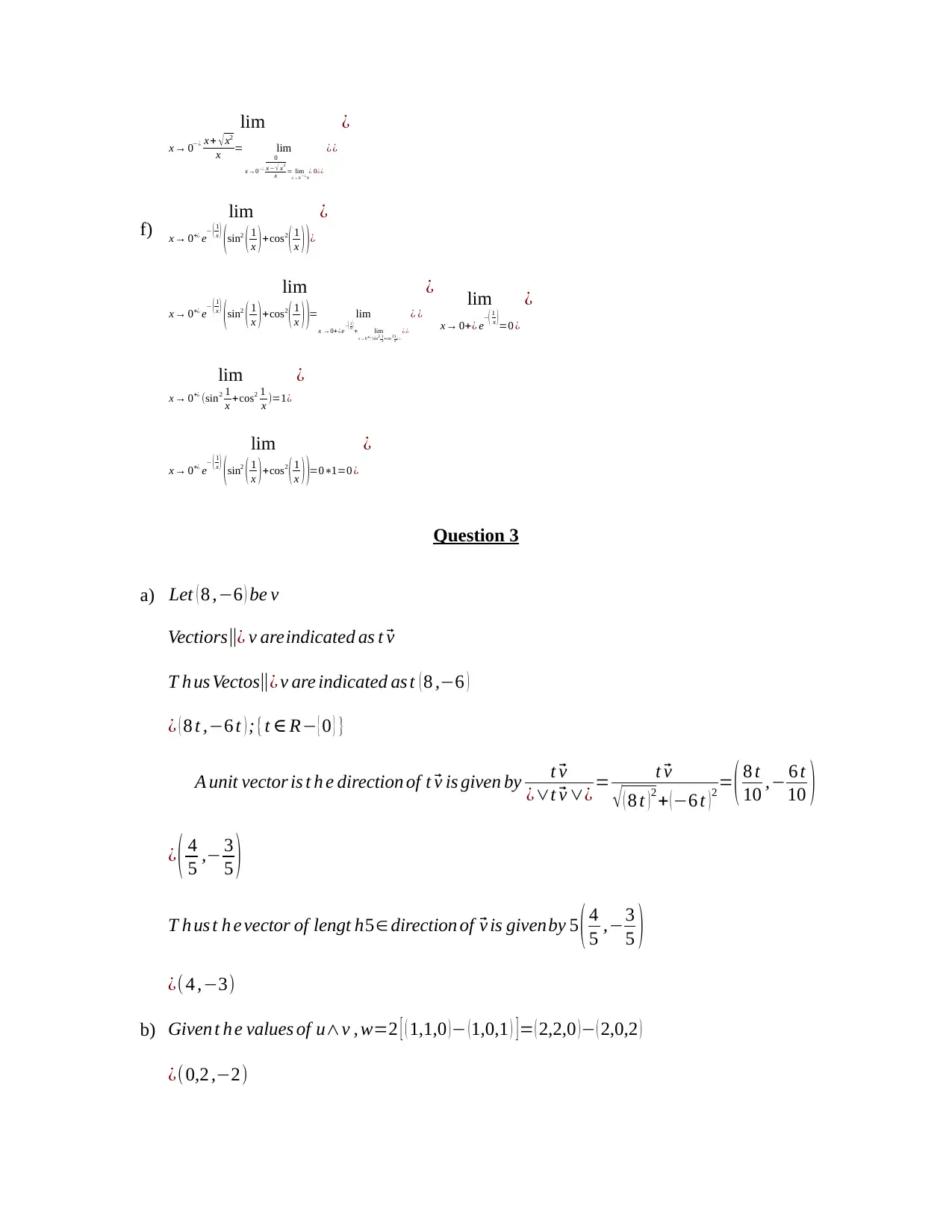
lim
x→ 0−¿ x+ √x2
x = lim
x→0−¿
0
x− √ x2
x = lim
x → 0−¿ 0
¿ 0¿¿
¿¿
¿
f) lim
x→ 0+¿ e− ( 1
x ) (sin2
( 1
x )+cos2
( 1
x ) )¿
¿
lim
x→ 0+¿ e− ( 1
x ) (sin2
( 1
x )+cos2
( 1
x ) )= lim
x →0+ ¿e−( 1
x )∗ lim
x → 0+¿ (sin2 1
x +cos 21
x ) ¿
¿¿
¿ ¿
¿ lim
x→ 0+¿ e−( 1
x )=0 ¿
¿
lim
x→ 0+¿ (sin2 1
x +cos2 1
x )=1¿
¿
lim
x→ 0+¿ e− ( 1
x ) ( sin2
( 1
x ) +cos2
( 1
x ) )=0∗1=0 ¿
¿
Question 3
a) Let ( 8 ,−6 ) be v
Vectiors∥¿ v areindicated as t⃗ v
T h us Vectos∥¿ v are indicated as t ( 8 ,−6 )
¿ ( 8 t ,−6 t ) ;{t ∈ R− { 0 } }
A unit vector is t h e direction of t⃗ v is given by t⃗ v
¿∨t⃗ v ∨¿ = t⃗ v
√ ( 8 t ) 2 + ( −6 t ) 2 = ( 8 t
10 ,− 6 t
10 )
¿ ( 4
5 ,− 3
5 )
T h us t h e vector of lengt h5∈ direction of⃗ v is givenby 5 ( 4
5 ,−3
5 )
¿( 4 ,−3)
b) Given t h e values of u∧v , w=2 [ ( 1,1,0 )− (1,0,1 ) ]= ( 2,2,0 )− ( 2,0,2 )
¿( 0,2 ,−2)

u . w=||u||||w||cos θ
W h ere θ is an ´angle between u∧w .
||u||= √ 12 +12 + ( 0 ) 2= √ 2
||w||= √02 +22 + (−2 )2=2 √2
u . w= ( 1,1,0 ) . ( 0,2 ,−2 )=2
T h us 2=√ 2 ( 2 √2 ) cos θ
cos θ= 2
4 =1
2
θ=cos−1 1
2 = π
3
c)
Let us assume t h at x anf y are scalar quantities . Additionally a is a linear combination of u∧v
suc h t h at ||a||= √ 3∧a . w=0 , we have t h at
a=xu+ yv
a= ( 2 x , x+ y , 2 y )
Given t h at a . w=0 , ( 2 x , x + y , 2 y ) . ( 1,2 ,−3 ) =0
2 x+2 ( x + y )−6 y =0
T h us 4 x−4 y=0
x= y
Additionally ,∨|a|= √ 3 ,
3=||a||
2
( 2 x )2 + ( x + y ) 2 + ( 2 y ) 2=3
Given t h at x= y ,

4 x2 + 4 x2+ 4 x2=3
12 x2=3
x=± √ 1
4 =± 1
2
y=x =± 1
2
T h us possible values of a are :
a=xu+ yu=xu+ xv
¿ x (u+v)
x [ ( 2,1,0 ) + ( 0,1,2 ) ] =x (2,2,2)
W h en x= 1
2 , a=1
2 ( 2,2,2 )=(1,1,1)
W h en x=−1
2 , a=−1
2 ( 2,2,2 ) =(−1 ,−1 ,−1)
T h us possible values of a are ( 1,1,1 )∧(−1 ,−1 ,−1)
Question 4
a) Suppose A= { … 0,1 }∧B= { 0,1 , … } , T h en A∧B have infinitely many numbers∧¿
A ∩ B={0,1}
b)
T h ere are numbers two sets t h at has infinitely many numbers w h o se unition is { 0,1 } . T h is is
because a∪of sets t h at have infinitely many numbers must have ∞ .
c) Suppose A= { 0,12,3,4 , … }∧B= { 2,3,4 , … } , t h en A ¿= { 0,1 } .
Question 5

a) Suppose f ( x )=cot θ , t h en lim
x →0−¿ cot θ , lim
x→ 0+¿ cot θ∧f (0 )does not exist¿
¿¿
¿
b) T h ere is no function g ( x ) suc h g ( 0 ) , t h e rig h t∧¿ exist ∧are different .
c) Suppose we have a function f ( x ) =
2
1+ex +1
( x−2 ) ( x−3 ) ( x−5 ) ,t h en f ( x ) has 2 h orizontal
asymptotes at y=1∧ y =3 ,∧3 vertical asymptotes at x=2,3∧5
d) A function cannot have more than 2 horizontal asymptotes. That is, the maximum
number of horizontal asymptotes that a function can have is 2. Thus you cannot create a
function with exactly 3 different asymptotes and three different vertical asymptotes.
e) Suppose we ha ve a piecewise f ( x ) = {0 ; x= 0∧1
x ; ot h erwise } ,
t h en t h e function is discontinuousat every integer
Question 6
Suppose A= {−100,2,3 } , B= { 0 ,−200,2 } ,C= ( 0,0 ,−300 ) an d D= {1,1,1 ) ,
T h en t h e ˙product between any two of t h ese four is negative . For Example ,
A . B=−100∗1+2∗1+3∗1=−95