Desklib - Online Library for Study Material with Solved Assignments
VerifiedAdded on 2023/06/13
|16
|1767
|171
AI Summary
Desklib is an online library for study material with solved assignments, essays, dissertations, etc. It offers a wide range of study materials for various subjects and courses. This article covers topics such as mathematics, functions, graphs, and velocity.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Running head: MATHEMATICS 1
Mathematics
Name
Institution
Mathematics
Name
Institution
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

MATHEMATICS 2
Question 1
Part a
f ( t )= A ekt (t ≥ 1)
Whent=2 hours , f (t)=195
When t=10 hours , f (t)=98
195= A e2 t … (i)
98=A e10t … (ii)
Dividing equation ( i )by equation ( ii ) we obtain
195
98 = A e2k
A e10 k
195
98 =e2 k−10 k=e−8 k
lne−8 k =ln ( 195
98 )
−8 k =ln ( 195
98 )
k =ln ( 195
98 ) ÷ ( −8 ) =−0.086
Substituting t into equation 1 we get
195= A e2 k
Question 1
Part a
f ( t )= A ekt (t ≥ 1)
Whent=2 hours , f (t)=195
When t=10 hours , f (t)=98
195= A e2 t … (i)
98=A e10t … (ii)
Dividing equation ( i )by equation ( ii ) we obtain
195
98 = A e2k
A e10 k
195
98 =e2 k−10 k=e−8 k
lne−8 k =ln ( 195
98 )
−8 k =ln ( 195
98 )
k =ln ( 195
98 ) ÷ ( −8 ) =−0.086
Substituting t into equation 1 we get
195= A e2 k

MATHEMATICS 3
A=195
e2 k = 195
e2(−0.086) =231.599
A=232 ,k =−0.086 (¿ 3 sf )
Part b
giventhat f ( t )=3=A ekt
232 e−0.086 t=3
e−0.086 t = 3
231
ln (e−0.086 t )=ln ( 3
232 )
−0.086 t=ln ( 3
232 )=−4.3481
t=−4.3481
−0.086 =50.56 hrs ≅ 51 hours
Question 2
5−2 x
3 x +4 <0
5−2 x=0 , x= 5
2
3 x+ 4=0 , x=−4
3
x ← 4
3 x=−4
3
−4
3 < x < 5
2 x= 5
2 x > 5
2
5−2 x + + + 0 -
A=195
e2 k = 195
e2(−0.086) =231.599
A=232 ,k =−0.086 (¿ 3 sf )
Part b
giventhat f ( t )=3=A ekt
232 e−0.086 t=3
e−0.086 t = 3
231
ln (e−0.086 t )=ln ( 3
232 )
−0.086 t=ln ( 3
232 )=−4.3481
t=−4.3481
−0.086 =50.56 hrs ≅ 51 hours
Question 2
5−2 x
3 x +4 <0
5−2 x=0 , x= 5
2
3 x+ 4=0 , x=−4
3
x ← 4
3 x=−4
3
−4
3 < x < 5
2 x= 5
2 x > 5
2
5−2 x + + + 0 -

MATHEMATICS 4
3 x+ 4 - 0 + + +
5−2 x
3 x +4
- undefined + 0 -
From the table, we chose the ranges that satisfy the required conditions. That is,
x ← 4
3 ∨x > 5
2
Question 3
Part a(i)
f ( x)=x2 + 4 x+1
f ( x )=(x +a)2−b=x2+2 xa+(a¿ ¿2−b) ¿
x2=x2 , 2 xa=4 x ,1=(a ¿¿ 2−b) ¿
2 xa=4 x ,a=2
1=(a¿ ¿2−b)=(2¿¿ 2−b)¿ ¿
1=4−b , b=3
f ( x )=(x +2)2 −3
Part a(ii)
The graph of f ( x)=x2 + 4 x+1can be obtained from f ( x)=x2 by a vertical stretch of 4 followed
by a vertical translation of +1 unit.
Part a(iii)
The image set of f ( x ) is undefined since we don’t have the range of x.
3 x+ 4 - 0 + + +
5−2 x
3 x +4
- undefined + 0 -
From the table, we chose the ranges that satisfy the required conditions. That is,
x ← 4
3 ∨x > 5
2
Question 3
Part a(i)
f ( x)=x2 + 4 x+1
f ( x )=(x +a)2−b=x2+2 xa+(a¿ ¿2−b) ¿
x2=x2 , 2 xa=4 x ,1=(a ¿¿ 2−b) ¿
2 xa=4 x ,a=2
1=(a¿ ¿2−b)=(2¿¿ 2−b)¿ ¿
1=4−b , b=3
f ( x )=(x +2)2 −3
Part a(ii)
The graph of f ( x)=x2 + 4 x+1can be obtained from f ( x)=x2 by a vertical stretch of 4 followed
by a vertical translation of +1 unit.
Part a(iii)
The image set of f ( x ) is undefined since we don’t have the range of x.
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
MATHEMATICS 5
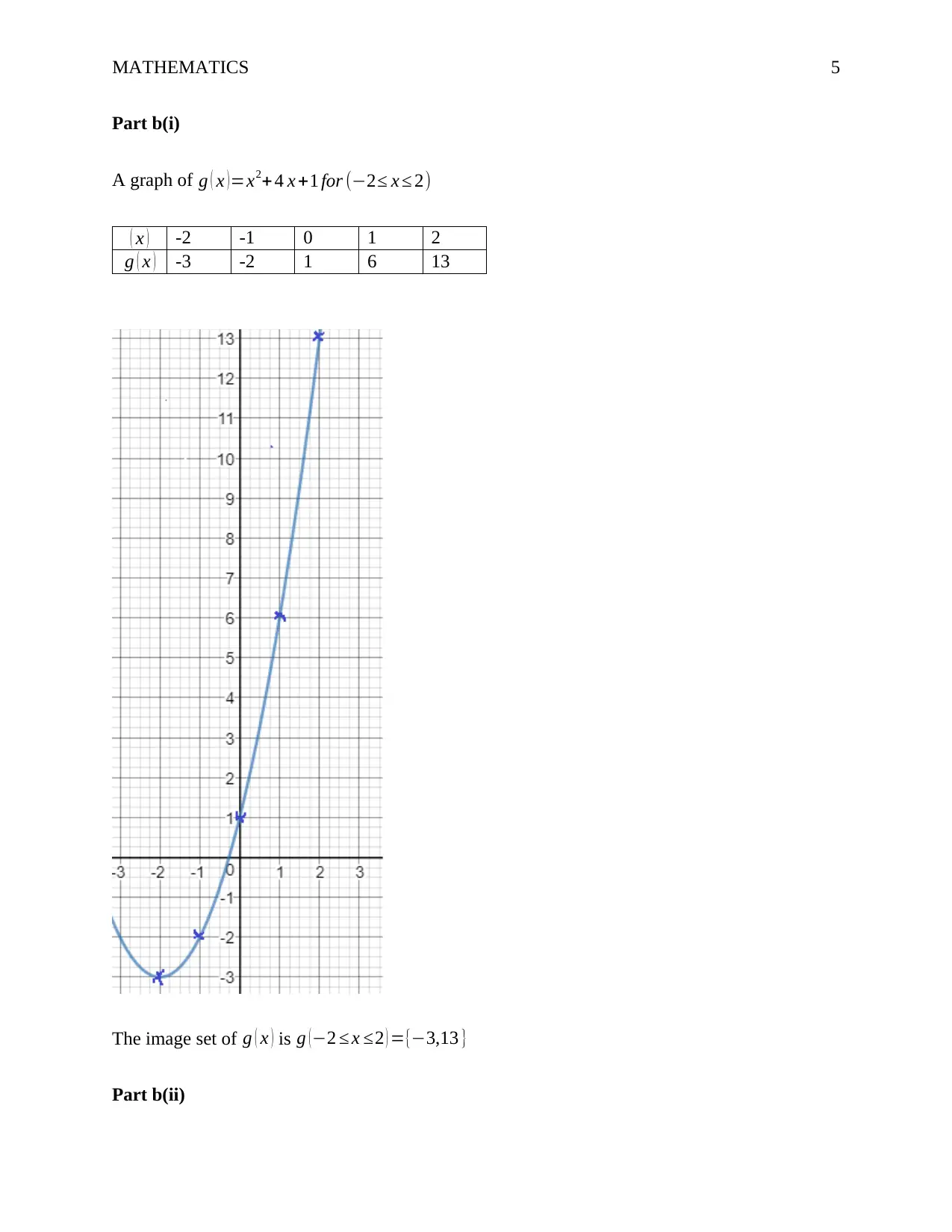
Part b(i)
A graph of g ( x )=x2+ 4 x +1 for (−2≤ x ≤ 2)
( x ) -2 -1 0 1 2
g ( x ) -3 -2 1 6 13
The image set of g ( x ) is g (−2 ≤ x ≤2 ) ={−3,13 }
Part b(ii)
Part b(i)
A graph of g ( x )=x2+ 4 x +1 for (−2≤ x ≤ 2)
( x ) -2 -1 0 1 2
g ( x ) -3 -2 1 6 13
The image set of g ( x ) is g (−2 ≤ x ≤2 ) ={−3,13 }
Part b(ii)

MATHEMATICS 6
Inverse of g ( x )=x2+4 x +1 for (−2≤ x ≤ 2)
y=x2 +4 x+1
Interchanging the variables
x= y2 +4 y +1
y2 +4 y+ ( 1−x )=0
Using the quadratic formula,
y=−b ± √b2−4 ac
2 a =−4 ± √ 42−4(1)(1−x )
2(1)
y=−4 ± √ 12+4 x
2 =−4 ± √ 4( 3+x)
2 =−4 ± 2 √ (3+x )
2 =−2± √ x+3
g ' ( x )=−2+ √ x+ 3 or g ' ( x )=−2− √ x +3
The domain of the function is x ≥−3. That is, (−2 ≤ x ≤ 2)
The image set of g '( x ) is
g' (−2 )=−2+ √−2+3=−1, g' (2 )=−2+ √2+3=0.236, g' ( x ) =[−1 ,0.236 ]
Or
g' (−2 )=−2− √−2+3=−3
g' (2 )=−2− √2+3=−4.236
g' ( x ) =[−3 ,−4.236]
Part b(iii)
Inverse of g ( x )=x2+4 x +1 for (−2≤ x ≤ 2)
y=x2 +4 x+1
Interchanging the variables
x= y2 +4 y +1
y2 +4 y+ ( 1−x )=0
Using the quadratic formula,
y=−b ± √b2−4 ac
2 a =−4 ± √ 42−4(1)(1−x )
2(1)
y=−4 ± √ 12+4 x
2 =−4 ± √ 4( 3+x)
2 =−4 ± 2 √ (3+x )
2 =−2± √ x+3
g ' ( x )=−2+ √ x+ 3 or g ' ( x )=−2− √ x +3
The domain of the function is x ≥−3. That is, (−2 ≤ x ≤ 2)
The image set of g '( x ) is
g' (−2 )=−2+ √−2+3=−1, g' (2 )=−2+ √2+3=0.236, g' ( x ) =[−1 ,0.236 ]
Or
g' (−2 )=−2− √−2+3=−3
g' (2 )=−2− √2+3=−4.236
g' ( x ) =[−3 ,−4.236]
Part b(iii)
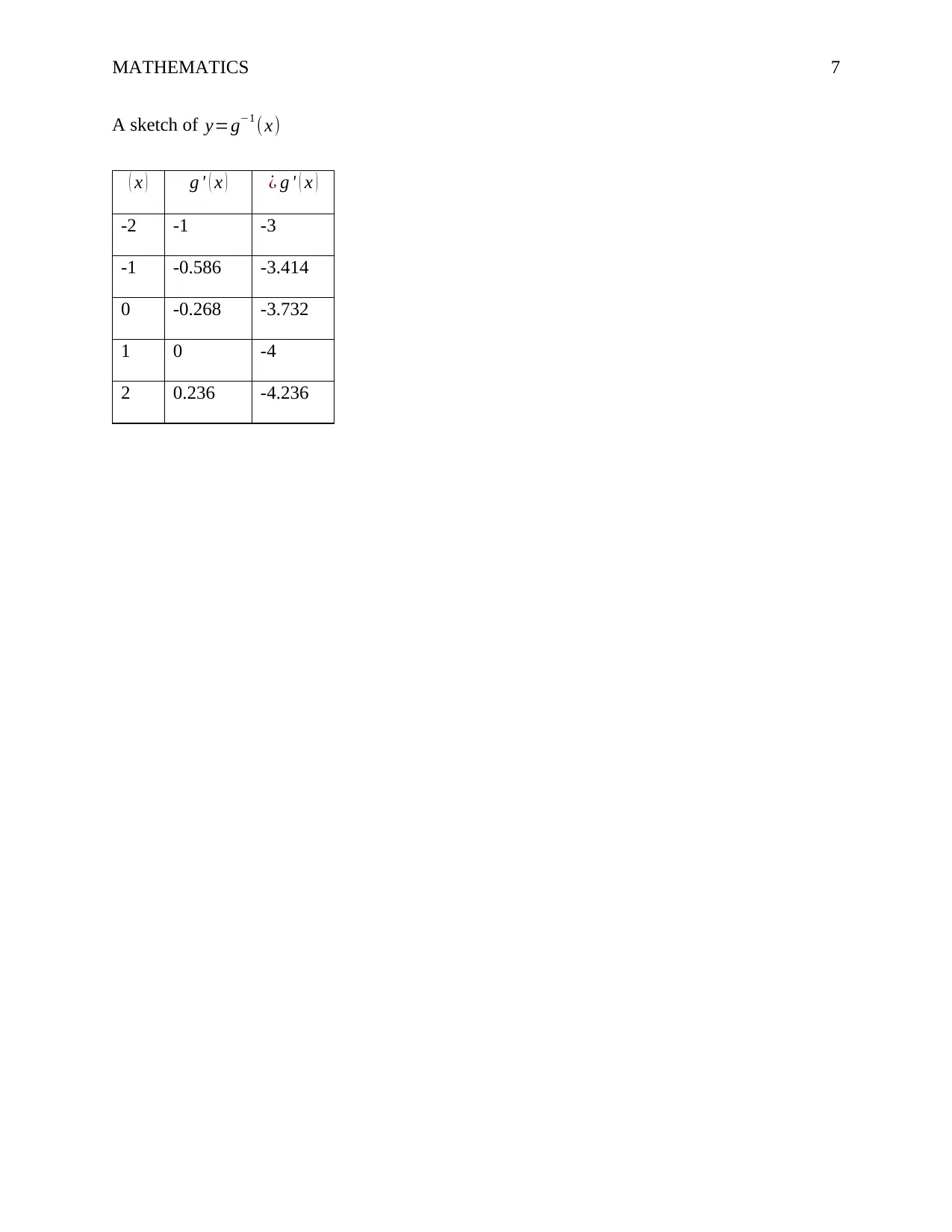
MATHEMATICS 7
A sketch of y=g−1 (x)
( x ) g ' ( x ) ¿ g ' ( x )
-2 -1 -3
-1 -0.586 -3.414
0 -0.268 -3.732
1 0 -4
2 0.236 -4.236
A sketch of y=g−1 (x)
( x ) g ' ( x ) ¿ g ' ( x )
-2 -1 -3
-1 -0.586 -3.414
0 -0.268 -3.732
1 0 -4
2 0.236 -4.236
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
MATHEMATICS 8
Question 4
Part a
cos ( 5 π
12 ), 3 π
4 , π
3
3 π
4 = 3 π × 3
4 ×3 = 9 π
12
Question 4
Part a
cos ( 5 π
12 ), 3 π
4 , π
3
3 π
4 = 3 π × 3
4 ×3 = 9 π
12

MATHEMATICS 9
π
3 = π ×4
3 × 4 = 4 π
12
sin ( 3 π
4 )=sin ( 9 π
12 )= √2
2
cos ( 3 π
4 )=cos ( 9 π
12 )=− √2
2
5 π
12 = 9 π
12 − 4 π
12
sin ( 4 π
12 )= √3
2
cos ( 4 π
12 )=1
2
Using the identity
cos ( A−B )=cosAcosB+ sinAsinB
cos ( 5 π
12 ) =cos ( 9 π
12 ) cos ( 4 π
12 ) +sin ( 9 π
12 ) sin ( 4 π
12 )
¿− √2
2 ( 1
2 )+ √2
2 ( √3
2 )= √ 6
4 − √2
4 = √6− √2
4
Part b
sin ( 5 π
12 )using half angle identity for sine} ¿ cos ( 5 π
6 )
cos ( 5 π
6 )=− √3
2
π
3 = π ×4
3 × 4 = 4 π
12
sin ( 3 π
4 )=sin ( 9 π
12 )= √2
2
cos ( 3 π
4 )=cos ( 9 π
12 )=− √2
2
5 π
12 = 9 π
12 − 4 π
12
sin ( 4 π
12 )= √3
2
cos ( 4 π
12 )=1
2
Using the identity
cos ( A−B )=cosAcosB+ sinAsinB
cos ( 5 π
12 ) =cos ( 9 π
12 ) cos ( 4 π
12 ) +sin ( 9 π
12 ) sin ( 4 π
12 )
¿− √2
2 ( 1
2 )+ √2
2 ( √3
2 )= √ 6
4 − √2
4 = √6− √2
4
Part b
sin ( 5 π
12 )using half angle identity for sine} ¿ cos ( 5 π
6 )
cos ( 5 π
6 )=− √3
2

MATHEMATICS 10
5 π
12 = ( 5 π
6 )
2
sin ( 5 π
12 )=sin ( ( 5 π
6 )
2 )
But we know, sin ( A
2 )= √ 1−cosA
2
sin ( 5 π
12 )=sin ( ( 5 π
6 )
2 )= √ 1−cos ( 5 π
6 )
2 = √ 1− ( − √ 3
2 )
2 = √ ( 1+ √ 3
2 )
2 = √ 2+ √ 3
2
Part c
cos ( 5 π
12 )= √6− √2
4
sin ( 5 π
12 )= √ 2+ √ 3
2
cos2 θ+ sin2 θ=1
cos2
(5 π
12 )+sin2
( 5 π
12 )= ( √6− √2
4 )2
+ ( √2+ √3
2 )2
¿ 6−2 √12+2
16 + 2+ √3
4
¿ 8−2 √ 4 (3)
16 + 2+ √ 3
4
¿ 8−4 √(3)
16 + 2+ √ 3
4
5 π
12 = ( 5 π
6 )
2
sin ( 5 π
12 )=sin ( ( 5 π
6 )
2 )
But we know, sin ( A
2 )= √ 1−cosA
2
sin ( 5 π
12 )=sin ( ( 5 π
6 )
2 )= √ 1−cos ( 5 π
6 )
2 = √ 1− ( − √ 3
2 )
2 = √ ( 1+ √ 3
2 )
2 = √ 2+ √ 3
2
Part c
cos ( 5 π
12 )= √6− √2
4
sin ( 5 π
12 )= √ 2+ √ 3
2
cos2 θ+ sin2 θ=1
cos2
(5 π
12 )+sin2
( 5 π
12 )= ( √6− √2
4 )2
+ ( √2+ √3
2 )2
¿ 6−2 √12+2
16 + 2+ √3
4
¿ 8−2 √ 4 (3)
16 + 2+ √ 3
4
¿ 8−4 √(3)
16 + 2+ √ 3
4
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
MATHEMATICS 11
¿ 8−4 √ ( 3 ) +4 ( 2+ √ 3 )
16
¿ 8−4 √3+8+ 4 √3
16 = 8+8−4 √3+4 √3
16 =16
16 =1
Question 5
Part a
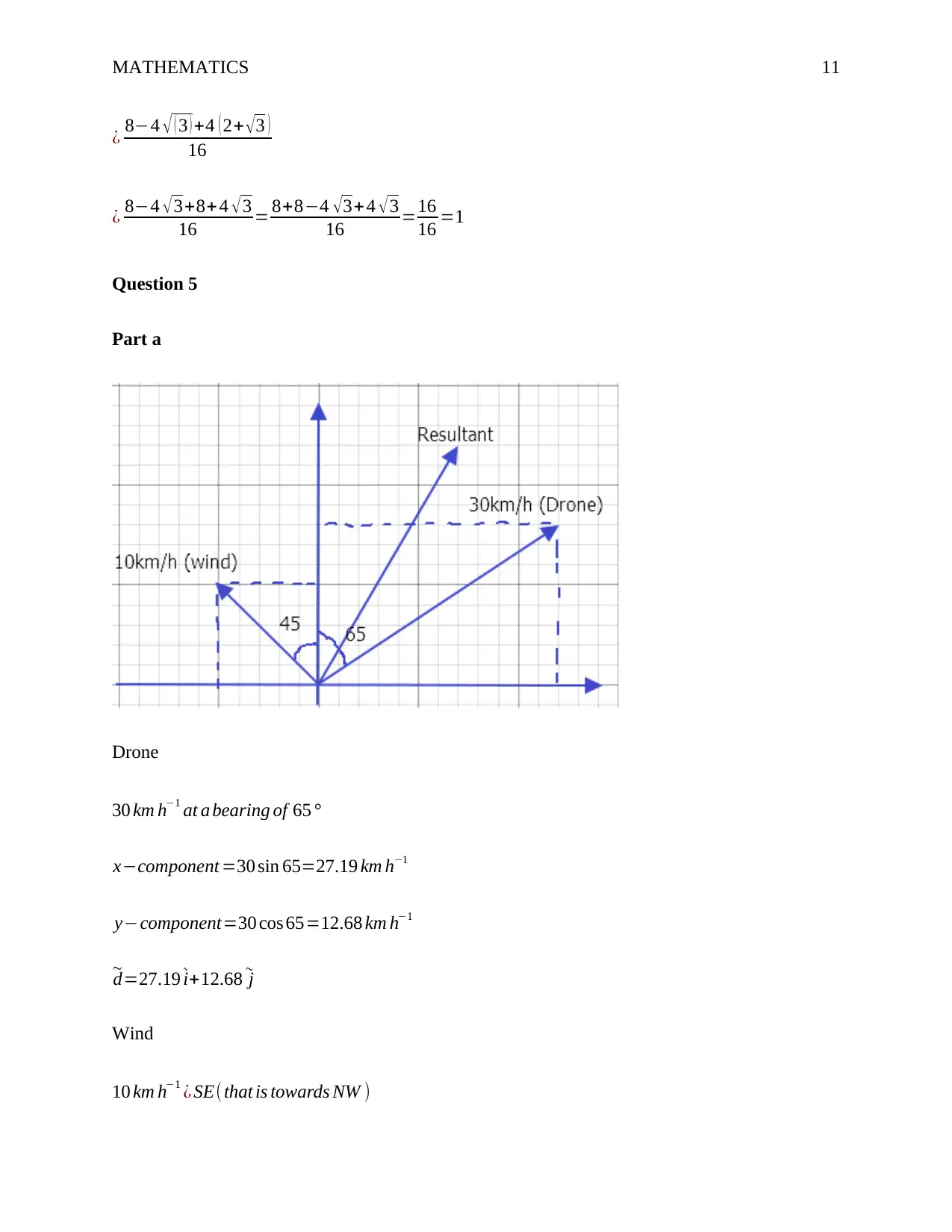
Drone
30 km h−1 at a bearing of 65 °
x−component =30 sin 65=27.19 km h−1
y−component=30 cos 65=12.68 km h−1
~
d=27.19 ~i+12.68 ~j
Wind
10 km h−1 ¿ SE(that is towards NW )
¿ 8−4 √ ( 3 ) +4 ( 2+ √ 3 )
16
¿ 8−4 √3+8+ 4 √3
16 = 8+8−4 √3+4 √3
16 =16
16 =1
Question 5
Part a
Drone
30 km h−1 at a bearing of 65 °
x−component =30 sin 65=27.19 km h−1
y−component=30 cos 65=12.68 km h−1
~
d=27.19 ~i+12.68 ~j
Wind
10 km h−1 ¿ SE(that is towards NW )

MATHEMATICS 12
x−component =10 sin 45=−7.07 km h−1
y−component=10 cos 45=7.07 kmh−1
~w=−7.07 ~
i+ 7.07 ~
j
Part b
Resultant velocity ¿ ~
d +~w
¿ ( 27.19 ~i+ 12.68 ~j ) + (−7.07 ~i+ 7.07 ~j )
¿ 20.12 ~
i+19.75 ~
j
Part c
magnitude= √ 20.122 +19.752=28.19 km h−1
tanθ= 20.12
19.75 =1.018734
θ=tan−1 1.018734=45.53 ≅ 46 °
The resultant velocity of the drone is 28.19 km h−1 at a bearing of 046 °
Question 6
f ( x )=x3 + 9
4 x2 −3 x−2
Part (a)
To get the stationary points, we determine the 1st derivative of the curve and then equate it to
zero as follows:
x−component =10 sin 45=−7.07 km h−1
y−component=10 cos 45=7.07 kmh−1
~w=−7.07 ~
i+ 7.07 ~
j
Part b
Resultant velocity ¿ ~
d +~w
¿ ( 27.19 ~i+ 12.68 ~j ) + (−7.07 ~i+ 7.07 ~j )
¿ 20.12 ~
i+19.75 ~
j
Part c
magnitude= √ 20.122 +19.752=28.19 km h−1
tanθ= 20.12
19.75 =1.018734
θ=tan−1 1.018734=45.53 ≅ 46 °
The resultant velocity of the drone is 28.19 km h−1 at a bearing of 046 °
Question 6
f ( x )=x3 + 9
4 x2 −3 x−2
Part (a)
To get the stationary points, we determine the 1st derivative of the curve and then equate it to
zero as follows:

MATHEMATICS 13
df
dx = d
dx ( x3 + 9
4 x2 −3 x−2
) =3 x2 + 9 ( −2 )
4 x3 −3
¿ 3 x2− 9
2 x3 −3
3 x2− 9
2 x3 −3=0
We multiply the equation by 2 x3 and simplify to get
(3 x ¿¿ 2− 9
2 x3 −3=0)2 x3 =6 x5−9−6 x3 =0 ¿
( x5−x3 )= 9
6 =1.5
x5−x3=1.5
Solving the above equation we obtain, x=1.29826
At x=1.29826 , f ( x )=1.298263 + 9
4 ×1.298262 −3 ( 1.29826 )−2=−2.3717
Hence, the stationary point is ( 1.29826 ,−2.3717 )
Part b
To determine whether the stationary point is a maximum or a minimum we choose values on
either sides of the turning point and evaluate the derivatives as summarized in the table below.
df
dx =3 x2− 9
2 x3 −3
df
dx = d
dx ( x3 + 9
4 x2 −3 x−2
) =3 x2 + 9 ( −2 )
4 x3 −3
¿ 3 x2− 9
2 x3 −3
3 x2− 9
2 x3 −3=0
We multiply the equation by 2 x3 and simplify to get
(3 x ¿¿ 2− 9
2 x3 −3=0)2 x3 =6 x5−9−6 x3 =0 ¿
( x5−x3 )= 9
6 =1.5
x5−x3=1.5
Solving the above equation we obtain, x=1.29826
At x=1.29826 , f ( x )=1.298263 + 9
4 ×1.298262 −3 ( 1.29826 )−2=−2.3717
Hence, the stationary point is ( 1.29826 ,−2.3717 )
Part b
To determine whether the stationary point is a maximum or a minimum we choose values on
either sides of the turning point and evaluate the derivatives as summarized in the table below.
df
dx =3 x2− 9
2 x3 −3
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
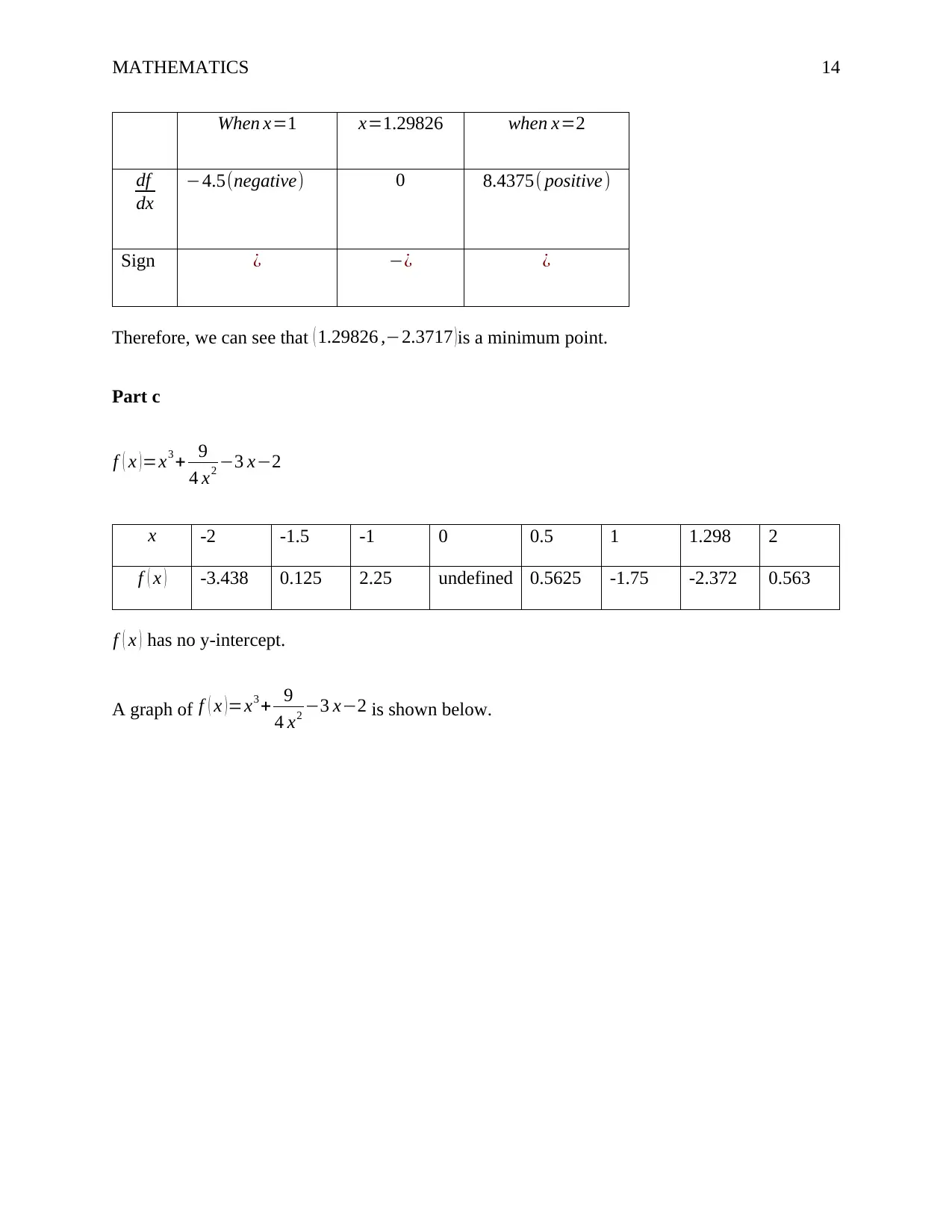
MATHEMATICS 14
When x=1 x=1.29826 when x=2
df
dx
−4.5(negative) 0 8.4375( positive)
Sign ¿ −¿ ¿
Therefore, we can see that ( 1.29826 ,−2.3717 )is a minimum point.
Part c
f ( x )=x3 + 9
4 x2 −3 x−2
x -2 -1.5 -1 0 0.5 1 1.298 2
f ( x ) -3.438 0.125 2.25 undefined 0.5625 -1.75 -2.372 0.563
f ( x ) has no y-intercept.
A graph of f ( x )=x3 + 9
4 x2 −3 x−2 is shown below.
When x=1 x=1.29826 when x=2
df
dx
−4.5(negative) 0 8.4375( positive)
Sign ¿ −¿ ¿
Therefore, we can see that ( 1.29826 ,−2.3717 )is a minimum point.
Part c
f ( x )=x3 + 9
4 x2 −3 x−2
x -2 -1.5 -1 0 0.5 1 1.298 2
f ( x ) -3.438 0.125 2.25 undefined 0.5625 -1.75 -2.372 0.563
f ( x ) has no y-intercept.
A graph of f ( x )=x3 + 9
4 x2 −3 x−2 is shown below.
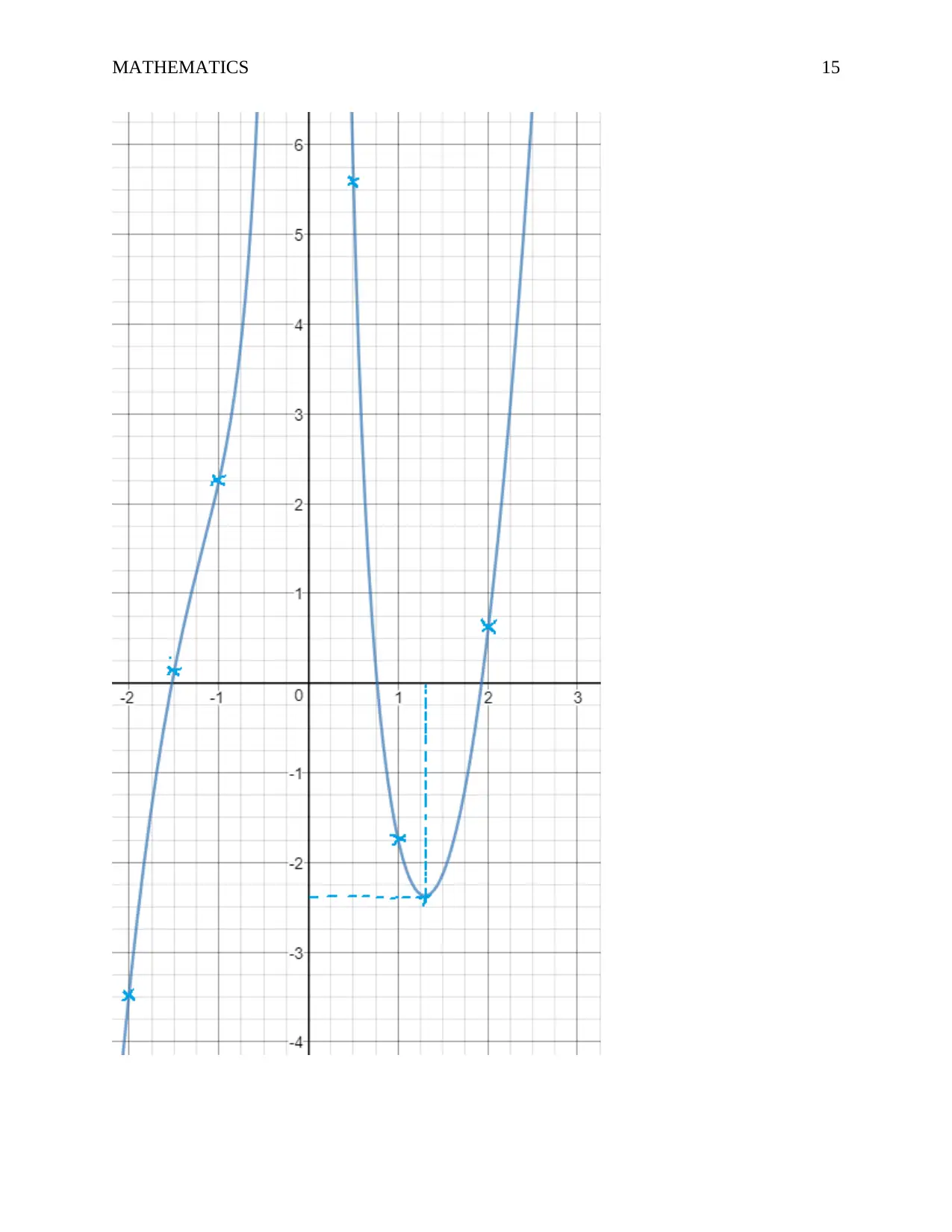
MATHEMATICS 15

MATHEMATICS 16
Part d
In the interval [-3,2] f ( x ) has the least value at x=−3. That is,
f ( −3 ) =(−3)3+ 9
4 (−3)2 −3 ( −3 ) −2=−19.75
However, the largest value approaches infinity (+ ∞) as x approaches zero.
Question 7
s ( t ) =t3 −12t2 +8 t(t ≥ 0)
Part a
Velocity=v (t )= ds ( t )
dt = d
dt ( t3−12 t2+ 8t )=3t2−12(2)t1 +8
v ( t ) =3 t2−24 t +8
At t=−2
v ( 2 )=3(2)2−24 ( 2 ) +8=−28 m s−1
Part b
Acceleration=a ( t )= dV (t )
dt = d
dt ( 3 t2 −24 t+ 8 )=3 ( 2 ) t−24+ 0
a ( t )=6 t−24
Acceleration is zero at t=4 and the minimum velocity at t=4 equals
v ( 4 )=3(4 )2−24 ( 4 ) +8=−40. Hence, the velocity decreases for 0 ≤ t ≤ 4
Part d
In the interval [-3,2] f ( x ) has the least value at x=−3. That is,
f ( −3 ) =(−3)3+ 9
4 (−3)2 −3 ( −3 ) −2=−19.75
However, the largest value approaches infinity (+ ∞) as x approaches zero.
Question 7
s ( t ) =t3 −12t2 +8 t(t ≥ 0)
Part a
Velocity=v (t )= ds ( t )
dt = d
dt ( t3−12 t2+ 8t )=3t2−12(2)t1 +8
v ( t ) =3 t2−24 t +8
At t=−2
v ( 2 )=3(2)2−24 ( 2 ) +8=−28 m s−1
Part b
Acceleration=a ( t )= dV (t )
dt = d
dt ( 3 t2 −24 t+ 8 )=3 ( 2 ) t−24+ 0
a ( t )=6 t−24
Acceleration is zero at t=4 and the minimum velocity at t=4 equals
v ( 4 )=3(4 )2−24 ( 4 ) +8=−40. Hence, the velocity decreases for 0 ≤ t ≤ 4
1 out of 16
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.




