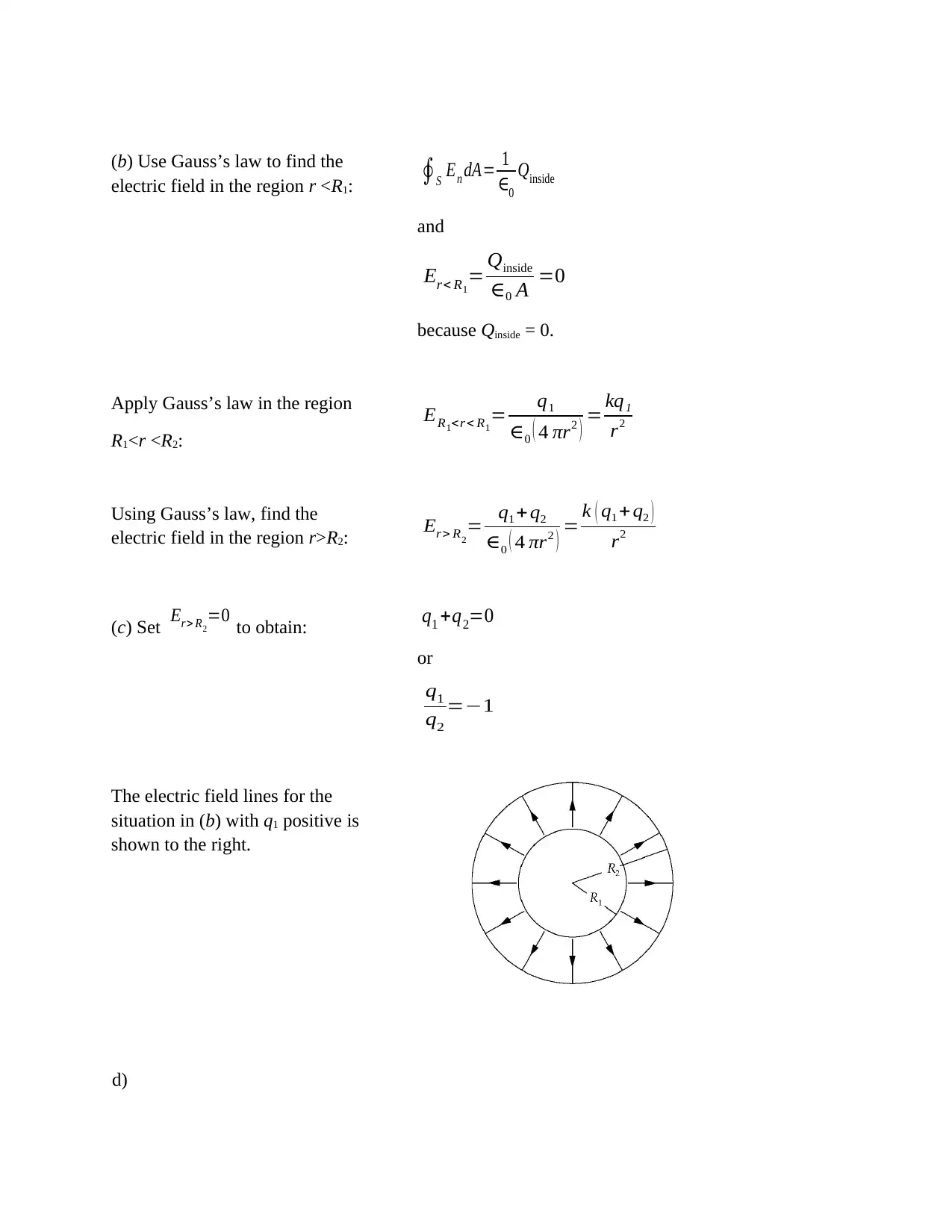
Solved Physics Assignment: Electric & Magnetic Fields & Gauss Law
VerifiedAdded on 2023/05/29
|12
|1466
|85
Homework Assignment
AI Summary
This document presents detailed solutions to various physics problems centered on electric and magnetic fields, along with applications of Gauss's law. The solutions cover scenarios involving calculating magnetic field contributions from current-carrying wires, determining electric fields due to charge distributions along arcs, and applying Gauss's law to find electric fields within and outside charged spheres and cylindrical shells. Each problem includes step-by-step derivations, integrations, and explanations to illustrate the underlying principles and methodologies. The solutions demonstrate the application of fundamental physics concepts to solve complex electromagnetic problems. Desklib offers a wealth of similar solved assignments and past papers to aid students in their studies.
1 out of 12