Dynamic Engineering Systems

This research paper discusses various topics in engineering science, including the calculation of energy expenditure, plotting graphs of kinetic energy, calculating the coefficient of friction, and more.

Dynamic Engineering Systems 1
DYNAMIC ENGINEERING SYSTEMS
A Research Paper on Engineering Science By
Student’s Name
Name of the Professor
Institutional Affiliation
City/State
Year/Month/Day

Dynamic Engineering Systems 2
A horizontal force of 80 N acts on a mass of 6 kg resting on a horizontal surface. The mass is
initially at rest and covers a distance of 5 m in 0.92 s under the action of the force. Assuming
there are no energy losses due to air resistance and therefore that the acceleration is constant:
(a) Calculate the total energy expended in the acceleration.
Force applied, F = 80N
Mass of box, m = 6kg
Distance covered, d = 5m
Time, t = 0.92s
Initial velocity of the box, u = 0m/s
Total energy expenditure = Work done x Distance
E = F x d
E = 80 x 5
= 400J
(b) Plot a graph of the kinetic energy of the mass against time.
Since acceleration, a = am/s2
Equation of motion; d = ut + 1
2 at2
Will be reduced to: 5 = 0 + 1
2 a (0.92)2
Therefore, a = 11.81m/s2
Velocity, v = u + at

Dynamic Engineering Systems 3
V = 0 + (11.81)t
= 11.81t
Therefore, KE of box = 1
2 MV2
= 1
2x 6 x (11.81t)2
= 418.43t2
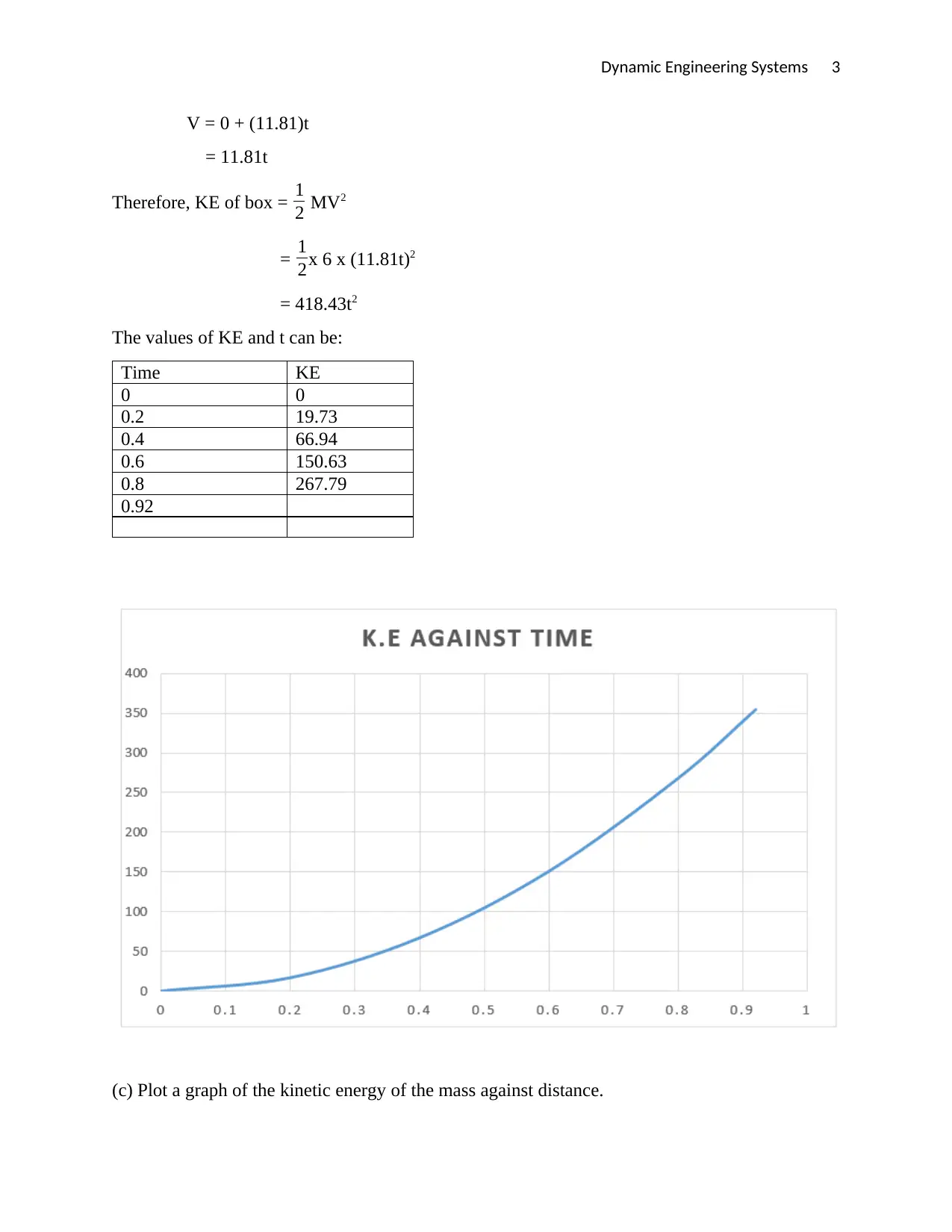
The values of KE and t can be:
Time KE
0 0
0.2 19.73
0.4 66.94
0.6 150.63
0.8 267.79
0.92
(c) Plot a graph of the kinetic energy of the mass against distance.

Dynamic Engineering Systems 4
Let s be distance covered after time t
V2 – U2 = 2as
V2 – (0)2 = 2 x 11.81 x s
V2 = 23.62S
KE = 1
2MV2
= 1
2 x 6 x 23.62 x S
= 70.86s
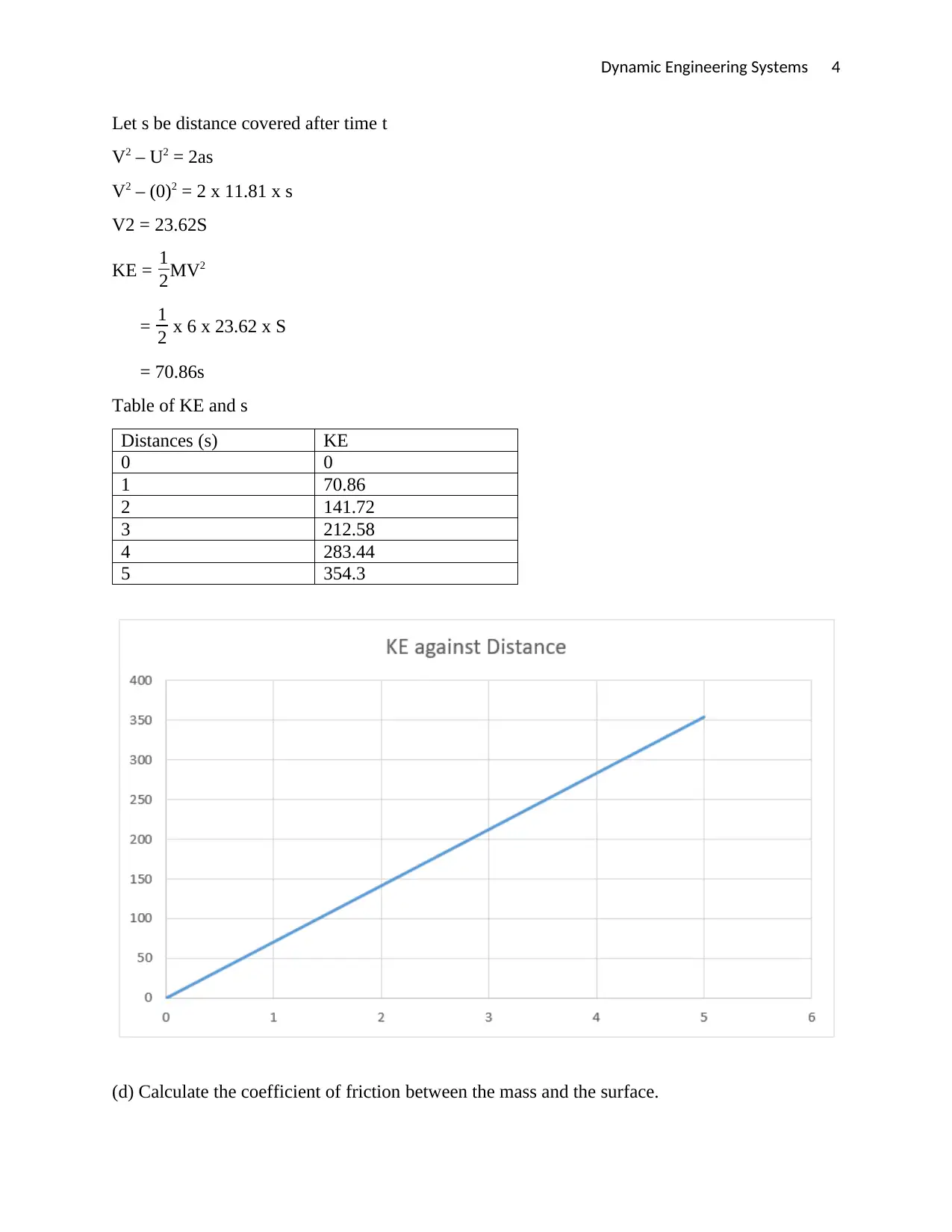
Table of KE and s
Distances (s) KE
0 0
1 70.86
2 141.72
3 212.58
4 283.44
5 354.3
(d) Calculate the coefficient of friction between the mass and the surface.

Dynamic Engineering Systems 5
Net Force, FN = ma
= 6 x 11.81
= 70.86N
Frictional force = F – FN
= 80 – 70.86
= 9.14N
Normal Reaction, R = mg = 6 x 9.81
R = 58.8N
Coefficient of friction, μ;
F = μR
μ = F/R = 9.14/58.8 = 0.155

Dynamic Engineering Systems 6
2. A mass of 0.5 kg is suspended from a flywheel as shown in FIGURE 2. If the mass is released
from rest and falls a distance of 0.5 m in 1.5 s, calculate:
(a) The linear acceleration of the mass.
Mass of wheel, Mw = 3kg
Radius = 0.3m
Radius of gyration = 0.212m
From equation: s = ut + 1
2 at2
U=0
S=0.5m
T=1.5s
0.5 = 1
2 x a x (1.5)2; a = 1
2.25
Linear acceleration, a = 0.44 m/s2
(b) The angular acceleration of the wheel.
Angular acceleration, α
T net = I α
m rꝭ o = mw k2 α
0.5 x 9.81 x 0.3 = 3 x (0.212)2 α
α = 10.9rad/s

Dynamic Engineering Systems 7
(c) The tension in the rope.
F net = ma
Mg – T = ma
0.5 x 9.81 – T = 0.5 x 0.44
T = 4.68 N
(d) The frictional torque, resisting motion.
T = mgro
T = 0.5 x 9.81 x 0.3
= 1.47N

Dynamic Engineering Systems 8
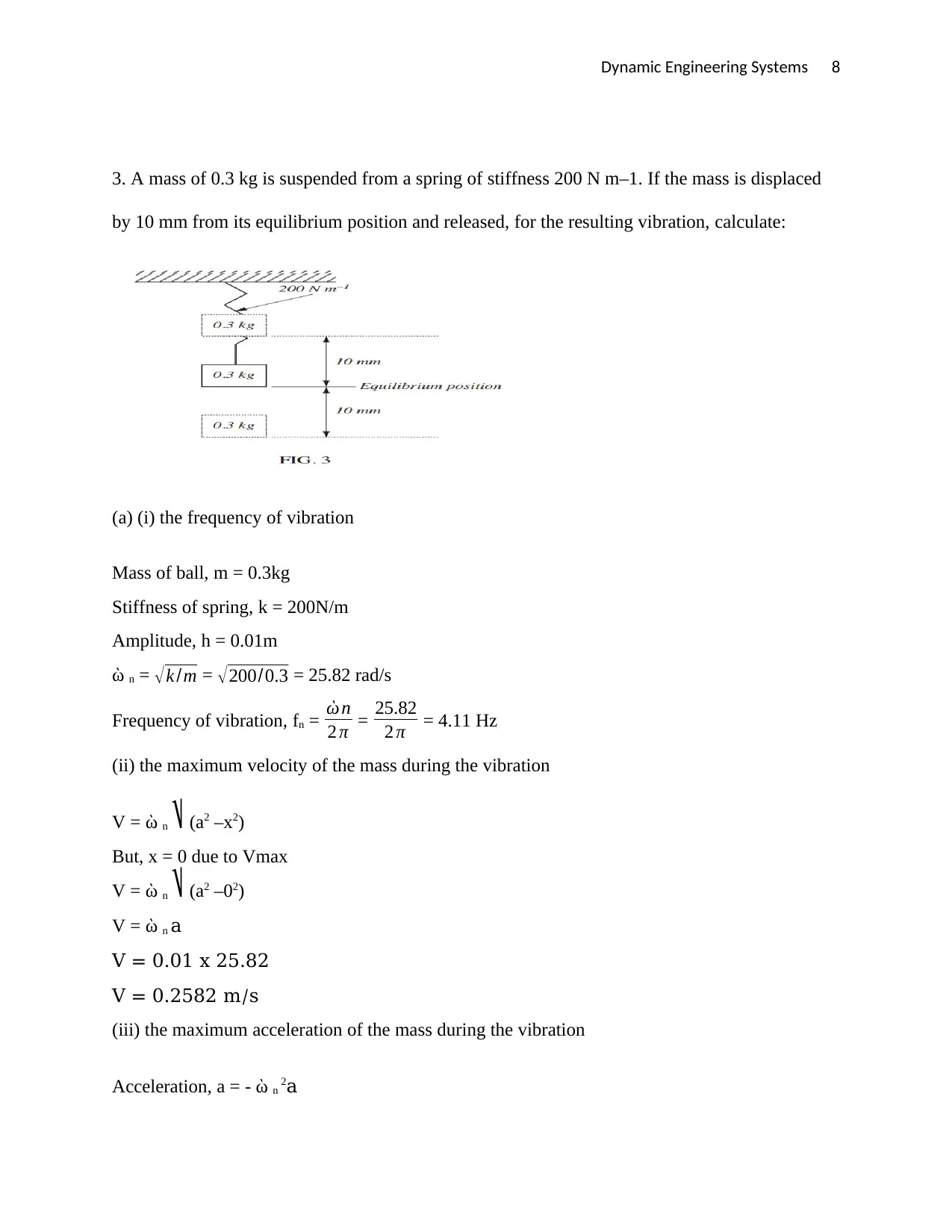
3. A mass of 0.3 kg is suspended from a spring of stiffness 200 N m–1. If the mass is displaced
by 10 mm from its equilibrium position and released, for the resulting vibration, calculate:
(a) (i) the frequency of vibration
Mass of ball, m = 0.3kg
Stiffness of spring, k = 200N/m
Amplitude, h = 0.01m
ὼ n = √ k /m = √200/0.3 = 25.82 rad/s
Frequency of vibration, fn = ὼ n
2 π = 25.82
2 π = 4.11 Hz
(ii) the maximum velocity of the mass during the vibration
V = ὼ n ⎷ (a2 –x2)
But, x = 0 due to Vmax
V = ὼ n ⎷ (a2 –02)
V = ὼ n a
V = 0.01 x 25.82
V = 0.2582 m/s
(iii) the maximum acceleration of the mass during the vibration
Acceleration, a = - ὼ n 2a

Dynamic Engineering Systems 9
When a max, x=a
amax = ὼ 2a
a = 25.82 x 0.01
a = 6.67 m/s2
(iv) the mass required to produce double the maximum velocity calculated in (ii) using the same
spring and initial deflection.
V = 2 x Vmax
V = 2 x 0.2582
V = 0.5164
V = a ὼ = a ⎷ k
m
M = ka 2
V 2 = 200 x 0.012/0.51642
M = 0.07499kg
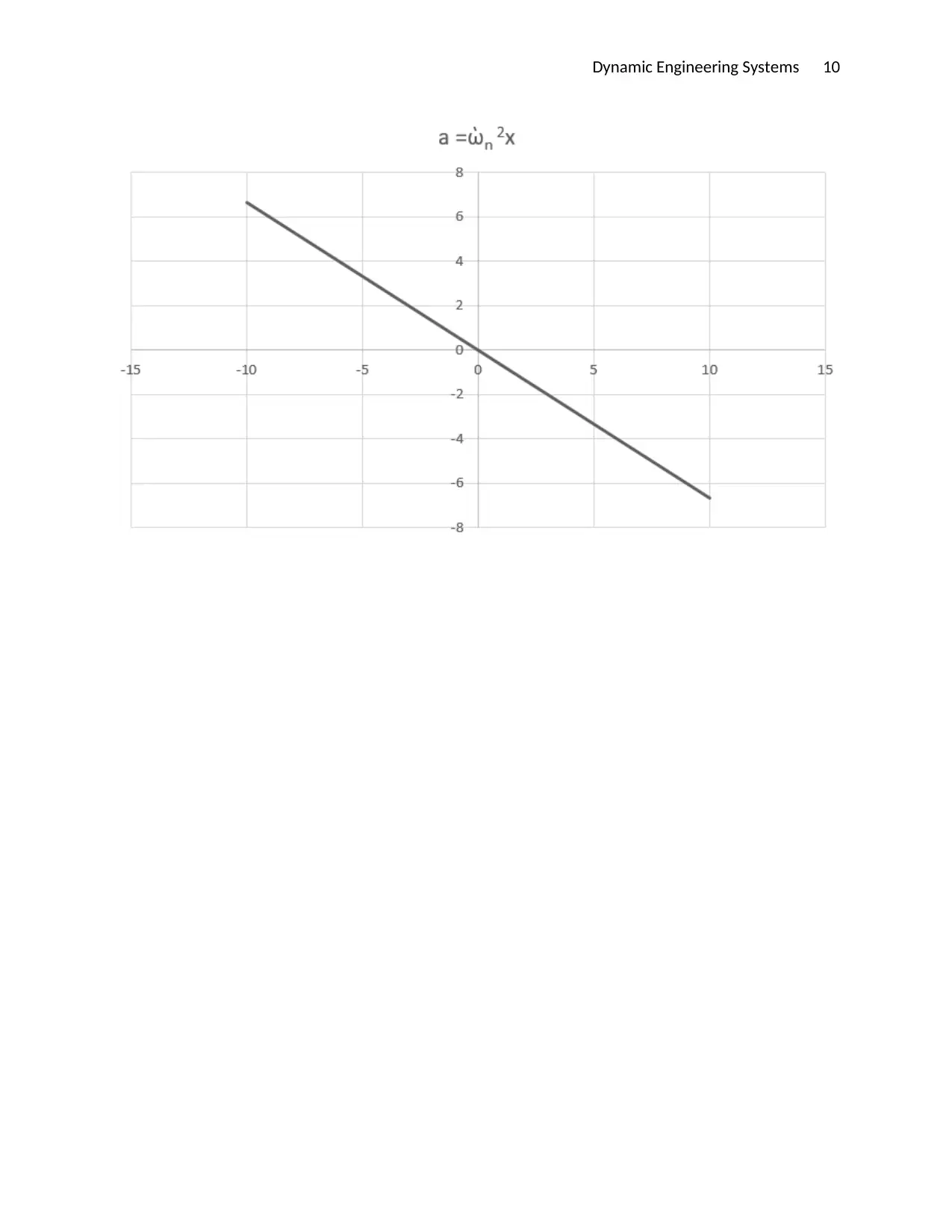
(b) Plot a graph of acceleration against displacement (x) (for values of x
from x = –10 mm to x = +10 mm)
For x = 10, a = -6.67m/s2
Fox x = -10, a = 6.67m/s2

Dynamic Engineering Systems 10

Dynamic Engineering Systems 11
BIBLIOGRAPHY
Brown, F., 2010. Engineering System Dynamics: A Unified Graph-Centered Approach, Second Edition.
Toledo: CRC Press.
Leonides, C., 2012. Control and Dynamic Systems V57: Multidisciplinary Engineering Systems: Design and
Optimization Techniques and Their Application: Advances in Theory and Applications. London: Academic
Press.
Woods, R. & Lawrence, K., 2011. Modeling and simulation of dynamic systems. New York: Prentice Hall.