Electrical Power 10 Aerodynamics Compressible Flow Assignment
VerifiedAdded on 2022/11/13
|29
|2945
|489
Homework Assignment
AI Summary
This assignment solution delves into advanced aerodynamics, specifically focusing on compressible flow principles. It explores the behavior of fluids in convergent-divergent nozzles, analyzing stagnation and static temperatures, and the conditions for choked flow. The solution includes detailed c...

Electrical power 1
AERODYNAMICS
Authors Name/s per 1st Affiliation (Author)
Dept. name of the organization
Name of organization, acronyms acceptable
City, Country
mail address
Authors Name/s per 2nd Affiliation (Author)
Dept. name of the organization
Name of organization, acronyms acceptable
City, Country
e-mail address
AERODYNAMICS
Authors Name/s per 1st Affiliation (Author)
Dept. name of the organization
Name of organization, acronyms acceptable
City, Country
mail address
Authors Name/s per 2nd Affiliation (Author)
Dept. name of the organization
Name of organization, acronyms acceptable
City, Country
e-mail address
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 2
SECTION 1 – CONVERGENT-DIVERGENT NOZZLES
Question 1.1
Explain the difference between stagnation temperature and static temperature.
[Stagnation temperature is literature reservoir temperature for the stream fluid which is being
measured. In most cases it measured by the help of thermal probe. While the static temperature is that
which is measured through a help of a sensor moving at the same speed of the fluid. In some cases it is
referred to as temperature without velocity effects].
Question 1.2
Under what conditions will the flow through a convergent-divergent nozzle become choked? What
happens to the mass flow rate when exit pressure is decreased on a choked nozzle?
[The fluid flow in the nozzle is chocked due to continues reduction in a back pressure in order to extent
that more reduction is not capable to move the point far from the throat. The mass flow rate will
decrease as the pressure at the exit decrease.]
Question 1.3
If a supersonic flow is run through a convergent duct will its velocity:
a) Decrease
b) Increase
c) Remain constant
d) It depends on the upstream Mach number
e) It depends on the upstream stagnation pressure and temperature.
[Increase]
Question 1.4
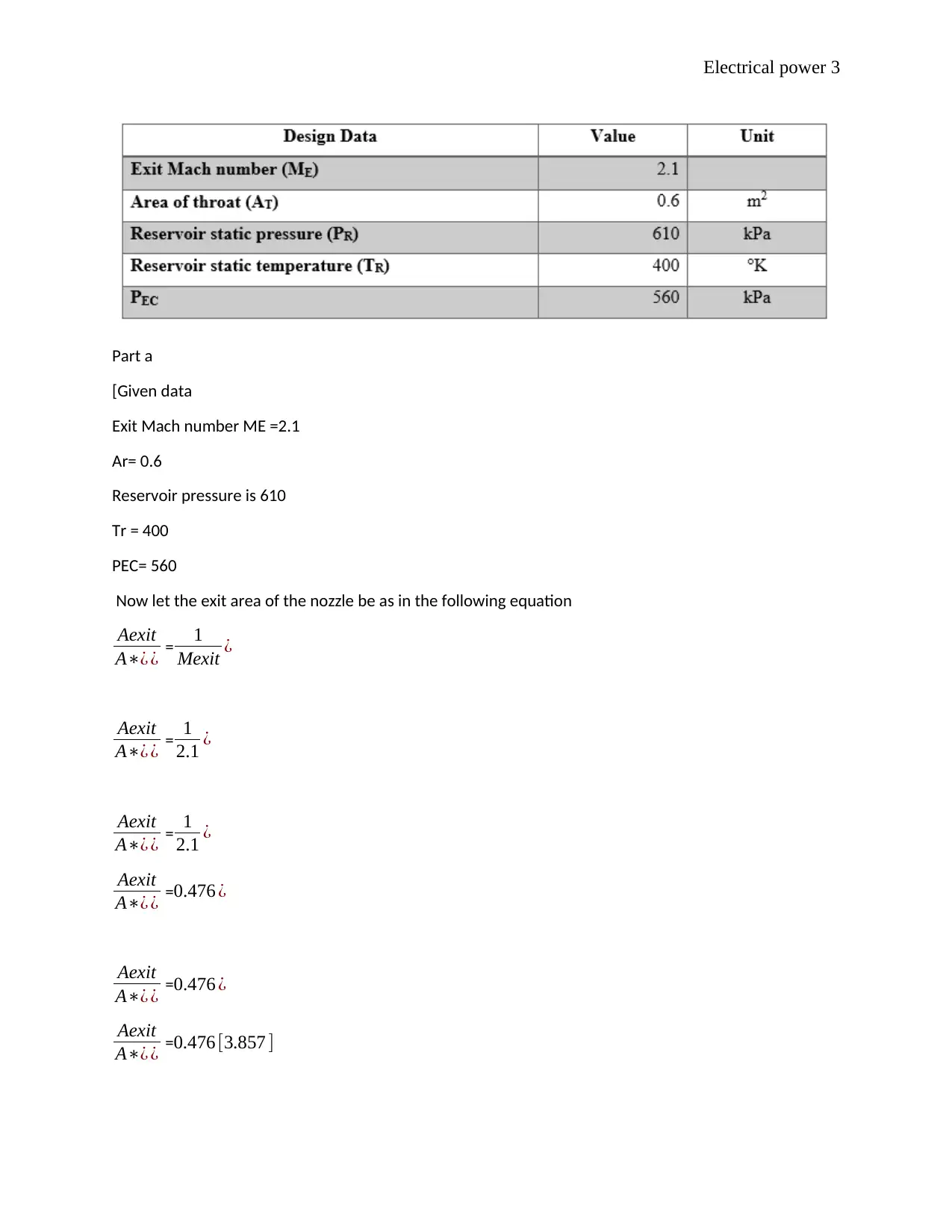
A convergent-divergent nozzle is designed to operate with isentropic flow with an exit Mach number,
ME. The flow in the nozzle is supplied from a reservoir of air with a static pressure of PR and a static
temperature of TR and the nozzle has a throat area, AT, as specified in the table below.
SECTION 1 – CONVERGENT-DIVERGENT NOZZLES
Question 1.1
Explain the difference between stagnation temperature and static temperature.
[Stagnation temperature is literature reservoir temperature for the stream fluid which is being
measured. In most cases it measured by the help of thermal probe. While the static temperature is that
which is measured through a help of a sensor moving at the same speed of the fluid. In some cases it is
referred to as temperature without velocity effects].
Question 1.2
Under what conditions will the flow through a convergent-divergent nozzle become choked? What
happens to the mass flow rate when exit pressure is decreased on a choked nozzle?
[The fluid flow in the nozzle is chocked due to continues reduction in a back pressure in order to extent
that more reduction is not capable to move the point far from the throat. The mass flow rate will
decrease as the pressure at the exit decrease.]
Question 1.3
If a supersonic flow is run through a convergent duct will its velocity:
a) Decrease
b) Increase
c) Remain constant
d) It depends on the upstream Mach number
e) It depends on the upstream stagnation pressure and temperature.
[Increase]
Question 1.4
A convergent-divergent nozzle is designed to operate with isentropic flow with an exit Mach number,
ME. The flow in the nozzle is supplied from a reservoir of air with a static pressure of PR and a static
temperature of TR and the nozzle has a throat area, AT, as specified in the table below.
Electrical power 3
Part a
[Given data
Exit Mach number ME =2.1
Ar= 0.6
Reservoir pressure is 610
Tr = 400
PEC= 560
Now let the exit area of the nozzle be as in the following equation
Aexit
A∗¿ ¿ = 1
Mexit ¿
Aexit
A∗¿ ¿ = 1
2.1 ¿
Aexit
A∗¿ ¿ = 1
2.1 ¿
Aexit
A∗¿ ¿ =0.476 ¿
Aexit
A∗¿ ¿ =0.476 ¿
Aexit
A∗¿ ¿ =0.476 [3.857 ]
Part a
[Given data
Exit Mach number ME =2.1
Ar= 0.6
Reservoir pressure is 610
Tr = 400
PEC= 560
Now let the exit area of the nozzle be as in the following equation
Aexit
A∗¿ ¿ = 1
Mexit ¿
Aexit
A∗¿ ¿ = 1
2.1 ¿
Aexit
A∗¿ ¿ = 1
2.1 ¿
Aexit
A∗¿ ¿ =0.476 ¿
Aexit
A∗¿ ¿ =0.476 ¿
Aexit
A∗¿ ¿ =0.476 [3.857 ]
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 4
Aexit
A∗¿ ¿ = 1.726
Part b
Now we calculate the exit area of the nozzle .
Area of throat is AT= 0.6
From Aexit
A∗¿ ¿ = 1.726
Aexit = A* × 1.726
A* = 0.6
Aexit = 0.6 × 1.726
Aexit = 1.03556 m2
Part c
Now we obtain the exit pressure at which the nozzle will become chocked
This is obtained by the use of the following equation
P∗¿
Po ¿=( 2
r +2 ¿
r
r −1
Stagnation pressure / Reseivor static pressure i.e
P∗¿
Po ¿=( 2
3.4 ¿
1.4
1.4−1
P∗¿
Po ¿= 0.15610
P* = 0.15610×610
P* = 95.221 kPa
Part d
The mass flow rate for the exit pressure of 0kpa is
Pexit = 0
So there will be no mass flow rate
M = 0kg/sec
Aexit
A∗¿ ¿ = 1.726
Part b
Now we calculate the exit area of the nozzle .
Area of throat is AT= 0.6
From Aexit
A∗¿ ¿ = 1.726
Aexit = A* × 1.726
A* = 0.6
Aexit = 0.6 × 1.726
Aexit = 1.03556 m2
Part c
Now we obtain the exit pressure at which the nozzle will become chocked
This is obtained by the use of the following equation
P∗¿
Po ¿=( 2
r +2 ¿
r
r −1
Stagnation pressure / Reseivor static pressure i.e
P∗¿
Po ¿=( 2
3.4 ¿
1.4
1.4−1
P∗¿
Po ¿= 0.15610
P* = 0.15610×610
P* = 95.221 kPa
Part d
The mass flow rate for the exit pressure of 0kpa is
Pexit = 0
So there will be no mass flow rate
M = 0kg/sec
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Electrical power 5
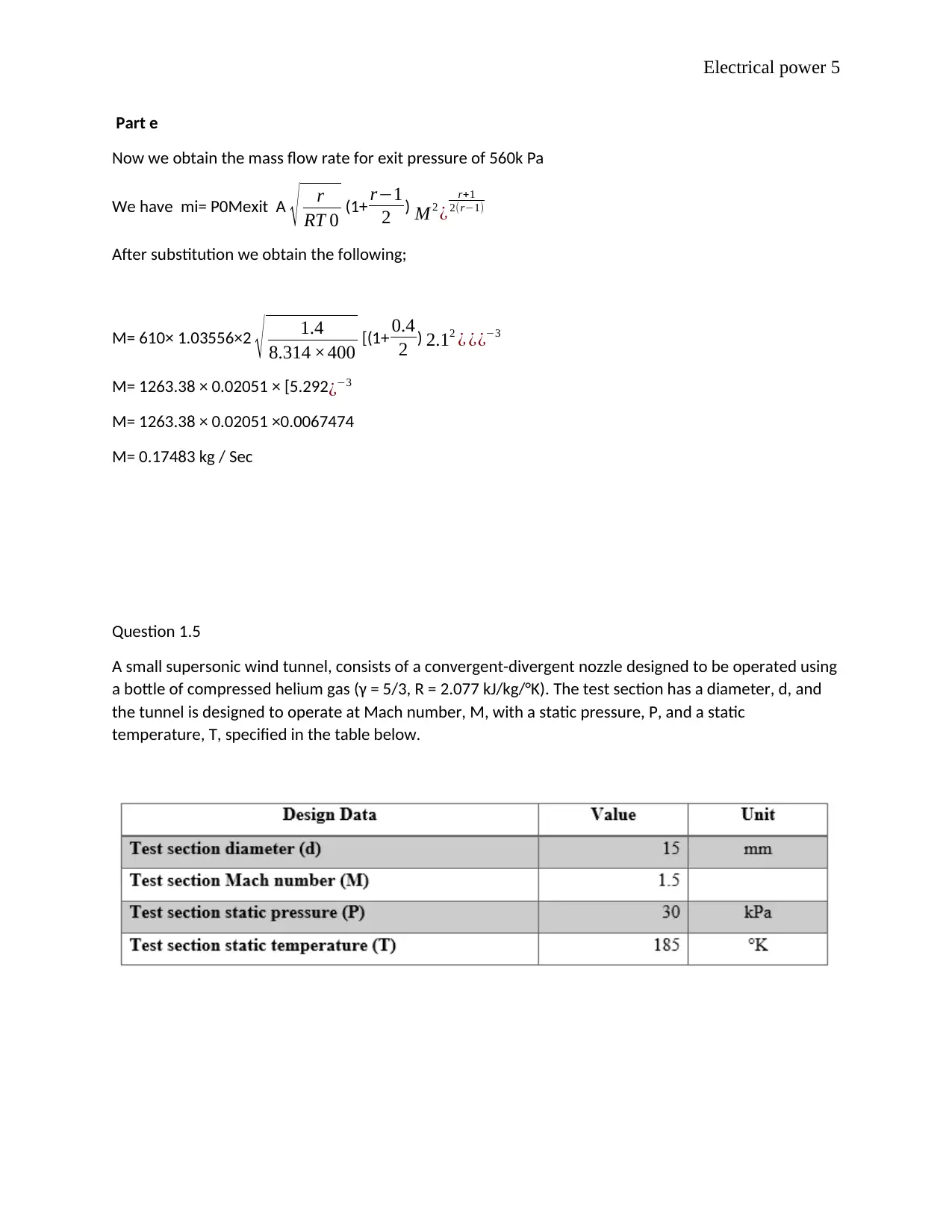
Part e
Now we obtain the mass flow rate for exit pressure of 560k Pa
We have mi= P0Mexit A √ r
RT 0 (1+ r−1
2 ) M 2 ¿
r+1
2(r−1)
After substitution we obtain the following;
M= 610× 1.03556×2 √ 1.4
8.314 ×400 [(1+ 0.4
2 ) 2.12 ¿ ¿¿−3
M= 1263.38 × 0.02051 × [5.292 ¿−3
M= 1263.38 × 0.02051 ×0.0067474
M= 0.17483 kg / Sec
Question 1.5
A small supersonic wind tunnel, consists of a convergent-divergent nozzle designed to be operated using
a bottle of compressed helium gas (γ = 5/3, R = 2.077 kJ/kg/°K). The test section has a diameter, d, and
the tunnel is designed to operate at Mach number, M, with a static pressure, P, and a static
temperature, T, specified in the table below.
Part e
Now we obtain the mass flow rate for exit pressure of 560k Pa
We have mi= P0Mexit A √ r
RT 0 (1+ r−1
2 ) M 2 ¿
r+1
2(r−1)
After substitution we obtain the following;
M= 610× 1.03556×2 √ 1.4
8.314 ×400 [(1+ 0.4
2 ) 2.12 ¿ ¿¿−3
M= 1263.38 × 0.02051 × [5.292 ¿−3
M= 1263.38 × 0.02051 ×0.0067474
M= 0.17483 kg / Sec
Question 1.5
A small supersonic wind tunnel, consists of a convergent-divergent nozzle designed to be operated using
a bottle of compressed helium gas (γ = 5/3, R = 2.077 kJ/kg/°K). The test section has a diameter, d, and
the tunnel is designed to operate at Mach number, M, with a static pressure, P, and a static
temperature, T, specified in the table below.

Electrical power 6
T 0
T = 1+ ( r−1
2 ¿ m2
P 0
P = [1+( r−1
2 ¿ m2 ¿
r
r−1
ρ 0
ρ = [1++( r−1
2 ¿ m2 ¿
r
r−1
At the given dimension
⍴= ρ
RT = 30× 1000
2077 ×185 = 30000
384245 = 0.0780kg/m3
Part a
m=⍴ AV = ⍴ ( π d2
4 )m√rRT
m= 0.0780 ( π 152 10−6
4 ) 1.5 √ 5
3 ×2.077 ×100017.5
m= 1.61
Part b
Area = A/At = 1/m ( 2+(r −1)( m2 )
r +1 ) r +1
2(r−1)
π ( 152 )
4
π ( d t2 )
4
= 1
1.5 ( 2+ ( 1.667−1 ) (1. 52 )
1.6667+1 ¿
1.6667+ 1
1.6667−1¿ ¿
152
d t2 = 1.1483 or simply
dt= 13.997≅ 14 mm
T 0
T = 1+ ( r−1
2 ¿ m2
P 0
P = [1+( r−1
2 ¿ m2 ¿
r
r−1
ρ 0
ρ = [1++( r−1
2 ¿ m2 ¿
r
r−1
At the given dimension
⍴= ρ
RT = 30× 1000
2077 ×185 = 30000
384245 = 0.0780kg/m3
Part a
m=⍴ AV = ⍴ ( π d2
4 )m√rRT
m= 0.0780 ( π 152 10−6
4 ) 1.5 √ 5
3 ×2.077 ×100017.5
m= 1.61
Part b
Area = A/At = 1/m ( 2+(r −1)( m2 )
r +1 ) r +1
2(r−1)
π ( 152 )
4
π ( d t2 )
4
= 1
1.5 ( 2+ ( 1.667−1 ) (1. 52 )
1.6667+1 ¿
1.6667+ 1
1.6667−1¿ ¿
152
d t2 = 1.1483 or simply
dt= 13.997≅ 14 mm
You're viewing a preview
Unlock full access by subscribing today!
Electrical power 7
Part C
T0= 185 (1+ m2
3 )
T0 = 185 (1+ 1.52
3 )
T0= 323.75 K
Part d
Pressure P 0 = 30 (1+ 1.52
3 ¿2.5
Pressure = 121.54kPa
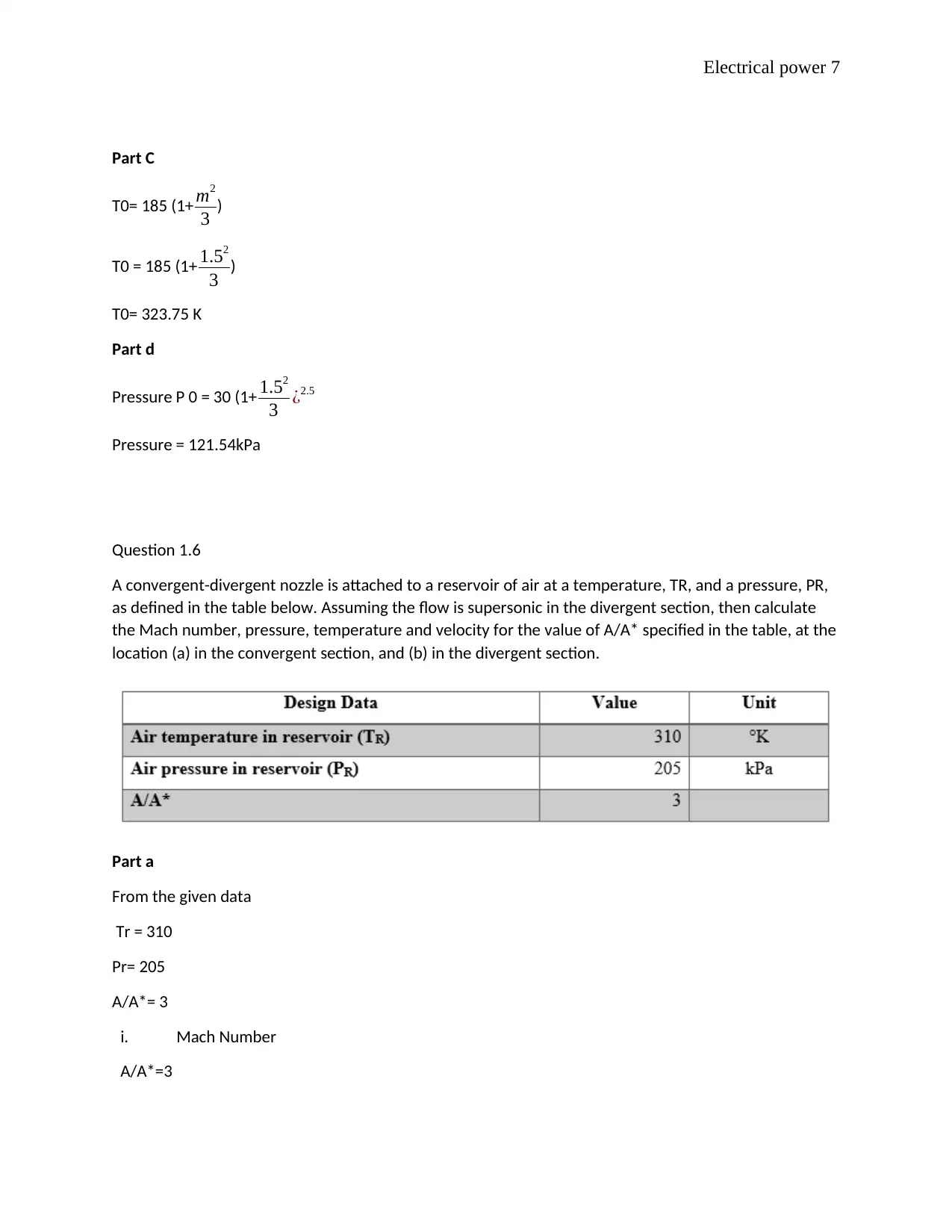
Question 1.6
A convergent-divergent nozzle is attached to a reservoir of air at a temperature, TR, and a pressure, PR,
as defined in the table below. Assuming the flow is supersonic in the divergent section, then calculate
the Mach number, pressure, temperature and velocity for the value of A/A* specified in the table, at the
location (a) in the convergent section, and (b) in the divergent section.
Part a
From the given data
Tr = 310
Pr= 205
A/A*= 3
i. Mach Number
A/A*=3
Part C
T0= 185 (1+ m2
3 )
T0 = 185 (1+ 1.52
3 )
T0= 323.75 K
Part d
Pressure P 0 = 30 (1+ 1.52
3 ¿2.5
Pressure = 121.54kPa
Question 1.6
A convergent-divergent nozzle is attached to a reservoir of air at a temperature, TR, and a pressure, PR,
as defined in the table below. Assuming the flow is supersonic in the divergent section, then calculate
the Mach number, pressure, temperature and velocity for the value of A/A* specified in the table, at the
location (a) in the convergent section, and (b) in the divergent section.
Part a
From the given data
Tr = 310
Pr= 205
A/A*= 3
i. Mach Number
A/A*=3
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 8
For the relation A/A* = 1
m [( 2
k +1 ¿ ¿
The value of k is assumed to be 1.4
3= 1
m [( 2
1.4+1 ¿ ¿
3= 1
m [(0.833 ¿ ¿
31/3= 1
m
1/3 [(0.833+0.16667 m2 ¿31/3
1.444m1/3 = [(0.833+0.16667 m2 ¿
1.444m1/3 -0.16667 m2= 0.833
M= 2.625
ii.
For A/A*=3
Thus P
P 0 = 21.557 ( From the table)
P= 21.557 ×P0
P= 21.557 ×205
P= 4419.185 kPa
iii.
For A/A*=3
T
T 0 = 2.4045 (from the table )
T=2.4045 × T0
T=2.4045 × 310
T= 745.395K
iv.
Velocity V1 = M x velocity of sound at 310 K
For the relation A/A* = 1
m [( 2
k +1 ¿ ¿
The value of k is assumed to be 1.4
3= 1
m [( 2
1.4+1 ¿ ¿
3= 1
m [(0.833 ¿ ¿
31/3= 1
m
1/3 [(0.833+0.16667 m2 ¿31/3
1.444m1/3 = [(0.833+0.16667 m2 ¿
1.444m1/3 -0.16667 m2= 0.833
M= 2.625
ii.
For A/A*=3
Thus P
P 0 = 21.557 ( From the table)
P= 21.557 ×P0
P= 21.557 ×205
P= 4419.185 kPa
iii.
For A/A*=3
T
T 0 = 2.4045 (from the table )
T=2.4045 × T0
T=2.4045 × 310
T= 745.395K
iv.
Velocity V1 = M x velocity of sound at 310 K

Electrical power 9
(1.27)×√ rRT
V1= 2.625 √1.4 ×287310
V1=2.625 √ 1124558
V1=2.625 ×352.92
V1= 926.41 m/s
Part b
Divergent part
For a flow to be super sank at all divergent part maximum mach number that it can realize at a flow
which is equal to 1.
i. Mach number M2 = M 12 (k +1)
2(k −1) M 12
M1 = 2.625
16.53
5.5125 = M2
M2= 2.998
M2≈ 3
ii.
Now P
Pdiv = ( 1+ k−1
2 )M2
P
Pdiv = 1+( 1+ 0.4
2 ) 9
P
Pdiv = 11.8
Pdiv = 11.8
2.392
Pdiv = 4.933 kPa
iii.
M= 3
Tdiv
Tinlet =0.417
(1.27)×√ rRT
V1= 2.625 √1.4 ×287310
V1=2.625 √ 1124558
V1=2.625 ×352.92
V1= 926.41 m/s
Part b
Divergent part
For a flow to be super sank at all divergent part maximum mach number that it can realize at a flow
which is equal to 1.
i. Mach number M2 = M 12 (k +1)
2(k −1) M 12
M1 = 2.625
16.53
5.5125 = M2
M2= 2.998
M2≈ 3
ii.
Now P
Pdiv = ( 1+ k−1
2 )M2
P
Pdiv = 1+( 1+ 0.4
2 ) 9
P
Pdiv = 11.8
Pdiv = 11.8
2.392
Pdiv = 4.933 kPa
iii.
M= 3
Tdiv
Tinlet =0.417
You're viewing a preview
Unlock full access by subscribing today!
Electrical power 10
Tdiv = 0.417×745.395
Tdiv= 310.8 K
iv.
Velocity = 2.625 √ 1.4 ×287 × 310
Velocity 2.625 √124558
V= 926.43 m/s
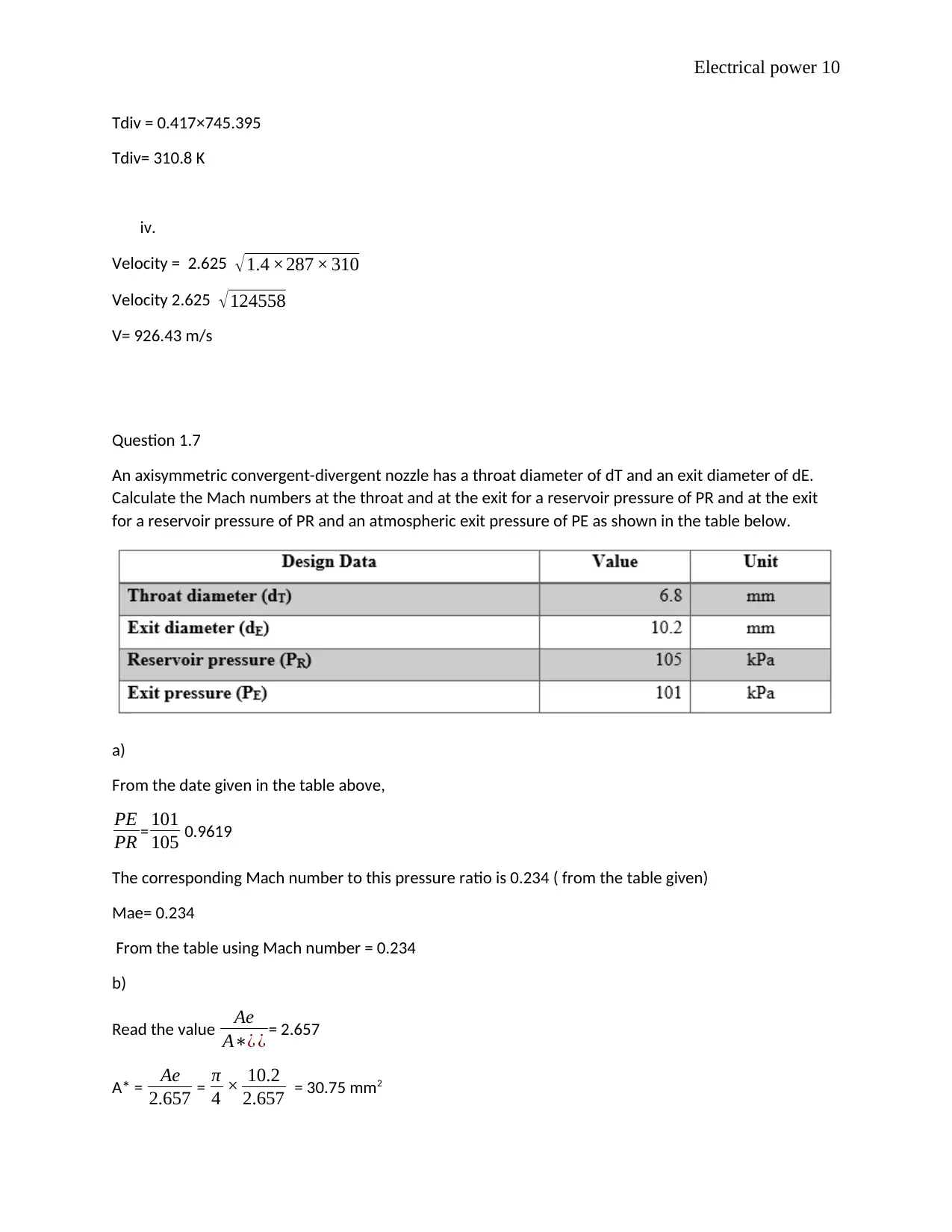
Question 1.7
An axisymmetric convergent-divergent nozzle has a throat diameter of dT and an exit diameter of dE.
Calculate the Mach numbers at the throat and at the exit for a reservoir pressure of PR and at the exit
for a reservoir pressure of PR and an atmospheric exit pressure of PE as shown in the table below.
a)
From the date given in the table above,
PE
PR = 101
105 0.9619
The corresponding Mach number to this pressure ratio is 0.234 ( from the table given)
Mae= 0.234
From the table using Mach number = 0.234
b)
Read the value Ae
A∗¿ ¿ = 2.657
A* = Ae
2.657 = π
4 × 10.2
2.657 = 30.75 mm2
Tdiv = 0.417×745.395
Tdiv= 310.8 K
iv.
Velocity = 2.625 √ 1.4 ×287 × 310
Velocity 2.625 √124558
V= 926.43 m/s
Question 1.7
An axisymmetric convergent-divergent nozzle has a throat diameter of dT and an exit diameter of dE.
Calculate the Mach numbers at the throat and at the exit for a reservoir pressure of PR and at the exit
for a reservoir pressure of PR and an atmospheric exit pressure of PE as shown in the table below.
a)
From the date given in the table above,
PE
PR = 101
105 0.9619
The corresponding Mach number to this pressure ratio is 0.234 ( from the table given)
Mae= 0.234
From the table using Mach number = 0.234
b)
Read the value Ae
A∗¿ ¿ = 2.657
A* = Ae
2.657 = π
4 × 10.2
2.657 = 30.75 mm2
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
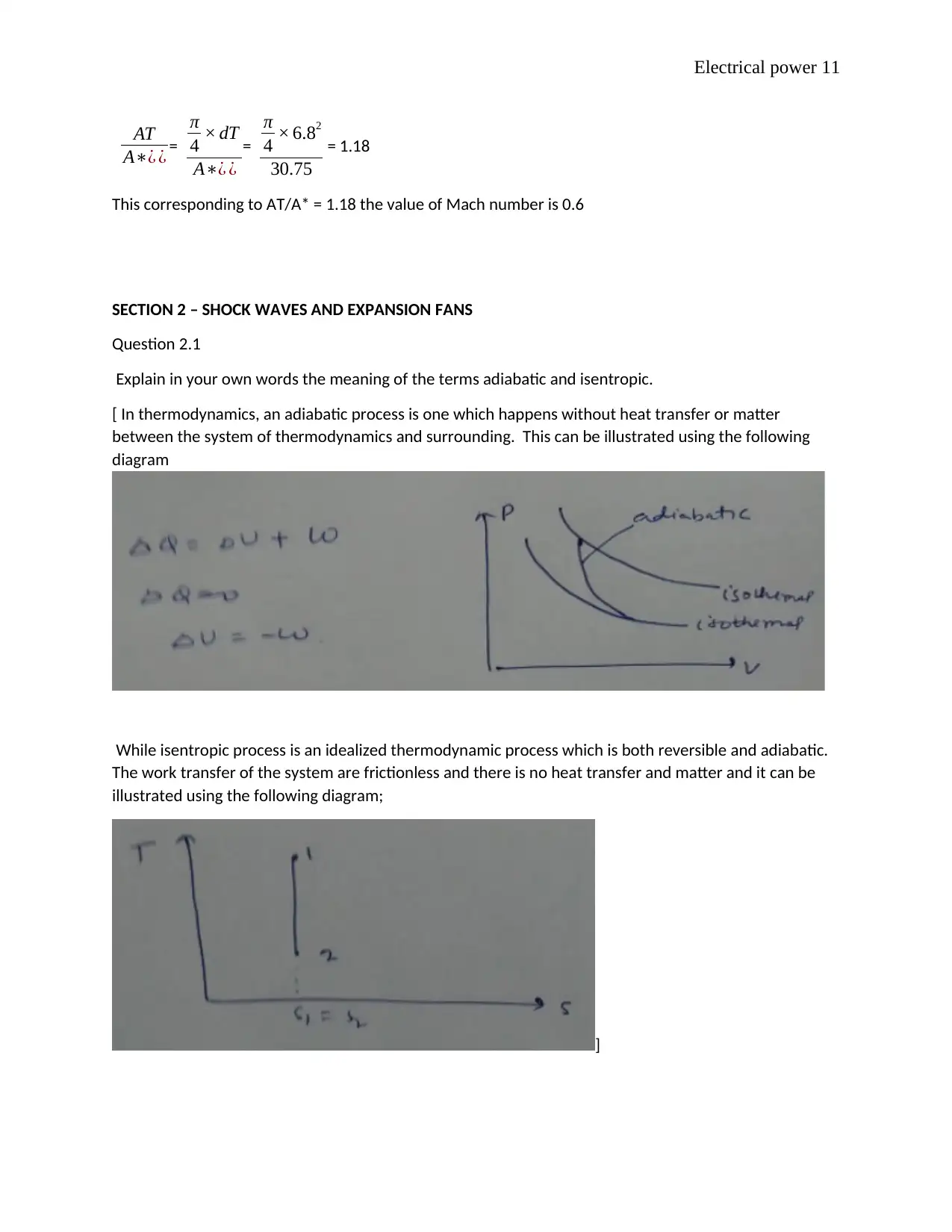
Electrical power 11
AT
A∗¿ ¿ =
π
4 × dT
A∗¿ ¿
=
π
4 × 6.82
30.75
= 1.18
This corresponding to AT/A* = 1.18 the value of Mach number is 0.6
SECTION 2 – SHOCK WAVES AND EXPANSION FANS
Question 2.1
Explain in your own words the meaning of the terms adiabatic and isentropic.
[ In thermodynamics, an adiabatic process is one which happens without heat transfer or matter
between the system of thermodynamics and surrounding. This can be illustrated using the following
diagram
While isentropic process is an idealized thermodynamic process which is both reversible and adiabatic.
The work transfer of the system are frictionless and there is no heat transfer and matter and it can be
illustrated using the following diagram;
]
AT
A∗¿ ¿ =
π
4 × dT
A∗¿ ¿
=
π
4 × 6.82
30.75
= 1.18
This corresponding to AT/A* = 1.18 the value of Mach number is 0.6
SECTION 2 – SHOCK WAVES AND EXPANSION FANS
Question 2.1
Explain in your own words the meaning of the terms adiabatic and isentropic.
[ In thermodynamics, an adiabatic process is one which happens without heat transfer or matter
between the system of thermodynamics and surrounding. This can be illustrated using the following
diagram
While isentropic process is an idealized thermodynamic process which is both reversible and adiabatic.
The work transfer of the system are frictionless and there is no heat transfer and matter and it can be
illustrated using the following diagram;
]
Electrical power 12
Question 2.2
Which of the following is true of a normal shock?
a) Downstream M will depend on upstream M, p, and T.
b) Downstream M will depend only on upstream p and T.
c) Downstream p and T will depend only on upstream M.
d) Downstream T will depend only on upstream T.
e) None of the above
[Downstream p and T will depend only on upstream M.]
Question 2.3
Which of the following is true of flow across an expansion wave?
a) p0 and T0 will both increase.
b) p0 will increase while T0 will decrease.
c) p0 will decrease while T0 will increase.
d) p0 and T0 will both decrease
e) p0 and T0 will both remain constant
[p0 and T0 will both decrease]
Question 2.4
Which of the following is true of a supersonic flow past a wedge?
[Increasing wedge angle will increase the chance of shock detaching]
Question 2.5
A normal shock is located inside the divergent section of a convergent-divergent nozzle at a location
where the ratio of duct area to throat area is specified in the table below. Given the exit area to throat
area ratio specified in the table below, calculate the following quantities.
From the data given in the table above;
i.
Question 2.2
Which of the following is true of a normal shock?
a) Downstream M will depend on upstream M, p, and T.
b) Downstream M will depend only on upstream p and T.
c) Downstream p and T will depend only on upstream M.
d) Downstream T will depend only on upstream T.
e) None of the above
[Downstream p and T will depend only on upstream M.]
Question 2.3
Which of the following is true of flow across an expansion wave?
a) p0 and T0 will both increase.
b) p0 will increase while T0 will decrease.
c) p0 will decrease while T0 will increase.
d) p0 and T0 will both decrease
e) p0 and T0 will both remain constant
[p0 and T0 will both decrease]
Question 2.4
Which of the following is true of a supersonic flow past a wedge?
[Increasing wedge angle will increase the chance of shock detaching]
Question 2.5
A normal shock is located inside the divergent section of a convergent-divergent nozzle at a location
where the ratio of duct area to throat area is specified in the table below. Given the exit area to throat
area ratio specified in the table below, calculate the following quantities.
From the data given in the table above;
i.
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 13
Shock occurs flow will be isentropic , therefore
Ao
Al = 3.09 for this Mo= 2. 6404
Therefore the Mach number just before Shock i.er upstream is 2. 6404
Part b
Mach number just downstream of the shock
My =
M r2 + 2
k−1
( 2 M r2 k
k−1 −1 ) =
√ 2.6404 +5
47.801 = 0.5004
Mach number downstream is hence 0.5004
Part c
Ratio of stagnation pressure after the shock to before the shock
PAft
Pbef = MAft
Mbef
=
[1+ Mbe f 2 ( k −1 )
2 ]
1+ Maf t2 ( k−1 )
2
¿
k+1
2(k−1)
Shock occurs flow will be isentropic , therefore
Ao
Al = 3.09 for this Mo= 2. 6404
Therefore the Mach number just before Shock i.er upstream is 2. 6404
Part b
Mach number just downstream of the shock
My =
M r2 + 2
k−1
( 2 M r2 k
k−1 −1 ) =
√ 2.6404 +5
47.801 = 0.5004
Mach number downstream is hence 0.5004
Part c
Ratio of stagnation pressure after the shock to before the shock
PAft
Pbef = MAft
Mbef
=
[1+ Mbe f 2 ( k −1 )
2 ]
1+ Maf t2 ( k−1 )
2
¿
k+1
2(k−1)
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 14
= 2.6404
0.5004 [ 1+ 0.500 42( 0.2)
1+2.640 42 ¿ ¿ ] = 0.4453
The ratio is hence = 0.4453
Part d
Aduct
Athrout = 3.09 ,
Aduct
P 0 = 0.0493
Similarly Aduct
Athrout = 5.15 ,
Pduct
P 0 = 0.0202
Pduct
P 0 × P 0
Pduct = 0.02
0.0493 = 0.4097
Pduct = 0.4097 × Pressure at downstream after shock
Therefore the ratio will be
1
0.0493 = 2.4405
Ratio = 2.4405
Part e
= 2.6404
0.5004 [ 1+ 0.500 42( 0.2)
1+2.640 42 ¿ ¿ ] = 0.4453
The ratio is hence = 0.4453
Part d
Aduct
Athrout = 3.09 ,
Aduct
P 0 = 0.0493
Similarly Aduct
Athrout = 5.15 ,
Pduct
P 0 = 0.0202
Pduct
P 0 × P 0
Pduct = 0.02
0.0493 = 0.4097
Pduct = 0.4097 × Pressure at downstream after shock
Therefore the ratio will be
1
0.0493 = 2.4405
Ratio = 2.4405
Part e
Electrical power 15
Ratio end pressure to reservoir pressure can be given by the following
= Pexit
P 0 = 0.0202 this has been calculated above
Ratio = 0.0202
Question 2.6
Air is flowing at supersonic speed over a two-dimensional wedge with an upstream static pressure, P,
and static temperature, T, which are defined in the table below.
From the data given in the table
For isentropic table mi=2.6
P1
P 01 =0.501
P01= 1965.1kPa
T 1
¿ 1 = 0.4252
T01= 625.23k
Part a
Wedged shocked half angle
Angle = 900
When θ= 900 normal shock will form detaching oblique shock
Ratio end pressure to reservoir pressure can be given by the following
= Pexit
P 0 = 0.0202 this has been calculated above
Ratio = 0.0202
Question 2.6
Air is flowing at supersonic speed over a two-dimensional wedge with an upstream static pressure, P,
and static temperature, T, which are defined in the table below.
From the data given in the table
For isentropic table mi=2.6
P1
P 01 =0.501
P01= 1965.1kPa
T 1
¿ 1 = 0.4252
T01= 625.23k
Part a
Wedged shocked half angle
Angle = 900
When θ= 900 normal shock will form detaching oblique shock
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 16
Part b
i. From oblique shock table
When n = 2.6 , θ= 200
Shock angles β= 41.620, m2= 1.72
ii.
Mach number upstream normal to wave
Min = m1sin β
Min = 2.6 sin 41.62 = 1.72688
iii. Mach number behind shock normal to it
M2n= m2 sin ( β ¿, m2 = 1.72688
M2n = 1.72688 sin 41.62
M2n = 1.146
Iv, Actual Mach nehind shock wave
This will be equal to m2
Therefore mach number behind shock wave = 1.7268
V, from neutral shock table at min = 1.72688
P 2
P 1 =3.325
P2 =3.325 × 104
P2= 345.8 kPa
Vi Static temperature
T 02
T 01 = 1.48
T02= 1.48×265
T02 = 392.2 K
Vii , Total pressure
P 02
P 01 = 0.8431
P02 = 0.8431× 104
Part b
i. From oblique shock table
When n = 2.6 , θ= 200
Shock angles β= 41.620, m2= 1.72
ii.
Mach number upstream normal to wave
Min = m1sin β
Min = 2.6 sin 41.62 = 1.72688
iii. Mach number behind shock normal to it
M2n= m2 sin ( β ¿, m2 = 1.72688
M2n = 1.72688 sin 41.62
M2n = 1.146
Iv, Actual Mach nehind shock wave
This will be equal to m2
Therefore mach number behind shock wave = 1.7268
V, from neutral shock table at min = 1.72688
P 2
P 1 =3.325
P2 =3.325 × 104
P2= 345.8 kPa
Vi Static temperature
T 02
T 01 = 1.48
T02= 1.48×265
T02 = 392.2 K
Vii , Total pressure
P 02
P 01 = 0.8431
P02 = 0.8431× 104
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 17
P02 = 87.68 kPa
Viii total temperature behind the shock
For this case T01 = T02
And being that T02 = 392.2 K
Therefore T01 = 392.2 K
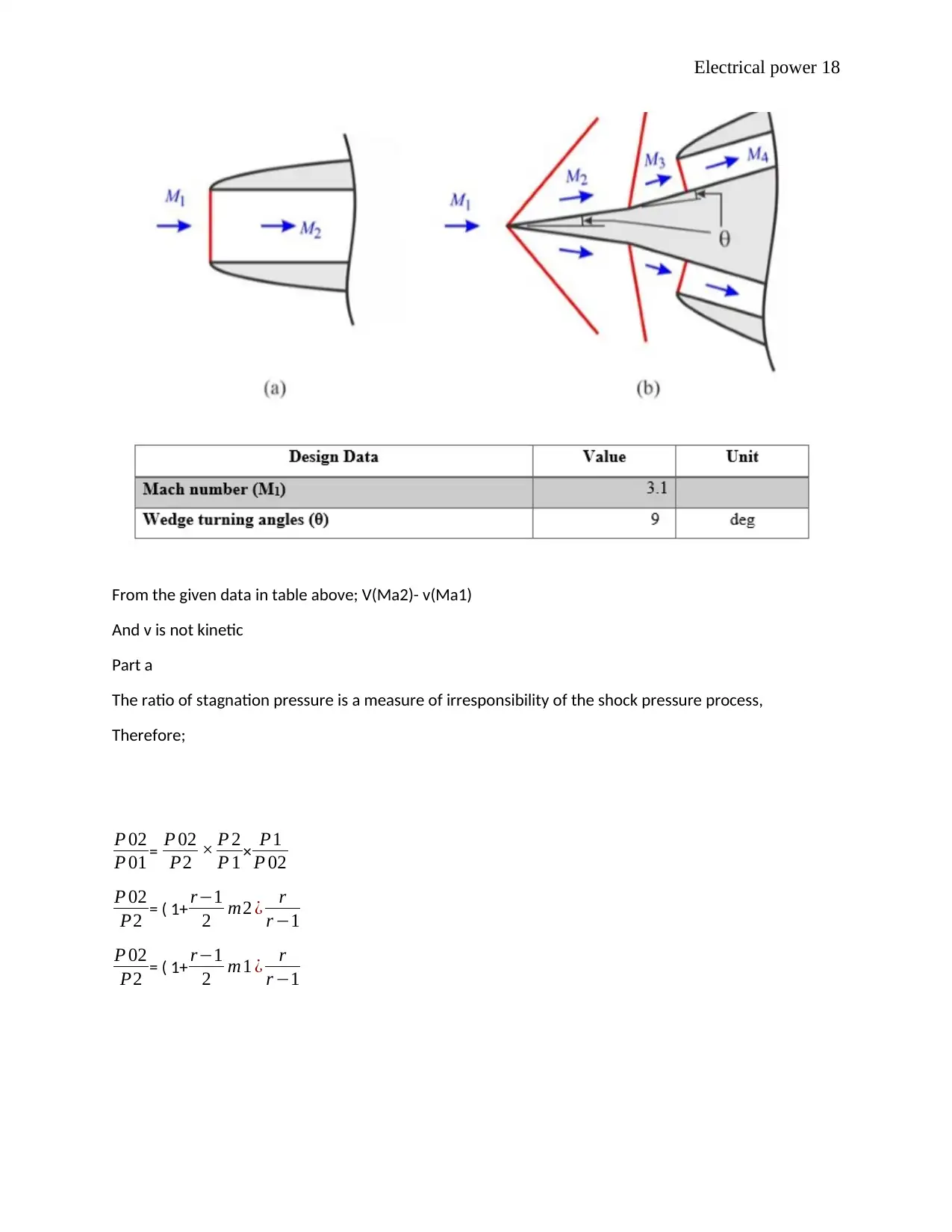
Question 2.7
Two possibilities are considered for the design of a two-dimensional jet engine inlet as shown in the
figures (a-b) below. The inlet is to operate at Mach number M1, shown in the table below. In the first
design configuration, shown in figure (a), the deceleration of the flow takes place through a normal
shock. For the second case, shown in figure (b), a wedge-shape diffuser is used to decelerate the flow
through two oblique shock waves followed by a normal shock wave, where the wedge turning angles are
both, θ, as shown in the figure and specified in the table.
Demonstrate the advantage of the ‘weak shock diffuser’ design [shown in (b)] over the simple inlet
[shown in (a)], by evaluating the loss of stagnation pressure for each case.
P02 = 87.68 kPa
Viii total temperature behind the shock
For this case T01 = T02
And being that T02 = 392.2 K
Therefore T01 = 392.2 K
Question 2.7
Two possibilities are considered for the design of a two-dimensional jet engine inlet as shown in the
figures (a-b) below. The inlet is to operate at Mach number M1, shown in the table below. In the first
design configuration, shown in figure (a), the deceleration of the flow takes place through a normal
shock. For the second case, shown in figure (b), a wedge-shape diffuser is used to decelerate the flow
through two oblique shock waves followed by a normal shock wave, where the wedge turning angles are
both, θ, as shown in the figure and specified in the table.
Demonstrate the advantage of the ‘weak shock diffuser’ design [shown in (b)] over the simple inlet
[shown in (a)], by evaluating the loss of stagnation pressure for each case.
Electrical power 18
From the given data in table above; V(Ma2)- v(Ma1)
And v is not kinetic
Part a
The ratio of stagnation pressure is a measure of irresponsibility of the shock pressure process,
Therefore;
P 02
P 01 = P 02
P2 × P 2
P 1 × P1
P 02
P 02
P2 = ( 1+ r−1
2 m2 ¿ r
r −1
P 02
P2 = ( 1+ r−1
2 m1 ¿ r
r −1
From the given data in table above; V(Ma2)- v(Ma1)
And v is not kinetic
Part a
The ratio of stagnation pressure is a measure of irresponsibility of the shock pressure process,
Therefore;
P 02
P 01 = P 02
P2 × P 2
P 1 × P1
P 02
P 02
P2 = ( 1+ r−1
2 m2 ¿ r
r −1
P 02
P2 = ( 1+ r−1
2 m1 ¿ r
r −1
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 19
M1 is given in the table above and the value of r = 1.4
P 02
P 01 = 0.36195
P 02
P 01 ≈ 0.36
Part b
Angle of the first shock for the weak shock diffuses
Angle θ 1= sin-1( 1
M 1)
Angle θ 1= sin-1( 1
3.1)
Angle θ 1= sin-1(0.3225)
Angle θ 1=18.8190
We have vividly approved the oblique shock diffusion angle equal to half angle ,
Therefore
θ=S = 70
Tan θ=2 cosβ ( m12 sinβ −1)
m 12 ¿ ¿
The above equation can be illustrated diagrammatically as below
M1 is given in the table above and the value of r = 1.4
P 02
P 01 = 0.36195
P 02
P 01 ≈ 0.36
Part b
Angle of the first shock for the weak shock diffuses
Angle θ 1= sin-1( 1
M 1)
Angle θ 1= sin-1( 1
3.1)
Angle θ 1= sin-1(0.3225)
Angle θ 1=18.8190
We have vividly approved the oblique shock diffusion angle equal to half angle ,
Therefore
θ=S = 70
Tan θ=2 cosβ ( m12 sinβ −1)
m 12 ¿ ¿
The above equation can be illustrated diagrammatically as below
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 20
The value of the oblique shock from the above given equation will be
βweak=390
βstrong=880
Part c
The weak shock diffusion
The value of the oblique shock from the above given equation will be
βweak=390
βstrong=880
Part c
The weak shock diffusion

Electrical power 21
Now the calculation for the upstream Normal Much number
Min ( weak shock )= M1 sin βweak
Min ( weak shock )= 3.1 sin 39
Min ( weak shock )= 1.951
Min ( strong shock )= M1 sin βweak
Min ( strong shock )= 3.1 sin 88
Min ( strong shock )= 3.098
P 02
P 01 = 1.951
3.098
P 02
P 01 = 0.629
Part d
Therefore in both cases the angle θ is the same hence the angle of the second shock for the weak shock
diffusion is 390
β max= 390
Now the calculation for the upstream Normal Much number
Min ( weak shock )= M1 sin βweak
Min ( weak shock )= 3.1 sin 39
Min ( weak shock )= 1.951
Min ( strong shock )= M1 sin βweak
Min ( strong shock )= 3.1 sin 88
Min ( strong shock )= 3.098
P 02
P 01 = 1.951
3.098
P 02
P 01 = 0.629
Part d
Therefore in both cases the angle θ is the same hence the angle of the second shock for the weak shock
diffusion is 390
β max= 390
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 22
Part e
M2 n = √ ( r −1 ) m2 n2 +2
2r mi n2−r +1
0.6121 (for the weak)
Now the downstream Mach number for weak shock
Weak shock m2 = M 2 n
sin(β−θ)
Weak shock m2 = 0.6121
sin(39−9)
Weak shock m2 =1.2242
Strong shock m2 = M 2 n
sin(β−θ)
Strong shock m2 = 0.4834
sin(88−9)
Strong shock m2 = 0.49244
Therefore P 03
P 02 = 0.49244
1.2242 = 0.4022
Part f
Weak shock m2 = M 2 n
sin(β )
Weak shock m2 = 0.4022
sin(88−39)
Part e
M2 n = √ ( r −1 ) m2 n2 +2
2r mi n2−r +1
0.6121 (for the weak)
Now the downstream Mach number for weak shock
Weak shock m2 = M 2 n
sin(β−θ)
Weak shock m2 = 0.6121
sin(39−9)
Weak shock m2 =1.2242
Strong shock m2 = M 2 n
sin(β−θ)
Strong shock m2 = 0.4834
sin(88−9)
Strong shock m2 = 0.49244
Therefore P 03
P 02 = 0.49244
1.2242 = 0.4022
Part f
Weak shock m2 = M 2 n
sin(β )
Weak shock m2 = 0.4022
sin(88−39)
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 23
Weak shock m2 = 0.4022
0.75471
Weak Shock M2 = 0.5329
Therefore P 04
P 03 = 0.4924
0.5329 = 0.9239
Part g
The overall ratio of P 04
P 01
P 04
P 01 = 0.4924
3.098 = 0.15894
Question 2.8
Referring to the figure below, a supersonic flow with upstream Mach number, M1, static pressure, p1,
and static temperature, T1, as specified in the table below, encounters a corner with a turning angle of
θ. Determine the angle of the oblique shock, β, the angle of the reflected wave, φ, the Mach numbers
M2 and M3, and the downstream static pressure p3 and static temperature T3.
Weak shock m2 = 0.4022
0.75471
Weak Shock M2 = 0.5329
Therefore P 04
P 03 = 0.4924
0.5329 = 0.9239
Part g
The overall ratio of P 04
P 01
P 04
P 01 = 0.4924
3.098 = 0.15894
Question 2.8
Referring to the figure below, a supersonic flow with upstream Mach number, M1, static pressure, p1,
and static temperature, T1, as specified in the table below, encounters a corner with a turning angle of
θ. Determine the angle of the oblique shock, β, the angle of the reflected wave, φ, the Mach numbers
M2 and M3, and the downstream static pressure p3 and static temperature T3.
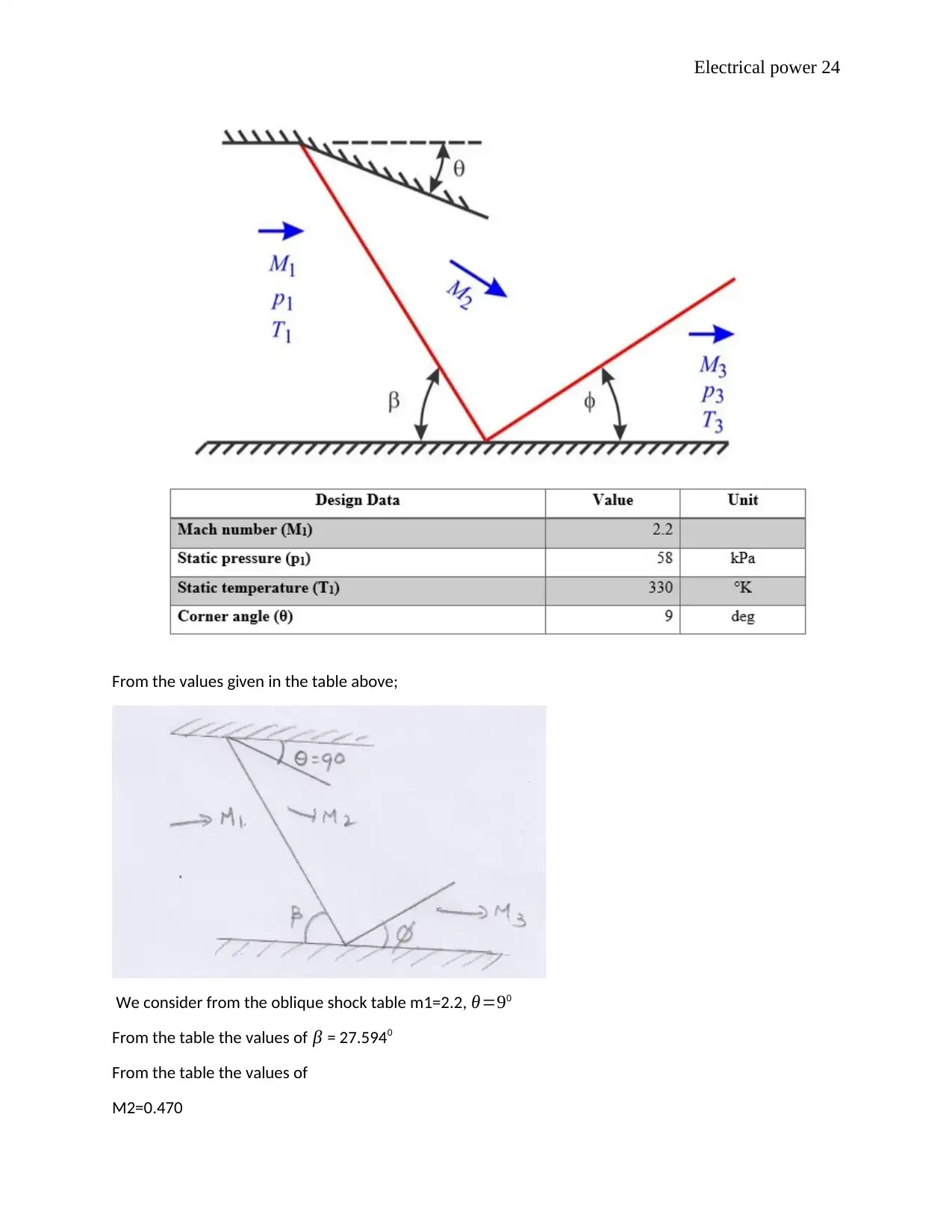
Electrical power 24
From the values given in the table above;
We consider from the oblique shock table m1=2.2, θ=90
From the table the values of β = 27.5940
From the table the values of
M2=0.470
From the values given in the table above;
We consider from the oblique shock table m1=2.2, θ=90
From the table the values of β = 27.5940
From the table the values of
M2=0.470
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 25
P 2
P 1 =5.48
P2= P1×5.48
P2= 58 ×5.48
P2= 317.84 kPa
Min = 2.2 Sin 27.594
Min= 1.0190
From the normal shock table we consider Min = 1
T 2
T 1 = 1
Since the ratio is 1 , it means that T1 = T2
Therefore the value of T2 = 1*T1
T2= 310 k
Now from the oblique shock table
M2 = 0.470
θ=9
β= 106.53
The value of M3 is hence = 2.345
∅ =β−θ
∅ =¿106.53-9
∅ =¿ 97.530
Now if we consider the normal shock table
M2n = M2 sinβ
M2n = 0.470 sin 106.53
M2n = 0.40505
P 3
P2 = 1.83
T 3
T 2 = 1.85
P 2
P 1 =5.48
P2= P1×5.48
P2= 58 ×5.48
P2= 317.84 kPa
Min = 2.2 Sin 27.594
Min= 1.0190
From the normal shock table we consider Min = 1
T 2
T 1 = 1
Since the ratio is 1 , it means that T1 = T2
Therefore the value of T2 = 1*T1
T2= 310 k
Now from the oblique shock table
M2 = 0.470
θ=9
β= 106.53
The value of M3 is hence = 2.345
∅ =β−θ
∅ =¿106.53-9
∅ =¿ 97.530
Now if we consider the normal shock table
M2n = M2 sinβ
M2n = 0.470 sin 106.53
M2n = 0.40505
P 3
P2 = 1.83
T 3
T 2 = 1.85
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Electrical power 26
P3= 1.83× P2
P3= 1.83× 317.84
P3 = 581.64kPa
T3= 1.185× T2
T3= 1.185× 330
T3 = 391.05 K
Question 2.9
A supersonic flow with M, p and T specified in the table below, encounters an expansion corner, which
deflects the flow by an angle of θ. Calculate the Mach number, the static pressure and static
temperature, and the stagnation pressure and stagnation temperature downstream of the expansion
fan, and the angles that the most forward and the most rearward Mach lines make with the upstream
flow.
P3= 1.83× P2
P3= 1.83× 317.84
P3 = 581.64kPa
T3= 1.185× T2
T3= 1.185× 330
T3 = 391.05 K
Question 2.9
A supersonic flow with M, p and T specified in the table below, encounters an expansion corner, which
deflects the flow by an angle of θ. Calculate the Mach number, the static pressure and static
temperature, and the stagnation pressure and stagnation temperature downstream of the expansion
fan, and the angles that the most forward and the most rearward Mach lines make with the upstream
flow.
Electrical power 27
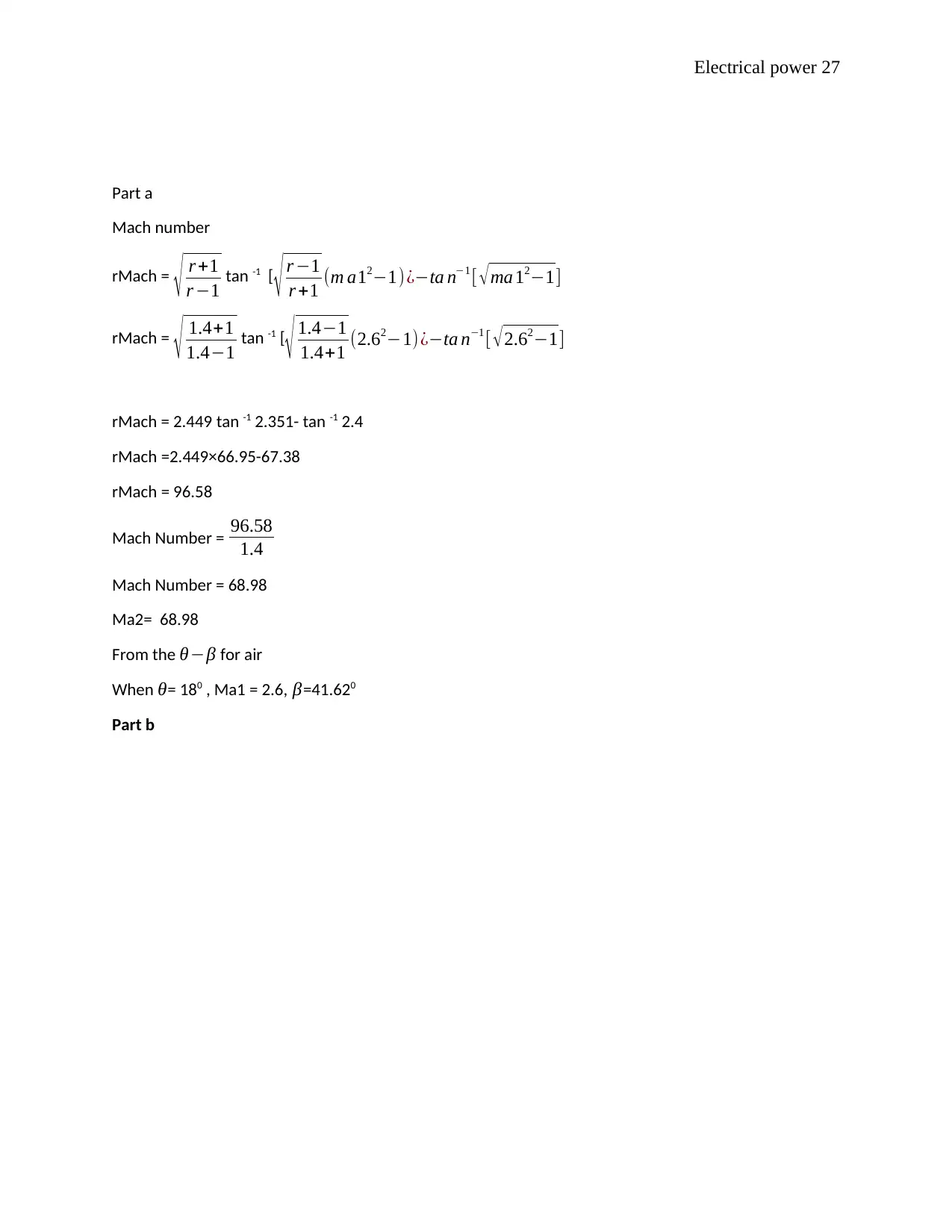
Part a
Mach number
rMach = √ r +1
r −1 tan -1 [ √ r −1
r +1 (m a12−1)¿−ta n−1 [ √ ma 12−1]
rMach = √ 1.4+1
1.4−1 tan -1 [
√ 1.4−1
1.4+1 (2.62−1)¿−ta n−1 [ √ 2.62−1]
rMach = 2.449 tan -1 2.351- tan -1 2.4
rMach =2.449×66.95-67.38
rMach = 96.58
Mach Number = 96.58
1.4
Mach Number = 68.98
Ma2= 68.98
From the θ−β for air
When θ= 180 , Ma1 = 2.6, β=41.620
Part b
Part a
Mach number
rMach = √ r +1
r −1 tan -1 [ √ r −1
r +1 (m a12−1)¿−ta n−1 [ √ ma 12−1]
rMach = √ 1.4+1
1.4−1 tan -1 [
√ 1.4−1
1.4+1 (2.62−1)¿−ta n−1 [ √ 2.62−1]
rMach = 2.449 tan -1 2.351- tan -1 2.4
rMach =2.449×66.95-67.38
rMach = 96.58
Mach Number = 96.58
1.4
Mach Number = 68.98
Ma2= 68.98
From the θ−β for air
When θ= 180 , Ma1 = 2.6, β=41.620
Part b
You're viewing a preview
Unlock full access by subscribing today!

Electrical power 28
Ma in = Ma 1 sin β = 2.6 sin 41.62
Ma in = 1.72688
P 2
P 1 = 2rm a2 −r +1
r +1
P 2
P 1 = 2× 1.4 ×1.7268 82−1.4 +1
14+1
P 2
P 1 = 3.312466
P2 = 3.312466P1
P2= 3.312466×52
P2= 172.248 kPa
Part c
T 2
T 1=[2+(r-1) ma2] [ 2rm a2 −r +1
¿ ¿ ]
T 2
T 1=[2+(r-1) ma2] [ 2rm a2 −r +1
¿ ¿ ]
T 2
T 1=[2+(0.4) 1.726882] [ 2× 1.4 ×1.726882−1.4 +1
¿ ¿ ]
T 2
T 1= 3.19284×0.4628
T 2
324 = 3.19284×0.4628
T2 = 478.75K
Part d
P01 = 1157.33kPa
Ma in = Ma 1 sin β = 2.6 sin 41.62
Ma in = 1.72688
P 2
P 1 = 2rm a2 −r +1
r +1
P 2
P 1 = 2× 1.4 ×1.7268 82−1.4 +1
14+1
P 2
P 1 = 3.312466
P2 = 3.312466P1
P2= 3.312466×52
P2= 172.248 kPa
Part c
T 2
T 1=[2+(r-1) ma2] [ 2rm a2 −r +1
¿ ¿ ]
T 2
T 1=[2+(r-1) ma2] [ 2rm a2 −r +1
¿ ¿ ]
T 2
T 1=[2+(0.4) 1.726882] [ 2× 1.4 ×1.726882−1.4 +1
¿ ¿ ]
T 2
T 1= 3.19284×0.4628
T 2
324 = 3.19284×0.4628
T2 = 478.75K
Part d
P01 = 1157.33kPa
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Electrical power 29
Part e
T 01
T 1 = 1+ r−1
2 ma12
T 01
T 1 = 1+ 1.4−1
2 2.62
T 01
324 =2.352
T01= 762.048K
P 01
P1 = [1+ r−1
2 ma12
¿
r
r −1
P 01
P1 = [1+ 1.4−1
2 2.62
¿
1.4
0.4
P02= 977kPa
T 02
T 2 = [1+ r−1
2 ma12
T 02
T 2 = 1+ 1.4−1
2 ×1.71982
T02 = 761.99 k
Part f
μ 1= sin-1 ( 1
ma1 ¿
μ 1= sin-1 ( 1
2.6 ¿
μ 1= sin-1 (0.3846315
μ 1= 22.61980
Part g
Part e
T 01
T 1 = 1+ r−1
2 ma12
T 01
T 1 = 1+ 1.4−1
2 2.62
T 01
324 =2.352
T01= 762.048K
P 01
P1 = [1+ r−1
2 ma12
¿
r
r −1
P 01
P1 = [1+ 1.4−1
2 2.62
¿
1.4
0.4
P02= 977kPa
T 02
T 2 = [1+ r−1
2 ma12
T 02
T 2 = 1+ 1.4−1
2 ×1.71982
T02 = 761.99 k
Part f
μ 1= sin-1 ( 1
ma1 ¿
μ 1= sin-1 ( 1
2.6 ¿
μ 1= sin-1 (0.3846315
μ 1= 22.61980
Part g
1 out of 29
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.


