Engineering Mathematics | Assignment

MATHEMATICS 1
ENGINEERING MATHEMATICS
by Student’s Name
Course Name
Professor’s Name
University Name
City, State
Date

MATHEMATICS 2
QUESTION 3
a) (sin θ+cos θ ¿2 -2 sin θ cos θ = 1
Solution
Manipulating left side
(sin θ+cos θ ¿2 -2 sin θ cos θ
Apply perfect square formula: (a +b)2 = a2+2ab+b2
a = cos θ and b = sin θ
= cos2θ + 2cos θ sin θ + sin2θ
= cos2θ + 2cos θ sin θ + sin2θ -2cos θ sin θ
Add similar elements: 2cos θ sin θ -2cos θ sin θ =0
= cos2θ + sin2θ
Use the following identity cos2x + sin2x = 1
Hence cos2θ + sin2θ = 1
∴ (sin θ+cos θ ¿2 -2 sin θ cos θ = 1 is True
b) tanθ cosecθ = secθ
Solution
Manipulating the left side
tanθ cosecθ
Using the basic trigonometric identity cosec x = 1
sin x

MATHEMATICS 3
= tanθ * 1
sinθ
Use the following identity: tan x = sinx
cos x
= sinθ
cos θ * 1
sinθ
Cancel the like terms
= 1
cos θ from identity: 1
cos θ = secθ
= secθ which is true
Therefore tanθ cosecθ = secθ
c) cosecθ(1-cosθ)(1+cosθ) = sinθ
Solution
Manipulating left side
cosecθ(1-cosθ)(1+cosθ)
Use the following identity: cosec x = 1
sin x
= 1
sin θ (1-cosθ)(1+cosθ)
Use the following identity: (1-cosθ)(1+cosθ) = 1-cos2θ
= 1
sin θ (1-cos2θ)
Simplify (1-cos2θ) = sin2θ
= 1
sin θ sin2θ

MATHEMATICS 4
= sinθ which is true
Therefore cosecθ(1-cosθ)(1+cosθ) = sinθ
d) cotθ secθ tan θ√ (1−sin2 θ) = 1
Solution
Manipulating left side
cotθ secθ tan θ√(1−sin2 θ)
Simplify
√ (1−sin2 θ) = cosθ
= 1
tanθ * 1
cosθ * tanθ * cosθ
Cancel the like terms
= 1 which is true
Therefore cotθ secθ tan θ √(1−sin2 θ) = 1
e) 1
sec2 θ + 1
cosec2 θ =1
Solution
Manipulating left side
1
sec2 θ + 1
cosec2 θ
Express with sin, cos
Using the basic trigonometric identity cosec x = 1
sin x

MATHEMATICS 5
=
1
( 1
sinθ ) 2 +
1
( 1
cosθ )2
Simplify by apply exponent rule and fraction rule
=
1
( 1
cosθ )2 = cos2
θ and
1
( 1
sinθ ) 2 = sin2
θ
= cos2θ + sin2
θ
Use the following identity: cos2θ + sin2
θ = 1
= 1 two sides could take the same form
Hence 1
sec2 θ + 1
cosec2 θ =1
f) secθ – cosθ = sinθtanθ
Solution
Manipulating left side
secθ – cosθ
Express with sin, cos
Using the basic trigonometric identity sec x = 1
cos x
= - cos θ + 1
cos θ
Simplify and applying exponent rule
= −cos θcosθ
cos θ + 1
cos θ
Since denominators are equal, combine the fraction:

MATHEMATICS 6
= −cos2 θ+1
cos θ
= 1−cos2 θ
cos θ
Use the following identity: 1−cos2 x = sin2 x
= sin2 θ
cos θ
Use the following identity: sinx
cos x = tan x
= sin θtanθ which is true
Hence secθ – cosθ = sin θtanθ
g) sin2θ + 2cos2θ = 2- sin2θ
Solution
Manipulating left side
sin2θ + 2cos2θ
Use the following identity: cos2 x = 1- sin2 x
= sin2θ + 2(1- sin2 θ)
Expand: 2(1- sin2 θ)
= 2- 2 sin2 θ
= sin2θ + 2- 2 sin2 θ
Simplify and group like terms
= sin2θ - 2sin2 θ+2

MATHEMATICS 7
Add similar elements:sin2θ - 2sin2 θ = - sin2θ
= - sin2θ + 2
= 2- sin2θ which is true
Therefore sin2θ + 2cos2θ = 2- sin2θ
h) tanθ + cotθ = 1
sin θcosθ
Solution
Manipulating the left side
tanθ + cotθ
Express with sin, cos:
= cosθ
sin θ + tanθ
Using the basic trigonometric identity tan x = sin x
cos x
= cosθ
sin θ + sin θ
cos θ
Simplify above
cosθ
sin θ = cos2 θ
sin θcosθ and sin θ
cos θ = sin2 θ
sin θcosθ
= cos2 θ
sin θcosθ + sin2 θ
sin θcosθ
Since the denominator are equal, combine the fraction
= cos2 θ+sin2 θ
sin θcosθ

MATHEMATICS 8
Use the following identity: cos2 x +sin2 x = 1
= 1
sin θcosθ which is true
Therefore tanθ + cotθ = 1
sin θcosθ
QUESTION 4
a) sec x = 2
Solution
sec x = 2, 0 ≤ x ≤ 3600
General solution for sec x = 2
x=600 +3600 n, x=3000 +3600 n
Solutions for range 0 ≤ x ≤ 3600
x=600, x=3000
b) cot x = √3
Solution
cot x = √3, 0 ≤ x ≤ 3600
General solution for sec x = √3
x=300 +1800 n,
Solutions for range 0 ≤ x ≤ 3600

MATHEMATICS 9
x=300, x=2100
c) cosec x = √ 2
Solution
cosec x = √ 2, 0 ≤ x ≤ 2π
cosec x can’t be greater than one for real solutions
No solution for x ∈ R
d) sec x = 1.2
Solution
sec x = 1.2, 0 ≤ x ≤ 3600
General solution for sec x =1.2
sec x=a → x = arcsec a + 3600 n, x= 3600 –arcsec a + 3600 n
x = arcsec 1.2 + 3600 n, x= 3600 –arcsec 1.2 + 3600 n
Solutions for range 0 ≤ x ≤ 3600
x=0.58568, x=5.69749
e) cot x =3
Solution
cot x = 3, 0 ≤ x ≤ 3600
General solution for cot x =3
cot x=a → x = arccot a + 1800 n
x = arccot 3 + 1800 n

MATHEMATICS 10
Solutions for range 0 ≤ x ≤ 3600
x= arccot 3, x= arccot 3 + 1800 n
Solution in decimal form
x=0.3215, x=0.32175…..+180
f) cosec x =1
Solution
cosec x =1, 0 ≤ x ≤ 2π
General solution for cosec x =1
Solve ec x = 0 + 2πn
x= 2 πn
ec
Solutions for range 0 ≤ x ≤ 2π
No solution for x ∈ R
QUESTION 5
a) cosecθ- sinθ = cotθ cosθ
Solution
Manipulating left side
cosecθ - sinθ
Using the basic Trigonometric identity cosec x = 1
sin x
= 1
sin θ – sinθ

MATHEMATICS 11
Simplify above
Since sinθ = sinθsinθ
sin θ
= 1
sin θ – sinθsinθ
sin θ since denominators are equal we combine
= 1−sinθsinθ
sin θ
= 1−sin2 θ
sin θ apply following identity 1-sin2x = cos2x
= cos2 θ
sin θ use the following identity cosx
sinx =cot x
= cosθ cotθ which is true
∴ cosecθ- sinθ = cotθ cosθ is true
b) cos2θ-sin2θ=2 cos2θ-1
Solution
Manipulating left side
cos2θ-sin2θ
Using the following identity factor sin2x =1-cos2x
= - (1- cos2θ) + cos2θ
Distribute parentheses
= -1-(- cos2θ)
Apply minus-plus rule
-(-a)=a, -(a)=-a

MATHEMATICS 12
= -1+ cos2θ
= -1+ cos2θ + cos2θ
Add similar elements
= -1+2 cos2θ which is true
∴ cos2θ-sin2θ=2 cos2θ-1
c) cosec2 θ+sec2 θ= cosec2 θsec2 θ
Solution
Manipulating left side
cosec2 θ+sec2 θ
= 1
sin 2θ + 1
cos 2 θ
= cos 2 θ+sin 2θ
sin 2 θcos 2 θ = 1
sin 2θcos 2 θθ
= cosec2 θsec2 θ which is true
Therefore cosec2 θ+sec2 θ= cosec2 θsec2 θ
d) sin2 θ
1−cosθ =1+cosθ
Solution
Manipulating left side
sin2 θ
1−cosθ
Using the following identity factor sin2x =1-cos2x

MATHEMATICS 13
¿ 1−cos2 θ
1−cosθ
Simplify
= cos2θ-1
Rewrite 1 as 12
= cos2θ-12
Apply difference of two square formula
= (cosθ +1) (cosθ -1)
= - (cosθ + 1) (cosθ -1)
= −( cosθ+1)(cosθ−1)θ
1−cosθ
= cosθ + 1
= 1 + cosθ which is true
∴ sin2 θ
1−cosθ =1+cosθ
e) sinθ
1+ sinθ =tanθ(secθ-tanθ)
Solution
Manipulating left side
sinθ
1+ sinθ
= sinθ
cos2 θ+ ¿ sin2 θ +sinθ ¿

MATHEMATICS 14
sinθ
cos2 θ + sinθ
sin2 θ + sinθ
sinθ
Simplifying
sinθ
1+ sinθ = tanθ(secθ-tanθ)
f) cosec2θ(tan2θ-sin2θ)=tan2θ
Solution
Manipulating left side
cosec2θ(tan2θ-sin2θ)
1
sin 2θ *( tan2θ-sin2θ)
Like terms cancels
= tan2θ which is true
Therefore cosec2θ(tan2θ-sin2θ)= tan2θ
g) secθ
tanθ+cotθ = sinθ
Solution
Manipulating left side
secθ
tanθ+cotθ
Using the basic trigonometric identity sec x = 1
cosx
=
1
cosθ
tanθ+cotθ

MATHEMATICS 15
Using the basic trigonometric identity tan x = sin x
cosx
=
1
cosθ
cosθ
sinθ + sinθ
cosθ
Simplify
=
1
cosθ ( cosθ
sinθ + sinθ
cosθ )
= sinθ
cosθ = sinθsinθ
cosθsinθ = sin2 θ
sinθcosθ
= cos2 θ
sinθcosθ + sin2 θ
sinθcosθ
Since the denominators are equal, combine the fractions
= cos2 θ+sin2 θ
sinθcosθ
=
1
cos2 θ+ sin2 θ
sinθcosθ cosθ
Multiply fractions;
= cos2 θ+sin2 θ cosθ
sinθcosθ
Cancel the common factor
= cos2 θ+sin2 θ
sinθ

MATHEMATICS 16
=
1
cos2 θ+sin2 θ
sinθ
Apply the fraction rule
= sinθ
cos2 θ+sin2 θ
Using the following identity cos2 x +sin2 x = 1
= sinθ which is true
∴ secθ
tanθ+cotθ
= sinθ
h) cosθ
sin θ+1 + sinθ +1
cosθ =2 secθ
Solution
Manipulating left side
cosθ
sin θ+1 + sinθ +1
cosθ
Simplify
= ( 1+ sinθ ) 2+ 1−sinθ2
( 1+sinθ ) cosθ
Cancel common factor ( 1+sinθ )
= 2
cosθ
Use the following identity: 1
cosx = secx
= 2 secθ which is true

MATHEMATICS 17
Therefore cosθ
sin θ+1 + sinθ +1
cosθ = 2 secθ
QUESTION 6
Curve y = sec x for 0≤ x < 900
Axis interception points of sec x, 0≤ x < 900: Y intercepts (0, 1)
Asymptotes sec x, 0≤ x < 900: None
Extreme points of sec x, 0≤ x < 900: Minimum (0, 1)
Plotting: y = sec x for 0≤ x < π
2

MATHEMATICS 18
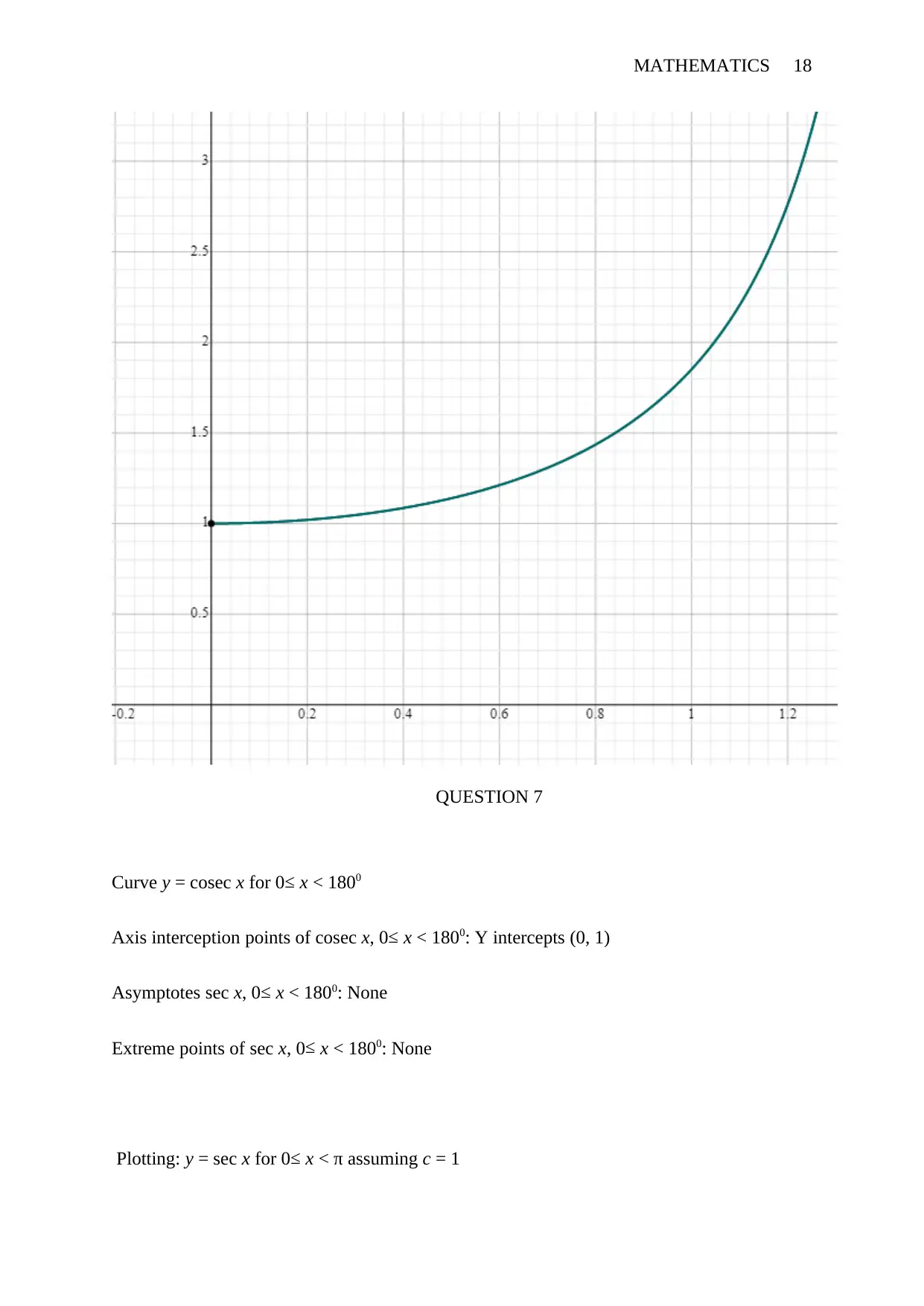
QUESTION 7
Curve y = cosec x for 0≤ x < 1800
Axis interception points of cosec x, 0≤ x < 1800: Y intercepts (0, 1)
Asymptotes sec x, 0≤ x < 1800: None
Extreme points of sec x, 0≤ x < 1800: None
Plotting: y = sec x for 0≤ x < π assuming c = 1

MATHEMATICS 19
QUESTION 8
a) 2cot2θ-3cotθ+1=0 for 0 ≤ θ ≤3600
Solution
Let cotθ = u
2u2θ-3u+1 = 0
Use quadratic equation
¿ −(−3) ± √−32−4∗2∗1
2∗2

MATHEMATICS 20
Apply rule – (-a)=a
= 3± √−32−4∗2∗1
2∗2
Simplify
u = 1, u = 1
2
Substitute back u = cotθ
cotθ = 1, cotθ = 1
2
General solution for cotθ = 1
θ = π
4 + πn
General solution for cotθ = 1
2
cotθ =a x = arccot 1
2 + πn
Combine all the solution
θ = π
4 + πn, θ = arccot 1
2 + πn
Show solution in decimal form
θ = π
4 + πn, θ=1.10714…+ πn
b) sec2θ-tan θ = 1 for -1800≤ θ ≤1800
Solution
Subtract 1 from both sides

MATHEMATICS 21
sec2θ-tan θ -1 = 0
Using identity
- tan θ + tan2θ=0
Let tan θ =u
-u2 + u = 0
Solve using quadratic equation
u= 1± √−12−4∗1∗0
2∗1
Simplify
u=1, u=0
Substitute back u = tan θ
tan θ =1, tan θ =0
tan θ =1, -1800≤ θ ≤1800
General solution for tan θ =1
θ = 450 + 1800
Solution for the range -1800≤ θ ≤1800
θ = 450, θ = -1350
θ =0, θ =1800,θ = -1800
Combine all the solutions
θ = 450, θ = -1350, θ =0, θ =1800,θ = -1800

MATHEMATICS 22
c) cot2θ-3cosec θ+3 = 0 0 ≤ θ ≤1800
Solution
Simplify: cot2θ-3cosec θ+3 = 0
= sinθ – 3 tan2 θ+3 tan2 θ sinθ
tan2 θsinθ
Express with sin, cos: sinθ – 3 tan2 θ +3 tan2 θ sinθ
= sinθ - ( sinθ
cosθ )
2
*3 + ( sinθ
cosθ )
2
*3sinθ
Simplify
= −3 sin2 θ+ 3 sin3 θ+ cos2 θsinθ
cos3 θ = 0
Factor −3 sin2 θ+3 sin3 θ+cos2 θsinθ
= sin θ(-3 sinθ + 3 sin2 θ+cos2 θ ¿=0
Solving each part separately
= sinθ = 0 or -3 sinθ + 3 sin2 θ+cos2 θ=0
sinθ = 0, 0 ≤ θ ≤1800 : θ = 0, θ=1800
-3 sin θ + 3 sin2 θ+cos2 θ =0, 0 ≤ θ ≤1800: θ=900 , θ=300, θ=1500
Combine all solutions
θ = 0, θ=1800, θ=900 , θ=300, θ=1500
Since the equation is undefined for 0, 1800, 900
θ=300, θ=1500

MATHEMATICS 23
QUESTION 9
a) sin4x-cos4x = sin2x-cos2x
Solution
Manipulating left side
sin4x-cos4x
= (- cos x + sin x) (cos x + sin x) (sin2x+cos2x)
Use the identity sin2x+cos2x = 1
= (- cos x + sin x) (cos x + sin x)
Expand
= - cos2x + sin2x which is true
∴sin4x-cos4x = sin2x-cos2
b) sec4x-tan4x = 1+2 tan2x
Solution
Manipulating left side
sec4x-tan4x
Factor sec4x-tan4x

MATHEMATICS 24
= (sec x + tan x) (sec x-tan x) (sec2 x-tan2 x)
Expand
= sec2 x-tan2 x
Using following identity: sec2 x-tan2 x = 1
= sec2 x +tan2 x
Using the following identity: sec2 x = 1+ tan2 x
= 1 + tan2 x + tan2 x
= 1 + 2tan2 x which is true
∴sec4 x - tan4 x = 1+2 tan2x
c) sinθ
1−cosθ + sinθ
1+ cosθ = 2 cosecθ
Solution
Manipulating left side
sinθ
1−cosθ + sinθ
1+ cosθ
Adjust fractions based on the LCM
= sinθ (cosθ−1)
(1−cosθ)(1+cosθ) + sinθ (−cosθ+1)
(1+cosθ )(−cosθ+ 1)
Since denominator are equal, we combine
= sinθ ( cosθ−1 ) +sinθ (−cosθ+1)
(1−cosθ)(1+ cosθ)
Factor above equation

MATHEMATICS 25
= 2 sinθ
(1−cosθ)(1+cosθ)
= 2 sinθ
sin2 θ
Cancel common factor
= 2 cosecθ
Therefore sinθ
1−cosθ + sinθ
1+ cosθ = 2 cosecθ
d) cot2 θ
1−cot2 θ = cos2 θ
Solution
We take the left side
cot2 θ
1−cot2 θ
= 1−cot2 θ = (- cotθ +1) (cotθ-1)
= cot2 θ
(−cotθ+1)(cotθ−1)
Simplify
= cosθ* cosθ
= cos2 θ which is the same
Therefore cot2 θ
1−cot2 θ = cos2 θ
e) 1−tan2 θ
1+tan2 θ = 1- 2sin θ

MATHEMATICS 26
Solution
Manipulating left side
1−tan2 θ
1+tan2 θ
Using identity: 1+ tan2 x = sec2x
= 1−tan2 θ
sec2 x
= −cotθ +1 ¿ ¿
= −cotθ +1 ¿(cotθ −1) ¿
sec 2θ
Simplify
= 1- 2sinθ
Hence 1−tan2 θ
1+tan2 θ =¿1- 2sin θ
f) 2tanθ
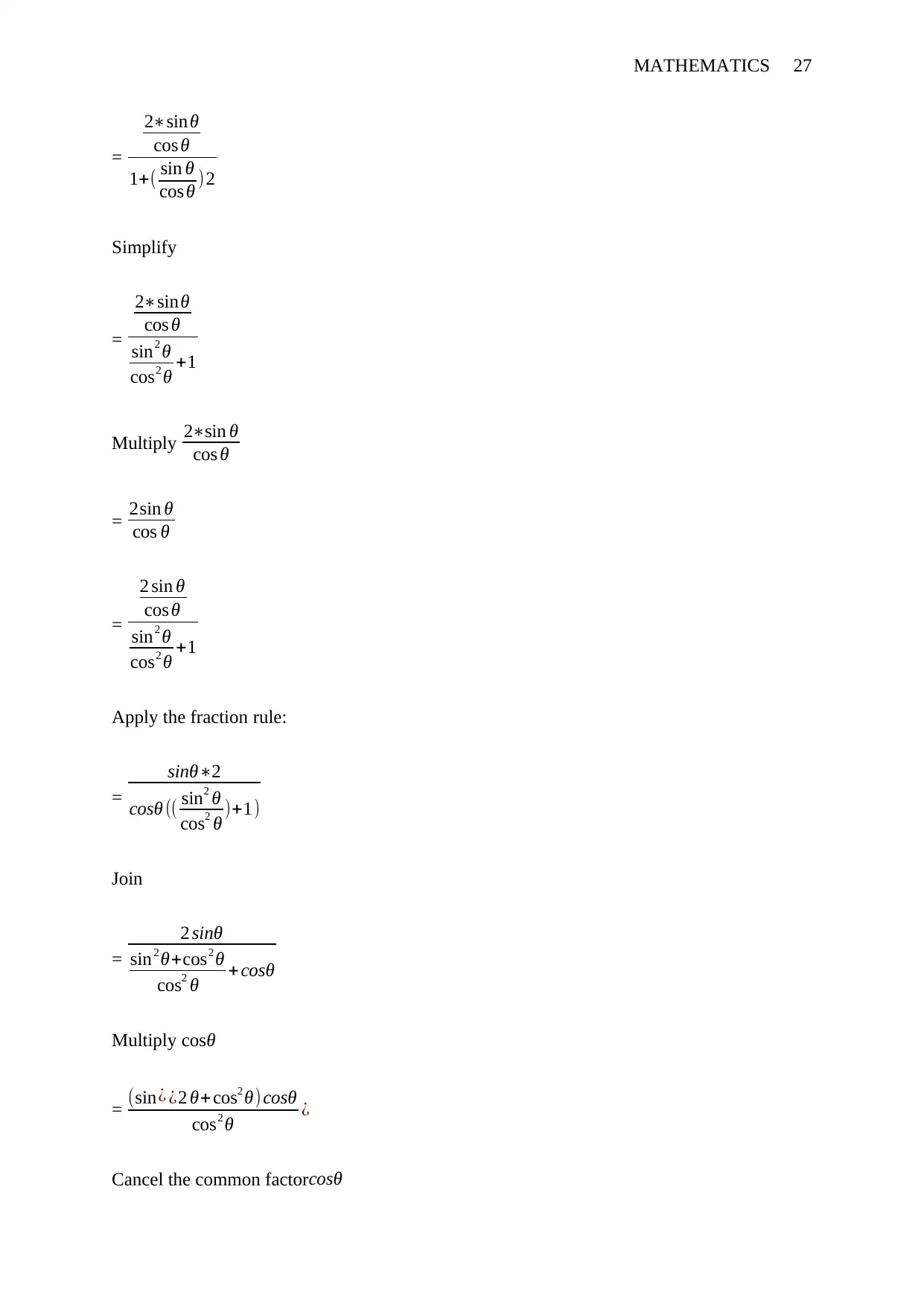
1+ tan2 θ = 2sinθcosθ
Solution
Manipulating left side
2tanθ
1+ tan2 θ
Express with sin, cos

MATHEMATICS 27
=
2∗sinθ
cos θ
1+( sin θ
cos θ )2
Simplify
=
2∗sinθ
cos θ
sin2 θ
cos2 θ +1
Multiply 2∗sin θ
cos θ
= 2sin θ
cos θ
=
2 sin θ
cos θ
sin2 θ
cos2 θ +1
Apply the fraction rule:
=
sinθ∗2
cosθ (( sin2 θ
cos2 θ )+1)
Join
=
2 sinθ
sin2 θ+cos2 θ
cos2 θ + cosθ
Multiply cosθ
= (sin¿ ¿2 θ+ cos2 θ) cosθ
cos2 θ ¿
Cancel the common factorcosθ

MATHEMATICS 28
= sin2 θ+ cos2 θ
cosθ
Apply the fraction rule:
= sinθ∗2 cosθ
sin2 θ+ cos2 θ
= 2 sinθcosθ
sin2 θ+ cos2 θ
Use identity sin2 θ+cos2 θ= 1
= 2 sinθcosθ which is true
∴ 2 tanθ
1+ tan2 θ = 2sinθcosθ
g) cot θ+tanθ
cosecθ+ secθ = 1
cos θ+sin θ
Solution
Manipulating the left side
cot θ+tanθ
cosecθ+ secθ
Simplify
= 2 cosecθ
cosecθ+ secθ
Simplify further
= 1
cos θ+sin θ which is true
Thus cot θ+tanθ
cosecθ+ secθ = 1
cos θ+sin θ

MATHEMATICS 29
h) sin3x+cos3x = (sin x + cos x) (1-sin x cos x)
Solution
Manipulating left side
sin3x+cos3x
Factor sin3x+cos3x
= (cos x +sin x) (cos2 x + sin2 x – cos x sin x)
Using the following identity cos2 x + sin2 = 1
= (1- cos x sin x) (cos x +sin x) which is true
∴ sin3x+cos3x = (sin x + cos x) (1-sin x cos x)
QUESTION 10
Solve inequality cosecθ < 2 for value θ between 0 and 1800
Solution
Subtract 2 from both sides
cosecθ -2 < 2-2
Simplify
cosecθ -2 < 0
No solution for c
QUESTION 11
a) simplify (1-sinθ)(1+sinθ) secθ
Solution

MATHEMATICS 30
Expand (1-sinθ) (1+sinθ)
Apply difference of two squares formula
= 12-sin2θ
Apply rule 1a = 1
12=1
= 1-sin2θ
= secθ (1-sin2θ)
Expand secθ (1-sin2θ)
Apply the distributive law:
= secθ. 1- secθ sin2θ
=1. Secθ - sin2θ secθ
Multiply: 1.secθ= secθ
= secθ - sin2θ secθ
b) solve inequality (1-sinθ) (1+sinθ) secθ > 1
2 for values of θ between -900 and 900
Solution
Express with sin, cos
(1-sinθ) (1+sinθ) 1
cosθ > 1
2
Simplify
= (1−sinθ )(1+ sinθ )
cosθ > 1
2

MATHEMATICS 31
Find the zeroes and undefined points of (1−sinθ )(1+sinθ )
cosθ for 0≤ θ ≤3600
Identify the intervals
0≤ θ ≤900, 90≤ θ ≤2700, 2700≤ θ ≤3600
Identify the intervals that satisfy the required condition :> 0
𝜃¿ 0 or, 0 ≤ θ ≤900 or 2700
≤ θ ≤3600 or
𝜃¿ 3600
Merge Overlapping intervals
0 ≤ θ ≤900 or 2700≤ θ ≤3600
Apply the periodicity of (1-sinθ) (1+sinθ) secθ
3600
n ≤ θ ≤900 + 3600n or 2700 + 3600
n < θ ≤ 3600 + 3600
n and -900 ≤ θ ≤900
2700-3600 < θ < 900
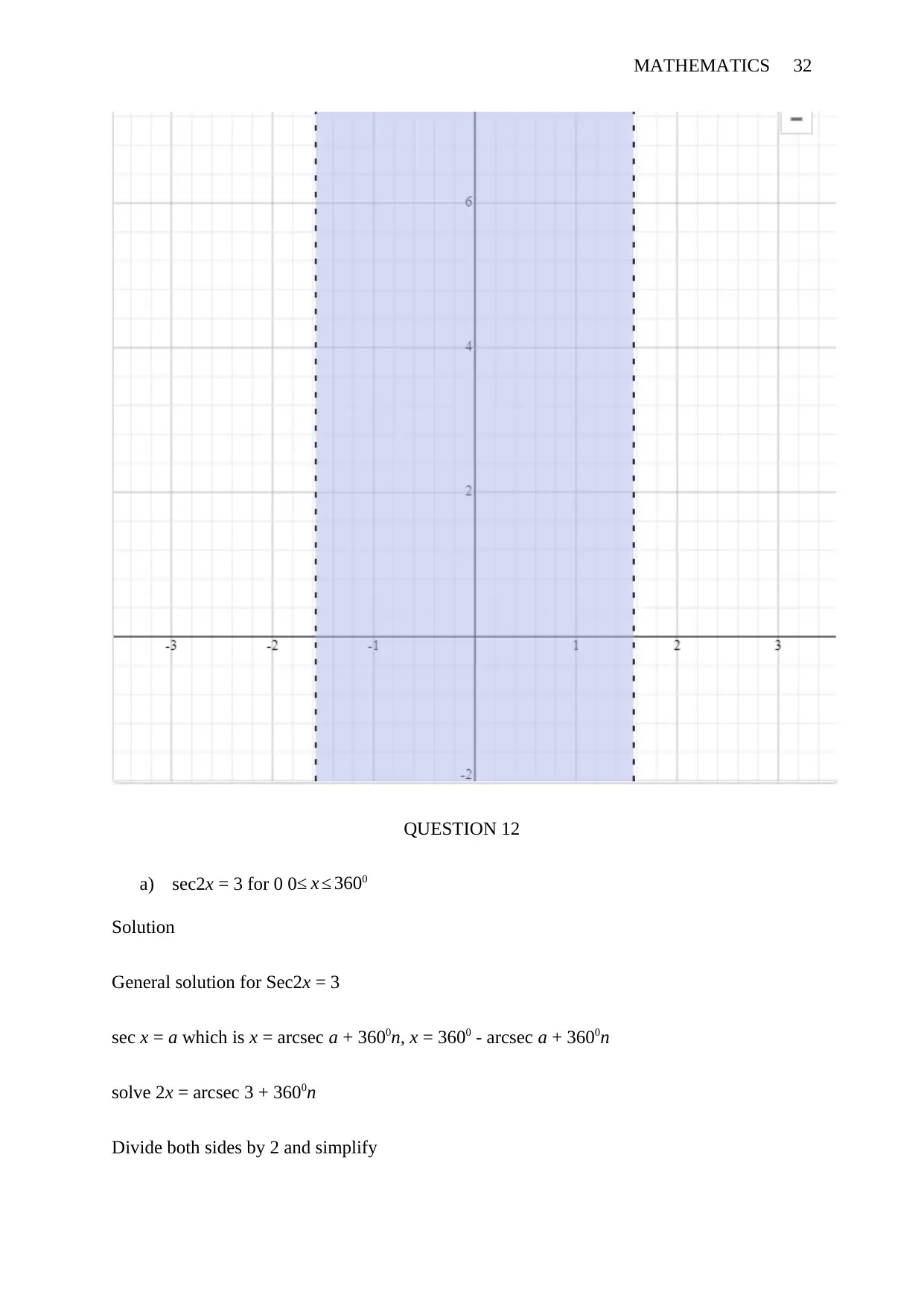
Number line
Graph

MATHEMATICS 32
QUESTION 12
a) sec2x = 3 for 0 0≤ x ≤ 3600
Solution
General solution for Sec2x = 3
sec x = a which is x = arcsec a + 3600n, x = 3600 - arcsec a + 3600n
solve 2x = arcsec 3 + 3600n
Divide both sides by 2 and simplify

MATHEMATICS 33
x = arcsec 3+360 n
2
Solve 2x
x = arcsec 3+360 n
2 , x = 360−arcsec 3+360 n
2
Solution for the range 0≤ θ ≤3600
x = arcsec 3
2 , x= 360−arcsec 3
2 , x= arcsec 3+360
2 ,x = 720−arcsec 3
2
Show solution in decimal form
x = 1.23095
2 , x= 360−1.23095
2 , x= 1.23095+360
2 , x = 720−1.23095
2
b) 3 cosec22x = 4 for 0 0≤ x ≤ 1800
Solution
3 cosec22x = 4
Dividing both sides by 6 cos (e) c2
= 3 cos( e) c2 2 x
6 cos(e)c2
= 4
6 cos( e) c2
Simplify
x = 2
3 cos( e) c2 ; c≠ 0

MATHEMATICS 34
QUESTION 13
Simultaneous equation
cosec 2x = √ 2
cot (x+y) = √3
Solution
cosec x can’t be greater than one for real solutions
no solution for cot (x+y) = √3
since x ε R