Engineering Mathematics Assignment Solution: Calculus Problems

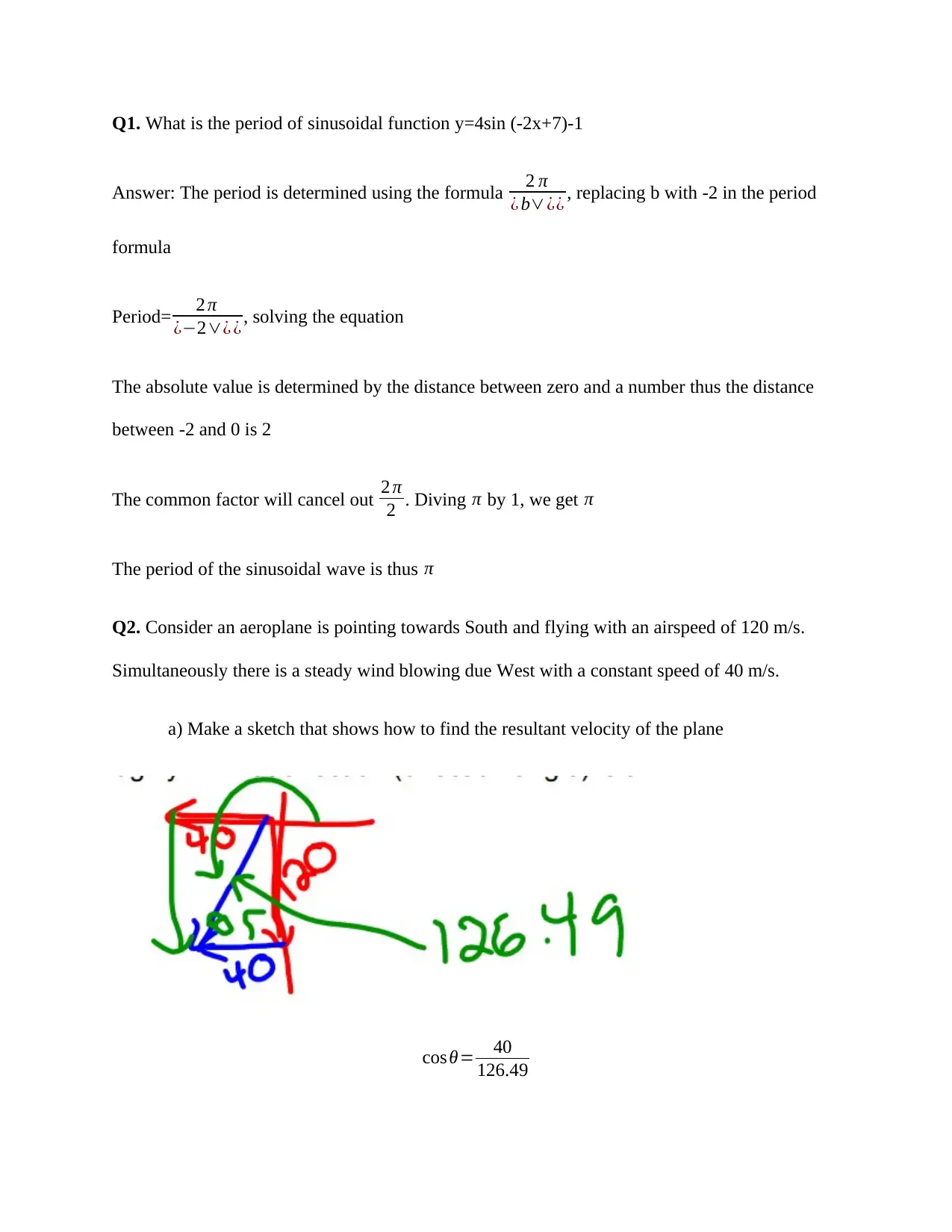
This document provides a detailed solution to an engineering mathematics assignment, focusing on calculus concepts. The assignment includes problems on sinusoidal functions, calculating the period of a wave, and determining the resultant velocity of an airplane with wind. It also covers the average rate of change of a function, finding the rate of change using natural logarithms, and calculating the rate of change over an interval. Furthermore, the solution addresses work done in compressing a spring, finding intervals where a function is increasing or decreasing based on its derivative, and separating a wave into distinct component waves using compound angle identities. The document concludes with a list of references.

ENGINEERING MATHEMATICS
By Name
Course
Instructor
Institution
Location
Date

Q1. What is the period of sinusoidal function y=4sin (-2x+7)-1
Answer: The period is determined using the formula 2 π
¿ b∨¿¿ , replacing b with -2 in the period
formula
Period= 2 π
¿−2∨¿ ¿ , solving the equation
The absolute value is determined by the distance between zero and a number thus the distance
between -2 and 0 is 2
The common factor will cancel out 2 π
2 . Diving π by 1, we get π
The period of the sinusoidal wave is thus π
Q2. Consider an aeroplane is pointing towards South and flying with an airspeed of 120 m/s.
Simultaneously there is a steady wind blowing due West with a constant speed of 40 m/s.
a) Make a sketch that shows how to find the resultant velocity of the plane
cos θ= 40
126.49

θ=71.56 °
tan θ= 120
40 , θ=251.56° which is the direction angle
b) What is the resultant speed of the aeroplane?
126.49 m/s
Q3.1. If f(x) =x3-9x, what is the average rate of change of f over the interval [1, 6]?
The average rate of change of a function is established by evaluating the change in the
values of y of the two pointes and then dividing the resultant by the change in the values of x of
the two points
f ( 0 ) −f ( 6)
( 0 )−(1)
Substituting the equation y=x3-9x for f (0) and f (6) and substituting the x in the function with the
corresponding values of x
{1 ¿ ¿=-8/-1=8
Q3.2 If f(x) =ln (x)/, find the rate of change of f(x) between 1 and 1.5
Answer: The average rate of change is determined by
f ( 1.5 )−f (1)
1.5−1 = ln (1.5 )−ln (1)
0.5 = ln( 0.5)
0.5 =−1.38629
Q3.3. What is the rate of change of the interval π ≤ x ≤ 4 π /3, for the function y=4 sin x-7
f ( 4 π /3 ) −f ( π)
( 0 ) −(1) = 0.969−2.624
1.047 =−1.581

Q4. A force of 1200 N compresses a spring from its natural length of 18 cm to a length of 16 cm.
How much work was done in compressing it from 16 cm to 14 cm?
Answer: F=k*x
1200=k*0.02
K=1200/0.02=60000 N/m
Work done in compressing it from 16 to 14 cm
X=16-14=2 cm=0.02 m
Work done=1/2*6000*0.022=12 joules
Q5.1. Consider the derivative of f is defined as f1=x2/(x-2)3. Find the intervals where f is
increasing
Answer: The intervals are determines using critical points which refer to the points at
which f1 is either 0 or undefined
f1=x2/(x-2)3=x2+2x-3
The critical points are x=-3 and x=1 which can divide the number line in three main groups as
shown
The intervals when f1 is decreasing is thus x<-3

Q5.2. Consider the derivative of g is defined as g1=x2/(x+3). Find the intervals where g is
decreasing.
Since g(x) is not continuous at x=1, it is not possible to differentiate it at that point thus x=1 is
one of the critical points. The provided derivative offers the other critical point in which the
negative exponents is preferred instead of the fractions
The critical points are x=-3/2 and x=1
The intervals when g1 is decreasing is thus x<1
Q6. Separate the wave Y =5 sin (θ+53.13 °) into distinct component waves using compound
angle identities
sin(θ +53.13° )= sin Ɵ cos 53.13⁰ + cos Ɵ sin 53.13⁰, [Applying the formula of sin (A+B) =sin A
cos B +cos A sin B
¿ 1 ×cos 53.13 ° +0 ×sin 53.13 ° [Since sin Ɵ=1 and cos Ɵ=0]
=cos 53.13⁰+0
=-0.9619

References
Spivak, M., 2018. Calculus on manifolds: a modern approach to classical theorems of advanced
calculus. CRC Press
Chartrand, G., Polimeni, A.D. and Zhang, P., 2017. Mathematical Proofs: A transition to
advanced mathematics. Pearson