Fixed Income Securities and Interest Rate Modelling | Assignment
VerifiedAdded on 2022/09/12
|8
|342
|16
AI Summary
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Fixed Income Securities and Interest Rate Modelling 1
FIXED INCOME SECURITIES AND INTEREST RATE MODELLING
by Student’s Name
Code + Course Name
Professor’s Name
University Name
City, State
Date
FIXED INCOME SECURITIES AND INTEREST RATE MODELLING
by Student’s Name
Code + Course Name
Professor’s Name
University Name
City, State
Date
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Fixed Income Securities and Interest Rate Modelling 2
Fixed Income Securities and Interest Rate Modelling
1.
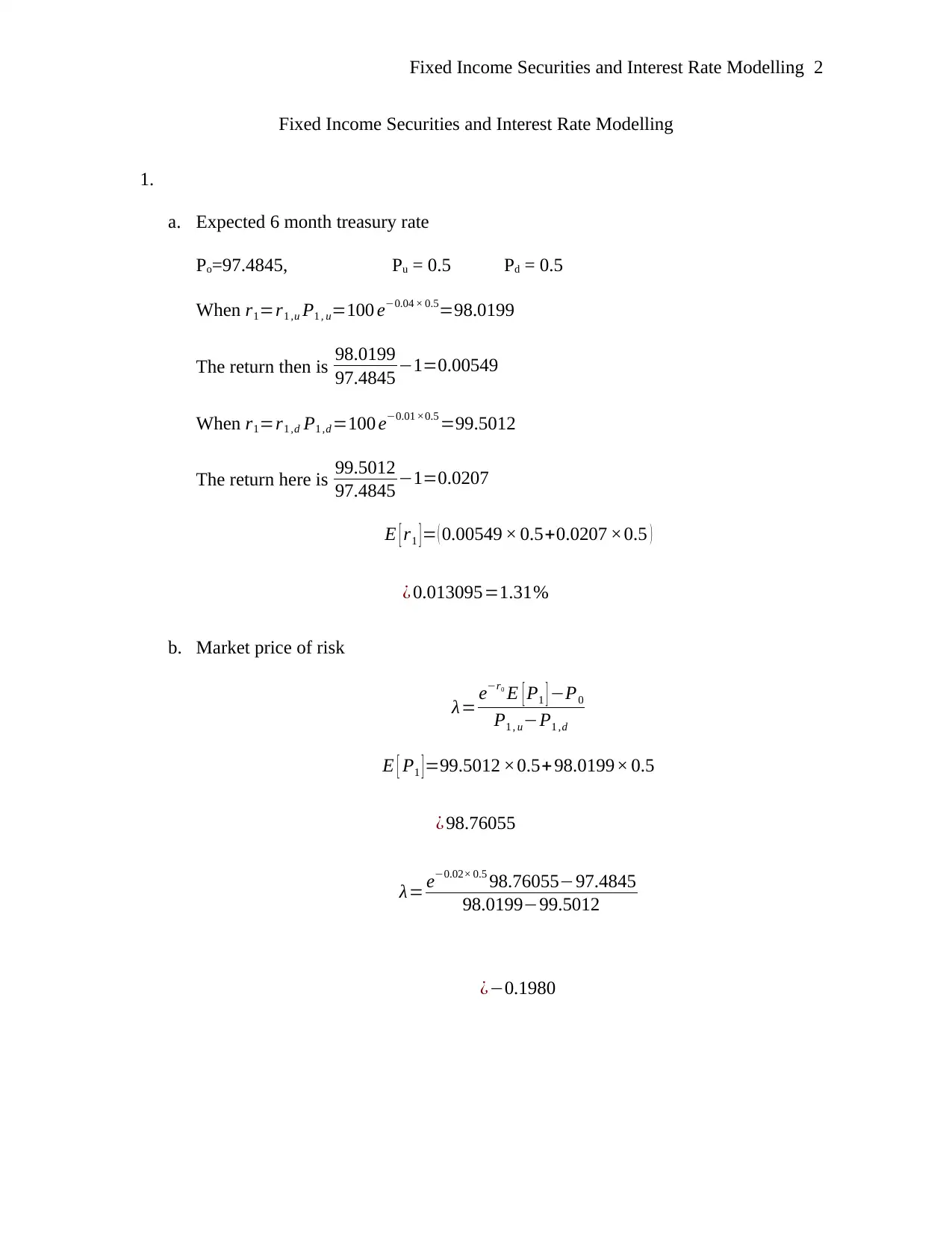
a. Expected 6 month treasury rate
Po=97.4845, Pu = 0.5 Pd = 0.5
When r1=r1 ,u P1 , u=100 e−0.04 × 0.5=98.0199
The return then is 98.0199
97.4845 −1=0.00549
When r1=r1 ,d P1 ,d =100 e−0.01 ×0.5 =99.5012
The return here is 99.5012
97.4845 −1=0.0207
E [ r1 ]= ( 0.00549 × 0.5+0.0207 ×0.5 )
¿ 0.013095=1.31%
b. Market price of risk
λ= e−r0
E [ P1 ] −P0
P1 , u−P1 ,d
E [ P1 ]=99.5012 ×0.5+98.0199× 0.5
¿ 98.76055
λ= e−0.02× 0.5 98.76055−97.4845
98.0199−99.5012
¿−0.1980
Fixed Income Securities and Interest Rate Modelling
1.
a. Expected 6 month treasury rate
Po=97.4845, Pu = 0.5 Pd = 0.5
When r1=r1 ,u P1 , u=100 e−0.04 × 0.5=98.0199
The return then is 98.0199
97.4845 −1=0.00549
When r1=r1 ,d P1 ,d =100 e−0.01 ×0.5 =99.5012
The return here is 99.5012
97.4845 −1=0.0207
E [ r1 ]= ( 0.00549 × 0.5+0.0207 ×0.5 )
¿ 0.013095=1.31%
b. Market price of risk
λ= e−r0
E [ P1 ] −P0
P1 , u−P1 ,d
E [ P1 ]=99.5012 ×0.5+98.0199× 0.5
¿ 98.76055
λ= e−0.02× 0.5 98.76055−97.4845
98.0199−99.5012
¿−0.1980
Fixed Income Securities and Interest Rate Modelling 3
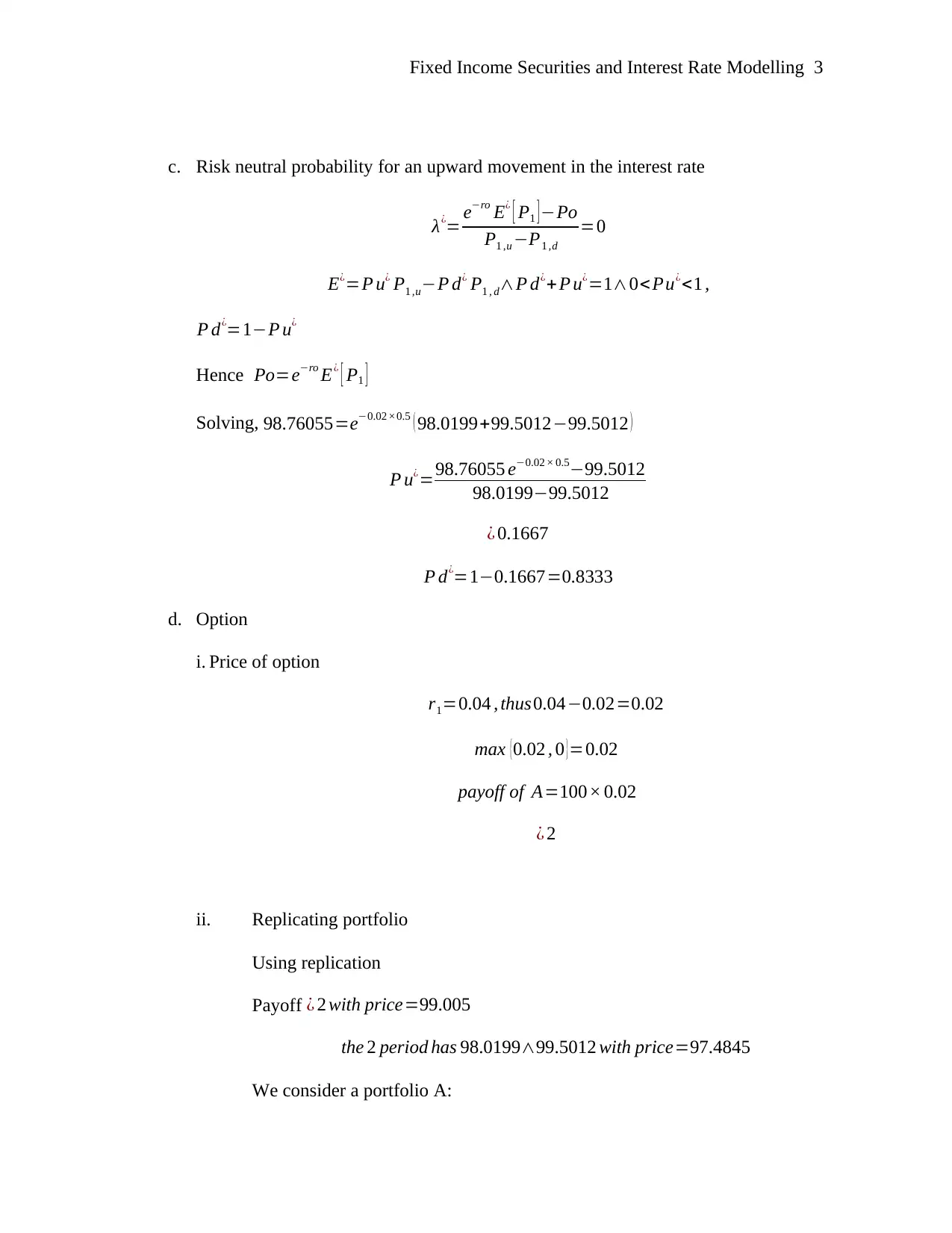
c. Risk neutral probability for an upward movement in the interest rate
λ¿= e−ro E¿ [ P1 ]−Po
P1 ,u −P1 ,d
=0
E¿=P u¿ P1 ,u−P d¿ P1 , d∧P d¿+P u¿=1∧0<Pu¿<1 ,
P d¿=1−P u¿
Hence Po=e−ro E¿ [ P1 ]
Solving, 98.76055=e−0.02 ×0.5 ( 98.0199+99.5012−99.5012 )
P u¿=98.76055 e−0.02 × 0.5−99.5012
98.0199−99.5012
¿ 0.1667
P d¿=1−0.1667=0.8333
d. Option
i. Price of option
r1=0.04 , thus0.04−0.02=0.02
max {0.02 , 0 }=0.02
payoff of A=100× 0.02
¿ 2
ii. Replicating portfolio
Using replication
Payoff ¿ 2 with price=99.005
the 2 period has 98.0199∧99.5012 with price=97.4845
We consider a portfolio A:
c. Risk neutral probability for an upward movement in the interest rate
λ¿= e−ro E¿ [ P1 ]−Po
P1 ,u −P1 ,d
=0
E¿=P u¿ P1 ,u−P d¿ P1 , d∧P d¿+P u¿=1∧0<Pu¿<1 ,
P d¿=1−P u¿
Hence Po=e−ro E¿ [ P1 ]
Solving, 98.76055=e−0.02 ×0.5 ( 98.0199+99.5012−99.5012 )
P u¿=98.76055 e−0.02 × 0.5−99.5012
98.0199−99.5012
¿ 0.1667
P d¿=1−0.1667=0.8333
d. Option
i. Price of option
r1=0.04 , thus0.04−0.02=0.02
max {0.02 , 0 }=0.02
payoff of A=100× 0.02
¿ 2
ii. Replicating portfolio
Using replication
Payoff ¿ 2 with price=99.005
the 2 period has 98.0199∧99.5012 with price=97.4845
We consider a portfolio A:

Fixed Income Securities and Interest Rate Modelling 4
Investing 25.89 and -26.677 of A in the above, we have
¿ 2 ×99.005−2× 97.4845
¿ 3.041
2. Risk-neutral interest rate tree
r0 ¿ 0.05
r1=0.055× 0.5+0.047 × 0.5=0.051
r2=0.06 ×0.5+0.05 × 0.5+0.045 ×0.5=0.1025
a. Interest rate swap at t=0
The fixed rate is 100 ×5 %=5
Float ¿ 5 ×100 ×r2 ( t−1)
The floating leg
t rates float fixed
0 5% 5
1 5.1% 5.0 5
2 10.25% 5.1 5
The swap rate c ¿ 0.5 ( 1−c ) =1
c=1 %
b. Price at t=0
Investing 25.89 and -26.677 of A in the above, we have
¿ 2 ×99.005−2× 97.4845
¿ 3.041
2. Risk-neutral interest rate tree
r0 ¿ 0.05
r1=0.055× 0.5+0.047 × 0.5=0.051
r2=0.06 ×0.5+0.05 × 0.5+0.045 ×0.5=0.1025
a. Interest rate swap at t=0
The fixed rate is 100 ×5 %=5
Float ¿ 5 ×100 ×r2 ( t−1)
The floating leg
t rates float fixed
0 5% 5
1 5.1% 5.0 5
2 10.25% 5.1 5
The swap rate c ¿ 0.5 ( 1−c ) =1
c=1 %
b. Price at t=0
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.

Fixed Income Securities and Interest Rate Modelling 5
K = 100
R = 5%
T = 3 years
P0 ¿ 100 e−0.05 ×3=86.0708
c. Value of the a 3 year callable bond
Par value = P
Coupon = c
Maturity = T
Vt= Ex-coupon price
V t=E¿ [ e−rt ( c +Vt +1 ) ]
VT=P
3. 6 month ZCB FV= 100 price = 100
1 year ZCB FV= 100 price =100
A two step Ho-Lee
r0 =100 e−r0 ×0.5 =98 →r 0=0.04
ru =0.04+∅ 0.5+0.01 √ 0.5
rd =0.04 +∅ 0.5−0.01 √ 0.5=ru −2× 0.01 √ 0.5
96=100 e−r0 × 0.5 [ 0.5 e−r u ×0.5+0.5 e− ( ru ×0.5 ) ×0.5 ]
e−ru × 0.5=
96
100 e−r 0 ×0.5
0.5+0.5 e−2 × 0.01 √0.5 × 0.5 =0.9829
ru =0.0345
K = 100
R = 5%
T = 3 years
P0 ¿ 100 e−0.05 ×3=86.0708
c. Value of the a 3 year callable bond
Par value = P
Coupon = c
Maturity = T
Vt= Ex-coupon price
V t=E¿ [ e−rt ( c +Vt +1 ) ]
VT=P
3. 6 month ZCB FV= 100 price = 100
1 year ZCB FV= 100 price =100
A two step Ho-Lee
r0 =100 e−r0 ×0.5 =98 →r 0=0.04
ru =0.04+∅ 0.5+0.01 √ 0.5
rd =0.04 +∅ 0.5−0.01 √ 0.5=ru −2× 0.01 √ 0.5
96=100 e−r0 × 0.5 [ 0.5 e−r u ×0.5+0.5 e− ( ru ×0.5 ) ×0.5 ]
e−ru × 0.5=
96
100 e−r 0 ×0.5
0.5+0.5 e−2 × 0.01 √0.5 × 0.5 =0.9829
ru =0.0345

Fixed Income Securities and Interest Rate Modelling 6
erd
=0.9829−2× 0.01 √ 0.5=0.9687
rd =0.0636
∅ = 0.0636−.04+0.01 √0.5
0.5 =0.0613
So, ru =0.0345, rd =0.0636, ∅ =0.0613
4. Vasicek interest rate model
With parameters γ= 0.3262, r= 0.0509, σ= 0.0221
risk-neutral process has parameters γ*= 0.4653, r¿= 0.0634
ro= 0.055
a. d rt =γ [ r−rt ] dt+σdWι
Ε [ r1 ]=Ε [ r0 e−γ +r (1−e−γ ) +σ ( γ∗+r¿ ) e− γ
]
¿ 0.055 e−0.3262+ 0.0509 ( 1−e−0.3262 )+ 0.0221 ( 0.4653+ 0.0634 ) e−0.3262
¿ 0.0655
r10=0.055 e−0.3262 ×10 +0.0509 ( 1−e−0.3262 ×10 ) +0.0221 ( 0.4653 e−10+ 0.0634 e−10 ) e−0.3262
¿ 0.050167
The slope shows a decreasing curve, the expectation is that the rates should increase over time
but the observation made show a decreasing curve which may be due to market segmentation
b. Market price
At t = 0;
The market price for 100 nominal will be
erd
=0.9829−2× 0.01 √ 0.5=0.9687
rd =0.0636
∅ = 0.0636−.04+0.01 √0.5
0.5 =0.0613
So, ru =0.0345, rd =0.0636, ∅ =0.0613
4. Vasicek interest rate model
With parameters γ= 0.3262, r= 0.0509, σ= 0.0221
risk-neutral process has parameters γ*= 0.4653, r¿= 0.0634
ro= 0.055
a. d rt =γ [ r−rt ] dt+σdWι
Ε [ r1 ]=Ε [ r0 e−γ +r (1−e−γ ) +σ ( γ∗+r¿ ) e− γ
]
¿ 0.055 e−0.3262+ 0.0509 ( 1−e−0.3262 )+ 0.0221 ( 0.4653+ 0.0634 ) e−0.3262
¿ 0.0655
r10=0.055 e−0.3262 ×10 +0.0509 ( 1−e−0.3262 ×10 ) +0.0221 ( 0.4653 e−10+ 0.0634 e−10 ) e−0.3262
¿ 0.050167
The slope shows a decreasing curve, the expectation is that the rates should increase over time
but the observation made show a decreasing curve which may be due to market segmentation
b. Market price
At t = 0;
The market price for 100 nominal will be
Fixed Income Securities and Interest Rate Modelling 7
100 ( e−0.0655 )
¿ 93.6599
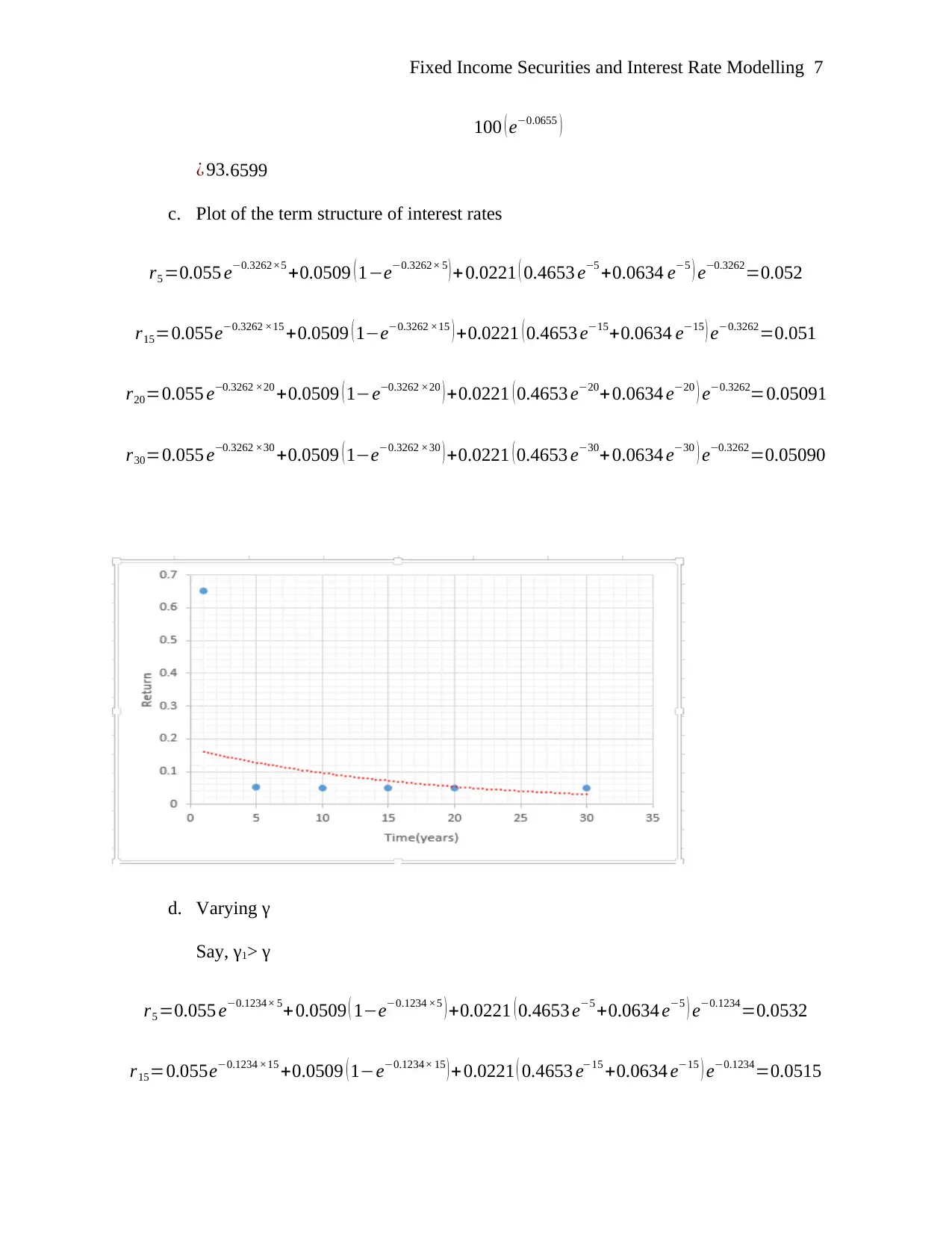
c. Plot of the term structure of interest rates
r5 =0.055 e−0.3262×5 +0.0509 ( 1−e−0.3262× 5 ) + 0.0221 ( 0.4653 e−5 +0.0634 e−5 ) e−0.3262=0.052
r15=0.055e−0.3262 ×15 +0.0509 ( 1−e−0.3262 ×15 ) +0.0221 ( 0.4653 e−15+0.0634 e−15 ) e−0.3262=0.051
r20=0.055 e−0.3262 ×20 +0.0509 ( 1−e−0.3262 ×20 ) +0.0221 ( 0.4653 e−20+0.0634 e−20 ) e−0.3262=0.05091
r30=0.055 e−0.3262 ×30 +0.0509 ( 1−e−0.3262 ×30 ) +0.0221 ( 0.4653 e−30+ 0.0634 e−30 ) e−0.3262=0.05090
d. Varying γ
Say, γ1> γ
r5 =0.055 e−0.1234× 5+ 0.0509 ( 1−e−0.1234 ×5 ) +0.0221 ( 0.4653 e−5 +0.0634 e−5 ) e−0.1234=0.0532
r15=0.055e−0.1234 ×15 +0.0509 ( 1−e−0.1234× 15 ) + 0.0221 ( 0.4653 e−15 +0.0634 e−15 ) e−0.1234=0.0515
100 ( e−0.0655 )
¿ 93.6599
c. Plot of the term structure of interest rates
r5 =0.055 e−0.3262×5 +0.0509 ( 1−e−0.3262× 5 ) + 0.0221 ( 0.4653 e−5 +0.0634 e−5 ) e−0.3262=0.052
r15=0.055e−0.3262 ×15 +0.0509 ( 1−e−0.3262 ×15 ) +0.0221 ( 0.4653 e−15+0.0634 e−15 ) e−0.3262=0.051
r20=0.055 e−0.3262 ×20 +0.0509 ( 1−e−0.3262 ×20 ) +0.0221 ( 0.4653 e−20+0.0634 e−20 ) e−0.3262=0.05091
r30=0.055 e−0.3262 ×30 +0.0509 ( 1−e−0.3262 ×30 ) +0.0221 ( 0.4653 e−30+ 0.0634 e−30 ) e−0.3262=0.05090
d. Varying γ
Say, γ1> γ
r5 =0.055 e−0.1234× 5+ 0.0509 ( 1−e−0.1234 ×5 ) +0.0221 ( 0.4653 e−5 +0.0634 e−5 ) e−0.1234=0.0532
r15=0.055e−0.1234 ×15 +0.0509 ( 1−e−0.1234× 15 ) + 0.0221 ( 0.4653 e−15 +0.0634 e−15 ) e−0.1234=0.0515
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Fixed Income Securities and Interest Rate Modelling 8
The rates are increasing with an increasing γ.
e. Varying r
Taking a greater r say 0.065>0.05091
r5 =0.055 e−0.3262×5 +0.065 ( 1−e−0.3262× 5 )+ 0.0221 ( 0.4653 e−5 +0.0634 e−5 ) e−0.3262=0.0596
r15=0.055e−0.3262 ×15 +0.065 ( 1−e−0.3262 ×15 ) +0.0221 ( 0.4653 e−15+0.0634 e−15 ) e−0.3262=0.06343
The rates are increasing with an increasing r
f. Varying σ
Taking a greater σ, say 0.035>0.0221
r5 =0.055 e−0.3262×5 +0.0509 ( 1−e−0.3262× 5 ) + 0.035 ( 0.4653 e−5+ 0.0634 e−5 ) e−0.3262=0.05322
r15=0.055e−0.3262 ×15 +0.0509 ( 1−e−0.3262 ×15 ) +0.035 ( 0.4653 e−15+0.0634 e−15 ) e−0.3262=0.0725
An increasing σ, leads to increased return rates.
The rates are increasing with an increasing γ.
e. Varying r
Taking a greater r say 0.065>0.05091
r5 =0.055 e−0.3262×5 +0.065 ( 1−e−0.3262× 5 )+ 0.0221 ( 0.4653 e−5 +0.0634 e−5 ) e−0.3262=0.0596
r15=0.055e−0.3262 ×15 +0.065 ( 1−e−0.3262 ×15 ) +0.0221 ( 0.4653 e−15+0.0634 e−15 ) e−0.3262=0.06343
The rates are increasing with an increasing r
f. Varying σ
Taking a greater σ, say 0.035>0.0221
r5 =0.055 e−0.3262×5 +0.0509 ( 1−e−0.3262× 5 ) + 0.035 ( 0.4653 e−5+ 0.0634 e−5 ) e−0.3262=0.05322
r15=0.055e−0.3262 ×15 +0.0509 ( 1−e−0.3262 ×15 ) +0.035 ( 0.4653 e−15+0.0634 e−15 ) e−0.3262=0.0725
An increasing σ, leads to increased return rates.
1 out of 8
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.


