MAF101: Fundamentals of Finance Assignment Solution
VerifiedAdded on 2023/06/07
|13
|2763
|378
Homework Assignment
AI Summary
This document presents a comprehensive solution to a Fundamentals of Finance assignment, addressing key financial concepts. The solution begins with calculations related to annuities and future value, applied to a savings plan. It then delves into bond valuation, calculating bond prices under diff...

FUNDAMENTALS OF FINANCE
[Type the document subtitle]
Student id/ name
[Pick the date]
[Type the document subtitle]
Student id/ name
[Pick the date]
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Question 1
PART A
(i) The savings would be in the form of annuity with payments being made at the end.
Annuity payment = 0.4*(91000/12) = $ 3,033.33
Total duration of annuity = 5 years or 5*12 = 60 months
Interest rate applicable = 2% p.a. or (2/12) or 0.16667% per month
The relevant formula to be applied pertains to future value of annuity.
Here, P = $3,033.33, n = 60 and r = 0.16667%
Thus, FV = 3033.33*(1.001666760-1)/0.0016667 = $ 191,243.6
(ii) The relevant formula to be applied is shown below.
FV = PV (1+r)n
PV = current value of house = $ 700,000
R= Rate of increase = 6% p.a.
N – Time period = 5 years
Hence, expected price of house after 5 years = 700000*1.065 = $ 936,757.9
Down-payment is 20% of the cost or 0.2*936,757.9 = $187,351.6
Since the amount computed in part (i) exceeds the above amount, hence it can be concluded
that the deposit requirement would be met.
(iii) Expected price of the house at the end of 5 years = $ 936,757.9
Deposit amount = (20/100)* 936,757.9 = $187,351.6
Hence, required loan from the bank = $ 936,757.9 - $187,351.6 = $749,406.32
PART B
i) Principal of bank loan (P)= $749,406.32
1
PART A
(i) The savings would be in the form of annuity with payments being made at the end.
Annuity payment = 0.4*(91000/12) = $ 3,033.33
Total duration of annuity = 5 years or 5*12 = 60 months
Interest rate applicable = 2% p.a. or (2/12) or 0.16667% per month
The relevant formula to be applied pertains to future value of annuity.
Here, P = $3,033.33, n = 60 and r = 0.16667%
Thus, FV = 3033.33*(1.001666760-1)/0.0016667 = $ 191,243.6
(ii) The relevant formula to be applied is shown below.
FV = PV (1+r)n
PV = current value of house = $ 700,000
R= Rate of increase = 6% p.a.
N – Time period = 5 years
Hence, expected price of house after 5 years = 700000*1.065 = $ 936,757.9
Down-payment is 20% of the cost or 0.2*936,757.9 = $187,351.6
Since the amount computed in part (i) exceeds the above amount, hence it can be concluded
that the deposit requirement would be met.
(iii) Expected price of the house at the end of 5 years = $ 936,757.9
Deposit amount = (20/100)* 936,757.9 = $187,351.6
Hence, required loan from the bank = $ 936,757.9 - $187,351.6 = $749,406.32
PART B
i) Principal of bank loan (P)= $749,406.32
1

Maturity of the loan (N) = 30 years or (30*12) = 360 months
Interest rate (R) = 5.5% p.a. or (5.5/12) % or 0.4583% per month
The formula for EMI computation is shown below.
EMI = P*R*(1+R)N/((1+R)N-1))
Substituting the given values, we get
EMI = (749,406.32*0.004583*1.004583360)/(1.004583360 -1) = $4,255.04
Monthly salary of Christine after promotion = (145000/12) = $ 12,083.33
Monthly savings = 0.4*12,083.33 = $ 4,833.33
Clearly, the monthly savings by Christine would be sufficient to pay the monthly instalment
on the bank loan.
(ii) Total repayment made by Christine during 30 years along with interest = $4,255.04*360
= $1,531,816.80
Amount of interest paid = Total repayment – Principal borrowed = 1,531,816.80 - 749,406.32
= $ 782,410.47
PART C
(i) The revised duration of the loan = 15 years or 15*12 = 180 months
The formula for EMI computation is shown below.
EMI = P*R*(1+R)N/((1+R)N-1))
Substituting the new values, we get
EMI = (749,406.32*0.004583*1.004583180)/(1.004583180 -1) = $6,123.28
(ii) Total repayment made by Christine during 15 years along with interest = $6,123.28*180
= $ 1,102,189.51
Amount of interest paid = Total repayment – Principal borrowed = 1,102,189.51- 749,406.32
= $ 352,783.19
(iii) Monthly salary of Christine after promotion = (145000/12) = $ 12,083.33
2
Interest rate (R) = 5.5% p.a. or (5.5/12) % or 0.4583% per month
The formula for EMI computation is shown below.
EMI = P*R*(1+R)N/((1+R)N-1))
Substituting the given values, we get
EMI = (749,406.32*0.004583*1.004583360)/(1.004583360 -1) = $4,255.04
Monthly salary of Christine after promotion = (145000/12) = $ 12,083.33
Monthly savings = 0.4*12,083.33 = $ 4,833.33
Clearly, the monthly savings by Christine would be sufficient to pay the monthly instalment
on the bank loan.
(ii) Total repayment made by Christine during 30 years along with interest = $4,255.04*360
= $1,531,816.80
Amount of interest paid = Total repayment – Principal borrowed = 1,531,816.80 - 749,406.32
= $ 782,410.47
PART C
(i) The revised duration of the loan = 15 years or 15*12 = 180 months
The formula for EMI computation is shown below.
EMI = P*R*(1+R)N/((1+R)N-1))
Substituting the new values, we get
EMI = (749,406.32*0.004583*1.004583180)/(1.004583180 -1) = $6,123.28
(ii) Total repayment made by Christine during 15 years along with interest = $6,123.28*180
= $ 1,102,189.51
Amount of interest paid = Total repayment – Principal borrowed = 1,102,189.51- 749,406.32
= $ 352,783.19
(iii) Monthly salary of Christine after promotion = (145000/12) = $ 12,083.33
2
You're viewing a preview
Unlock full access by subscribing today!

Monthly savings = 0.4*12,083.33 = $ 4,833.33
Clearly, the monthly savings is lesser than the EMI of $ 6,123.28 and hence Christine would
not be able to pay the new EMI if she decides to save only 40%.
PART D
(i) Extra monthly cash flow from tenants = $ 2,200
Extra living expenses payable to parents per month = $ 1,000
Hence, incremental cash available each month = 2200-1000 = $1,200
Monthly savings from salary = 0.4*12,083.33 = $ 4,833.33
Total available cash = 4833.33 + 1200 = $ 6,033.33
Clearly, the EMI amount in case of 15 year loan would still be greater and hence Christine
would not be able to meet the same.
(ii) Since, she would not be able to meet the EMI for a 15 year old, hence Christine must not
accept the offer.
Question 2
(a)Bonds A, B and C would be trading at a premium to their par value. This is because the
coupon rate offered by these bonds tends to exceed the respective yield. On the other hand,
Bond D would be trading at a discount to the par value since the coupon rate offered by
this bond is lower than the respective yield (Arnold, 2015).
(b)The current price of the bonds would be equal to the present value of the principal and all
the coupon payments.
Bond A
Face value = $ 100
Maturity period = 5 years
Coupon payment = 2.75% *100 = $2.75 pa. paid semi-annually
The given yield = 2.33% p.a. or (2.33/2) or 1.165% per half year
The applicable formula is shown below.
3
Clearly, the monthly savings is lesser than the EMI of $ 6,123.28 and hence Christine would
not be able to pay the new EMI if she decides to save only 40%.
PART D
(i) Extra monthly cash flow from tenants = $ 2,200
Extra living expenses payable to parents per month = $ 1,000
Hence, incremental cash available each month = 2200-1000 = $1,200
Monthly savings from salary = 0.4*12,083.33 = $ 4,833.33
Total available cash = 4833.33 + 1200 = $ 6,033.33
Clearly, the EMI amount in case of 15 year loan would still be greater and hence Christine
would not be able to meet the same.
(ii) Since, she would not be able to meet the EMI for a 15 year old, hence Christine must not
accept the offer.
Question 2
(a)Bonds A, B and C would be trading at a premium to their par value. This is because the
coupon rate offered by these bonds tends to exceed the respective yield. On the other hand,
Bond D would be trading at a discount to the par value since the coupon rate offered by
this bond is lower than the respective yield (Arnold, 2015).
(b)The current price of the bonds would be equal to the present value of the principal and all
the coupon payments.
Bond A
Face value = $ 100
Maturity period = 5 years
Coupon payment = 2.75% *100 = $2.75 pa. paid semi-annually
The given yield = 2.33% p.a. or (2.33/2) or 1.165% per half year
The applicable formula is shown below.
3
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Here, C = $ 1.375, I = 1.165%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=$ 101.97
Bond B
Face value = $ 100
Maturity period = 7 years
Coupon payment = 3.25% *100 = $3.25 pa paid semi-annually
The given yield = 2.38% p.a. or (2.38/2) or 1.19% per half year
The applicable formula is shown below.
Here, C = $ 1.625, I = 1.19%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=$ 105.58
Bond C
Face value = $ 100
Maturity period = 10 years
Coupon payment = 3.25% *100 = $3.25 pa. paid semi-annually
The given yield = 2.58% p.a. or (2.58/2) or 1.29% per half year
The applicable formula is shown below.
4
Bond price for A=1.375∗¿ ¿
Bond price for A=$ 101.97
Bond B
Face value = $ 100
Maturity period = 7 years
Coupon payment = 3.25% *100 = $3.25 pa paid semi-annually
The given yield = 2.38% p.a. or (2.38/2) or 1.19% per half year
The applicable formula is shown below.
Here, C = $ 1.625, I = 1.19%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=$ 105.58
Bond C
Face value = $ 100
Maturity period = 10 years
Coupon payment = 3.25% *100 = $3.25 pa. paid semi-annually
The given yield = 2.58% p.a. or (2.58/2) or 1.29% per half year
The applicable formula is shown below.
4

Here, C = $ 1.625, I = 1.29%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=$ 105.87
Bond D
Face value = $ 100
Maturity period = 20 years
Coupon payment = 2.75% *100 = $2.75pa paid semi-annually
The given yield = 2.88% p.a. or (2.88/2) or 1.44% per half year
The applicable formula is shown below.
Here, C = $ 1.375, I = 1.44%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=$ 98.03
(c) Let the market interest rate has increased across all the four bonds by 1%.
Bond A
Here, C = $ 1.375, I = 1.165% + 0.5% = 1.67%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=$ 97.35
.
Bond B
Here, C = $ 1.625, I = 1.19%+ 0.5%=1.69%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=$ 99.20
5
Bond price for C=1.625∗¿ ¿
Bond price for C=$ 105.87
Bond D
Face value = $ 100
Maturity period = 20 years
Coupon payment = 2.75% *100 = $2.75pa paid semi-annually
The given yield = 2.88% p.a. or (2.88/2) or 1.44% per half year
The applicable formula is shown below.
Here, C = $ 1.375, I = 1.44%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=$ 98.03
(c) Let the market interest rate has increased across all the four bonds by 1%.
Bond A
Here, C = $ 1.375, I = 1.165% + 0.5% = 1.67%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=$ 97.35
.
Bond B
Here, C = $ 1.625, I = 1.19%+ 0.5%=1.69%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=$ 99.20
5
You're viewing a preview
Unlock full access by subscribing today!
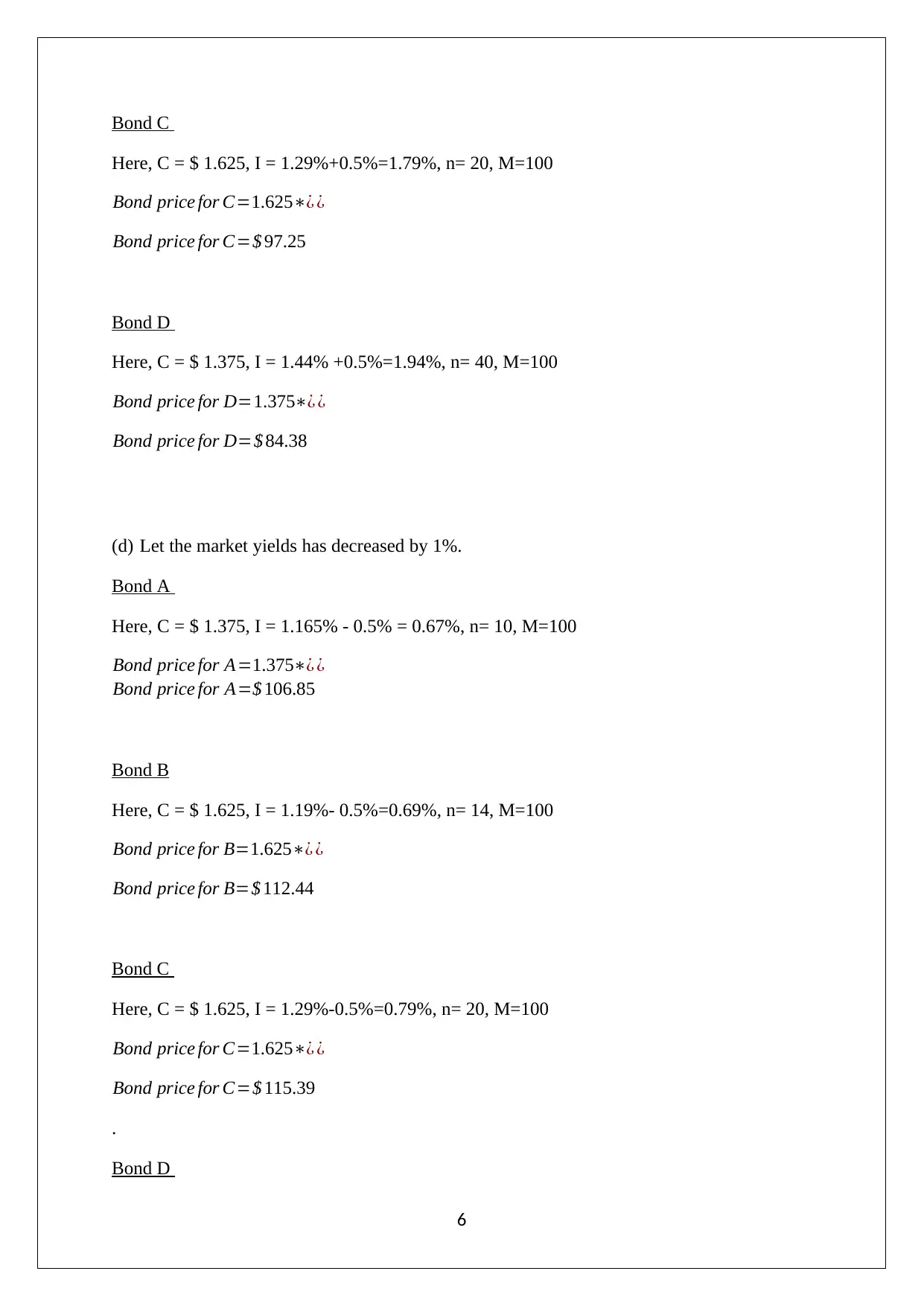
Bond C
Here, C = $ 1.625, I = 1.29%+0.5%=1.79%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=$ 97.25
Bond D
Here, C = $ 1.375, I = 1.44% +0.5%=1.94%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=$ 84.38
(d) Let the market yields has decreased by 1%.
Bond A
Here, C = $ 1.375, I = 1.165% - 0.5% = 0.67%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=$ 106.85
Bond B
Here, C = $ 1.625, I = 1.19%- 0.5%=0.69%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=$ 112.44
Bond C
Here, C = $ 1.625, I = 1.29%-0.5%=0.79%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=$ 115.39
.
Bond D
6
Here, C = $ 1.625, I = 1.29%+0.5%=1.79%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=$ 97.25
Bond D
Here, C = $ 1.375, I = 1.44% +0.5%=1.94%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=$ 84.38
(d) Let the market yields has decreased by 1%.
Bond A
Here, C = $ 1.375, I = 1.165% - 0.5% = 0.67%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=$ 106.85
Bond B
Here, C = $ 1.625, I = 1.19%- 0.5%=0.69%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=$ 112.44
Bond C
Here, C = $ 1.625, I = 1.29%-0.5%=0.79%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=$ 115.39
.
Bond D
6
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
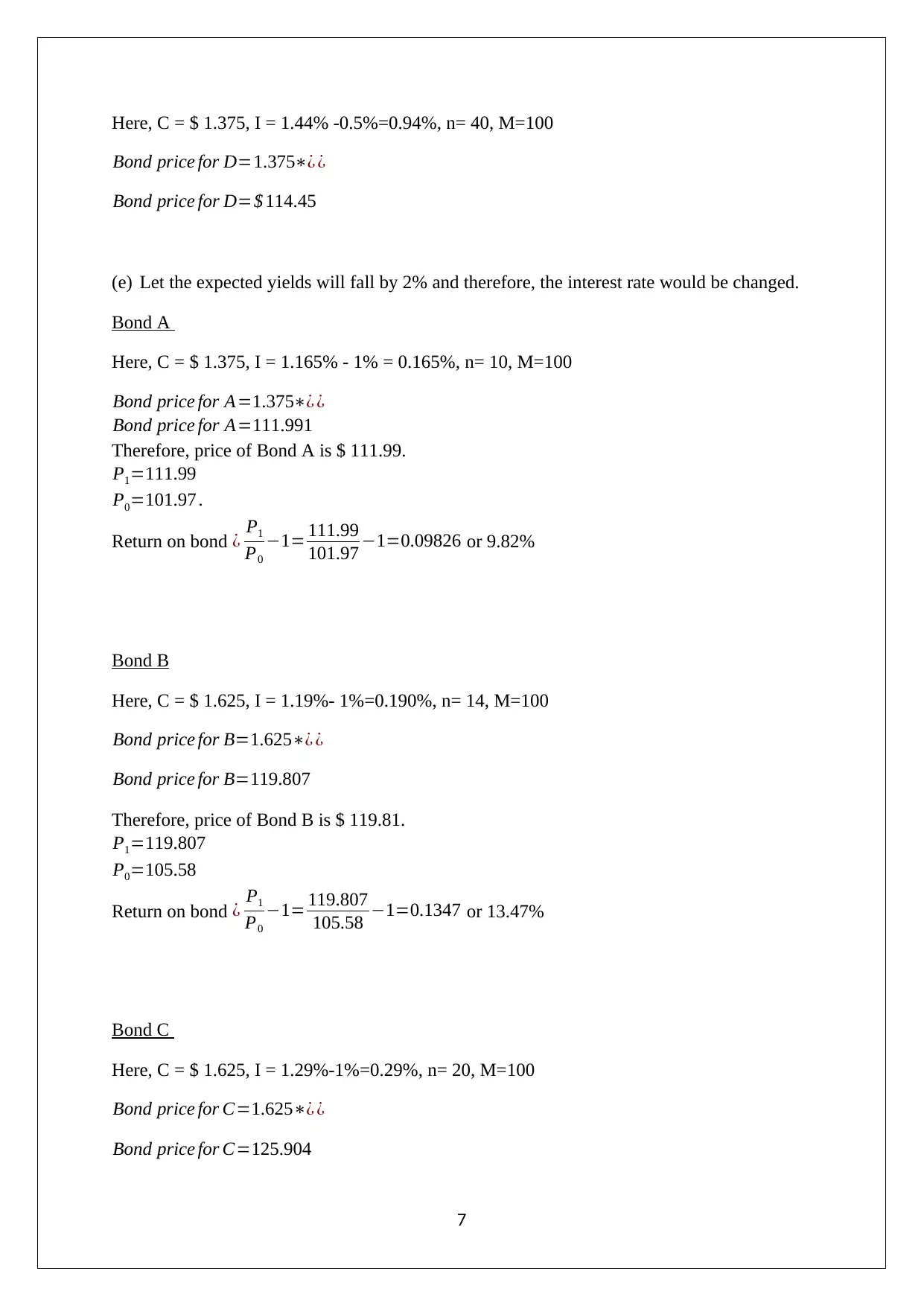
Here, C = $ 1.375, I = 1.44% -0.5%=0.94%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=$ 114.45
(e) Let the expected yields will fall by 2% and therefore, the interest rate would be changed.
Bond A
Here, C = $ 1.375, I = 1.165% - 1% = 0.165%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=111.991
Therefore, price of Bond A is $ 111.99.
P1=111.99
P0=101.97 .
Return on bond ¿ P1
P0
−1= 111.99
101.97 −1=0.09826 or 9.82%
Bond B
Here, C = $ 1.625, I = 1.19%- 1%=0.190%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=119.807
Therefore, price of Bond B is $ 119.81.
P1=119.807
P0=105.58
Return on bond ¿ P1
P0
−1= 119.807
105.58 −1=0.1347 or 13.47%
Bond C
Here, C = $ 1.625, I = 1.29%-1%=0.29%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=125.904
7
Bond price for D=1.375∗¿ ¿
Bond price for D=$ 114.45
(e) Let the expected yields will fall by 2% and therefore, the interest rate would be changed.
Bond A
Here, C = $ 1.375, I = 1.165% - 1% = 0.165%, n= 10, M=100
Bond price for A=1.375∗¿ ¿
Bond price for A=111.991
Therefore, price of Bond A is $ 111.99.
P1=111.99
P0=101.97 .
Return on bond ¿ P1
P0
−1= 111.99
101.97 −1=0.09826 or 9.82%
Bond B
Here, C = $ 1.625, I = 1.19%- 1%=0.190%, n= 14, M=100
Bond price for B=1.625∗¿ ¿
Bond price for B=119.807
Therefore, price of Bond B is $ 119.81.
P1=119.807
P0=105.58
Return on bond ¿ P1
P0
−1= 119.807
105.58 −1=0.1347 or 13.47%
Bond C
Here, C = $ 1.625, I = 1.29%-1%=0.29%, n= 20, M=100
Bond price for C=1.625∗¿ ¿
Bond price for C=125.904
7

Therefore, price of bond C is $ 125.90.
P1=125.90
P0=105.872
Return on bond ¿ P1
P0
−1= 125.90
105.872−1=0.1891 or 18.91%
Bond D
Here, C = $ 1.375, I = 1.44% -1%=0.44%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=134.225
Therefore, price of bond D is $ 134.22
P1=134.22
P0=98.03
Return on bond ¿ P1
P0
−1= 134.22
98.03 −1=0.3691 or 36.91%
Maximum return on bond has observed in case of bond D and hence, it can be concluded that
it would be most profitable to purchase bond D.
(f) Risk free implies when there is no risk of default on the underlying payments. This is
taken as a reference using which the applicable rates for risky clients is computed. The
government debt is considered to be risk free as the risk of the government defaulting
would be zero. This is because of the underlying resources available with the government
coupled with the underlying credibility. Risk free government debt is essentially
applicable to governments having AAA credit rating (Damodaran, 2015).
Question 3
(a) The relevant screenshot is highlighted as follows (Northington, 2015).
8
P1=125.90
P0=105.872
Return on bond ¿ P1
P0
−1= 125.90
105.872−1=0.1891 or 18.91%
Bond D
Here, C = $ 1.375, I = 1.44% -1%=0.44%, n= 40, M=100
Bond price for D=1.375∗¿ ¿
Bond price for D=134.225
Therefore, price of bond D is $ 134.22
P1=134.22
P0=98.03
Return on bond ¿ P1
P0
−1= 134.22
98.03 −1=0.3691 or 36.91%
Maximum return on bond has observed in case of bond D and hence, it can be concluded that
it would be most profitable to purchase bond D.
(f) Risk free implies when there is no risk of default on the underlying payments. This is
taken as a reference using which the applicable rates for risky clients is computed. The
government debt is considered to be risk free as the risk of the government defaulting
would be zero. This is because of the underlying resources available with the government
coupled with the underlying credibility. Risk free government debt is essentially
applicable to governments having AAA credit rating (Damodaran, 2015).
Question 3
(a) The relevant screenshot is highlighted as follows (Northington, 2015).
8
You're viewing a preview
Unlock full access by subscribing today!
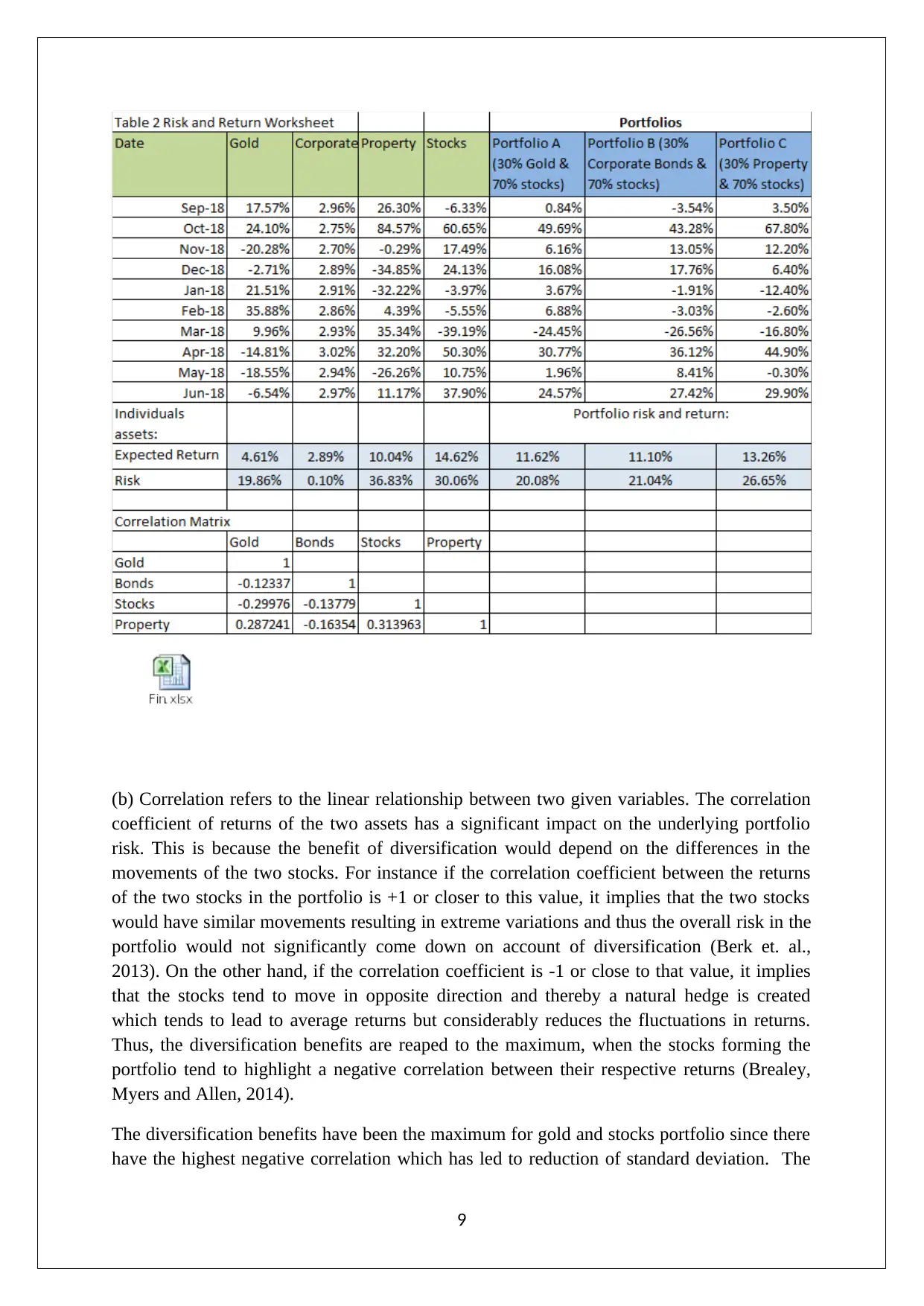
(b) Correlation refers to the linear relationship between two given variables. The correlation
coefficient of returns of the two assets has a significant impact on the underlying portfolio
risk. This is because the benefit of diversification would depend on the differences in the
movements of the two stocks. For instance if the correlation coefficient between the returns
of the two stocks in the portfolio is +1 or closer to this value, it implies that the two stocks
would have similar movements resulting in extreme variations and thus the overall risk in the
portfolio would not significantly come down on account of diversification (Berk et. al.,
2013). On the other hand, if the correlation coefficient is -1 or close to that value, it implies
that the stocks tend to move in opposite direction and thereby a natural hedge is created
which tends to lead to average returns but considerably reduces the fluctuations in returns.
Thus, the diversification benefits are reaped to the maximum, when the stocks forming the
portfolio tend to highlight a negative correlation between their respective returns (Brealey,
Myers and Allen, 2014).
The diversification benefits have been the maximum for gold and stocks portfolio since there
have the highest negative correlation which has led to reduction of standard deviation. The
9
coefficient of returns of the two assets has a significant impact on the underlying portfolio
risk. This is because the benefit of diversification would depend on the differences in the
movements of the two stocks. For instance if the correlation coefficient between the returns
of the two stocks in the portfolio is +1 or closer to this value, it implies that the two stocks
would have similar movements resulting in extreme variations and thus the overall risk in the
portfolio would not significantly come down on account of diversification (Berk et. al.,
2013). On the other hand, if the correlation coefficient is -1 or close to that value, it implies
that the stocks tend to move in opposite direction and thereby a natural hedge is created
which tends to lead to average returns but considerably reduces the fluctuations in returns.
Thus, the diversification benefits are reaped to the maximum, when the stocks forming the
portfolio tend to highlight a negative correlation between their respective returns (Brealey,
Myers and Allen, 2014).
The diversification benefits have been the maximum for gold and stocks portfolio since there
have the highest negative correlation which has led to reduction of standard deviation. The
9
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

diversification benefits would be comparatively lesser for property and stocks portfolio since
the underlying correlation is positive for these asset classes (Petty et. al., 2015).
(c ) Diversification refers to the formation of a portfolio which tends to include different type
of assets or stocks. In finance, diversification is recommended for reduction of unsystematic
risk related to the portfolio. This typically happens since in a well-diversified portfolio, the
risk associated with a particular asset class or industry may be hedged by corresponding
movements in the other asset class or industry. For instance, gold and stocks tend to have
negative correlation and hence a diversified portfolio containing both these asset classes
would result in lower risk both assets would act as natural hedges to each other (Parrino and
Kidwell, 2014).
In case of the given portfolio, diversification has led to reduced risk which is apparent from
the value of the standard deviations of the portfolios when compared with the original asset
classes. Further, the returns have also reduced in comparison with the highest return asset.
However, it is noteworthy that the return per unit risk has actually improved which augers
well for the investors (Brealey, Myers and Allen, 2014).
(d) Risk aversion implies that the investors would tend to avoid risk and thus given two
investments with same returns, they would choose the investment having lower risk. Thus,
whenever there is uncertainty, the investors take step to reduce the same as the investors are
averse to risk Further, it also implies that in order to invest in a risky investment, the
investors would have to be given incentive in the form of higher returns. Thus, higher the
perceived risk associated with an investment, higher would be the demanded returns
(Damodaran, 2015). The risk averse nature of human beings tends to form the basis of
modern portfolio theory which is based on the central tenet that risk and return are correlated.
In real life also, it is seen that the returns associated with debt tend to be lower than those
compared with equity. The prime reason for the same is that lower risk is associated with
debt in comparison with equity (Petty et. al., 2015).
(e) In order to select the best portfolio from the given three choices, it is essential to
determine the return per unit risk for all the three portfolios (Arnold, 2015). This is carried
out below.
Portfolio A = 11.62/20.08 = 0.58
Portfolio B = 11.10/21.04 = 0.53
Portfolio C = 13.26/26.65 = 0.50
It may be concluded that Portfolio A would be the best choice.
10
the underlying correlation is positive for these asset classes (Petty et. al., 2015).
(c ) Diversification refers to the formation of a portfolio which tends to include different type
of assets or stocks. In finance, diversification is recommended for reduction of unsystematic
risk related to the portfolio. This typically happens since in a well-diversified portfolio, the
risk associated with a particular asset class or industry may be hedged by corresponding
movements in the other asset class or industry. For instance, gold and stocks tend to have
negative correlation and hence a diversified portfolio containing both these asset classes
would result in lower risk both assets would act as natural hedges to each other (Parrino and
Kidwell, 2014).
In case of the given portfolio, diversification has led to reduced risk which is apparent from
the value of the standard deviations of the portfolios when compared with the original asset
classes. Further, the returns have also reduced in comparison with the highest return asset.
However, it is noteworthy that the return per unit risk has actually improved which augers
well for the investors (Brealey, Myers and Allen, 2014).
(d) Risk aversion implies that the investors would tend to avoid risk and thus given two
investments with same returns, they would choose the investment having lower risk. Thus,
whenever there is uncertainty, the investors take step to reduce the same as the investors are
averse to risk Further, it also implies that in order to invest in a risky investment, the
investors would have to be given incentive in the form of higher returns. Thus, higher the
perceived risk associated with an investment, higher would be the demanded returns
(Damodaran, 2015). The risk averse nature of human beings tends to form the basis of
modern portfolio theory which is based on the central tenet that risk and return are correlated.
In real life also, it is seen that the returns associated with debt tend to be lower than those
compared with equity. The prime reason for the same is that lower risk is associated with
debt in comparison with equity (Petty et. al., 2015).
(e) In order to select the best portfolio from the given three choices, it is essential to
determine the return per unit risk for all the three portfolios (Arnold, 2015). This is carried
out below.
Portfolio A = 11.62/20.08 = 0.58
Portfolio B = 11.10/21.04 = 0.53
Portfolio C = 13.26/26.65 = 0.50
It may be concluded that Portfolio A would be the best choice.
10

References
Arnold, G. (2015) Corporate Financial Management. 3rd ed. Sydney: Financial Times
Management.
Berk, J., DeMarzo, P., Harford, J., Ford, G., Mollica, V., & Finch, N. (2013) Fundamentals of
corporate finance, London: Pearson Higher Education
Brealey, R. A., Myers, S. C., & Allen, F. (2014) Principles of corporate finance, 2nd ed. New
York: McGraw-Hill Inc.
Damodaran, A. (2015). Applied corporate finance: A user’s manual 3rd ed. New York:
Wiley, John & Sons.
Northington, S. (2015) Finance, 4th ed. New York: Ferguson
11
Arnold, G. (2015) Corporate Financial Management. 3rd ed. Sydney: Financial Times
Management.
Berk, J., DeMarzo, P., Harford, J., Ford, G., Mollica, V., & Finch, N. (2013) Fundamentals of
corporate finance, London: Pearson Higher Education
Brealey, R. A., Myers, S. C., & Allen, F. (2014) Principles of corporate finance, 2nd ed. New
York: McGraw-Hill Inc.
Damodaran, A. (2015). Applied corporate finance: A user’s manual 3rd ed. New York:
Wiley, John & Sons.
Northington, S. (2015) Finance, 4th ed. New York: Ferguson
11
You're viewing a preview
Unlock full access by subscribing today!

Parrino, R. and Kidwell, D. (2014) Fundamentals of Corporate Finance, 3rd ed. London:
Wiley Publications
Petty, J.W., Titman, S., Keown, A., Martin, J.D., Martin, P., Burrow, M., & Nguyen, H. (2015).
Financial Management, Principles and Applications, 6th ed.. NSW: Pearson Education, French
Forest Australia
12
Wiley Publications
Petty, J.W., Titman, S., Keown, A., Martin, J.D., Martin, P., Burrow, M., & Nguyen, H. (2015).
Financial Management, Principles and Applications, 6th ed.. NSW: Pearson Education, French
Forest Australia
12
1 out of 13
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.




