Fundamentals of Working Mathematically

This document explores the fundamentals of working mathematically through tutorials and investigations on Yowie, Dice Difference, and Creepy Critters. It includes descriptions of the activities, mathematical calculations, and extensions of the investigations. The document also discusses the use of mathematics and mathematical language in each activity.

FUNDAMENTALS OF
WORKING
MATHEMATICALLY

TABLE OF CONTENTS
MAIN BODY..................................................................................................................................3
TUTORIAL INVESTIGATION 1: YOWIE...................................................................................3
Description of the activity...........................................................................................................3
Extending the investigation.........................................................................................................3
Mathematics and mathematical language used............................................................................4
TUTORIAL INVESTIGATION 3: DICE DIFFERENCE..............................................................5
Description of the activity...........................................................................................................5
Extending the investigation.........................................................................................................6
Mathematics and mathematical language used............................................................................6
TUTORIAL INVESTIGATION 4: CREEPY CRITTERS.............................................................7
Description of the activity...........................................................................................................7
Extending the investigation.........................................................................................................8
Mathematics and mathematical language used............................................................................8
REFLECTION.................................................................................................................................9
REFERENCES..............................................................................................................................11

MAIN BODY
TUTORIAL INVESTIGATION 1: YOWIE
Description of the activity
The Yowie measurement activity is basically an activity to measure different aspects
related to humans and take measurements of their different body parts. This activity basically
originated from the measurements of the Yowie animals which are giant gorilla like creatures
that walk on their two feet (Tuniz, Gillespie & Jones, 2016). In this mathematical activity as well
the different body parts of all the members of the activity have been recorded. During our
solutions that we derived, we identified that the arm span was exactly equal to the height of all
the four individuals. This was concluded that the fact that a person’s height is equal to their arm
was proved o be true. We calculated our stride by stooping over and then measuring foot to foot.
We then added both the numbers and then divided them by 2 which was the number of
strides that we took. The formulas that we used to calculate the stride was:
= Add all the measurements from one foot to another / divide by the number of strides that were
taken
This helped us in ascertaining correct stride of the Yowie if they were assumed to be
walking. Additionally, in order to ascertain Yowie measurements for all the factors we totalled
up the data of all the members and divided it by 4 because we were four members (Townsend,
2016). The formula that we used for it was:
= Addition of all the measurements / number of people measured.
The fact that scientists again measured the Yowie footprint and found it to be 1 cm long
i.e. rather than the earlier estimate of a little over 43 cm, the actual length is 44 cm affects the
entire done on the Yowie heavily. Scientists were basing their earlier research on the basis of this
particular measurement i.e. the height and estimated weight.
Extending the investigation
There are some major questions that can lead to further extension of the study that has been
undertaken in the form of Yowie measurements. The main question that arises is that the faulty
measurement of the footprint of Yowie i.e. 43 cm as compared to the new measurements of 44
cm presents a major case of extension for this study (Alyazichi, Jones & McLean, 2015). The
scientists have already been building up on the past data and they have derived various other
details related to Yowie based on this specific calculation but all the research has been proved to
3

be fruitless due to the new discovery that has been made regarding the measurements. The
further are of extension or research in this field is that whether the fact that the arm span of the
hand is equivalent t to the height of an individual is true in case of Yowie as well or not. This can
also be evaluated because in humans this is a truly proven fact that has been tested by us as well
but the same can be evaluated in context of humans as well. Another major area of research that
this test presents is that the evaluation in the consistency of the average of the measurement so fo
different Yowie together as well. This can be done by collectively evaluating the measurement
data of different Yowie’s as well and then it can be identified that whether the Yowie also
measure in consistency as it was identified in our case (Lewis & Bartlett, 2019). For instance it
was identified that the ratio between the base of hand to tip of the congest finger as compared to
the head to the base of the chin was usually forum to be 1 point i.e. the denominator exceeded by
approximately 1 point in case of humans. Same can be identified for Yowie’s as well thus
presenting further points of research in this area.
Mathematics and mathematical language used
The mathematics of ratio was used in order to calculate the data related to the Yowie
measurement an under this the main calculation that was done as regarding the Yowie
measurement was by adding up the individual values and then taking up the average of such
individual values. The ratios were calculated by comparing two values where one value was kept
in the numerator and another value was kept in denominator so that the ratio between the two
values could be identified.
x/ y = ratio
This was done in the case of base of hand to longest tip as compared to that of head to
base of chin. This was done to identify the ratios and it was find that approximately 1 is the most
consistent value in all the participants.
Another mathematical expression used in the calculation was the average where the
individual values were summed up and divided by the total number of values. For instance if
there are four values that are to be averaged, then the calculation can be done as:
(a + b + c + d) / 4
This average helped in ascertaining the Yowie’s measurements based on our measurements.
4

TUTORIAL INVESTIGATION 3: DICE DIFFERENCE
Description of the activity
The game of dice difference was influential in ascertaining the basic concepts of
probability and data collection as well as analysis. In the dice difference game, there are mainly
two players who play with two dices. The two dices collectively are thrown consecutively by the
players. Then the number from 1 to 6 which is depicted in both the dices is recorded and the
differences of both the numbers are ascertained (Sharma, 2019). Then this differences was used
to ascertain the points that have been gained by either player A or the player B by the difference
number, i.e., if the difference number thus obtained is either 0, 1 or 2, then the point was given to
player A and if the resultant difference showed 3, 4, or 5, then the point was given to Player B.
I can say that the game we played was fair right from the beginning to the end because there
were 50% chances of the number on dice coming out odd or even and thus affecting the
difference between both the numbers. There were no external factors that could be used to
predict the future outcome of any of the dice.
The theoretical probability for player A was calculated to be 2/ 3 and for player B, it was
identified as 1/ 3. This shows that the game is not fair because the chances of Player A winning
are twice more likely as compared to that of Mr. A. The reason is that the probability chances is
not fair i.e. for player A only outcomes 0, 1 and 2 have been taken and for Player B, outcomes 3,
4 and 5 have been taken. The experimental probability showed that the actual results were
different from the ones that were expected in accordance with the theoretical probability. In the
experimental probability, we identified that out of the 36 probable outcomes that were identified
in theoretical probability and the expected chances of player A having twice more probability of
winning was proven to be false (Robson, Phillips & Guerrero, 2018). In the actual experiment,
we obtained different outcomes which were not in accordance with the theoretical probability
and were found to be almost 50: 50.
The manner in which this game can be made fair is by:
 Giving points on the basis of even or odd numbers difference. For examples: if 0, 2, 4 comes
then player a will be given a point and if 1, 3 or 5 comes then player B will be given the due
point. This insures theoretical probability of both players wining the round.
 Another method is by giving one player a point if the difference is 0, 3, 4 or 5 and one point
to second player if difference is 1, 2. This is in accordance with the expected outcomes.
5

 The third action that can be recommended is not keeping equivalent point’s i.e. when
difference is 0 player can be given 7 points and when difference is 1 or 2 player B can be
allotted 11 points.
 Another alternate is that one i.e. with 1to 6 numbers can be labelled and another with only
three 0 or with three 6.
 Therefore, the change in winning strategies can help in increasing the fairness in the game of
dice difference.
Extending the investigation
The investigation can be further extended in the form of data analysis where the
implication of fairness on the dice rolling and its outcomes can be further researched and studies
can be conducted for better formulation of more realistic formulas (Isaksen & et.al., 2016). This
will help in further enhancing the fairness in probabilistic formulas and the dice game.
Mathematics and mathematical language used
The first step was to order the pairs on the basis of different outcomes and this was done in
a following manner:
Then the tabular formats as per the differences were identified in a following manner:
6

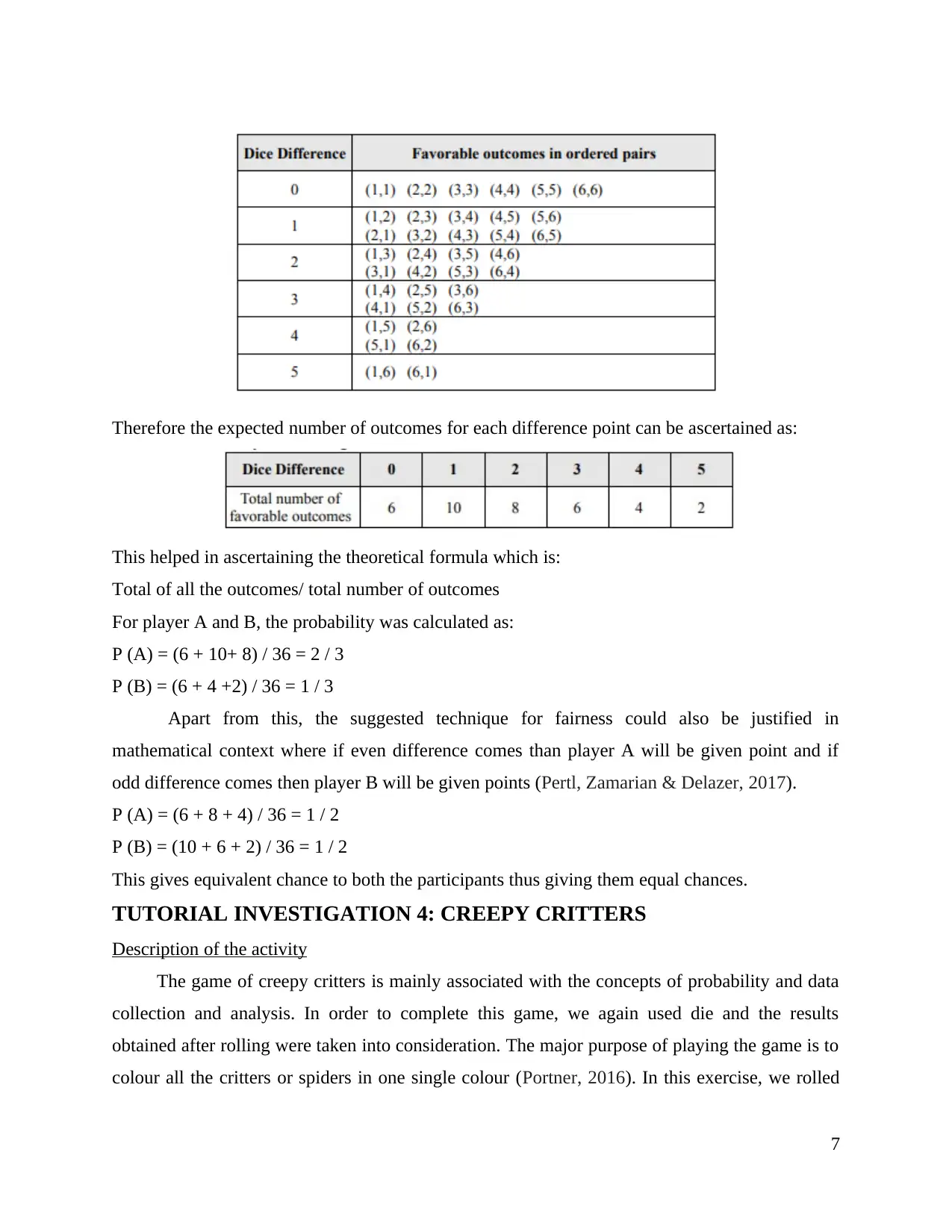
Therefore the expected number of outcomes for each difference point can be ascertained as:
This helped in ascertaining the theoretical formula which is:
Total of all the outcomes/ total number of outcomes
For player A and B, the probability was calculated as:
P (A) = (6 + 10+ 8) / 36 = 2 / 3
P (B) = (6 + 4 +2) / 36 = 1 / 3
Apart from this, the suggested technique for fairness could also be justified in
mathematical context where if even difference comes than player A will be given point and if
odd difference comes then player B will be given points (Pertl, Zamarian & Delazer, 2017).
P (A) = (6 + 8 + 4) / 36 = 1 / 2
P (B) = (10 + 6 + 2) / 36 = 1 / 2
This gives equivalent chance to both the participants thus giving them equal chances.
TUTORIAL INVESTIGATION 4: CREEPY CRITTERS
Description of the activity
The game of creepy critters is mainly associated with the concepts of probability and data
collection and analysis. In order to complete this game, we again used die and the results
obtained after rolling were taken into consideration. The major purpose of playing the game is to
colour all the critters or spiders in one single colour (Portner, 2016). In this exercise, we rolled
7

the die consecutively and the condition for beginning it requires that there should be the digit 1.
Only when the digit 1 is obtained, we could colour the body of the relevant colour which is the
first requirement. The next mandatory requirement is to obtain a 2 on the die so that the head can
be coloured next. After these two body parts are coloured, then all the remaining parts of the
spider can be coloured according to the pre decided criteria. We structured a proper format where
obtaining 3 would indicate the every eye could be filled, 4 indicates that each antenna could be
filled now, then number 5 shows that every leg- roll could b coloured and on obtaining 6 on the
die, the last remaining part proboscis could be coloured. This enabled us in completing the
colouring of entire spider. The data set that we were able to obtain can be termed as a
complicated set of outcomes that made us took a long time to complete the entire game. The
stem and leaf diagram also helps in developing a uni modal diagram (YORKE, 2019). The centre
of the data set was obtained through the stem and leaf diagram that further assisted in
ascertaining the mean median and mode of the data set that was collected through the evaluation
of the die roll in order to complete the game. However, it can be ascertained that the graphical
representation of the data sets in the form of uni - modal or bi - modal graphs could help in
effectively developing the better presentation of the game.
Extending the investigation
The investigation that has been conducted in the game of creepy critters can be further
extended on the representation in the form of graph format. I identified that there were not many
clear guidelines on the manner in which different graphs can be created as a substitute for
representing the information collected through data set in a better manner. Therefore, this is one
field of research that can be further developed and evaluated. Another major point of research
that can be further extended through this investigation is through assumptions and predictions
related to the data set that was collected for the game of creepy critters (Mabbett, 2018). The
assumption is too restricting in the completion in the game and restricts the possibilities that
could otherwise have occurred. Therefore, this is also an area of extended research where the
rules can be further relaxed so that game can be completed more effectively.
Mathematics and mathematical language used
The major mathematic formulas were involved in the calculation of mean, median and
mode that can be ascertained or detailed in following manner:
The mean is the average value and can be ascertained as:
8

Total of all the values in the data sets/ number of times the dice was rolled.
The median is the central value and this can be calculated as:
= (N+ 1) / 2 where N signifies the number of values in the data set collectively.
The mode is the most frequently occurring number in the data set and can be calculated as:
Sort out the numbers i.e. data sets from lowest to highest and then count occurrence of each
number. The number which occurred for the maximum time on the die will be termed as mode.
Apart from this another major calculation that was required to complete the investigation
was of stem and leaf where the first digits are all put into stem and the remaining last digit is
treated as leaf (Myers, 2018). Here the digits with common stem are put in the same leaf in front
of them. An example of the single digit stem and leaf can be presented as:
Values: 0, 6, 1, 4, 2, 6, 0, 5, 4, 1, 3, 5
The stem and leaf for this would therefore be:
Stem Leaf
0 0 6 1 4 2 6 0 5 4 1 3 5
REFLECTION
I can conclude that on an overall basis, the entire experience of getting involved in these
tests and then evaluating the results was mostly positive for me. The learning that I was able to
develop in different fields helped me in widening my thinking perspective. For instance, through
the Yowie game I came to know about the species called Yowie and how the measurements of
this species are being taken into consideration. Another game of dice different taught me about
the probability related concepts and the importance of data recording was also highlighted for
me. This was instrumental in learning that how outcomes can be predicted on the basis of
probability mechanisms and what role does the chances taken play. The third game which I
indulged in was Creepy critters where I identified that the statistics, data collection and the data
analysis tactic can be implemented in which manner. I realised that earlier I was not particularly
organised in the manner of data collection techniques and I found myself making mistakes
frequently. However, the frequency of such mistakes declined as I continued m process of data
collection and therefore, I was able to successfully resolve this problem. On the other hand, I
found that the analysis and interpretation of the data along with the understanding that is required
for the games was excellently developed in me and I was in the position of directing my other
9

teammates as well. Therefore, through the process of learning I was able to gain insights into
many of the aspects particularly in relation to the requirement of data collection skills that I need
to develop.
10

REFERENCES
Books and Journals
Alyazichi, Y. M., Jones, B. G., & McLean, E. J. (2015). Spatial distribution of heavy metal
contaminations in Yowie Bay sediments and their environmental impacts. Water Resour
Manag. 8(196). 363.
Isaksen, A., & et.al., A. (2016). Characterising score distributions in dice games. Game and
Puzzle Design, 2(1), 59.
Lewis, J., & Bartlett, A. (2019). Losing Bigfoot'. The Lost Ethnographies: Methodological
Insights from Projects that Never Were (Studies in Qualitative Methodology, Volume 17).
Emerald Publishing Limited, 47-63.
Mabbett, K. L. (2018). Kinder Tools: the effectiveness of a 12-week response to intervention
approach to improve fine motor and visual motor perceptual skills in kindergarten
students (Doctoral dissertation, Boston University).
Myers, K. (2018). Gendered Interactions in School. In Handbook of the Sociology of Gender (pp.
199-214). Springer, Cham.
Pertl, M. T., Zamarian, L., & Delazer, M. (2017). Reasoning and mathematical skills contribute
to normatively superior decision making under risk: evidence from the game of dice
task. Cognitive processing, 18(3), 249-260.
Portner, B. M. (2016). What Words Cannot Say: The Power of Art and Play in Children? s
Expression and Mental Health (Doctoral dissertation).
Robson, D., Phillips, J., & Guerrero, S. (2018). Don’t just roll the dice: simple solutions for
circulating tabletop game collections effectively in your library. Library Resources &
Technical Services. 62(2). 80.
Sharma, S. (2019). Probability exploration in a game context: The dice difference game.
Townsend, M. (2016). Is Bigfoot Real? Emerging Scientific Evidence.
Tuniz, C., Gillespie, R., & Jones, C. (2016). The bone readers: science and politics in human
origins research. Routledge.
YORKE, G. (2019). Therapeutic Games to Treat Grief. Game Play: Therapeutic Use of Games
with Children and Adolescents, 173.
11

12