Geometry Assignment: Exploring Lines, Angles, Midpoints, and Distance

This assignment provides detailed solutions to ten geometry problems. The first question involves determining the length of a line segment given collinear points. The second question calculates the coordinates of an endpoint given a midpoint and another endpoint. The third question uses the Pytha...Read More

Question 1
Given that ABCD are collinear, implying they are on the same line, and
¿ x +14 , BC=x +11 ,CD=x +13 . Since these points are collinear, AD= AB+ BC+CD
Therefore, AD= ( x+ 14 ) + ( x +11 ) + ( x+ 13 ) =29
¿ 3 x+ 38=29
3 x=−9
x=−3
BC=x +11=−3+11=8
BC=8
Question 2
Given two endpoints with coordinates (x1 , y1 ¿ and (x2 , y2 ), we can obtain the coordinates of the
midpoint as ( x1 + x2
2 , y1 + y2
2 ). Hence, given a midpoint and one endpoint, we can obtain the other
endpoint.
End point1(6,-3) and midpoint(-2,-5)
Implying that 6+x2
2 =−2 and −3+ y2
2 =−5.
Solving for these equations gives the following results:
x2= ( −2∗2 ) −6=−10 and y2= ( −5∗2 ) +3=−7
Coordinates to the other endpoint are (−10 ,−7)
Question 3
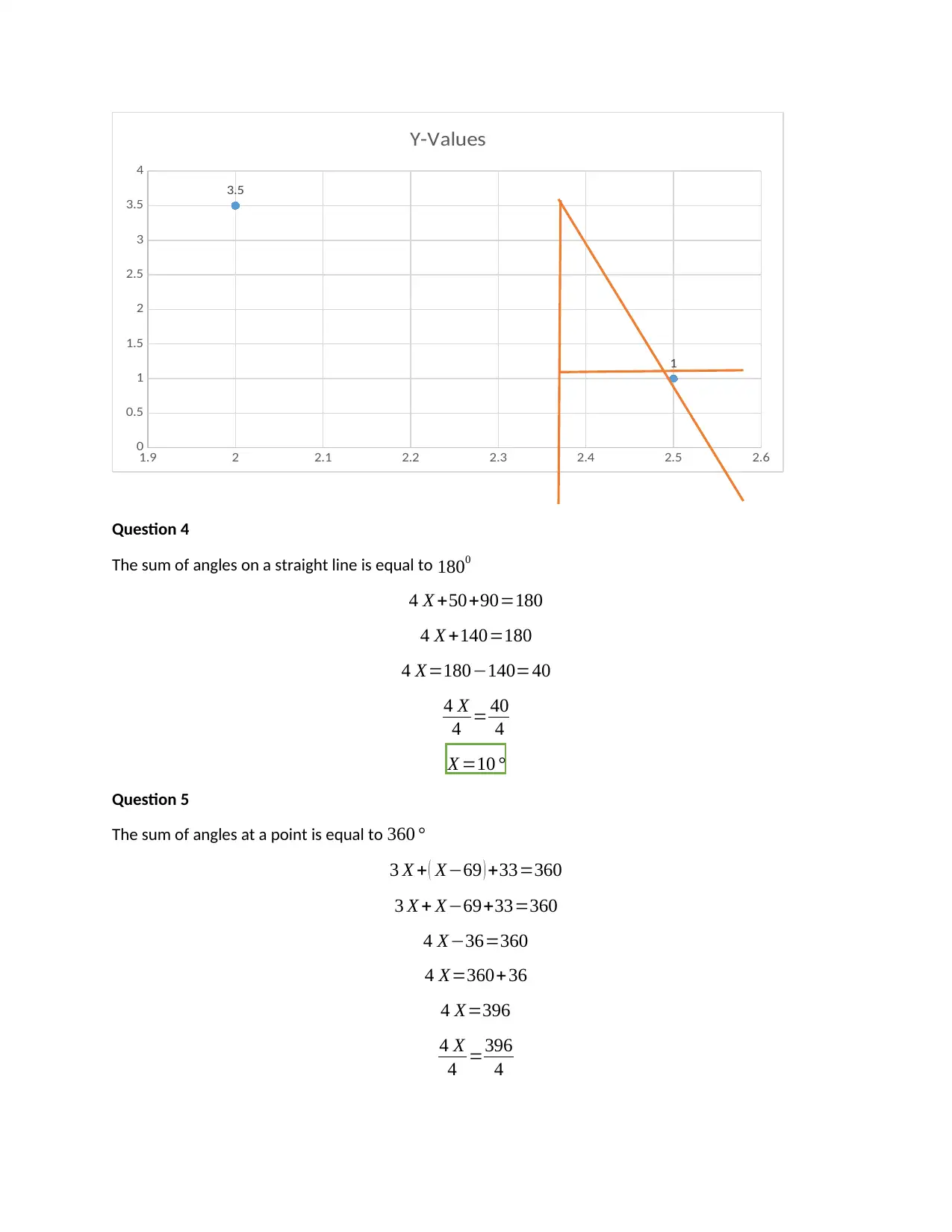
First, plot the two points. To solve for the distance between the two points, we draw a right angled
triangle for ease of using the Pythagorean Theorem.
From the plot below, we obtain the values of the length of the base as 0.5 and the length of the height
as 2.5.
The Pythagorean rule states that a2+b2=c2
Substituting the values gives 0.52 +2.52 =6.5
Solving for c gives the distance between the two points as √6.5=2.5495

1.9 2 2.1 2.2 2.3 2.4 2.5 2.6
0
0.5
1
1.5
2
2.5
3
3.5
4
3.5
1
Y-Values
Question 4
The sum of angles on a straight line is equal to 1800
4 X +50+90=180
4 X +140=180
4 X=180−140=40
4 X
4 = 40
4
X =10 °
Question 5
The sum of angles at a point is equal to 360 °
3 X + ( X−69 ) +33=360
3 X + X−69+33=360
4 X−36=360
4 X=360+36
4 X=396
4 X
4 =396
4

X =99 °
Question 6
Corresponding anglesare equal
14 x +2=100
14 x=100−2=98
14 x
14 = 98
14
x=7 °
Question 7
Sum of interior angles is equal to 180°
75+9 x +15=180
9 x +90=180
9 x=180−90=90
9 x
9 = 90
9
x=10 °
Question 8
Vertically opposite angles are equal
11 x−7=70
11 x=70+7=77
11 x
11 =77
11
x=7 °
Question 9
Alternate exterior angles are equal
7 x +11=8 x +4
8 x−7 x=11−4
x=7 °

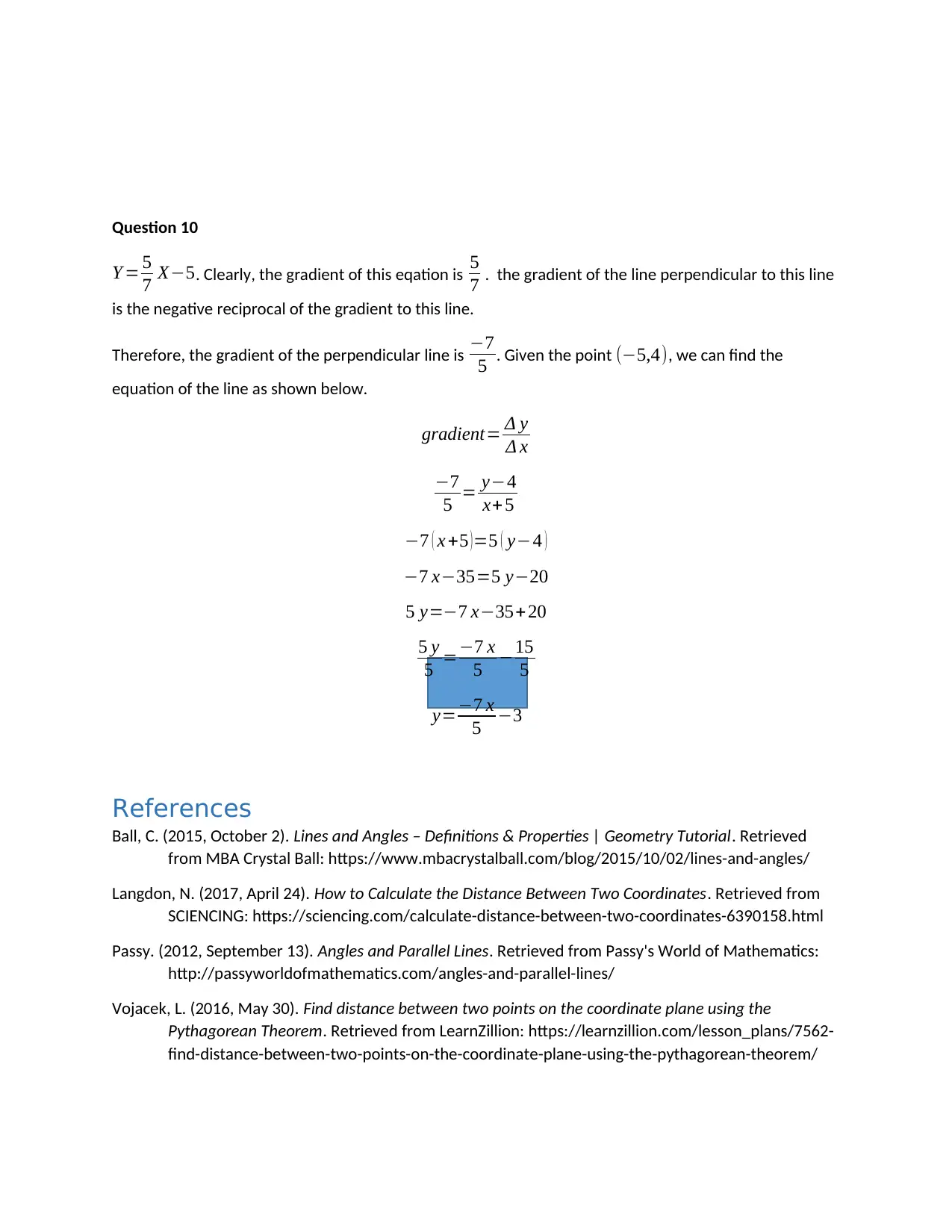
Question 10
Y = 5
7 X−5. Clearly, the gradient of this eqation is 5
7 . the gradient of the line perpendicular to this line
is the negative reciprocal of the gradient to this line.
Therefore, the gradient of the perpendicular line is −7
5 . Given the point (−5,4), we can find the
equation of the line as shown below.
gradient= Δ y
Δ x
−7
5 = y−4
x+ 5
−7 ( x +5 ) =5 ( y−4 )
−7 x−35=5 y−20
5 y=−7 x−35+20
5 y
5 =−7 x
5 −15
5
y=−7 x
5 −3
References
Ball, C. (2015, October 2). Lines and Angles – Definitions & Properties | Geometry Tutorial. Retrieved
from MBA Crystal Ball: https://www.mbacrystalball.com/blog/2015/10/02/lines-and-angles/
Langdon, N. (2017, April 24). How to Calculate the Distance Between Two Coordinates. Retrieved from
SCIENCING: https://sciencing.com/calculate-distance-between-two-coordinates-6390158.html
Passy. (2012, September 13). Angles and Parallel Lines. Retrieved from Passy's World of Mathematics:
http://passyworldofmathematics.com/angles-and-parallel-lines/
Vojacek, L. (2016, May 30). Find distance between two points on the coordinate plane using the
Pythagorean Theorem. Retrieved from LearnZillion: https://learnzillion.com/lesson_plans/7562-
find-distance-between-two-points-on-the-coordinate-plane-using-the-pythagorean-theorem/