Heat Transfer: Heat Flow Through Rectangular Plate

This document discusses the heat flow through a rectangular plate with a uniform cross-sectional area. It covers the assumptions, equations, and boundary conditions involved in heat transfer. The document also provides references for further study.

Running head: HEAT TRANSFER
HEAT TRANSFER
Name of Student
Institution Affiliation

HEAT TRANSFER 2
HEAT FLOW THROUGH RECTANGULAR PLATE HAVING UNIFORM CROSS
SECTIONAL AREA.
Assumptions
i. K is constant throughout the material.
ii. HT is unidirectional.
iii. There is no heat generation in the field
t0= temperature at the base of the fin
ta= ambient temperature
l= is the length of the fin
Acs= is the crossectional area of the beam
As = surface area of the beam
P= perimeter of the fin
And these can be illustrated using the following diagram

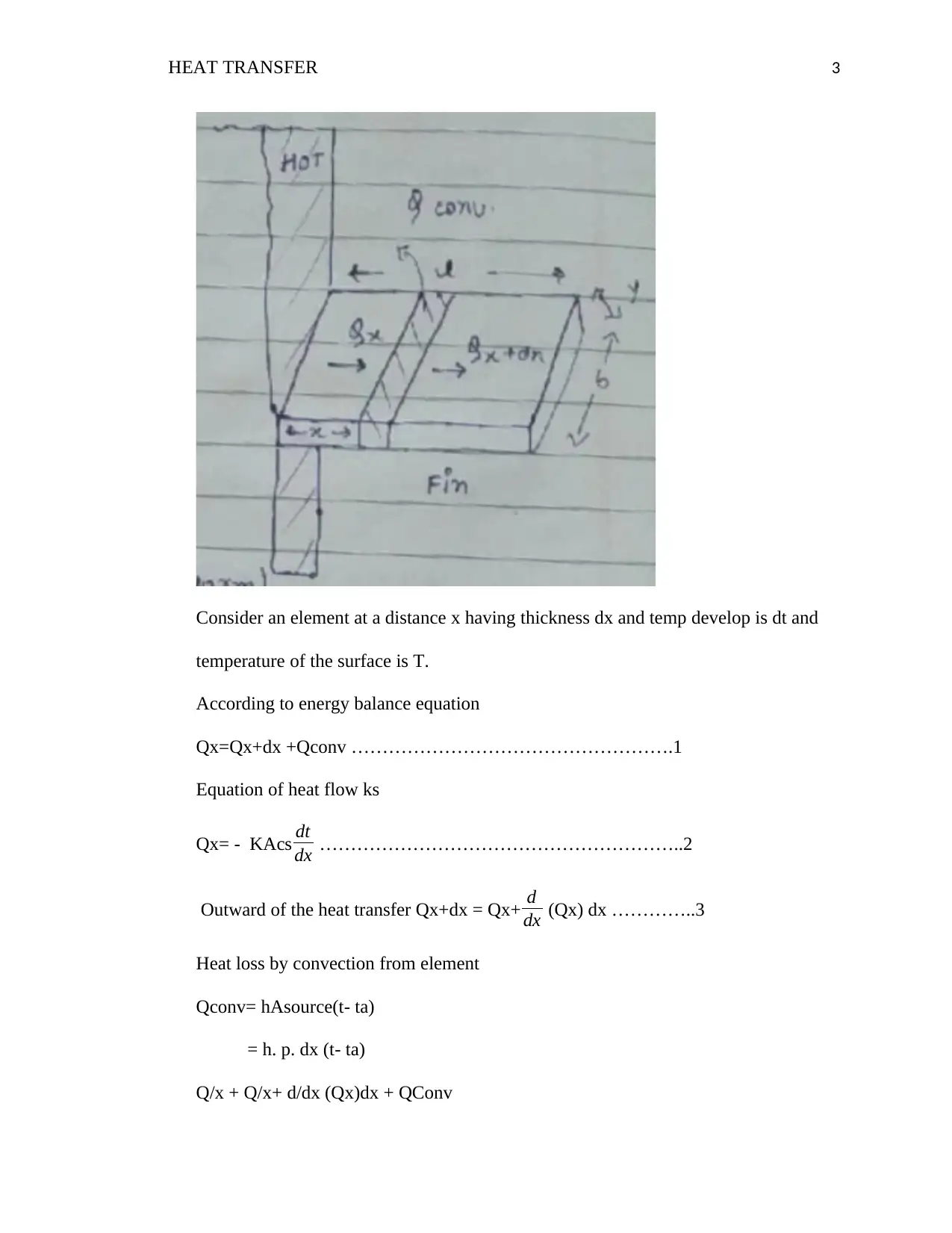
HEAT TRANSFER 3
Consider an element at a distance x having thickness dx and temp develop is dt and
temperature of the surface is T.
According to energy balance equation
Qx=Qx+dx +Qconv …………………………………………….1
Equation of heat flow ks
Qx= - KAcs dt
dx …………………………………………………..2
Outward of the heat transfer Qx+dx = Qx+ d
dx (Qx) dx …………..3
Heat loss by convection from element
Qconv= hAsource(t- ta)
= h. p. dx (t- ta)
Q/x + Q/x+ d/dx (Qx)dx + QConv

HEAT TRANSFER 4
d (1−KA cs )
dx . dt d / x
dx = - hpdx (t – ta)
KAcs d2 t
d x2 = ph (t-ta)
θ= t-ta
dθ
dx = dt
dx ( t is variant )
d2 θ
d x2 = ph
KAcs , Consider ph
KAcs = m2
d2 θ
d x2 – m2 θ=0
Second order differential equation
D=±m
PI= = C.F = Ce mx + C2e –mx
θ= Ce mx + C2e –mx
Boundary conditions,
X= 0 , θ−θ 0=to - ta
FIN LOSING HEAT AT TIP
Let t1 be the temperature at the tip
i. at x=0

HEAT TRANSFER 5
θ−θ 0=to - ta
ii. Qconv ( at x=u ) = Qconv ( at x = u)
−KAcs ¿ ) x= u =hA(tl−¿ta)
dt
dx )x=u = −h
k . Qu
From the above equation we obtain
(tl−¿ta) =Ce mx + C2e –mx
dt
dx = 0+ mCe mx −¿ mC2e –mx
−h
k . Qu=m(Ce mx −¿ C2e –mx)
−h
k . Qu=m(Ce ml + C2e –ml)= (Ce ml −¿ C2e –ml)
Where θl= (Ce mx +C2e –mx)= (Ce mx −¿ C2e –mx) and θ 0=C1+C2
−h
k .(Ce ml + C2e –ml)=(Ce mx −¿ θo e−ml+ Ce−ml)
θ e−ml( 1−h
km ) = αC 1 [coshml+ h
km sin hml ]
C1= θ e−ml (1− h
km )
2 [ coshml +hkm . sinhml ]
C2= θo−C 1
= θ ¿

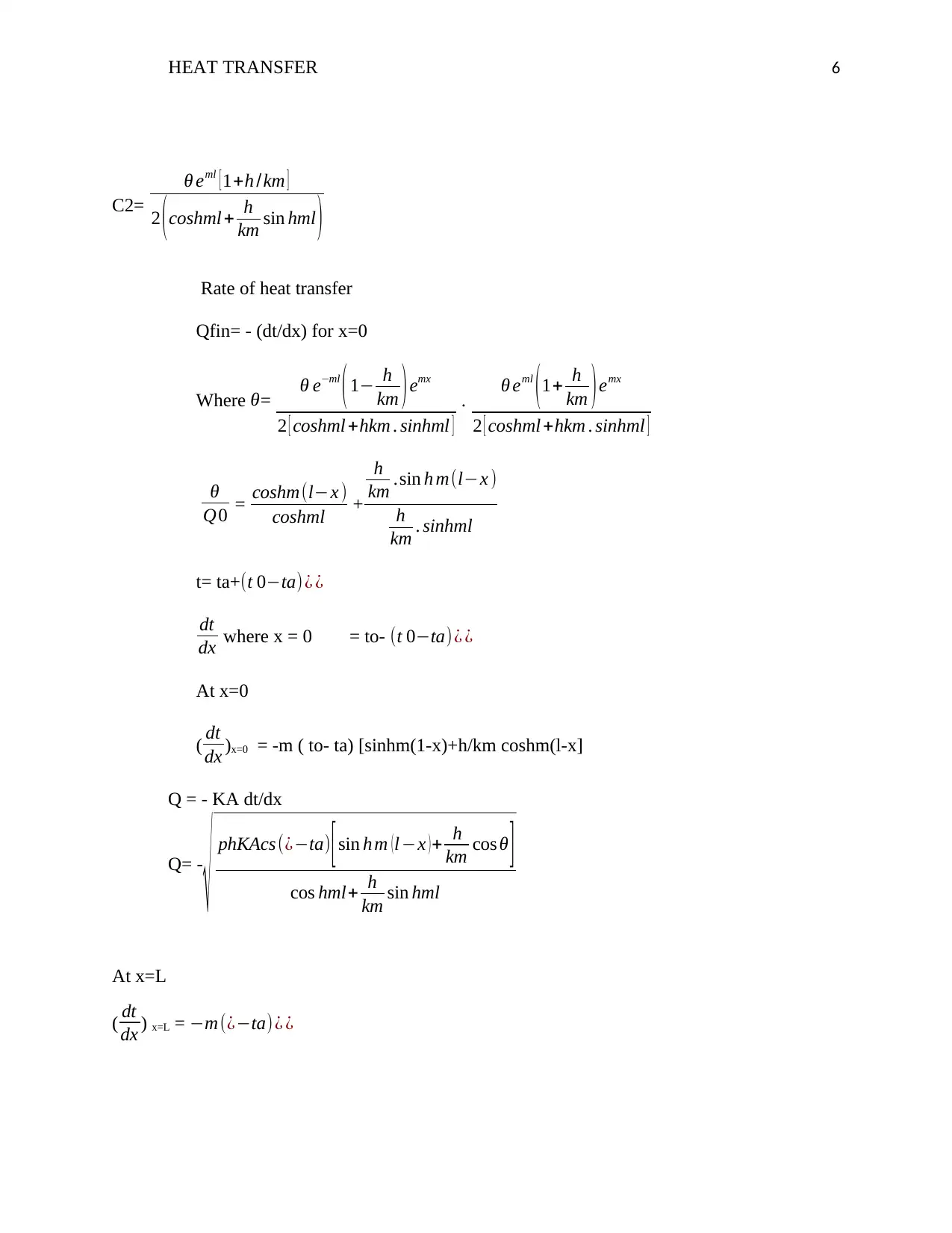
HEAT TRANSFER 6
C2=
θ eml [ 1+h /km ]
2 (coshml + h
km sin hml )
Rate of heat transfer
Qfin= - (dt/dx) for x=0
Where θ= θ e−ml
( 1− h
km ) emx
2 [ coshml +hkm . sinhml ]
. θ eml
(1+ h
km )emx
2 [ coshml +hkm . sinhml ]
θ
Q0 = coshm(l−x )
coshml +
h
km .sin h m(l−x )
h
km . sinhml
t= ta+(t 0−ta)¿ ¿
dt
dx where x = 0 = to- (t 0−ta)¿ ¿
At x=0
( dt
dx )x=0 = -m ( to- ta) [sinhm(1-x)+h/km coshm(l-x]
Q = - KA dt/dx
Q= -
√ phKAcs(¿−ta) [sin h m (l−x )+ h
km cos θ ]
cos hml+ h
km sin hml
At x=L
( dt
dx ) x=L = −m(¿−ta)¿ ¿

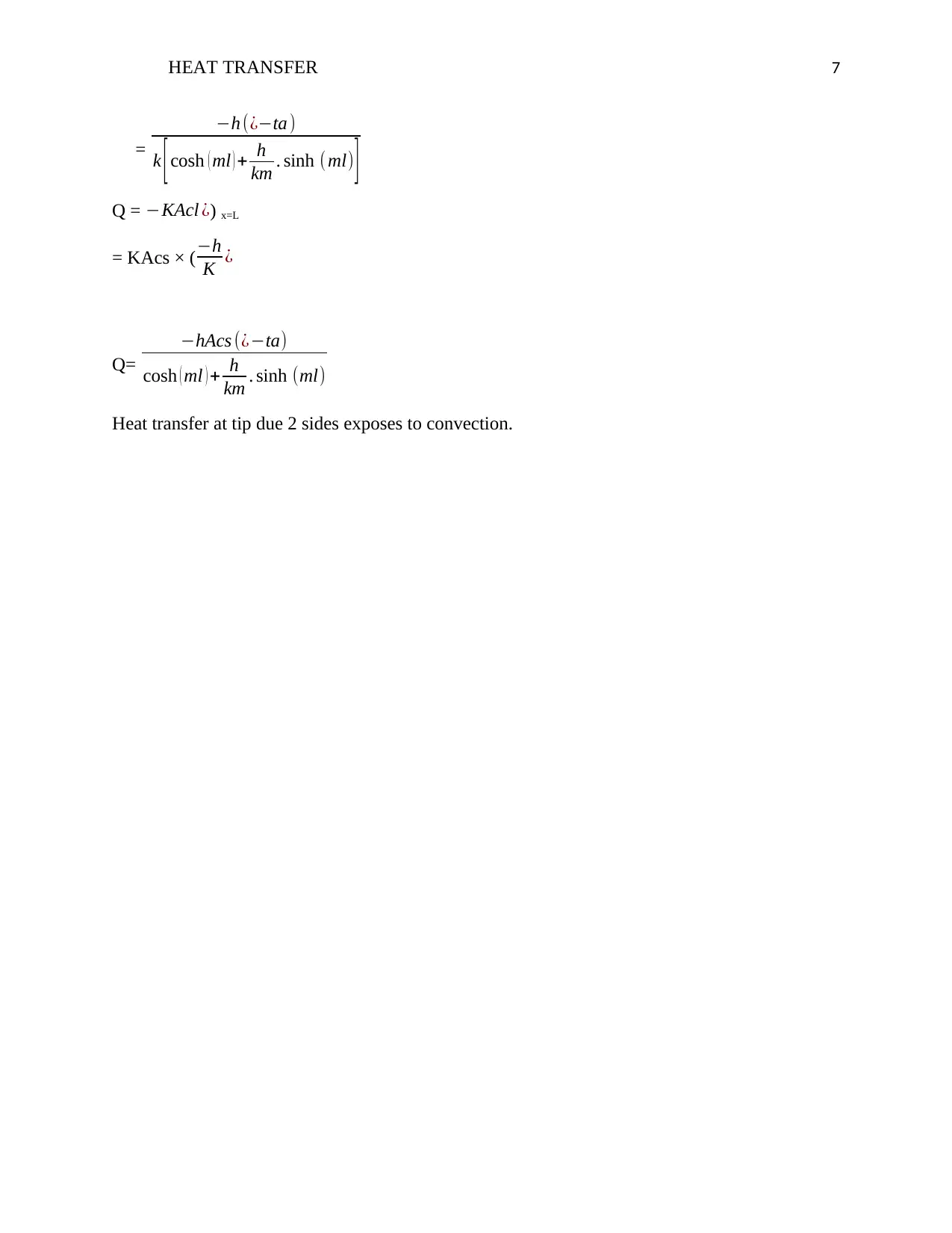
HEAT TRANSFER 7
=
−h (¿−ta )
k [ cosh ( ml ) + h
km . sinh ( ml) ]
Q = −KAcl ¿) x=L
= KAcs × ( −h
K ¿
Q=
−hAcs (¿−ta)
cosh ( ml ) + h
km . sinh (ml)
Heat transfer at tip due 2 sides exposes to convection.

HEAT TRANSFER 8
References
Naterer, G. (2018). Advanced Heat Transfer. Hull: Taylor & Francis, CRC Press.
Thomas, G. (2012). Heat Transfer: A Problem Solving Approach. Chicago: Routledge.