Calculus II Final Exam Solutions - Double Integrals and Theorems
VerifiedAdded on 2022/12/14
|20
|4348
|341
Homework Assignment
AI Summary
This document presents the complete solutions to a Calculus II final exam. The solutions cover a variety of topics including the evaluation of double integrals and an analysis of whether the answers contradict Fubini's Theorem. The assignment also explores the similarities between Fubini's and Clairaut's Theorems, and provides a proof demonstrating the equality of mixed partial derivatives. Furthermore, the document provides solutions to several true/false questions, with detailed explanations, calculations, and counterexamples where applicable. These solutions involve the application of Fubini's Theorem, the evaluation of double integrals in polar coordinates, and concepts related to vector calculus, including the divergence and curl operators, and the application of Stokes' Theorem. Finally, the document includes a proof related to Laplace operators and harmonic functions, and demonstrates path independence of line integrals.

i)
I =
Z 1
0
Z 1
0
x − y
(x + y)3 dxdy
Using Wolfram Alpha Calculator, we get
Z 1
0
Z 1
0
x − y
(x + y)3 dxdy = −
1
2
ii)
I =
Z 1
0
Z 1
0
x − y
(x + y)3 dydx
Using Wolfram Alpha Calculator, we get
Z 1
0
Z 1
0
x − y
(x + y)3 dydx =1
2
Even though both integrals are not equal, Fubini’s Theorem is not violated.
This is because, (B, Hass, Heil and Weir, 2018) “Fubini’s Theorem states if,
f is continuous in the region R : [a, b] × [c, d] and ”
ZZ
R
|f (x, y)| d(x, y) ≤ ∞
then Z b
a
Z d
c
f (x, y) dx dy =
Z d
c
Z b
a
f (x, y) dy dx
1
I =
Z 1
0
Z 1
0
x − y
(x + y)3 dxdy
Using Wolfram Alpha Calculator, we get
Z 1
0
Z 1
0
x − y
(x + y)3 dxdy = −
1
2
ii)
I =
Z 1
0
Z 1
0
x − y
(x + y)3 dydx
Using Wolfram Alpha Calculator, we get
Z 1
0
Z 1
0
x − y
(x + y)3 dydx =1
2
Even though both integrals are not equal, Fubini’s Theorem is not violated.
This is because, (B, Hass, Heil and Weir, 2018) “Fubini’s Theorem states if,
f is continuous in the region R : [a, b] × [c, d] and ”
ZZ
R
|f (x, y)| d(x, y) ≤ ∞
then Z b
a
Z d
c
f (x, y) dx dy =
Z d
c
Z b
a
f (x, y) dy dx
1
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

For the given integral(s)
ZZ
R
x − y
(x + y)3 d(x, y) ≥
Z 1
0
Z x
0
x − y
(x + y)3 dx dy
≥
Z 1
0
− −y + x
2 (y + x)
2 − 1
2y+ x
2y (x + y)
dy
[the term,
Z 1
0
1
2y dy = divergent]
≥ ∞
Thus, Fubini’s Theorem criteria is not met for this particular integral and it
cannot be applied to equate the results of i) and ii).
Answer
According to B,Hass,Heil and Weir (2018) “Fubini’s Theorem states that
the order of integration of a function of two variables does not affect the value
of the double integral.Clairaut’s theorem states that the order of differen-
tiation of such a function does not affect the value of the second derivative.
Both of the theorems require continuity (although Fubini’s allows a finite
number of smooth curves to contain discontinuities).”
Now,
gxy = ∂
∂x
∂
∂yg(x, y)
= ∂
∂x
∂
∂y
Z x
a
Z y
c
f (s, t) dtds
2
ZZ
R
x − y
(x + y)3 d(x, y) ≥
Z 1
0
Z x
0
x − y
(x + y)3 dx dy
≥
Z 1
0
− −y + x
2 (y + x)
2 − 1
2y+ x
2y (x + y)
dy
[the term,
Z 1
0
1
2y dy = divergent]
≥ ∞
Thus, Fubini’s Theorem criteria is not met for this particular integral and it
cannot be applied to equate the results of i) and ii).
Answer
According to B,Hass,Heil and Weir (2018) “Fubini’s Theorem states that
the order of integration of a function of two variables does not affect the value
of the double integral.Clairaut’s theorem states that the order of differen-
tiation of such a function does not affect the value of the second derivative.
Both of the theorems require continuity (although Fubini’s allows a finite
number of smooth curves to contain discontinuities).”
Now,
gxy = ∂
∂x
∂
∂yg(x, y)
= ∂
∂x
∂
∂y
Z x
a
Z y
c
f (s, t) dtds
2

= ∂
∂x
Z x
a
Z y
c
∂
∂yf (s, t) dt ds
[Using Fundamental Theorem:
Z y
c
∂
∂yf (s, t) dt = f (s, y) − f (s, c)]
[f (s, c) is outside the region of interest since, c < y < d]
[∴ f (s, y) − f (s, c) = f (s, y)]
= ∂
∂x
Z x
a
f (s, y) ds
= f (x, y)
By Clairaut’s theorem, we have
gxy = gyx
Therefore,
gxy = gyx = f (x, y)
3
∂x
Z x
a
Z y
c
∂
∂yf (s, t) dt ds
[Using Fundamental Theorem:
Z y
c
∂
∂yf (s, t) dt = f (s, y) − f (s, c)]
[f (s, c) is outside the region of interest since, c < y < d]
[∴ f (s, y) − f (s, c) = f (s, y)]
= ∂
∂x
Z x
a
f (s, y) ds
= f (x, y)
By Clairaut’s theorem, we have
gxy = gyx
Therefore,
gxy = gyx = f (x, y)
3
You're viewing a preview
Unlock full access by subscribing today!

Answer
a)
If f is continuous on [0,1] then
Z 1
0
Z 1
0
f (x)f (y) dxdy =
Z 1
0
f (x) dx
2
We use Fubini’s Theorem corollary that if f = g(x)h(y) and f is continuous
in R : [a, b] × [c, d], then
Z b
a
Z d
c
f dxdy =
Z b
a
g(x) dx
Z d
c
h(y) dy
For the given problem, g = f and h = f and [a, b] = [c, d] = [0, 1].Therefore,
by Fubini’s Theorem corollary, the given statement is True.
4
a)
If f is continuous on [0,1] then
Z 1
0
Z 1
0
f (x)f (y) dxdy =
Z 1
0
f (x) dx
2
We use Fubini’s Theorem corollary that if f = g(x)h(y) and f is continuous
in R : [a, b] × [c, d], then
Z b
a
Z d
c
f dxdy =
Z b
a
g(x) dx
Z d
c
h(y) dy
For the given problem, g = f and h = f and [a, b] = [c, d] = [0, 1].Therefore,
by Fubini’s Theorem corollary, the given statement is True.
4
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

b)
Integral to be evaluated is
ZZ
D
p 4 − x2 − y2 dA
D is a disk given by x2 + y2 ≤ 4, which implies that D is centered at origin
and has radius = 2.
Setting up the integral in polar coordinates:
Z 2π
0
Z 2
0
√ 4 − r2 rdrdθ
This integral is evaluated as
Z 2π
0
Z 2
0
√ 4 − r2 rdrdθ =
Z 2π
0
Z 2
0
r√ 4 − r2 drdθ
[Substitute: 4 − r2 = t]
=
Z 2π
0
Z 2
0
−
√ t
2 dt dθ
=
Z 2π
0
− (4 − r2)3/2
3
2
0
dθ
= 8
3
Z 2π
0
dθ
= 16π
3
Therefore, the given statement is True.
c)
div(curl(∇f )) = 0
Let,
F = ∇f = ∂f
∂x , ∂f
∂y, ∂f
∂z
5
Integral to be evaluated is
ZZ
D
p 4 − x2 − y2 dA
D is a disk given by x2 + y2 ≤ 4, which implies that D is centered at origin
and has radius = 2.
Setting up the integral in polar coordinates:
Z 2π
0
Z 2
0
√ 4 − r2 rdrdθ
This integral is evaluated as
Z 2π
0
Z 2
0
√ 4 − r2 rdrdθ =
Z 2π
0
Z 2
0
r√ 4 − r2 drdθ
[Substitute: 4 − r2 = t]
=
Z 2π
0
Z 2
0
−
√ t
2 dt dθ
=
Z 2π
0
− (4 − r2)3/2
3
2
0
dθ
= 8
3
Z 2π
0
dθ
= 16π
3
Therefore, the given statement is True.
c)
div(curl(∇f )) = 0
Let,
F = ∇f = ∂f
∂x , ∂f
∂y, ∂f
∂z
5

Curl of vector field F = hF1, F2, F3i is
curl(F) = ∂F 3
∂y − ∂F2
∂z , ∂F 1
∂z − ∂F3
∂x , ∂F 2
∂x − ∂F 1
∂y
Therefore, curl ofF = ∇f is
curl(∇f ) = ∂2f
∂y∂z − ∂2f
∂z∂y , ∂2f
∂z∂x − ∂2f
∂x∂z , ∂2f
∂x∂y − ∂2f
∂y∂x
Since f has continuous partialderivatives of allorder on R3, by Clairaut’s
Theorem the mixed partial derivative
f xy = fyx
similarly,the mixed partialderivatives in x,z and y, z are equal. This
implies,
curl(∇f ) = 0
Further,
div(curl(∇f )) = 0
Hence, the given statement is True.
d)
cur(F · G) = curl(F) · curl(G)
F and G are vector fields.
F = hF1, F2, F3i and G = hG1, G2, G3i
Dot product is
F · G = F1G1 + F2G2 + F 3G3
is a scalar function.
Curl of scalar function is undefined.Therefore the left side of the equation
is undefined.
Since, F and G are vector fields, their curl exists and are well defined.Let,
curl(F) = hH1, H2, H3i & curl(G) = hI1, I2, I3i
6
curl(F) = ∂F 3
∂y − ∂F2
∂z , ∂F 1
∂z − ∂F3
∂x , ∂F 2
∂x − ∂F 1
∂y
Therefore, curl ofF = ∇f is
curl(∇f ) = ∂2f
∂y∂z − ∂2f
∂z∂y , ∂2f
∂z∂x − ∂2f
∂x∂z , ∂2f
∂x∂y − ∂2f
∂y∂x
Since f has continuous partialderivatives of allorder on R3, by Clairaut’s
Theorem the mixed partial derivative
f xy = fyx
similarly,the mixed partialderivatives in x,z and y, z are equal. This
implies,
curl(∇f ) = 0
Further,
div(curl(∇f )) = 0
Hence, the given statement is True.
d)
cur(F · G) = curl(F) · curl(G)
F and G are vector fields.
F = hF1, F2, F3i and G = hG1, G2, G3i
Dot product is
F · G = F1G1 + F2G2 + F 3G3
is a scalar function.
Curl of scalar function is undefined.Therefore the left side of the equation
is undefined.
Since, F and G are vector fields, their curl exists and are well defined.Let,
curl(F) = hH1, H2, H3i & curl(G) = hI1, I2, I3i
6
You're viewing a preview
Unlock full access by subscribing today!

therefore,
curl(F) · curl(G) = H1I 1 + H2I 2 + H3I 3
which is a scalar function.
Thus the left side of the given equation is meaningless and right side is a well
defined scalar function, therefore the equality does not hold and it is False.
e)
F is a constant vector field and S is sphere.
Flux of F through the surface of S is given by integral
Flux =
ZZ
S
F · d~S
Divergence Theorem relates this flux to the divergence of field inside S
ZZ
S
F · d~S =
ZZZ
V
div(F) dV
V is the volume occupied by S.
Divergence of a constant vector field is zero.Therefore
ZZ
S
F · d~S =
ZZZ
V
0 dV = 0
Therefore, the given statement is True.
f )
Divergence of a curl is zero
div(curl(F)) = 0
For F = hF1, F2, F3i
curl(F) = ∂F 3
∂y − ∂F2
∂z , ∂F 1
∂z − ∂F3
∂x , ∂F 2
∂x − ∂F 1
∂y
7
curl(F) · curl(G) = H1I 1 + H2I 2 + H3I 3
which is a scalar function.
Thus the left side of the given equation is meaningless and right side is a well
defined scalar function, therefore the equality does not hold and it is False.
e)
F is a constant vector field and S is sphere.
Flux of F through the surface of S is given by integral
Flux =
ZZ
S
F · d~S
Divergence Theorem relates this flux to the divergence of field inside S
ZZ
S
F · d~S =
ZZZ
V
div(F) dV
V is the volume occupied by S.
Divergence of a constant vector field is zero.Therefore
ZZ
S
F · d~S =
ZZZ
V
0 dV = 0
Therefore, the given statement is True.
f )
Divergence of a curl is zero
div(curl(F)) = 0
For F = hF1, F2, F3i
curl(F) = ∂F 3
∂y − ∂F2
∂z , ∂F 1
∂z − ∂F3
∂x , ∂F 2
∂x − ∂F 1
∂y
7
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
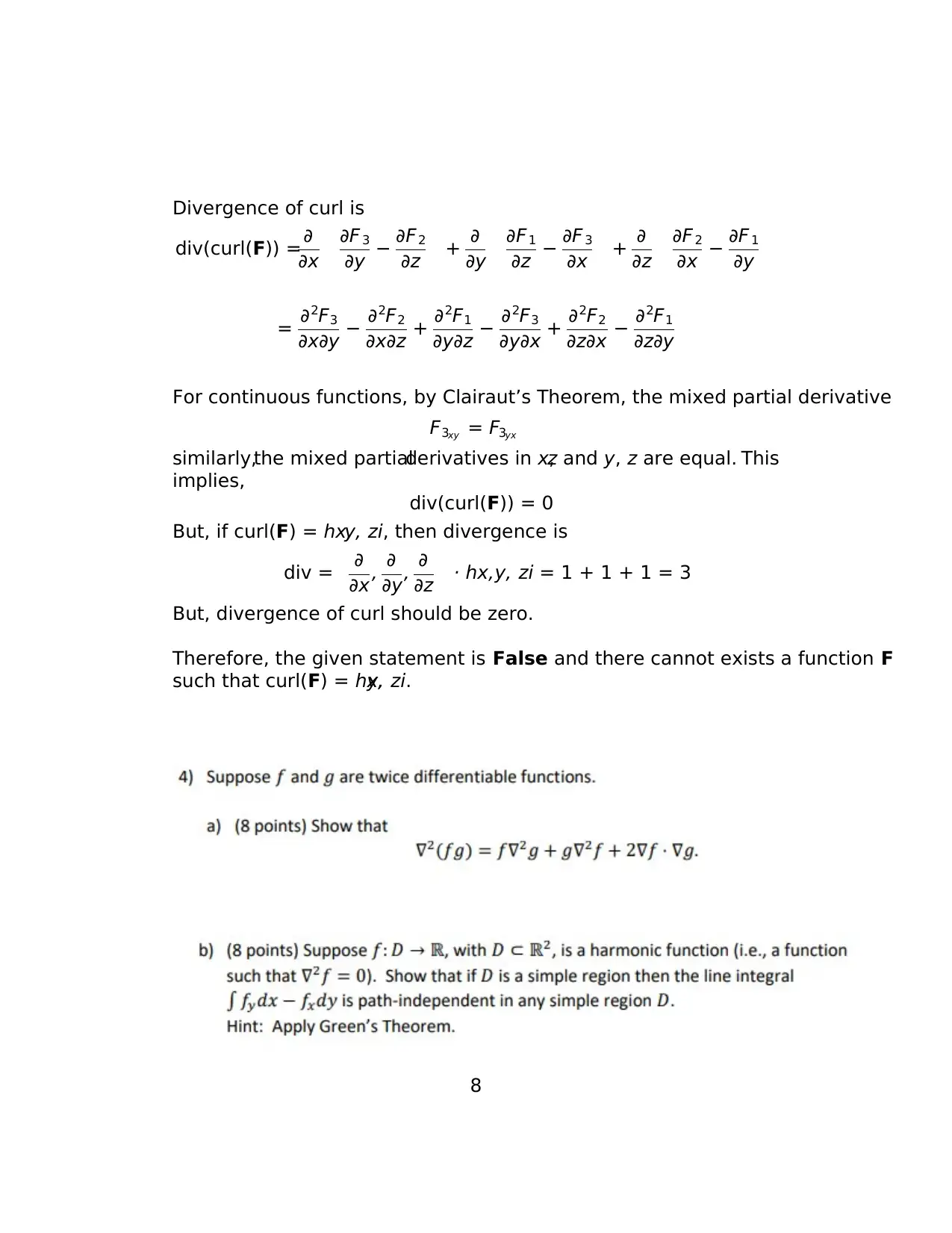
Divergence of curl is
div(curl(F)) = ∂
∂x
∂F 3
∂y − ∂F2
∂z + ∂
∂y
∂F1
∂z − ∂F 3
∂x + ∂
∂z
∂F 2
∂x − ∂F 1
∂y
= ∂2F3
∂x∂y − ∂2F2
∂x∂z + ∂2F1
∂y∂z − ∂2F3
∂y∂x + ∂2F2
∂z∂x − ∂2F1
∂z∂y
For continuous functions, by Clairaut’s Theorem, the mixed partial derivative
F3xy = F3yx
similarly,the mixed partialderivatives in x,z and y, z are equal. This
implies,
div(curl(F)) = 0
But, if curl(F) = hx,y, zi, then divergence is
div = ∂
∂x , ∂
∂y, ∂
∂z · hx,y, zi = 1 + 1 + 1 = 3
But, divergence of curl should be zero.
Therefore, the given statement is False and there cannot exists a function F
such that curl(F) = hx,y, zi.
8
div(curl(F)) = ∂
∂x
∂F 3
∂y − ∂F2
∂z + ∂
∂y
∂F1
∂z − ∂F 3
∂x + ∂
∂z
∂F 2
∂x − ∂F 1
∂y
= ∂2F3
∂x∂y − ∂2F2
∂x∂z + ∂2F1
∂y∂z − ∂2F3
∂y∂x + ∂2F2
∂z∂x − ∂2F1
∂z∂y
For continuous functions, by Clairaut’s Theorem, the mixed partial derivative
F3xy = F3yx
similarly,the mixed partialderivatives in x,z and y, z are equal. This
implies,
div(curl(F)) = 0
But, if curl(F) = hx,y, zi, then divergence is
div = ∂
∂x , ∂
∂y, ∂
∂z · hx,y, zi = 1 + 1 + 1 = 3
But, divergence of curl should be zero.
Therefore, the given statement is False and there cannot exists a function F
such that curl(F) = hx,y, zi.
8

Answer
a)
To prove
∇ 2(fg) = f∇ 2g + g∇2f + 2∇f · ∇g
We prove this result considering f and g as function in two variables (x,y).
This proof can be easily extended to a general function in three variables (x,
y, z).
Proof
Definition of a Laplace operator
∇ 2 = d2
dx2 + d2
dy2
Therefore,
∇ 2(f g) =d2(f g)
dx2 + d2(f g)
dy2
= d
dx
d(f g)
dx + d
dy
d(f g)
dy
[Apply Product rule inside the bracketed terms]
[Notation: df
dx = fx]
= d
dx (f gx + gfx) + d
dy(f gy + gfy)
[Again apply Product rule to each term inside each bracket]
[Notation:
d2f
dx2 = fxx]
= fgxx + fxgx + gfxx + gxf x + fgyy + fygy + gfyy + gyf y
= f (gxx + gyy) + g(fxx + fyy) + 2(fxgx + fygy)
∴ ∇ 2(fg) = f∇ 2g + g∇2f + 2(fxgx + fygy) (1)
Definition of a gradient operator
∇ = ∂
∂x , ∂
∂y
9
a)
To prove
∇ 2(fg) = f∇ 2g + g∇2f + 2∇f · ∇g
We prove this result considering f and g as function in two variables (x,y).
This proof can be easily extended to a general function in three variables (x,
y, z).
Proof
Definition of a Laplace operator
∇ 2 = d2
dx2 + d2
dy2
Therefore,
∇ 2(f g) =d2(f g)
dx2 + d2(f g)
dy2
= d
dx
d(f g)
dx + d
dy
d(f g)
dy
[Apply Product rule inside the bracketed terms]
[Notation: df
dx = fx]
= d
dx (f gx + gfx) + d
dy(f gy + gfy)
[Again apply Product rule to each term inside each bracket]
[Notation:
d2f
dx2 = fxx]
= fgxx + fxgx + gfxx + gxf x + fgyy + fygy + gfyy + gyf y
= f (gxx + gyy) + g(fxx + fyy) + 2(fxgx + fygy)
∴ ∇ 2(fg) = f∇ 2g + g∇2f + 2(fxgx + fygy) (1)
Definition of a gradient operator
∇ = ∂
∂x , ∂
∂y
9
You're viewing a preview
Unlock full access by subscribing today!

Now,
∇f · ∇g = hfx, f yi · hgx, gyi
[Expanding the vector dot product]
= fxgx + fygy (2)
Comparing (2) with the last term inside the bracket in (1), we find that the
two are equal.Therefore,
∇ 2(fg) = f∇ 2g + g∇2f + 2∇f · ∇g
Hence proved.
b)
f is a harmonic function in two variables (x, y).Implies
∇ 2f = 0
Let,
F = hfy, −fxi
Let, C be a closed curve in D.Position vector of C is:r = hx,yi. Implies,
dr = hdx,dyi. Circulation of F around C is given by
I
C
F · dr =
I
C
hfy, −fxi · hdx,dy
=
I
C
f ydx − fxdy (1)
Green’s Theorem for circulation ofa vector field F = hM,N i, around a
closed curve C in a simple region D is
I
C
F · dr =
I
C
Mdx + Ndy =
ZZ
D
Nx − My dA (2)
10
∇f · ∇g = hfx, f yi · hgx, gyi
[Expanding the vector dot product]
= fxgx + fygy (2)
Comparing (2) with the last term inside the bracket in (1), we find that the
two are equal.Therefore,
∇ 2(fg) = f∇ 2g + g∇2f + 2∇f · ∇g
Hence proved.
b)
f is a harmonic function in two variables (x, y).Implies
∇ 2f = 0
Let,
F = hfy, −fxi
Let, C be a closed curve in D.Position vector of C is:r = hx,yi. Implies,
dr = hdx,dyi. Circulation of F around C is given by
I
C
F · dr =
I
C
hfy, −fxi · hdx,dy
=
I
C
f ydx − fxdy (1)
Green’s Theorem for circulation ofa vector field F = hM,N i, around a
closed curve C in a simple region D is
I
C
F · dr =
I
C
Mdx + Ndy =
ZZ
D
Nx − My dA (2)
10
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
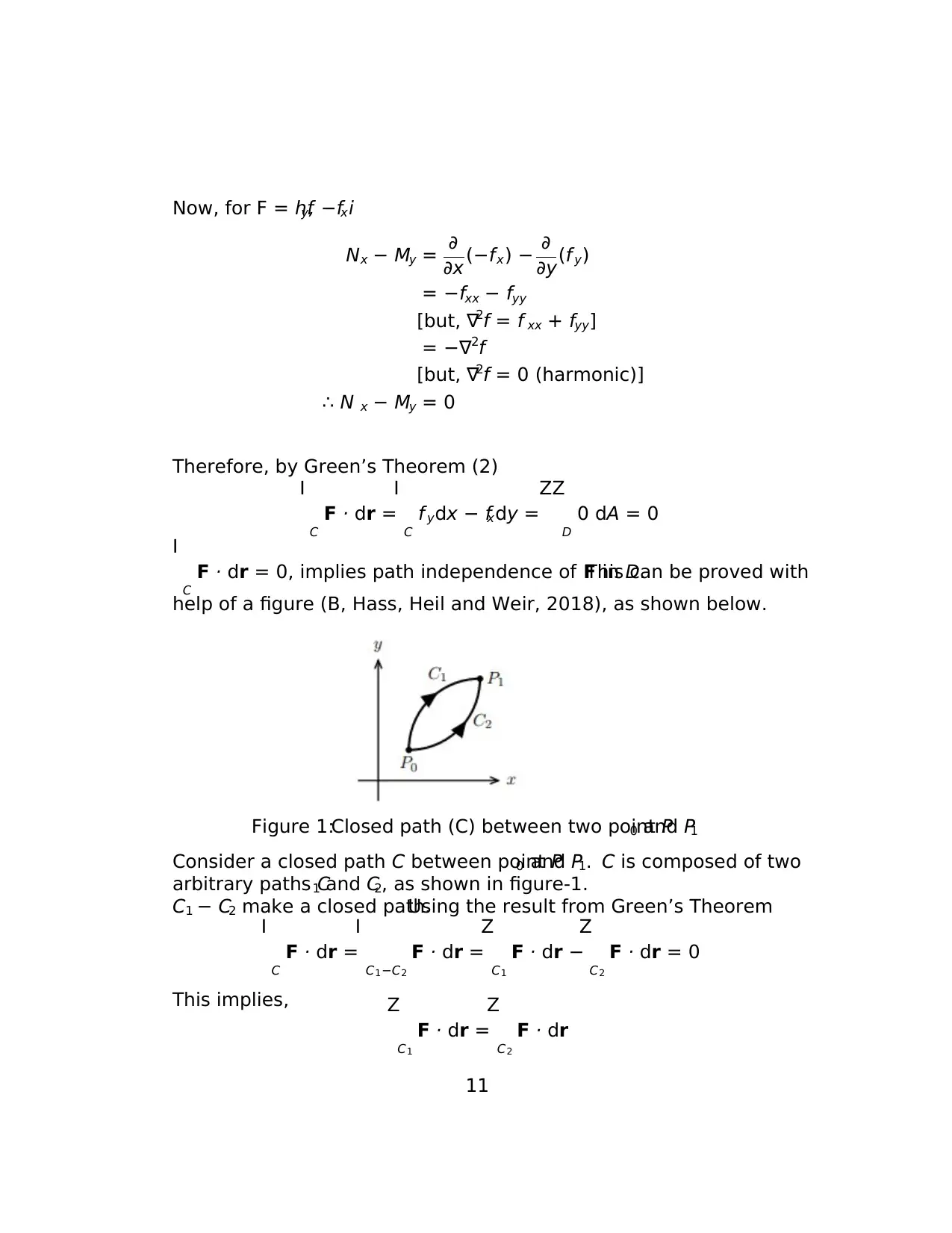
Now, for F = hfy, −fxi
Nx − My = ∂
∂x (−fx) − ∂
∂y(f y)
= −fxx − fyy
[but, ∇2f = f xx + fyy]
= −∇2f
[but, ∇2f = 0 (harmonic)]
∴ N x − My = 0
Therefore, by Green’s Theorem (2)
I
C
F · dr =
I
C
f ydx − fxdy =
ZZ
D
0 dA = 0
I
C
F · dr = 0, implies path independence of F in D.This can be proved with
help of a figure (B, Hass, Heil and Weir, 2018), as shown below.
Figure 1:Closed path (C) between two point P0 and P1
Consider a closed path C between point P0 and P1. C is composed of two
arbitrary paths C1 and C2, as shown in figure-1.
C1 − C2 make a closed path.Using the result from Green’s Theorem
I
C
F · dr =
I
C1−C2
F · dr =
Z
C1
F · dr −
Z
C2
F · dr = 0
This implies, Z
C1
F · dr =
Z
C2
F · dr
11
Nx − My = ∂
∂x (−fx) − ∂
∂y(f y)
= −fxx − fyy
[but, ∇2f = f xx + fyy]
= −∇2f
[but, ∇2f = 0 (harmonic)]
∴ N x − My = 0
Therefore, by Green’s Theorem (2)
I
C
F · dr =
I
C
f ydx − fxdy =
ZZ
D
0 dA = 0
I
C
F · dr = 0, implies path independence of F in D.This can be proved with
help of a figure (B, Hass, Heil and Weir, 2018), as shown below.
Figure 1:Closed path (C) between two point P0 and P1
Consider a closed path C between point P0 and P1. C is composed of two
arbitrary paths C1 and C2, as shown in figure-1.
C1 − C2 make a closed path.Using the result from Green’s Theorem
I
C
F · dr =
I
C1−C2
F · dr =
Z
C1
F · dr −
Z
C2
F · dr = 0
This implies, Z
C1
F · dr =
Z
C2
F · dr
11

which means, line integral joining any two points P0 and P1, is independent
of the path connecting P0 and P1. Hence proves path independence.
Thus the line integral I
C
f ydx − fxdy
is path independent in D, when f is harmonic.
Answer
a)
Given vector field is
F = hxy,yz, zxi
Stoke’s Theorem
According to B,Hass,Heil and Weir (2018),“Let S be a oriented smooth
surface that is bounded by a simple, closed, smooth boundary curve C with
positive orientation, then circulation of vector filed F around C is given by”
I
C
F · dr =
ZZ
S
curl(F) · ˆndS (1)
Surface S is a paraboloid given by:z = 1 − x2 − y2, in the first octant and C
is the boundary of S.We assume S to be positively oriented, which implies
12
of the path connecting P0 and P1. Hence proves path independence.
Thus the line integral I
C
f ydx − fxdy
is path independent in D, when f is harmonic.
Answer
a)
Given vector field is
F = hxy,yz, zxi
Stoke’s Theorem
According to B,Hass,Heil and Weir (2018),“Let S be a oriented smooth
surface that is bounded by a simple, closed, smooth boundary curve C with
positive orientation, then circulation of vector filed F around C is given by”
I
C
F · dr =
ZZ
S
curl(F) · ˆndS (1)
Surface S is a paraboloid given by:z = 1 − x2 − y2, in the first octant and C
is the boundary of S.We assume S to be positively oriented, which implies
12
You're viewing a preview
Unlock full access by subscribing today!
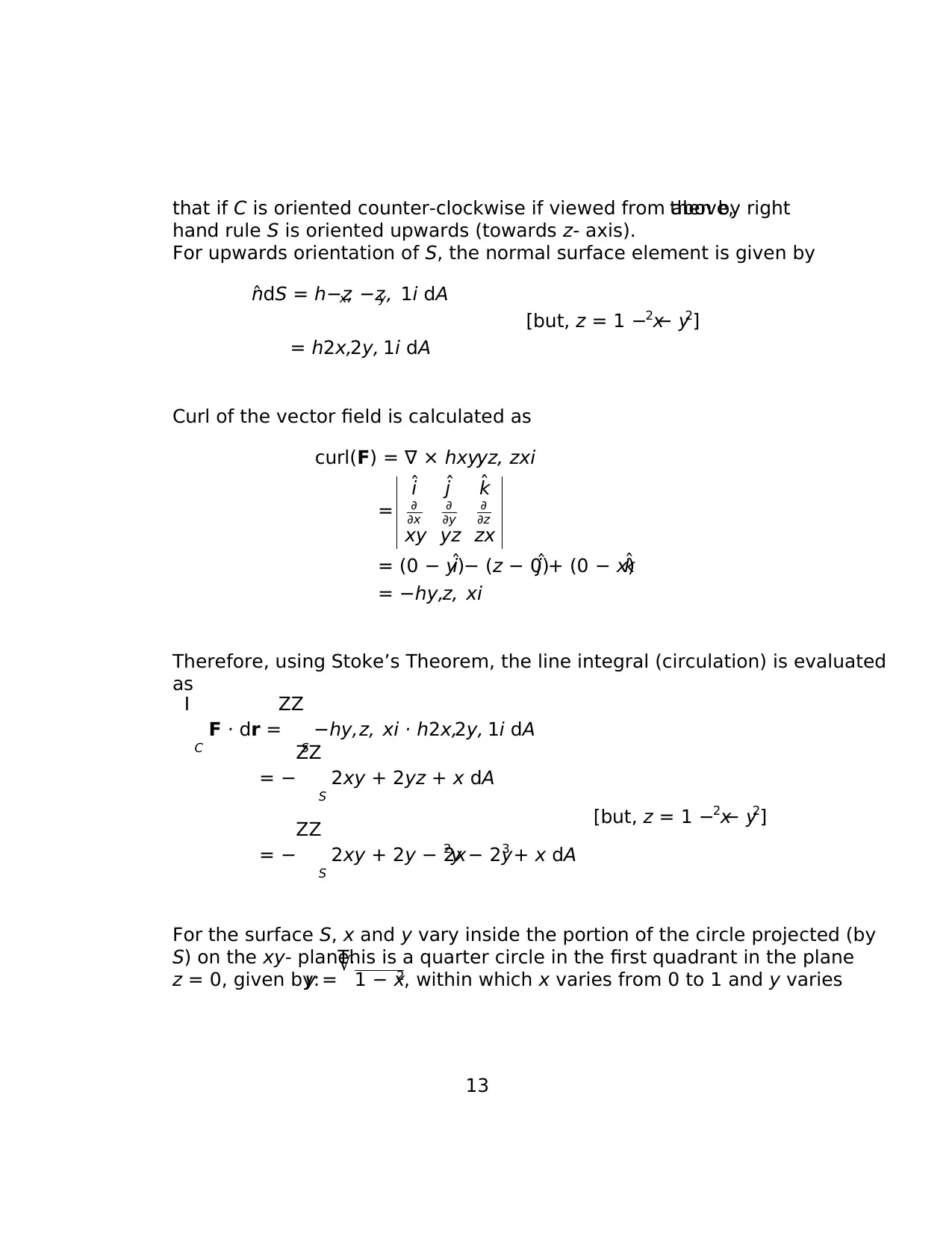
that if C is oriented counter-clockwise if viewed from above,then by right
hand rule S is oriented upwards (towards z- axis).
For upwards orientation of S, the normal surface element is given by
ˆndS = h−zx, −zy, 1i dA
[but, z = 1 − x2 − y2]
= h2x,2y, 1i dA
Curl of the vector field is calculated as
curl(F) = ∇ × hxy,yz, zxi
=
ˆi ˆj ˆk
∂
∂x
∂
∂y
∂
∂z
xy yz zx
= (0 − y)ˆi − (z − 0)ˆj + (0 − x)ˆk
= −hy,z, xi
Therefore, using Stoke’s Theorem, the line integral (circulation) is evaluated
as
I
C
F · dr =
ZZ
S
−hy,z, xi · h2x,2y, 1i dA
= −
ZZ
S
2xy + 2yz + x dA
[but, z = 1 − x2 − y2]
= −
ZZ
S
2xy + 2y − 2x2y − 2y3 + x dA
For the surface S, x and y vary inside the portion of the circle projected (by
S) on the xy- plane.This is a quarter circle in the first quadrant in the plane
z = 0, given by:y =√ 1 − x2, within which x varies from 0 to 1 and y varies
13
hand rule S is oriented upwards (towards z- axis).
For upwards orientation of S, the normal surface element is given by
ˆndS = h−zx, −zy, 1i dA
[but, z = 1 − x2 − y2]
= h2x,2y, 1i dA
Curl of the vector field is calculated as
curl(F) = ∇ × hxy,yz, zxi
=
ˆi ˆj ˆk
∂
∂x
∂
∂y
∂
∂z
xy yz zx
= (0 − y)ˆi − (z − 0)ˆj + (0 − x)ˆk
= −hy,z, xi
Therefore, using Stoke’s Theorem, the line integral (circulation) is evaluated
as
I
C
F · dr =
ZZ
S
−hy,z, xi · h2x,2y, 1i dA
= −
ZZ
S
2xy + 2yz + x dA
[but, z = 1 − x2 − y2]
= −
ZZ
S
2xy + 2y − 2x2y − 2y3 + x dA
For the surface S, x and y vary inside the portion of the circle projected (by
S) on the xy- plane.This is a quarter circle in the first quadrant in the plane
z = 0, given by:y =√ 1 − x2, within which x varies from 0 to 1 and y varies
13
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
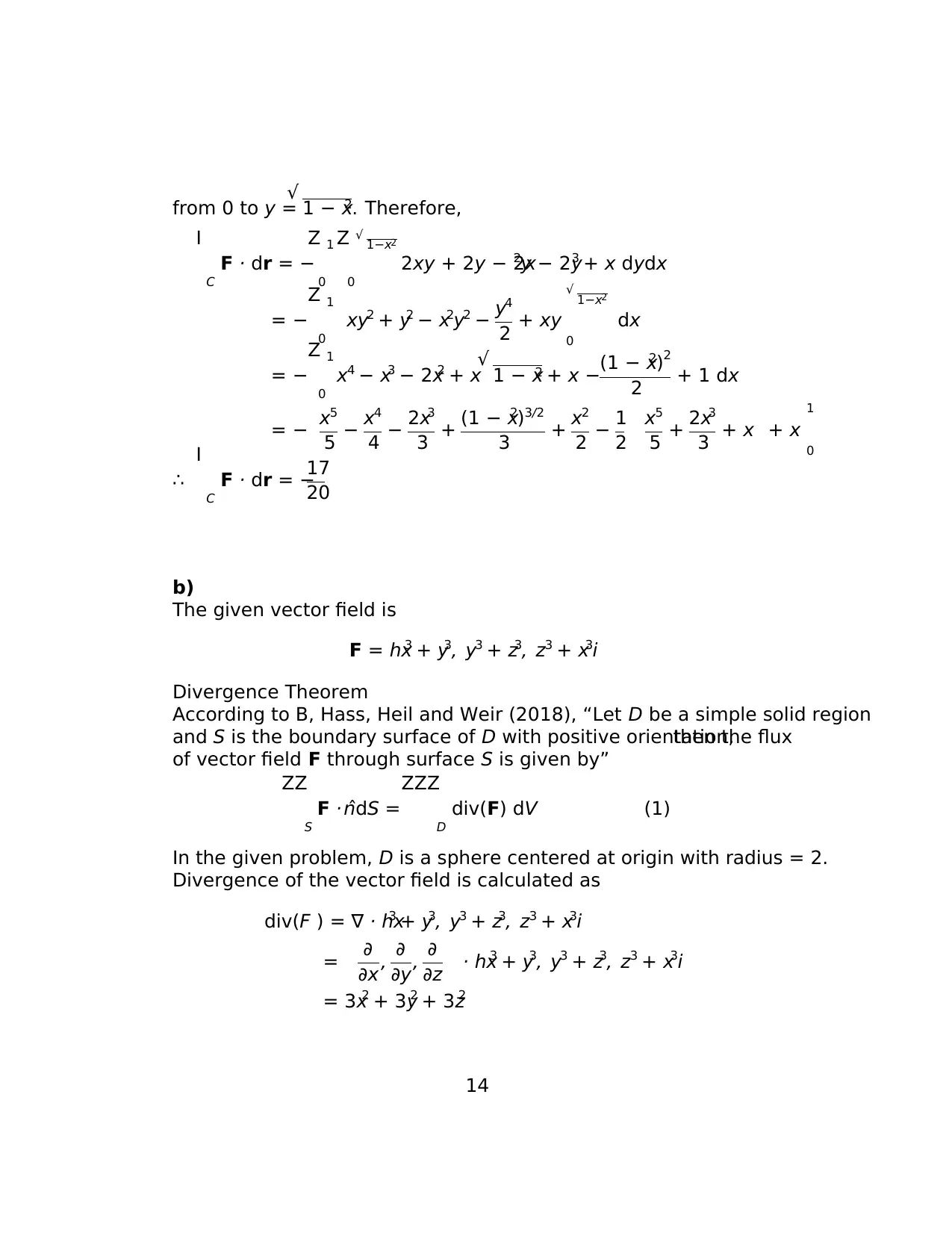
from 0 to y =
√ 1 − x2. Therefore,
I
C
F · dr = −
Z 1
0
Z √ 1−x2
0
2xy + 2y − 2x2y − 2y3 + x dydx
= −
Z 1
0
xy2 + y2 − x2y2 − y4
2 + xy
√ 1−x2
0
dx
= −
Z 1
0
x4 − x3 − 2x2 + x
√ 1 − x2 + x −(1 − x2)2
2 + 1 dx
= − x5
5 − x4
4 − 2x3
3 + (1 − x2)3/2
3 + x2
2 − 1
2
x5
5 + 2x3
3 + x + x
1
0
∴
I
C
F · dr = −
17
20
b)
The given vector field is
F = hx3 + y3, y3 + z3, z3 + x3i
Divergence Theorem
According to B, Hass, Heil and Weir (2018), “Let D be a simple solid region
and S is the boundary surface of D with positive orientation,then the flux
of vector field F through surface S is given by”
ZZ
S
F · ˆndS =
ZZZ
D
div(F) dV (1)
In the given problem, D is a sphere centered at origin with radius = 2.
Divergence of the vector field is calculated as
div(F ) = ∇ · hx3 + y3, y3 + z3, z3 + x3i
= ∂
∂x , ∂
∂y, ∂
∂z · hx3 + y3, y3 + z3, z3 + x3i
= 3x2 + 3y2 + 3z2
14
√ 1 − x2. Therefore,
I
C
F · dr = −
Z 1
0
Z √ 1−x2
0
2xy + 2y − 2x2y − 2y3 + x dydx
= −
Z 1
0
xy2 + y2 − x2y2 − y4
2 + xy
√ 1−x2
0
dx
= −
Z 1
0
x4 − x3 − 2x2 + x
√ 1 − x2 + x −(1 − x2)2
2 + 1 dx
= − x5
5 − x4
4 − 2x3
3 + (1 − x2)3/2
3 + x2
2 − 1
2
x5
5 + 2x3
3 + x + x
1
0
∴
I
C
F · dr = −
17
20
b)
The given vector field is
F = hx3 + y3, y3 + z3, z3 + x3i
Divergence Theorem
According to B, Hass, Heil and Weir (2018), “Let D be a simple solid region
and S is the boundary surface of D with positive orientation,then the flux
of vector field F through surface S is given by”
ZZ
S
F · ˆndS =
ZZZ
D
div(F) dV (1)
In the given problem, D is a sphere centered at origin with radius = 2.
Divergence of the vector field is calculated as
div(F ) = ∇ · hx3 + y3, y3 + z3, z3 + x3i
= ∂
∂x , ∂
∂y, ∂
∂z · hx3 + y3, y3 + z3, z3 + x3i
= 3x2 + 3y2 + 3z2
14
Therefore, by Divergence Theorem
ZZ
S
F · ˆndS =
ZZZ
D
3x2 + 3y2 + 3z2 dV
For convenience, switch to spherical coordinates (r, θ, φ).
x = r cos θ sin φ,y = r sin θ sin φ,z = r cos φ
Also,
x2 + y2 + z2 = r2 & dV = r 2 sin φ dφdrdθ
Within D, r varies from 0 to 2, φ varies from 0 to π and θ varies from 0 to
2π.
Therefore, ZZ
S
F · ˆndS =
ZZZ
D
3r2 · r2 sin φdφdrdθ
[Applying limits]
= 3
Z 2π
0
Z 2
0
Z π
0
r4 sin φ dφdrdθ
= 3
Z 2π
0
Z 2
0
r4
h
− cos φ
i π
0
drdθ
= 3
Z 2π
0
Z 2
0
2r4 drdθ
= 6
Z 2π
0
r5
5
2
0
dθ
= 192
5
Z 2π
0
dθ
∴
ZZ
S
F · ˆndS = 384π
5
15
ZZ
S
F · ˆndS =
ZZZ
D
3x2 + 3y2 + 3z2 dV
For convenience, switch to spherical coordinates (r, θ, φ).
x = r cos θ sin φ,y = r sin θ sin φ,z = r cos φ
Also,
x2 + y2 + z2 = r2 & dV = r 2 sin φ dφdrdθ
Within D, r varies from 0 to 2, φ varies from 0 to π and θ varies from 0 to
2π.
Therefore, ZZ
S
F · ˆndS =
ZZZ
D
3r2 · r2 sin φdφdrdθ
[Applying limits]
= 3
Z 2π
0
Z 2
0
Z π
0
r4 sin φ dφdrdθ
= 3
Z 2π
0
Z 2
0
r4
h
− cos φ
i π
0
drdθ
= 3
Z 2π
0
Z 2
0
2r4 drdθ
= 6
Z 2π
0
r5
5
2
0
dθ
= 192
5
Z 2π
0
dθ
∴
ZZ
S
F · ˆndS = 384π
5
15
You're viewing a preview
Unlock full access by subscribing today!
Answer
Fundamental Theorem of line integrals states
Z b
a
∇f (r(t)) · r0
(t) = f (r(b)) − f (r(a)) (1)
where a and b are end points of a path C and r(t) is a vector valued function
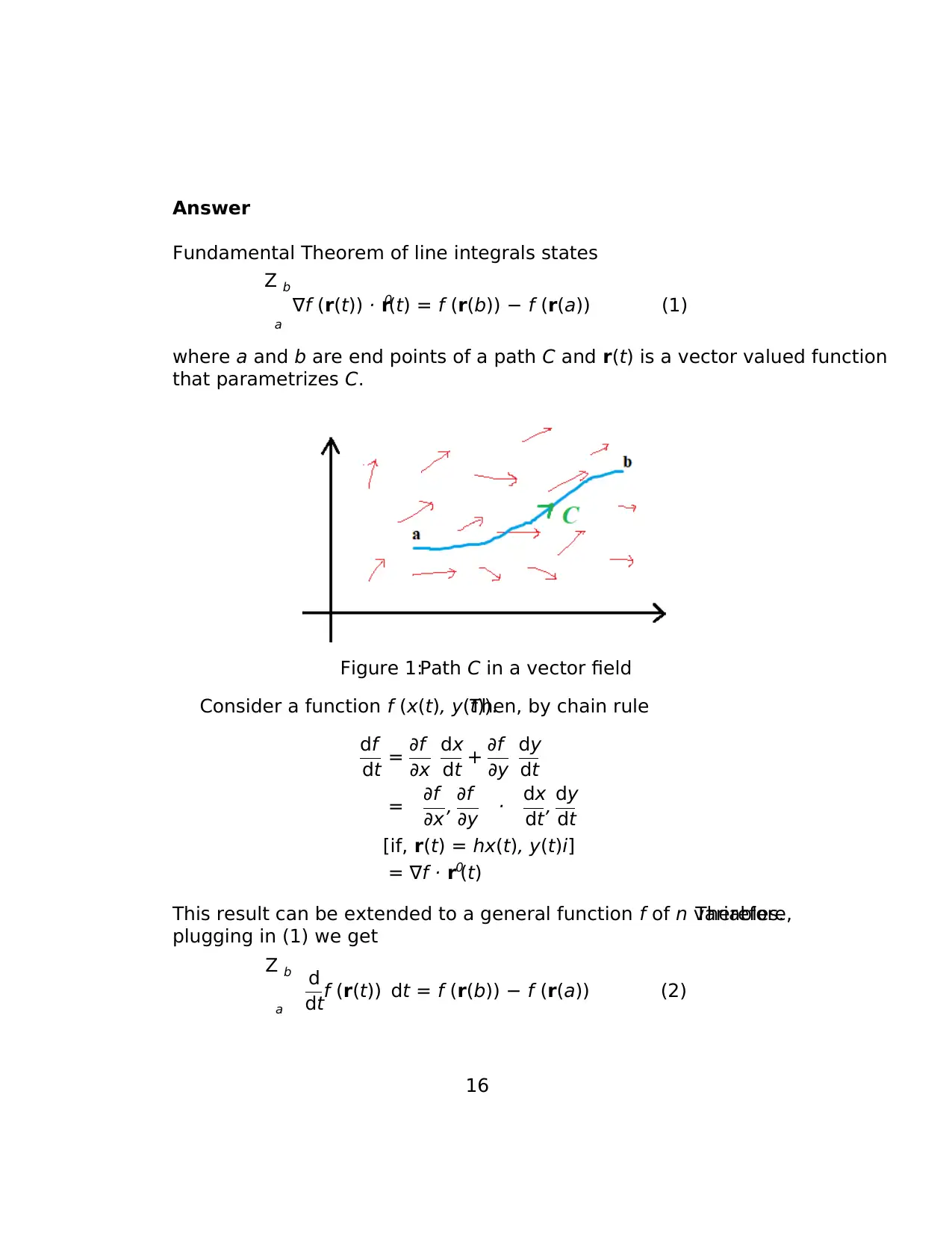
that parametrizes C.
Figure 1:Path C in a vector field
Consider a function f (x(t), y(t)).Then, by chain rule
df
dt = ∂f
∂x
dx
dt + ∂f
∂y
dy
dt
= ∂f
∂x , ∂f
∂y · dx
dt, dy
dt
[if, r(t) = hx(t), y(t)i]
= ∇f · r0
(t)
This result can be extended to a general function f of n variables.Therefore,
plugging in (1) we get
Z b
a
d
dtf (r(t)) dt = f (r(b)) − f (r(a)) (2)
16
Fundamental Theorem of line integrals states
Z b
a
∇f (r(t)) · r0
(t) = f (r(b)) − f (r(a)) (1)
where a and b are end points of a path C and r(t) is a vector valued function
that parametrizes C.
Figure 1:Path C in a vector field
Consider a function f (x(t), y(t)).Then, by chain rule
df
dt = ∂f
∂x
dx
dt + ∂f
∂y
dy
dt
= ∂f
∂x , ∂f
∂y · dx
dt, dy
dt
[if, r(t) = hx(t), y(t)i]
= ∇f · r0
(t)
This result can be extended to a general function f of n variables.Therefore,
plugging in (1) we get
Z b
a
d
dtf (r(t)) dt = f (r(b)) − f (r(a)) (2)
16
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
Fundamental Theorem of Calculus for single variable states
Z b
a
d
dtg(t) dt = g(b) − g(a) (3)
Fundamental Theorem for line integral (2) takes a form very similar to Funda-
mental Theorem of Calculus (3), but in (2) the function f is being evaluated
along path C parametrized by r(t) while in (1), the function g is being inte-
grated along the horizontal t axis.
To further understand the equivalence between the two Theorems, consider
a simple example of function h(t) = sin t,along the horizontalt- axis from
t = 0 to t = π/2.
By the Fundamental Theorem of Calculus (3)
Z b
a
d
dt sin(t) dt = sin (π/2) − sin (0) = 1
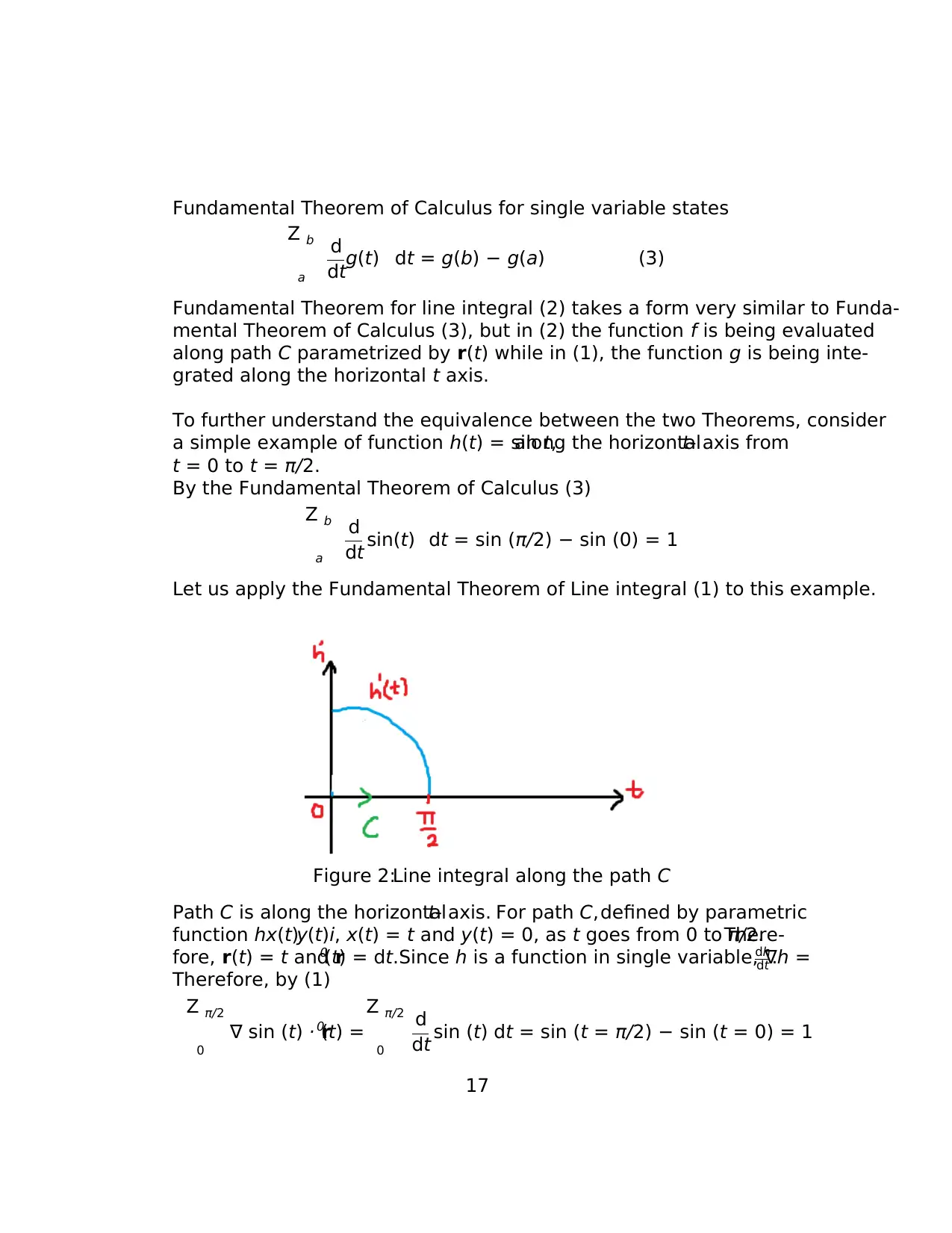
Let us apply the Fundamental Theorem of Line integral (1) to this example.
Figure 2:Line integral along the path C
Path C is along the horizontalt- axis. For path C,defined by parametric
function hx(t),y(t)i, x(t) = t and y(t) = 0, as t goes from 0 to π/2.There-
fore, r(t) = t and r0
(t) = dt.Since h is a function in single variable, ∇h =dh
dt .
Therefore, by (1)
Z π/2
0
∇ sin (t) · r0
(t) =
Z π/2
0
d
dt sin (t) dt = sin (t = π/2) − sin (t = 0) = 1
17
Z b
a
d
dtg(t) dt = g(b) − g(a) (3)
Fundamental Theorem for line integral (2) takes a form very similar to Funda-
mental Theorem of Calculus (3), but in (2) the function f is being evaluated
along path C parametrized by r(t) while in (1), the function g is being inte-
grated along the horizontal t axis.
To further understand the equivalence between the two Theorems, consider
a simple example of function h(t) = sin t,along the horizontalt- axis from
t = 0 to t = π/2.
By the Fundamental Theorem of Calculus (3)
Z b
a
d
dt sin(t) dt = sin (π/2) − sin (0) = 1
Let us apply the Fundamental Theorem of Line integral (1) to this example.
Figure 2:Line integral along the path C
Path C is along the horizontalt- axis. For path C,defined by parametric
function hx(t),y(t)i, x(t) = t and y(t) = 0, as t goes from 0 to π/2.There-
fore, r(t) = t and r0
(t) = dt.Since h is a function in single variable, ∇h =dh
dt .
Therefore, by (1)
Z π/2
0
∇ sin (t) · r0
(t) =
Z π/2
0
d
dt sin (t) dt = sin (t = π/2) − sin (t = 0) = 1
17
Answer
Stoke’s Theorem is a 3D generalization of Green’s Theorem for work done in
2D.
According to B, Hass,Heil and Weir (2018),“Green’s Theorem for work
done by force F = hM,N i, along a closed curve C says”
I
C
F · dr =
I
C
M dx + N dy =
ZZ
D
Nx − My dA (1)
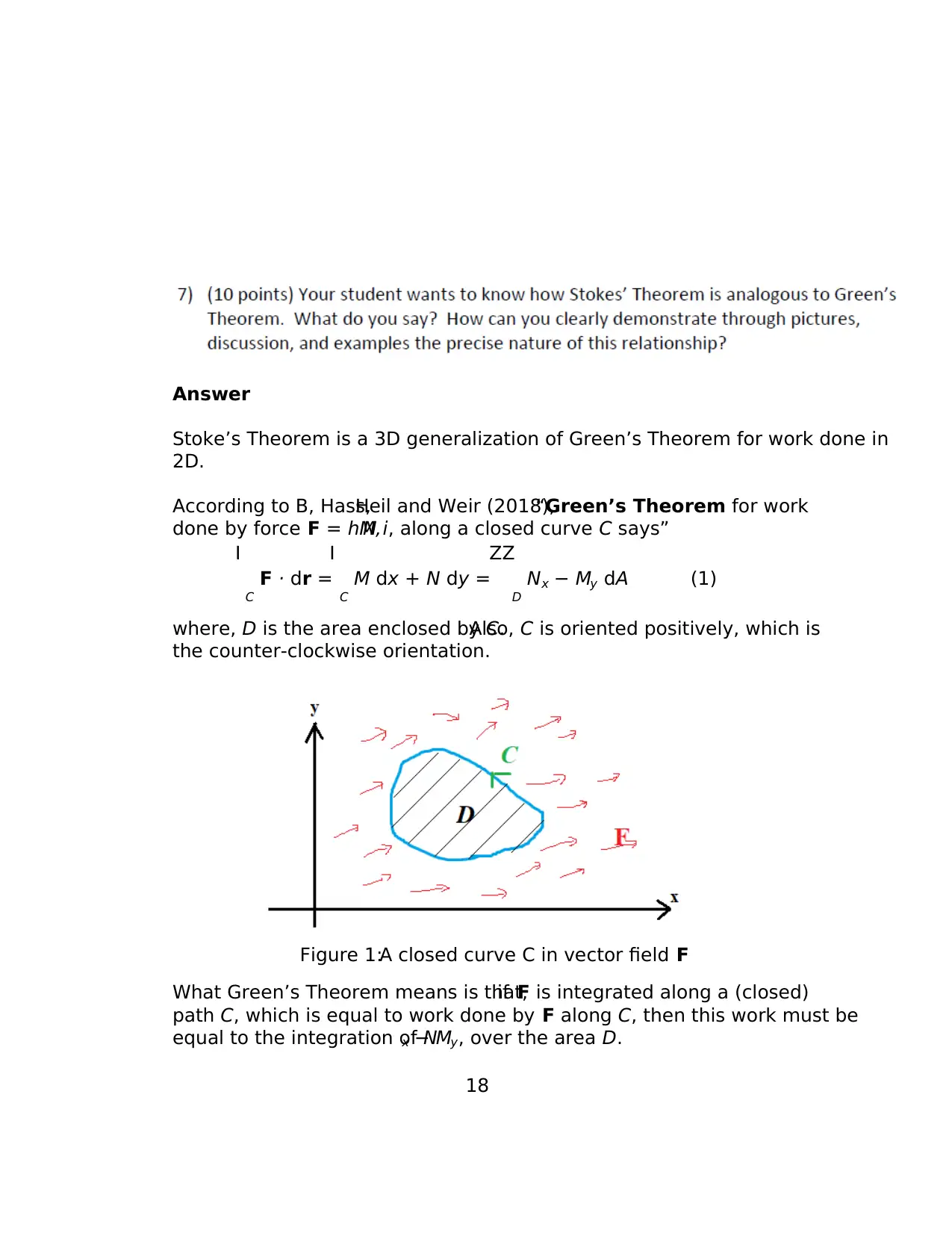
where, D is the area enclosed by C.Also, C is oriented positively, which is
the counter-clockwise orientation.
Figure 1:A closed curve C in vector field F
What Green’s Theorem means is that,if F is integrated along a (closed)
path C, which is equal to work done by F along C, then this work must be
equal to the integration of Nx − My, over the area D.
18
Stoke’s Theorem is a 3D generalization of Green’s Theorem for work done in
2D.
According to B, Hass,Heil and Weir (2018),“Green’s Theorem for work
done by force F = hM,N i, along a closed curve C says”
I
C
F · dr =
I
C
M dx + N dy =
ZZ
D
Nx − My dA (1)
where, D is the area enclosed by C.Also, C is oriented positively, which is
the counter-clockwise orientation.
Figure 1:A closed curve C in vector field F
What Green’s Theorem means is that,if F is integrated along a (closed)
path C, which is equal to work done by F along C, then this work must be
equal to the integration of Nx − My, over the area D.
18
You're viewing a preview
Unlock full access by subscribing today!

Stoke’s Theorem:According to B,Hass,Heil and Weir (2018),“Let C be
any closed curve and let S be any surface bounding C.Let F be a vector
field on S, then”
I
C
F · dr =
ZZ
S
curl(F) · ˆndS (2)
S is an oriented surface and it’s orientation is determined using right hand
rule (will be demonstrated shortly).
There is some similarity visible between the Green’s Theorem (1) and the
Stoke’s Theorem (2),at this point.The line integralon the left side of (1)
and (2) look same, but they are not necessarily the same as F in (2) is in the
more general 3D form.The right side of (1) and (2) look completely different
at this point, but they too are similar and will be demonstrated through an
example.
To demonstrate the equivalence between these two Theorems,let us again
consider the example that was used for Green’s Theorem and apply Stoke’s
Theorem this time.
F = hM, Ni
As shown in figure-1, surface D is bounded by curve C.
The orientation of D is determined using right hand rule.It states that,if
our index finger points along C,the middle finger points into D then the
thumb points in the direction ofsurface normalˆn. This implies that D is
positively oriented upwards (towards z- axis).Since the z- axis is normal to
D (as it lies in xy- plane),ˆn = ˆk.
Therefore, by Stoke’s Theorem
I
C
F · dr =
ZZ
D
curl(F) ·ˆkdA
as D lies in the xy- plane, we replace dS by dA.
Curl of F is
curl(F) = h0,0, Nx − Myi
19
any closed curve and let S be any surface bounding C.Let F be a vector
field on S, then”
I
C
F · dr =
ZZ
S
curl(F) · ˆndS (2)
S is an oriented surface and it’s orientation is determined using right hand
rule (will be demonstrated shortly).
There is some similarity visible between the Green’s Theorem (1) and the
Stoke’s Theorem (2),at this point.The line integralon the left side of (1)
and (2) look same, but they are not necessarily the same as F in (2) is in the
more general 3D form.The right side of (1) and (2) look completely different
at this point, but they too are similar and will be demonstrated through an
example.
To demonstrate the equivalence between these two Theorems,let us again
consider the example that was used for Green’s Theorem and apply Stoke’s
Theorem this time.
F = hM, Ni
As shown in figure-1, surface D is bounded by curve C.
The orientation of D is determined using right hand rule.It states that,if
our index finger points along C,the middle finger points into D then the
thumb points in the direction ofsurface normalˆn. This implies that D is
positively oriented upwards (towards z- axis).Since the z- axis is normal to
D (as it lies in xy- plane),ˆn = ˆk.
Therefore, by Stoke’s Theorem
I
C
F · dr =
ZZ
D
curl(F) ·ˆkdA
as D lies in the xy- plane, we replace dS by dA.
Curl of F is
curl(F) = h0,0, Nx − Myi
19
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Therefore,
I
C
F · dr =
ZZ
D
h0,0, Nx − Myi · ˆkdA =
ZZ
D
Nx − My dA
We got the same result as the Green’s Theorem, by applying Stoke’s Theo-
rem to the problem.
References:
B, G., Hass,J., Heil, C. R. and Weir,M. D. (2018). Thomas’Calculus:
Multivariable.Boston:Pearson.
Zill, D. G., Wright,W. S. (2011).Multivariable Calculus.Sudbury,Mass:
Jones and Bartlett Publishers.
20
I
C
F · dr =
ZZ
D
h0,0, Nx − Myi · ˆkdA =
ZZ
D
Nx − My dA
We got the same result as the Green’s Theorem, by applying Stoke’s Theo-
rem to the problem.
References:
B, G., Hass,J., Heil, C. R. and Weir,M. D. (2018). Thomas’Calculus:
Multivariable.Boston:Pearson.
Zill, D. G., Wright,W. S. (2011).Multivariable Calculus.Sudbury,Mass:
Jones and Bartlett Publishers.
20
1 out of 20
Related Documents
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





