INDUSTRIAL INSTRUMENTATION.
VerifiedAdded on 2023/01/12
|4
|480
|24
AI Summary
ITS AN PRACTICAL ASSIGNMENT INVOLVED WITH P AND ID DIAGRAM PLEASE READ THE ATTACHED DOCUMENT
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.
INDUSTRIAL INSTRUMENTATION
Author
Department
Institution
City, Country
Address
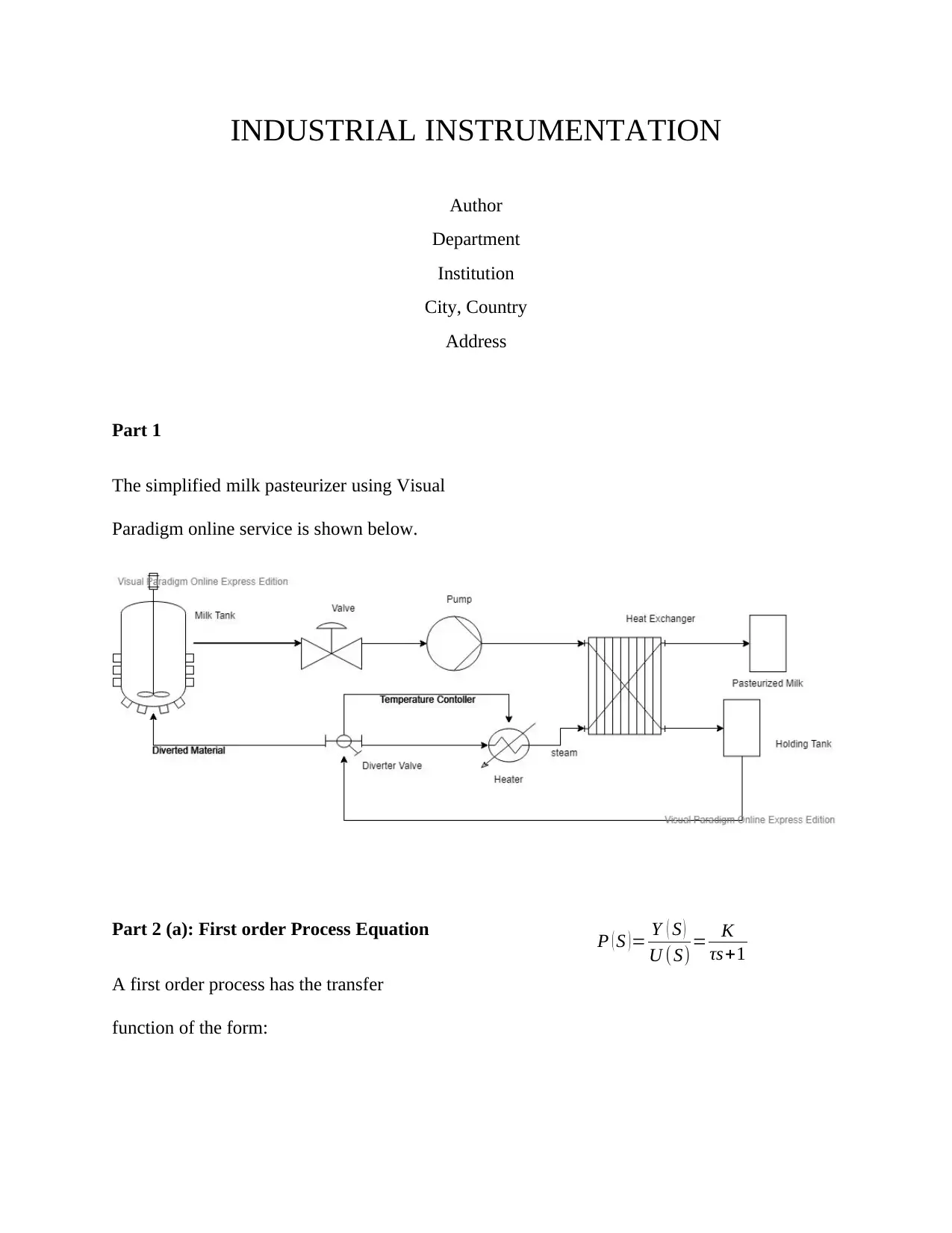
Part 1
The simplified milk pasteurizer using Visual
Paradigm online service is shown below.
Part 2 (a): First order Process Equation
A first order process has the transfer
function of the form:
P ( S ) = Y ( S )
U ( S) = K
τs+1
Author
Department
Institution
City, Country
Address
Part 1
The simplified milk pasteurizer using Visual
Paradigm online service is shown below.
Part 2 (a): First order Process Equation
A first order process has the transfer
function of the form:
P ( S ) = Y ( S )
U ( S) = K
τs+1
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
Where K represents the DC gain and τ is the
time constant of the first order process.
From the given data, the ambient
temperature (initial temperature) is 19 ℃
while the maximum (steady state)
temperature is 80 ℃. Therefore, the DC gain
is approximately equal to:
K=80−19=61℃
From the definition of time constant, we
know that it is the time required for the
temperature to rise to 63.2% of the final
value.
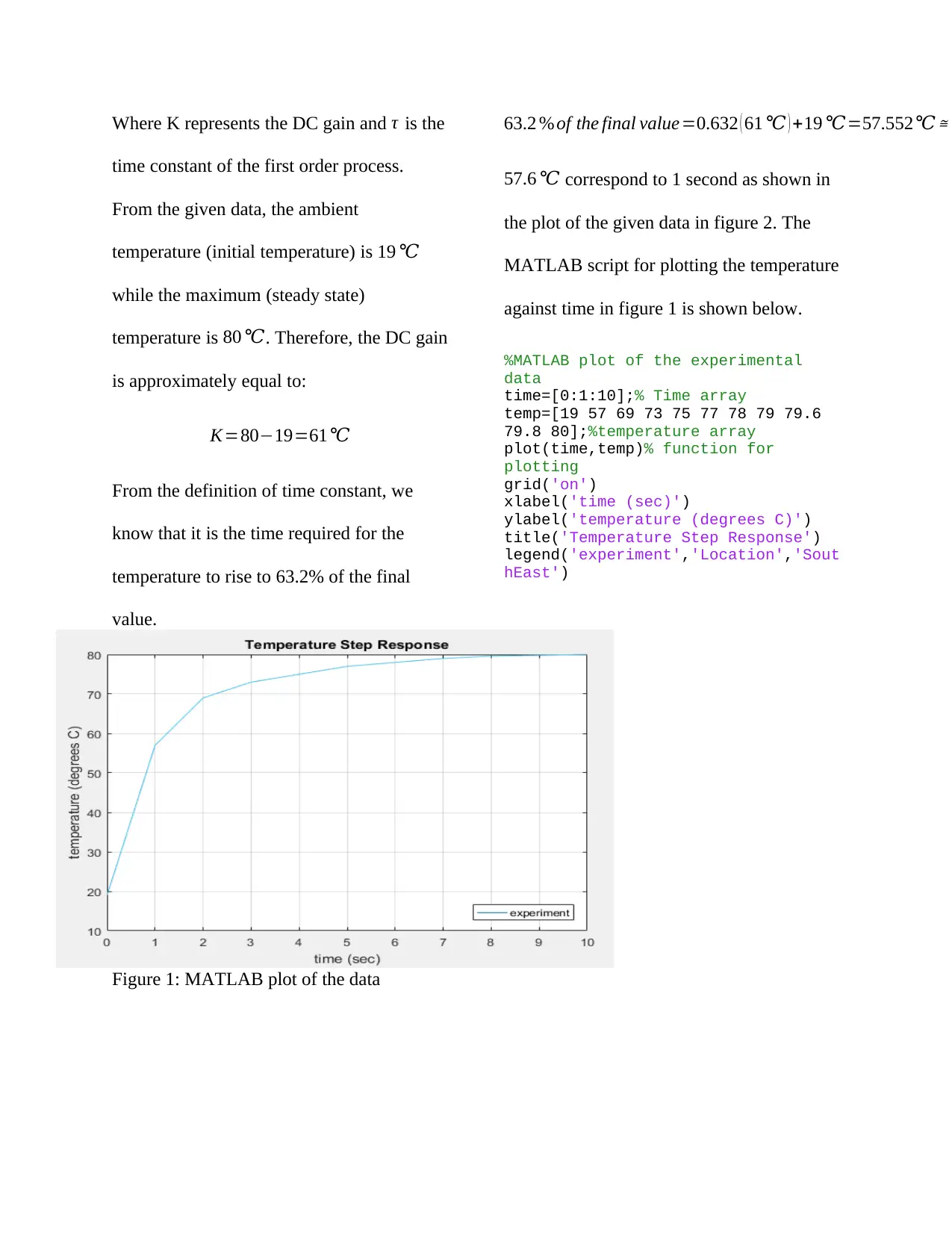
63.2 % of the final value=0.632 ( 61 ℃ ) +19 ℃=57.552℃ ≅ 5
57.6 ℃ correspond to 1 second as shown in
the plot of the given data in figure 2. The
MATLAB script for plotting the temperature
against time in figure 1 is shown below.
%MATLAB plot of the experimental
data
time=[0:1:10];% Time array
temp=[19 57 69 73 75 77 78 79 79.6
79.8 80];%temperature array
plot(time,temp)% function for
plotting
grid('on')
xlabel('time (sec)')
ylabel('temperature (degrees C)')
title('Temperature Step Response')
legend('experiment','Location','Sout
hEast')
Figure 1: MATLAB plot of the data
time constant of the first order process.
From the given data, the ambient
temperature (initial temperature) is 19 ℃
while the maximum (steady state)
temperature is 80 ℃. Therefore, the DC gain
is approximately equal to:
K=80−19=61℃
From the definition of time constant, we
know that it is the time required for the
temperature to rise to 63.2% of the final
value.
63.2 % of the final value=0.632 ( 61 ℃ ) +19 ℃=57.552℃ ≅ 5
57.6 ℃ correspond to 1 second as shown in
the plot of the given data in figure 2. The
MATLAB script for plotting the temperature
against time in figure 1 is shown below.
%MATLAB plot of the experimental
data
time=[0:1:10];% Time array
temp=[19 57 69 73 75 77 78 79 79.6
79.8 80];%temperature array
plot(time,temp)% function for
plotting
grid('on')
xlabel('time (sec)')
ylabel('temperature (degrees C)')
title('Temperature Step Response')
legend('experiment','Location','Sout
hEast')
Figure 1: MATLAB plot of the data
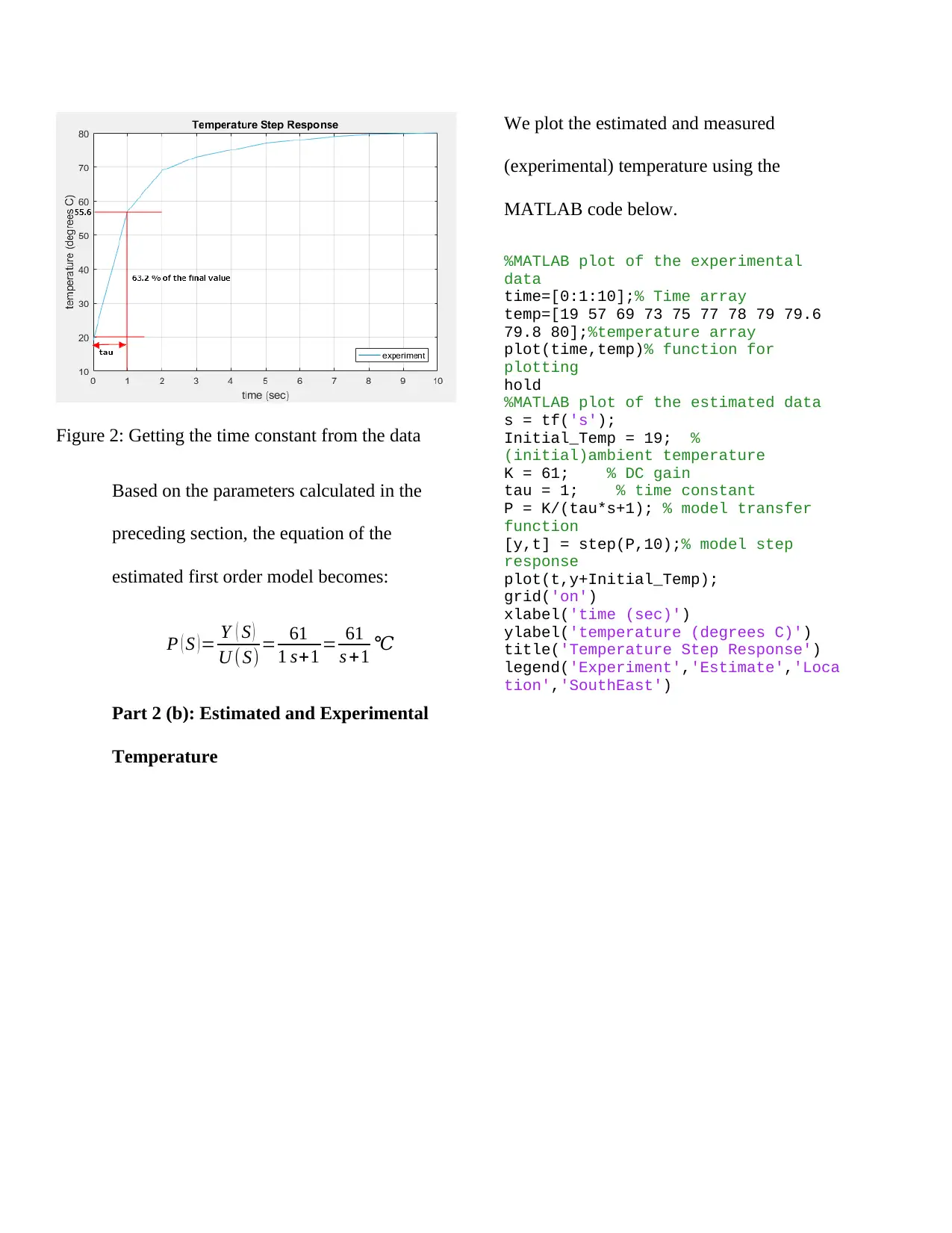
Figure 2: Getting the time constant from the data
Based on the parameters calculated in the
preceding section, the equation of the
estimated first order model becomes:
P ( S ) = Y ( S )
U ( S) = 61
1 s+1 = 61
s +1 ℃
Part 2 (b): Estimated and Experimental
Temperature
We plot the estimated and measured
(experimental) temperature using the
MATLAB code below.
%MATLAB plot of the experimental
data
time=[0:1:10];% Time array
temp=[19 57 69 73 75 77 78 79 79.6
79.8 80];%temperature array
plot(time,temp)% function for
plotting
hold
%MATLAB plot of the estimated data
s = tf('s');
Initial_Temp = 19; %
(initial)ambient temperature
K = 61; % DC gain
tau = 1; % time constant
P = K/(tau*s+1); % model transfer
function
[y,t] = step(P,10);% model step
response
plot(t,y+Initial_Temp);
grid('on')
xlabel('time (sec)')
ylabel('temperature (degrees C)')
title('Temperature Step Response')
legend('Experiment','Estimate','Loca
tion','SouthEast')
Based on the parameters calculated in the
preceding section, the equation of the
estimated first order model becomes:
P ( S ) = Y ( S )
U ( S) = 61
1 s+1 = 61
s +1 ℃
Part 2 (b): Estimated and Experimental
Temperature
We plot the estimated and measured
(experimental) temperature using the
MATLAB code below.
%MATLAB plot of the experimental
data
time=[0:1:10];% Time array
temp=[19 57 69 73 75 77 78 79 79.6
79.8 80];%temperature array
plot(time,temp)% function for
plotting
hold
%MATLAB plot of the estimated data
s = tf('s');
Initial_Temp = 19; %
(initial)ambient temperature
K = 61; % DC gain
tau = 1; % time constant
P = K/(tau*s+1); % model transfer
function
[y,t] = step(P,10);% model step
response
plot(t,y+Initial_Temp);
grid('on')
xlabel('time (sec)')
ylabel('temperature (degrees C)')
title('Temperature Step Response')
legend('Experiment','Estimate','Loca
tion','SouthEast')
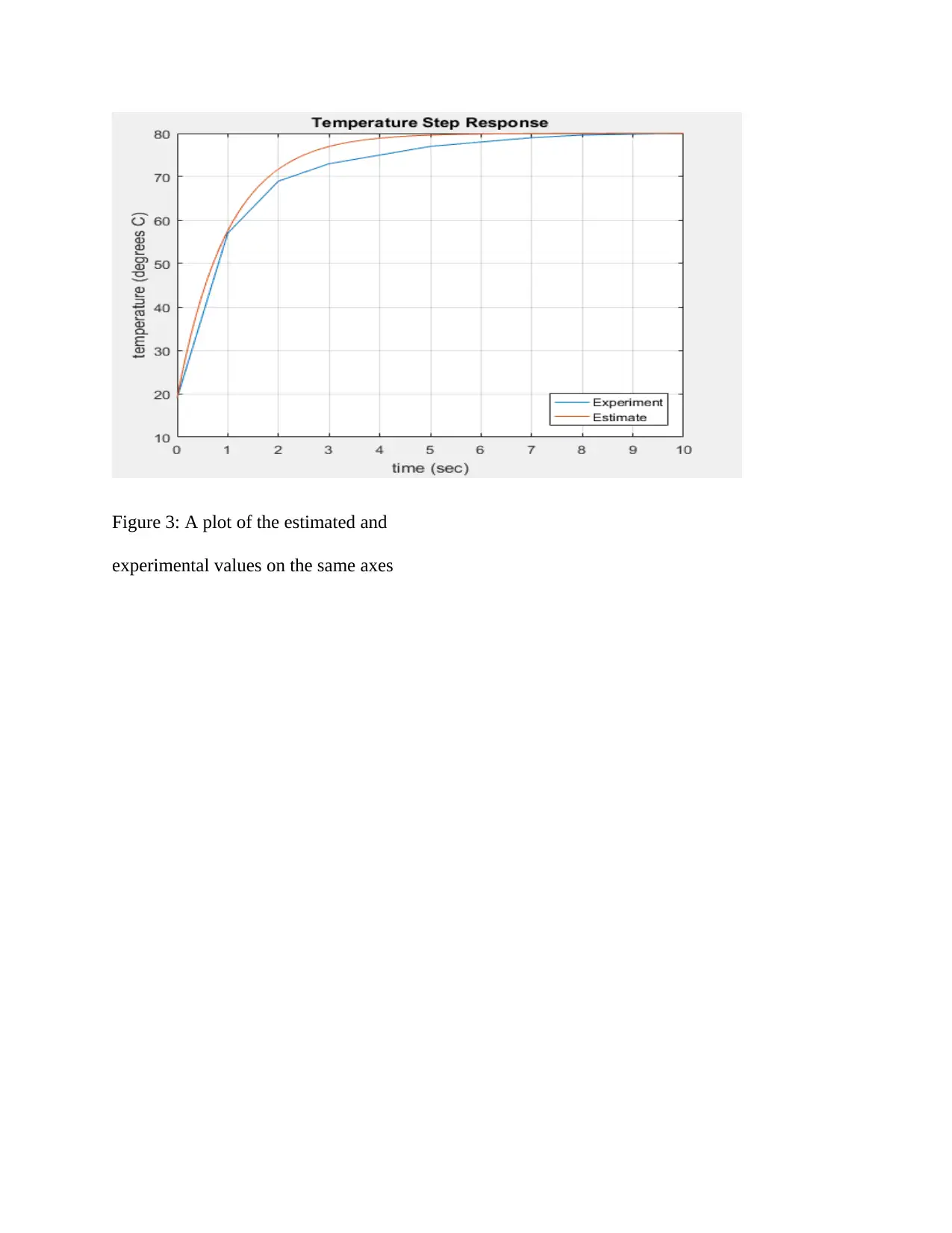
Figure 3: A plot of the estimated and
experimental values on the same axes
experimental values on the same axes
1 out of 4
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.
