Math Homework: Solving Inverse Functions and Logarithmic Equations
VerifiedAdded on 2023/06/10
|9
|1540
|310
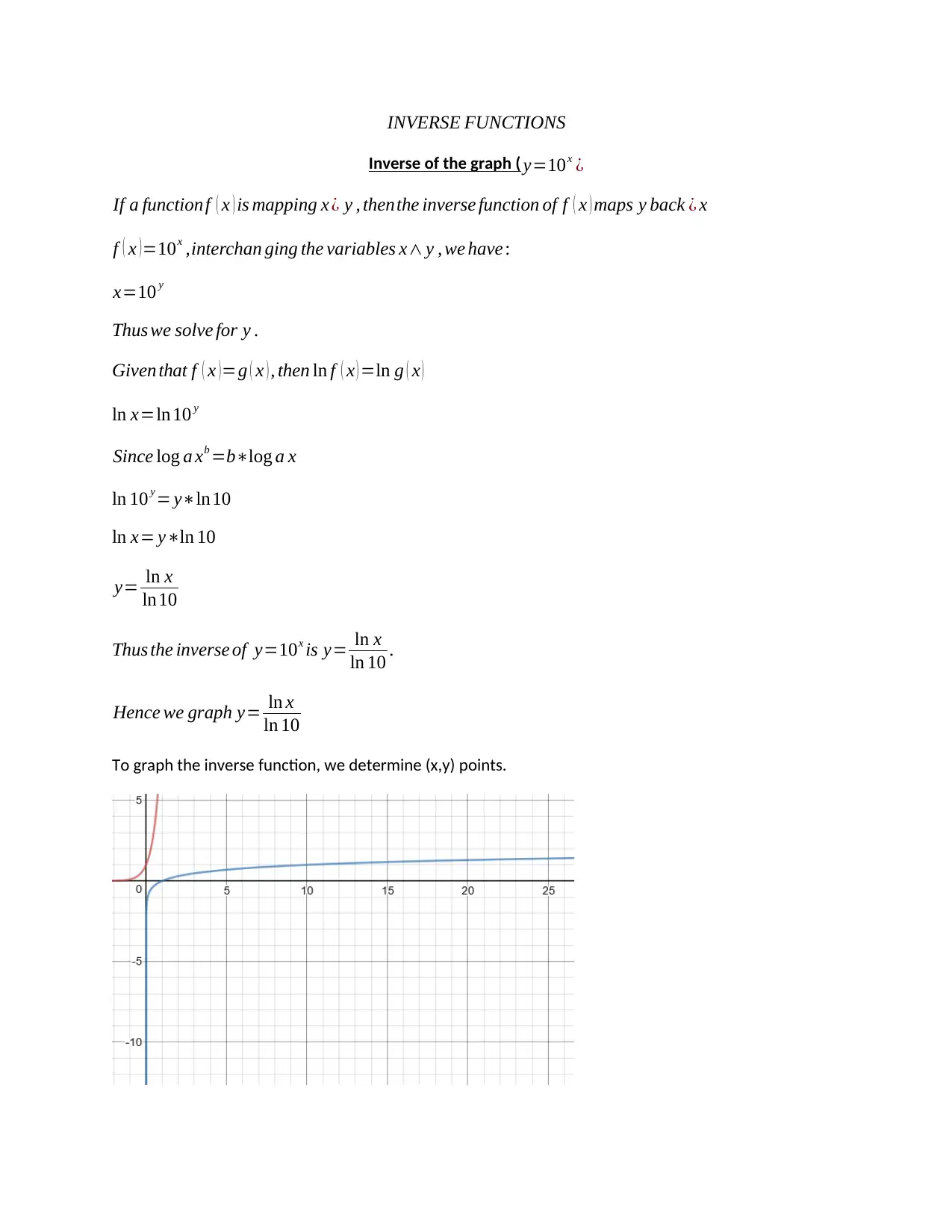
Homework Assignment
AI Summary
This assignment provides detailed solutions to various problems related to inverse functions and logarithmic equations. The solutions cover finding the inverse of different functions, including exponential and polynomial functions, and verifying inverse relationships through composition. The assignment also addresses logarithmic functions, including solving equations using logarithmic properties, the change of base formula, and expanding and contracting logarithmic expressions. Furthermore, the document includes solutions for solving exponential and logarithmic equations, applying logarithms to real-world problems involving compound interest and exponential decay, and determining the time it takes for a quantity to change based on an exponential model. The assignment demonstrates a solid understanding of algebraic concepts and problem-solving techniques.
1 out of 9












![[object Object]](/_next/static/media/star-bottom.7253800d.svg)