Vibrations & Damping in Mechanical Engineering: Problem Set
VerifiedAdded on 2023/04/23
|3
|728
|299
Homework Assignment
AI Summary
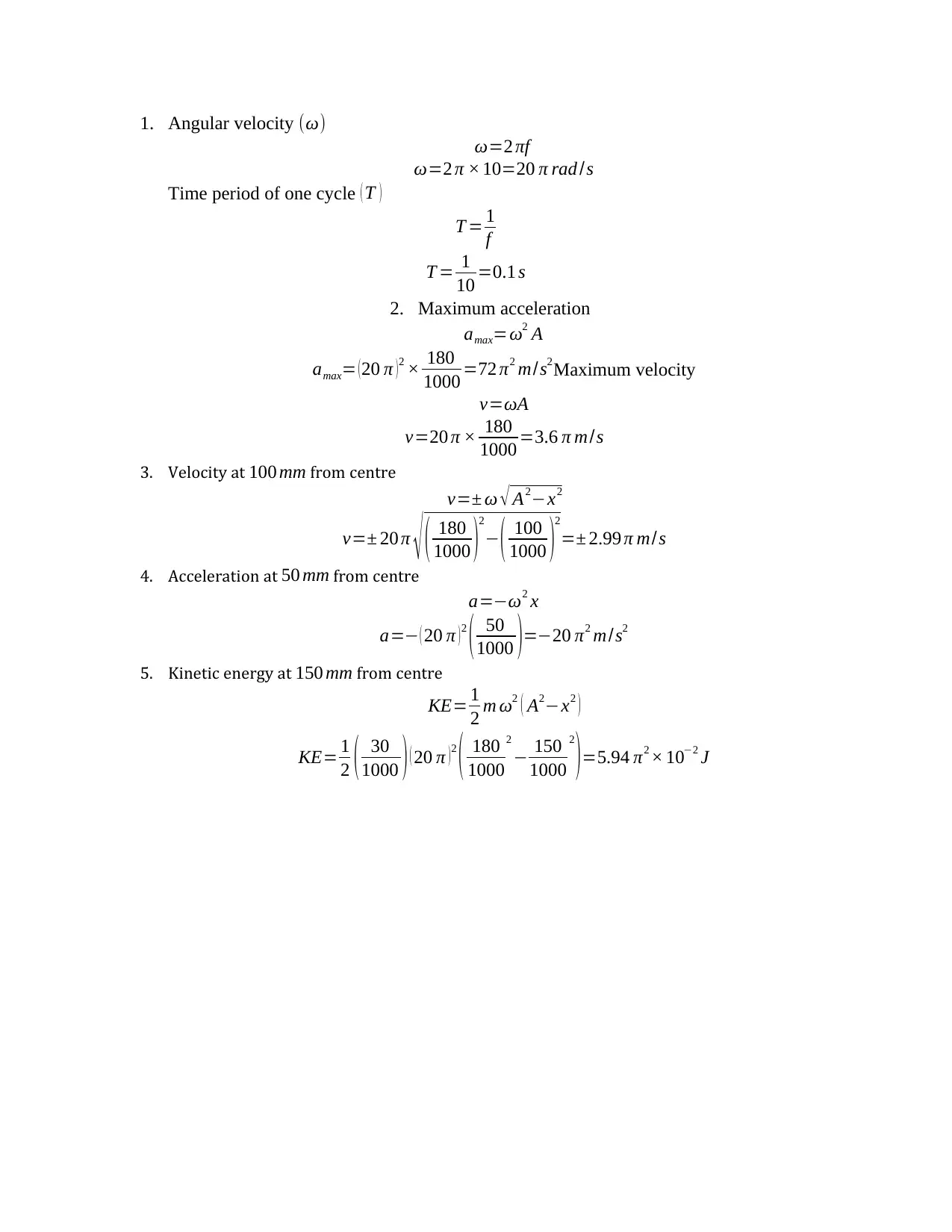
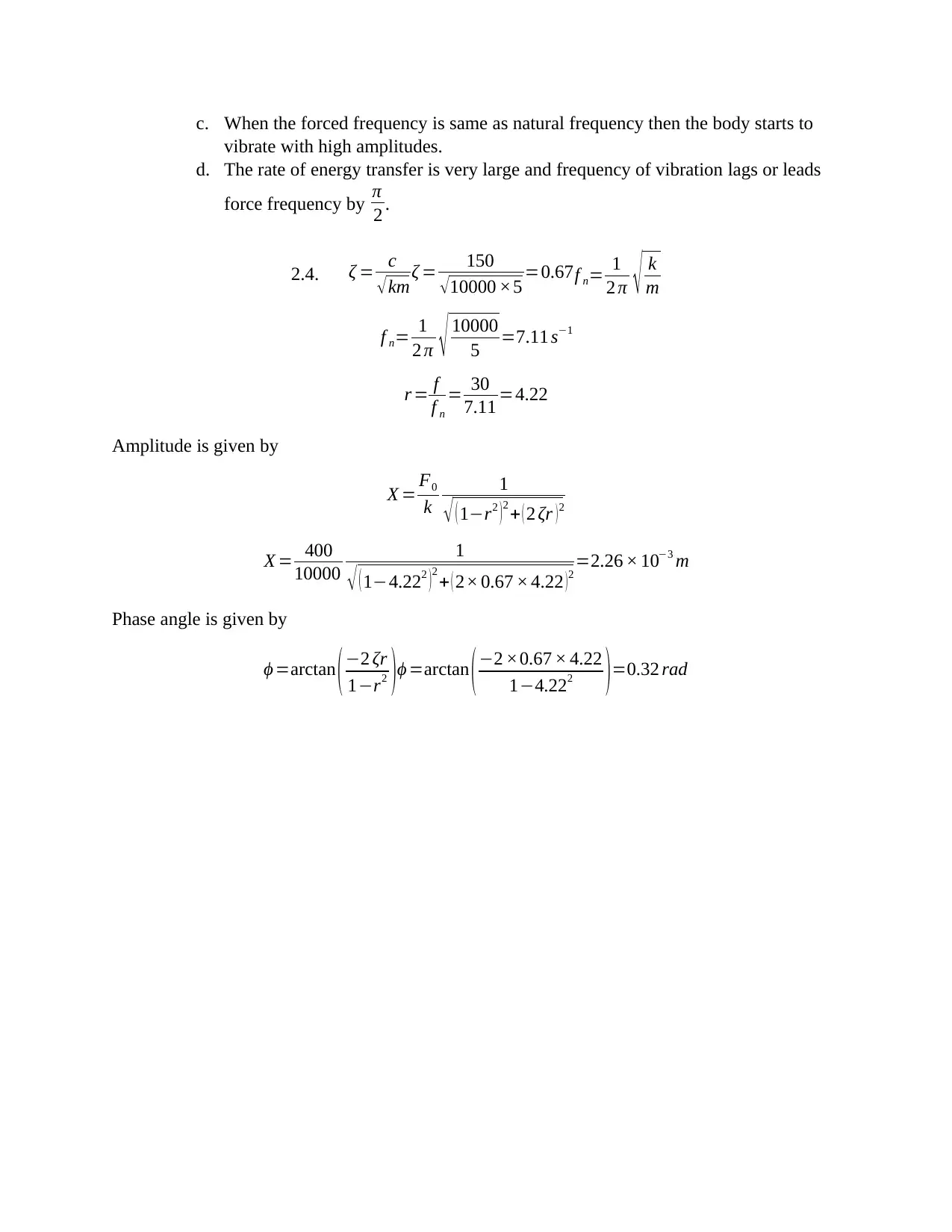
This assignment solution provides detailed calculations and explanations for mechanical vibration problems. It covers topics such as angular velocity, time period, maximum acceleration and velocity, velocity and acceleration at specific distances from the center, and kinetic energy calculations. Furthermore, it delves into the concept of vibration as a periodic conversion of elastic and kinetic energy, explaining undamped natural frequency and deriving the equation for a damped forced system. The solution explores different damping scenarios (no damping, underdamped, critically damped, and overdamped) and their impact on system behavior. Finally, it addresses forced vibration, discussing the system's response to external forces, resonance conditions, and the relationship between forced frequency and natural frequency. The amplitude and phase angle for a specific forced vibration problem are also calculated. Desklib offers a wealth of similar solved assignments and past papers to aid students in their studies.
1 out of 3








![[object Object]](/_next/static/media/star-bottom.7253800d.svg)