Business Decision Analysis Assignment
VerifiedAdded on 2023/01/20
|15
|1791
|75
AI Summary
This document is an individual problem assignment for the course BUSI 2013 Business Decision Analysis at Yorkville University. It includes a question about forecasting and regression analysis, with step-by-step solutions and calculations. The assignment is due on April 19th, 2019.
Contribute Materials
Your contribution can guide someone’s learning journey. Share your
documents today.

Kwaku Tweneboa Kodua
Yorkville University
BUSI 2013 Business Decision Analysis
Individual Problem 2
Professor: Aniket Mahanti
April 19th, 2019
Yorkville University
BUSI 2013 Business Decision Analysis
Individual Problem 2
Professor: Aniket Mahanti
April 19th, 2019
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
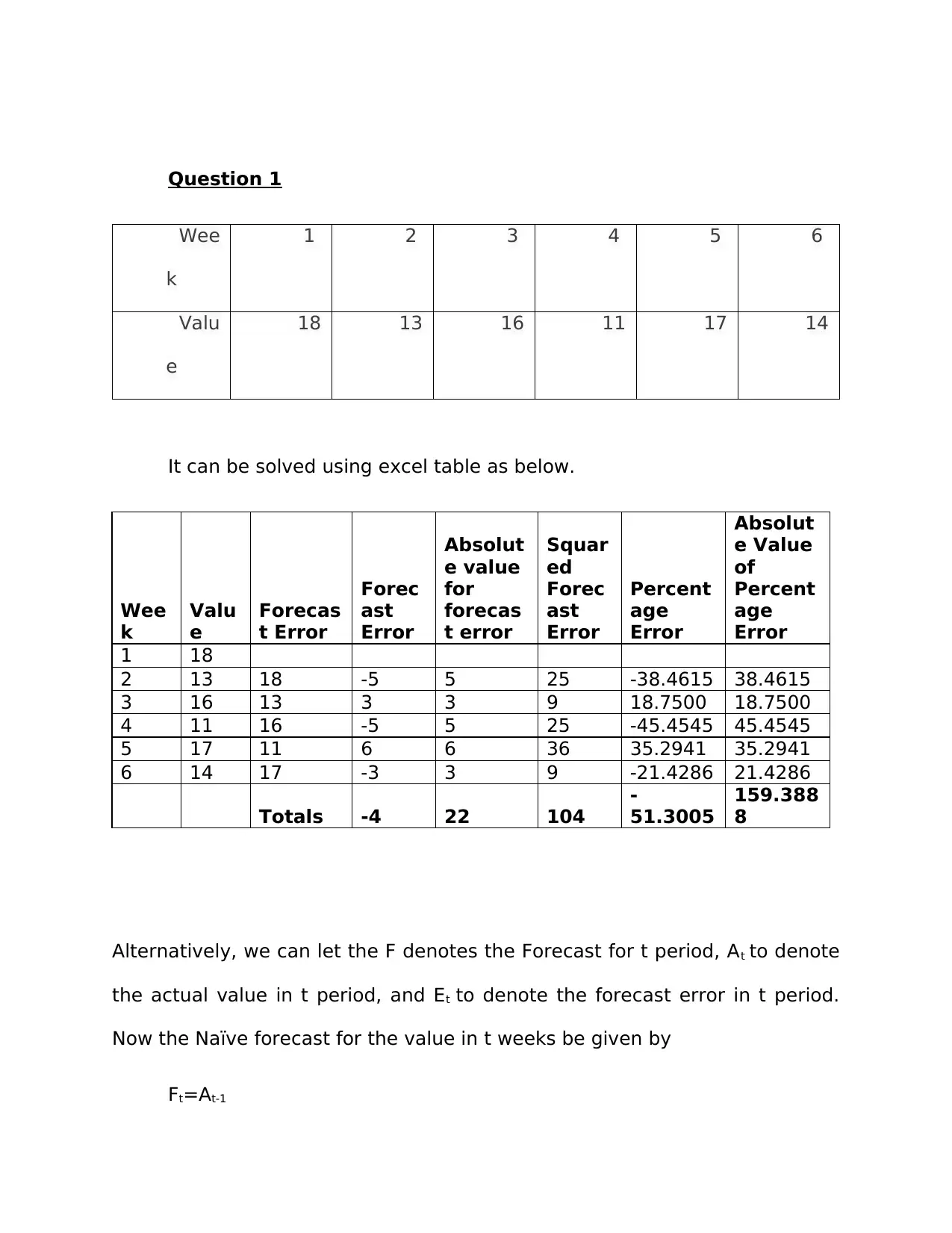
Question 1
Wee
k
1 2 3 4 5 6
Valu
e
18 13 16 11 17 14
It can be solved using excel table as below.
Wee
k
Valu
e
Forecas
t Error
Forec
ast
Error
Absolut
e value
for
forecas
t error
Squar
ed
Forec
ast
Error
Percent
age
Error
Absolut
e Value
of
Percent
age
Error
1 18
2 13 18 -5 5 25 -38.4615 38.4615
3 16 13 3 3 9 18.7500 18.7500
4 11 16 -5 5 25 -45.4545 45.4545
5 17 11 6 6 36 35.2941 35.2941
6 14 17 -3 3 9 -21.4286 21.4286
Totals -4 22 104
-
51.3005
159.388
8
Alternatively, we can let the F denotes the Forecast for t period, At to denote
the actual value in t period, and Et to denote the forecast error in t period.
Now the Naïve forecast for the value in t weeks be given by
Ft=At-1
Wee
k
1 2 3 4 5 6
Valu
e
18 13 16 11 17 14
It can be solved using excel table as below.
Wee
k
Valu
e
Forecas
t Error
Forec
ast
Error
Absolut
e value
for
forecas
t error
Squar
ed
Forec
ast
Error
Percent
age
Error
Absolut
e Value
of
Percent
age
Error
1 18
2 13 18 -5 5 25 -38.4615 38.4615
3 16 13 3 3 9 18.7500 18.7500
4 11 16 -5 5 25 -45.4545 45.4545
5 17 11 6 6 36 35.2941 35.2941
6 14 17 -3 3 9 -21.4286 21.4286
Totals -4 22 104
-
51.3005
159.388
8
Alternatively, we can let the F denotes the Forecast for t period, At to denote
the actual value in t period, and Et to denote the forecast error in t period.
Now the Naïve forecast for the value in t weeks be given by
Ft=At-1

F2=A1=18
E2=A2-F1=13-18=-5
F3=A2=13
E3=A3-F2=16-13=3
F4=A3=16
E4=A4-F3=11-16=-5
F5 =A4 =11
E5 =A5-F4=17- 11=6
F6=A5 =17
E6=A6 -F5=14-17=-3
Part a
Mean Absolute Error (MAE) =∑
i=2
6 |Ei|
5 = (5+ 3+5+6+3 )
5 =4.40
Part b
Mean Squared Error (MSE) =∑
i=2
6 ( Ei)2
5 ¿
[52 +32+52+62 +32 ] /5=20.80
Part c
Mean Absolute Percentage Error (MAPE) =100 % /5 ∑
i=2
6
¿ Et
At ∨¿
E2=A2-F1=13-18=-5
F3=A2=13
E3=A3-F2=16-13=3
F4=A3=16
E4=A4-F3=11-16=-5
F5 =A4 =11
E5 =A5-F4=17- 11=6
F6=A5 =17
E6=A6 -F5=14-17=-3
Part a
Mean Absolute Error (MAE) =∑
i=2
6 |Ei|
5 = (5+ 3+5+6+3 )
5 =4.40
Part b
Mean Squared Error (MSE) =∑
i=2
6 ( Ei)2
5 ¿
[52 +32+52+62 +32 ] /5=20.80
Part c
Mean Absolute Percentage Error (MAPE) =100 % /5 ∑
i=2
6
¿ Et
At ∨¿
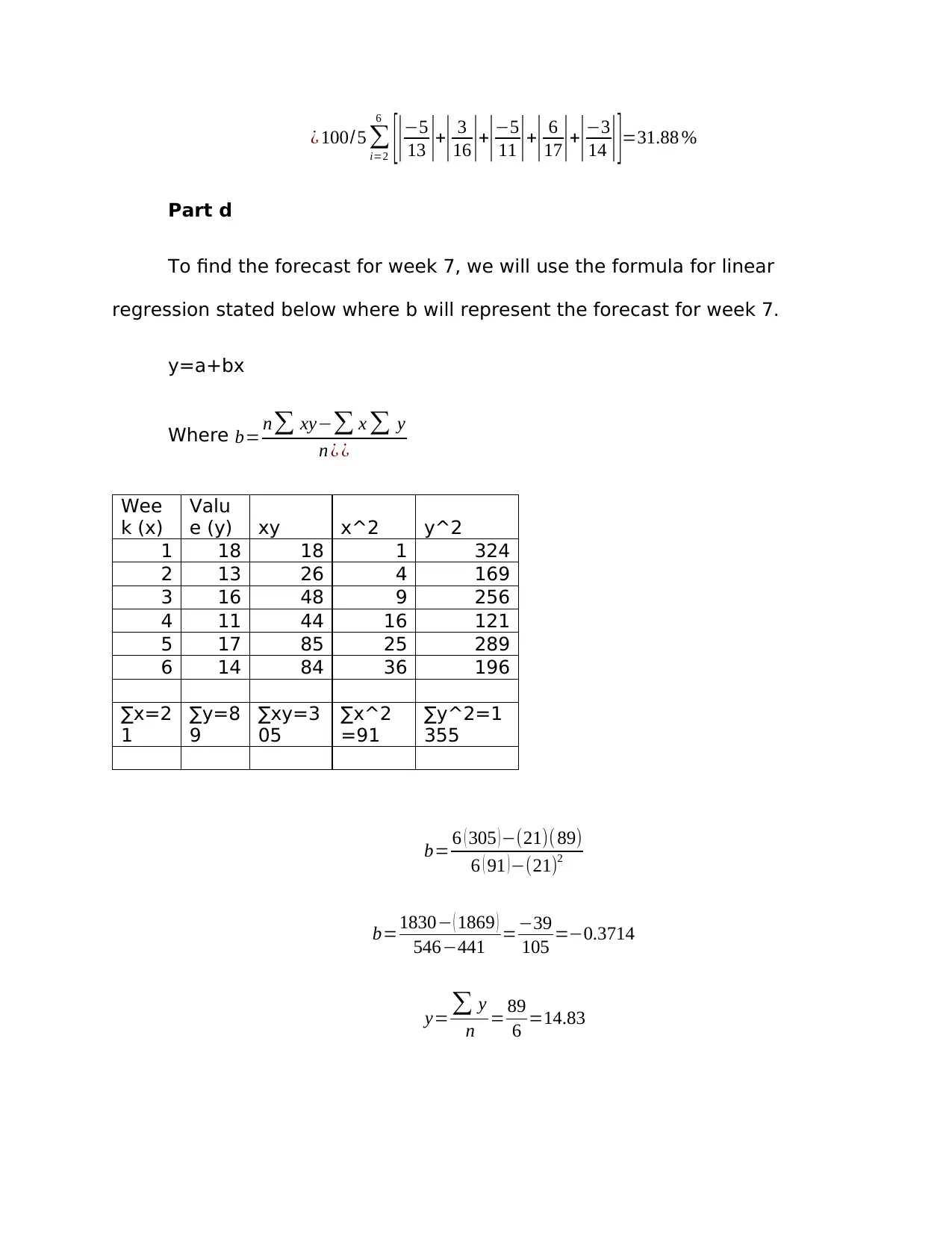
¿ 100/5 ∑
i=2
6
[|−5
13 |+| 3
16 |+|−5
11 |+| 6
17|+|−3
14 |]=31.88 %
Part d
To find the forecast for week 7, we will use the formula for linear
regression stated below where b will represent the forecast for week 7.
y=a+bx
Where b= n∑ xy−∑ x ∑ y
n ¿ ¿
Wee
k (x)
Valu
e (y) xy x^2 y^2
1 18 18 1 324
2 13 26 4 169
3 16 48 9 256
4 11 44 16 121
5 17 85 25 289
6 14 84 36 196
∑x=2
1
∑y=8
9
∑xy=3
05
∑x^2
=91
∑y^2=1
355
b= 6 ( 305 )−(21)( 89)
6 ( 91 )−(21)2
b=1830− ( 1869 )
546−441 =−39
105 =−0.3714
y= ∑ y
n = 89
6 =14.83
i=2
6
[|−5
13 |+| 3
16 |+|−5
11 |+| 6
17|+|−3
14 |]=31.88 %
Part d
To find the forecast for week 7, we will use the formula for linear
regression stated below where b will represent the forecast for week 7.
y=a+bx
Where b= n∑ xy−∑ x ∑ y
n ¿ ¿
Wee
k (x)
Valu
e (y) xy x^2 y^2
1 18 18 1 324
2 13 26 4 169
3 16 48 9 256
4 11 44 16 121
5 17 85 25 289
6 14 84 36 196
∑x=2
1
∑y=8
9
∑xy=3
05
∑x^2
=91
∑y^2=1
355
b= 6 ( 305 )−(21)( 89)
6 ( 91 )−(21)2
b=1830− ( 1869 )
546−441 =−39
105 =−0.3714
y= ∑ y
n = 89
6 =14.83
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
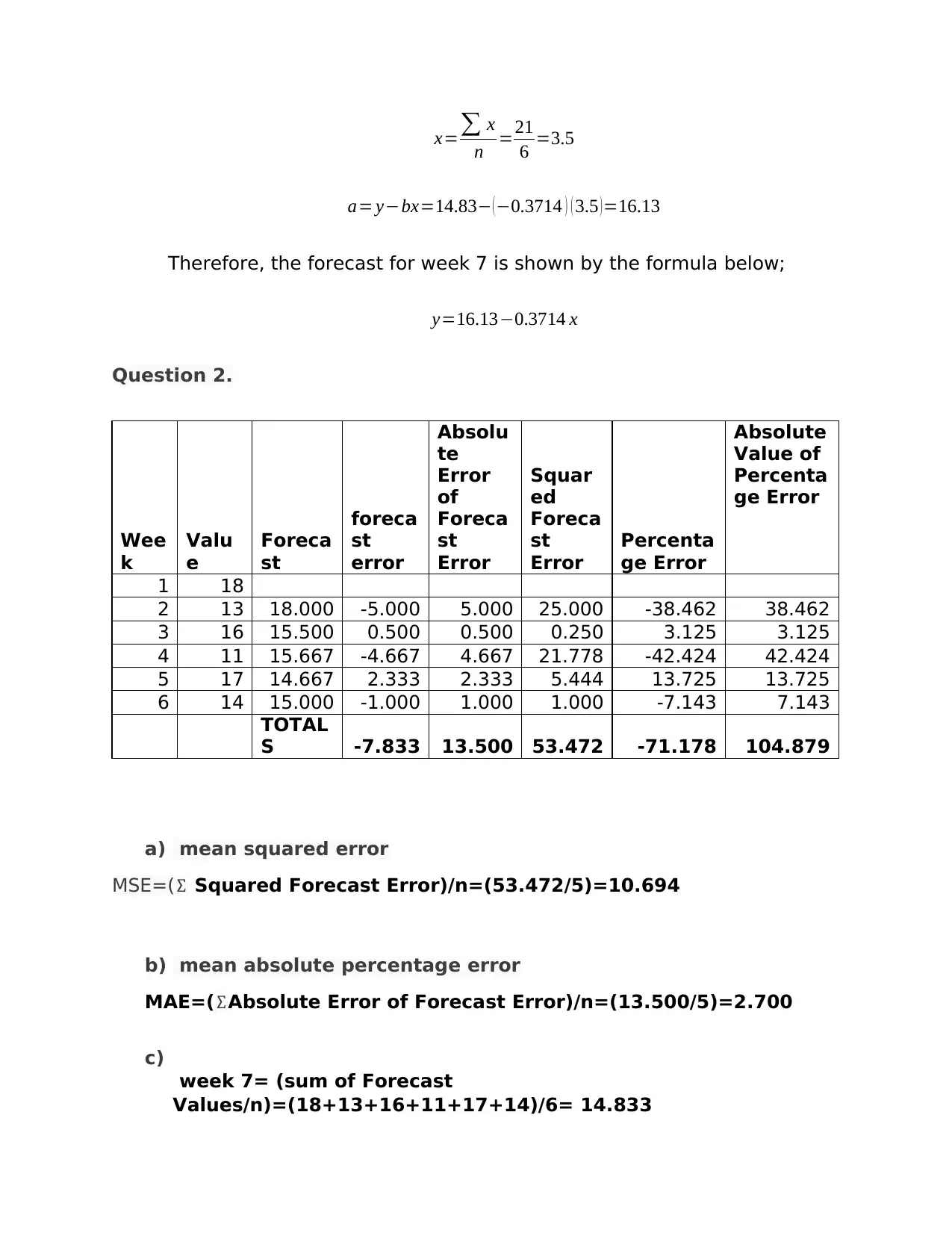
x=∑ x
n =21
6 =3.5
a= y−bx=14.83− (−0.3714 ) ( 3.5 )=16.13
Therefore, the forecast for week 7 is shown by the formula below;
y=16.13−0.3714 x
Question 2.
Wee
k
Valu
e
Foreca
st
foreca
st
error
Absolu
te
Error
of
Foreca
st
Error
Squar
ed
Foreca
st
Error
Percenta
ge Error
Absolute
Value of
Percenta
ge Error
1 18
2 13 18.000 -5.000 5.000 25.000 -38.462 38.462
3 16 15.500 0.500 0.500 0.250 3.125 3.125
4 11 15.667 -4.667 4.667 21.778 -42.424 42.424
5 17 14.667 2.333 2.333 5.444 13.725 13.725
6 14 15.000 -1.000 1.000 1.000 -7.143 7.143
TOTAL
S -7.833 13.500 53.472 -71.178 104.879
a) mean squared error
MSE=(∑ Squared Forecast Error)/n=(53.472/5)=10.694
b) mean absolute percentage error
MAE=(∑Absolute Error of Forecast Error)/n=(13.500/5)=2.700
c)
week 7= (sum of Forecast
Values/n)=(18+13+16+11+17+14)/6= 14.833
n =21
6 =3.5
a= y−bx=14.83− (−0.3714 ) ( 3.5 )=16.13
Therefore, the forecast for week 7 is shown by the formula below;
y=16.13−0.3714 x
Question 2.
Wee
k
Valu
e
Foreca
st
foreca
st
error
Absolu
te
Error
of
Foreca
st
Error
Squar
ed
Foreca
st
Error
Percenta
ge Error
Absolute
Value of
Percenta
ge Error
1 18
2 13 18.000 -5.000 5.000 25.000 -38.462 38.462
3 16 15.500 0.500 0.500 0.250 3.125 3.125
4 11 15.667 -4.667 4.667 21.778 -42.424 42.424
5 17 14.667 2.333 2.333 5.444 13.725 13.725
6 14 15.000 -1.000 1.000 1.000 -7.143 7.143
TOTAL
S -7.833 13.500 53.472 -71.178 104.879
a) mean squared error
MSE=(∑ Squared Forecast Error)/n=(53.472/5)=10.694
b) mean absolute percentage error
MAE=(∑Absolute Error of Forecast Error)/n=(13.500/5)=2.700
c)
week 7= (sum of Forecast
Values/n)=(18+13+16+11+17+14)/6= 14.833
Question3
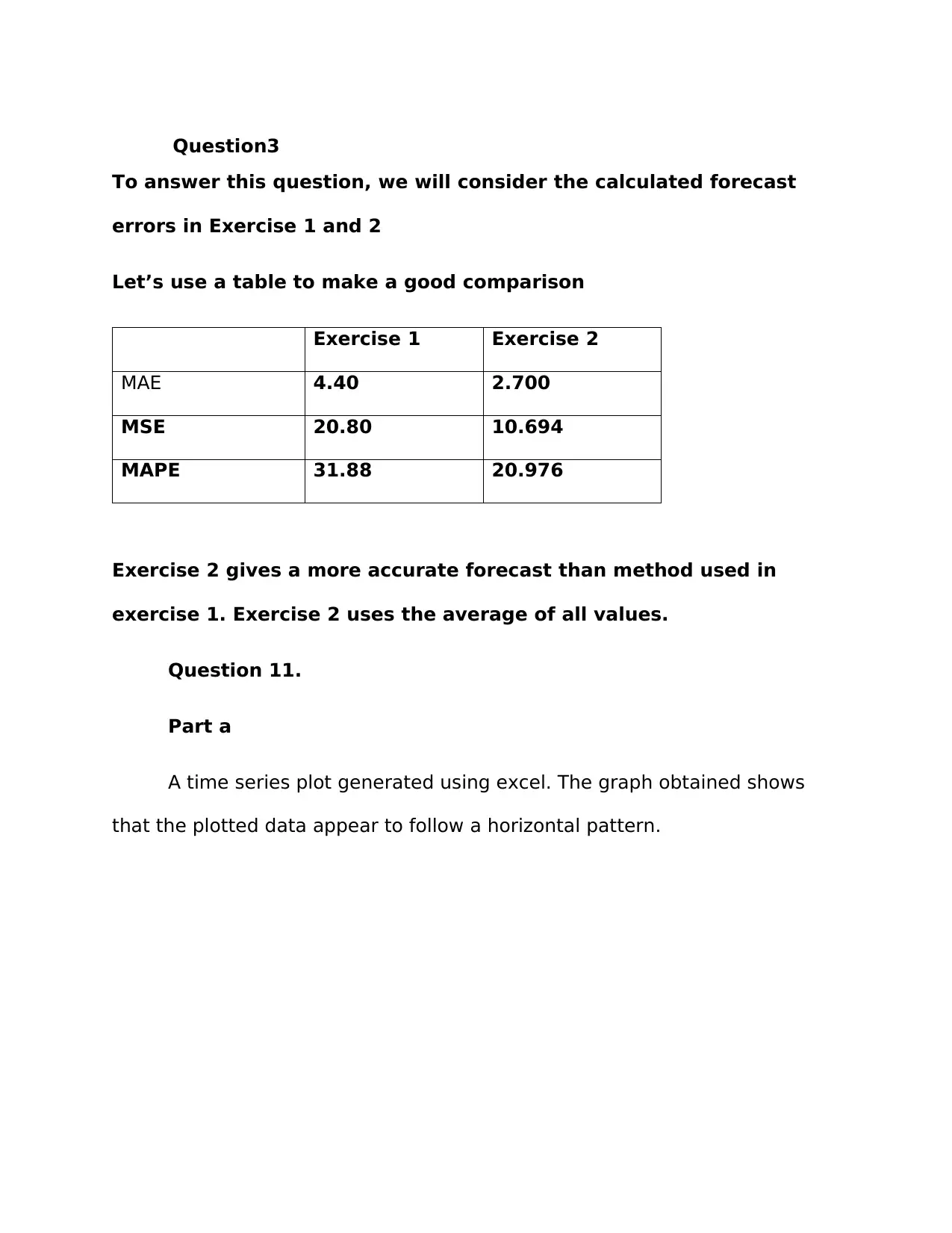
To answer this question, we will consider the calculated forecast
errors in Exercise 1 and 2
Let’s use a table to make a good comparison
Exercise 1 Exercise 2
MAE 4.40 2.700
MSE 20.80 10.694
MAPE 31.88 20.976
Exercise 2 gives a more accurate forecast than method used in
exercise 1. Exercise 2 uses the average of all values.
Question 11.
Part a
A time series plot generated using excel. The graph obtained shows
that the plotted data appear to follow a horizontal pattern.
To answer this question, we will consider the calculated forecast
errors in Exercise 1 and 2
Let’s use a table to make a good comparison
Exercise 1 Exercise 2
MAE 4.40 2.700
MSE 20.80 10.694
MAPE 31.88 20.976
Exercise 2 gives a more accurate forecast than method used in
exercise 1. Exercise 2 uses the average of all values.
Question 11.
Part a
A time series plot generated using excel. The graph obtained shows
that the plotted data appear to follow a horizontal pattern.
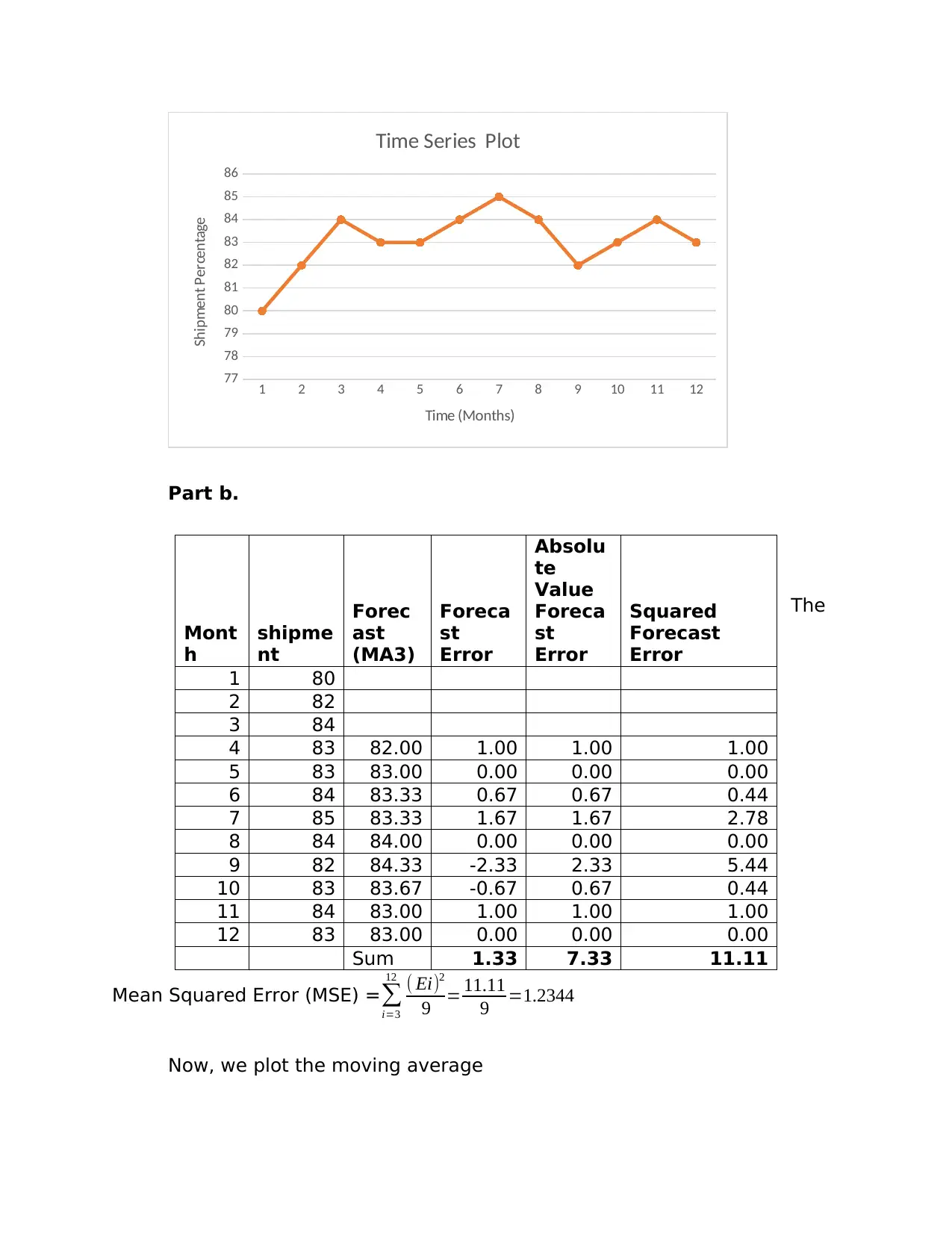
1 2 3 4 5 6 7 8 9 10 11 12
77
78
79
80
81
82
83
84
85
86
Time Series Plot
Time (Months)
Shipment Percentage
Part b.
The
Mean Squared Error (MSE) =∑
i=3
12 ( Ei)2
9 = 11.11
9 =1.2344
Now, we plot the moving average
Mont
h
shipme
nt
Forec
ast
(MA3)
Foreca
st
Error
Absolu
te
Value
Foreca
st
Error
Squared
Forecast
Error
1 80
2 82
3 84
4 83 82.00 1.00 1.00 1.00
5 83 83.00 0.00 0.00 0.00
6 84 83.33 0.67 0.67 0.44
7 85 83.33 1.67 1.67 2.78
8 84 84.00 0.00 0.00 0.00
9 82 84.33 -2.33 2.33 5.44
10 83 83.67 -0.67 0.67 0.44
11 84 83.00 1.00 1.00 1.00
12 83 83.00 0.00 0.00 0.00
Sum 1.33 7.33 11.11
77
78
79
80
81
82
83
84
85
86
Time Series Plot
Time (Months)
Shipment Percentage
Part b.
The
Mean Squared Error (MSE) =∑
i=3
12 ( Ei)2
9 = 11.11
9 =1.2344
Now, we plot the moving average
Mont
h
shipme
nt
Forec
ast
(MA3)
Foreca
st
Error
Absolu
te
Value
Foreca
st
Error
Squared
Forecast
Error
1 80
2 82
3 84
4 83 82.00 1.00 1.00 1.00
5 83 83.00 0.00 0.00 0.00
6 84 83.33 0.67 0.67 0.44
7 85 83.33 1.67 1.67 2.78
8 84 84.00 0.00 0.00 0.00
9 82 84.33 -2.33 2.33 5.44
10 83 83.67 -0.67 0.67 0.44
11 84 83.00 1.00 1.00 1.00
12 83 83.00 0.00 0.00 0.00
Sum 1.33 7.33 11.11
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser
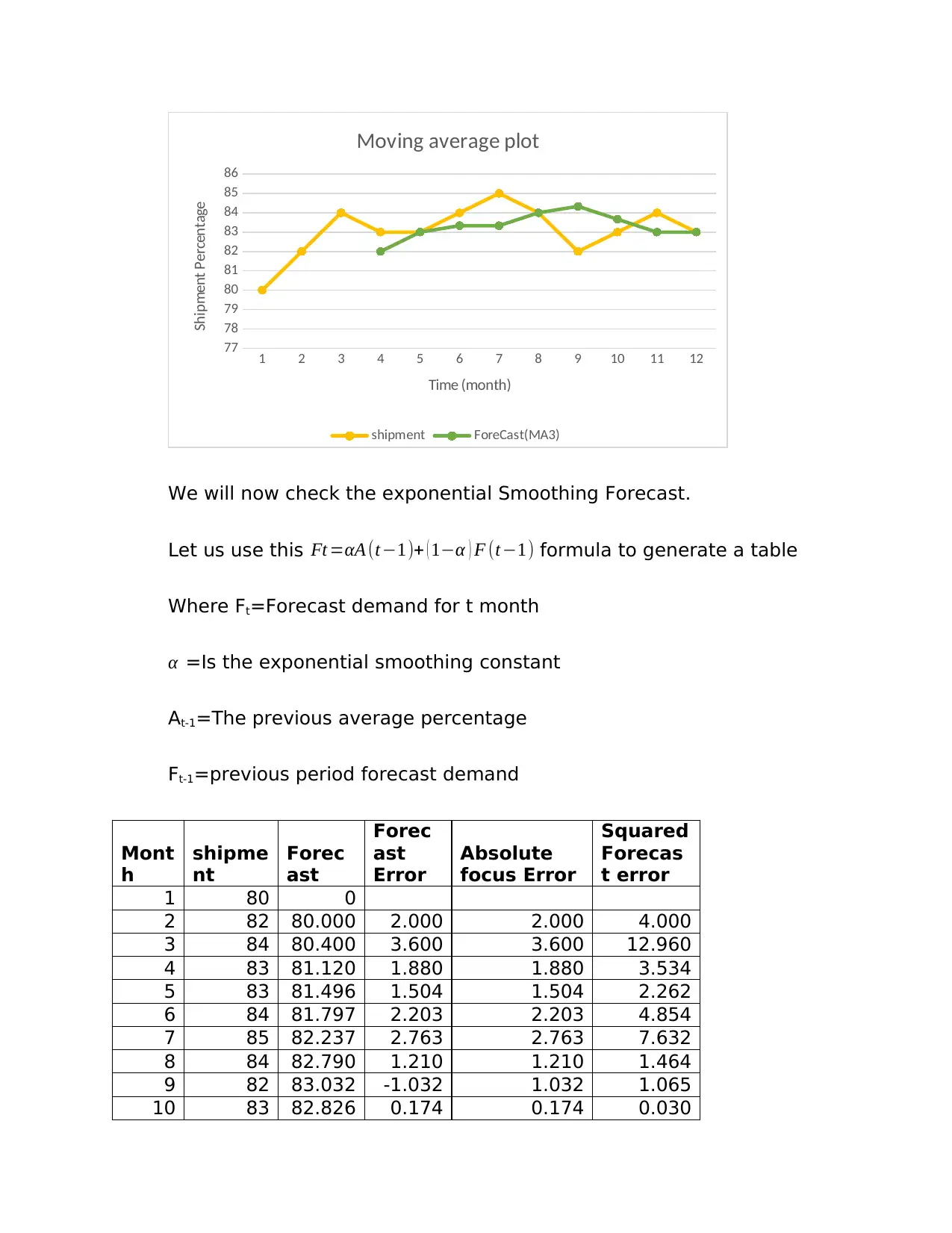
1 2 3 4 5 6 7 8 9 10 11 12
77
78
79
80
81
82
83
84
85
86
Moving average plot
shipment ForeCast(MA3)
Time (month)
Shipment Percentage
We will now check the exponential Smoothing Forecast.
Let us use this Ft =αA (t−1)+ ( 1−α ) F (t−1) formula to generate a table
Where Ft=Forecast demand for t month
α =Is the exponential smoothing constant
At-1=The previous average percentage
Ft-1=previous period forecast demand
Mont
h
shipme
nt
Forec
ast
Forec
ast
Error
Absolute
focus Error
Squared
Forecas
t error
1 80 0
2 82 80.000 2.000 2.000 4.000
3 84 80.400 3.600 3.600 12.960
4 83 81.120 1.880 1.880 3.534
5 83 81.496 1.504 1.504 2.262
6 84 81.797 2.203 2.203 4.854
7 85 82.237 2.763 2.763 7.632
8 84 82.790 1.210 1.210 1.464
9 82 83.032 -1.032 1.032 1.065
10 83 82.826 0.174 0.174 0.030
77
78
79
80
81
82
83
84
85
86
Moving average plot
shipment ForeCast(MA3)
Time (month)
Shipment Percentage
We will now check the exponential Smoothing Forecast.
Let us use this Ft =αA (t−1)+ ( 1−α ) F (t−1) formula to generate a table
Where Ft=Forecast demand for t month
α =Is the exponential smoothing constant
At-1=The previous average percentage
Ft-1=previous period forecast demand
Mont
h
shipme
nt
Forec
ast
Forec
ast
Error
Absolute
focus Error
Squared
Forecas
t error
1 80 0
2 82 80.000 2.000 2.000 4.000
3 84 80.400 3.600 3.600 12.960
4 83 81.120 1.880 1.880 3.534
5 83 81.496 1.504 1.504 2.262
6 84 81.797 2.203 2.203 4.854
7 85 82.237 2.763 2.763 7.632
8 84 82.790 1.210 1.210 1.464
9 82 83.032 -1.032 1.032 1.065
10 83 82.826 0.174 0.174 0.030
11 84 82.860 1.140 1.140 1.299
12 83 83.088 -0.088 0.088 0.008
Sum
15.35
3 17.594 39.108
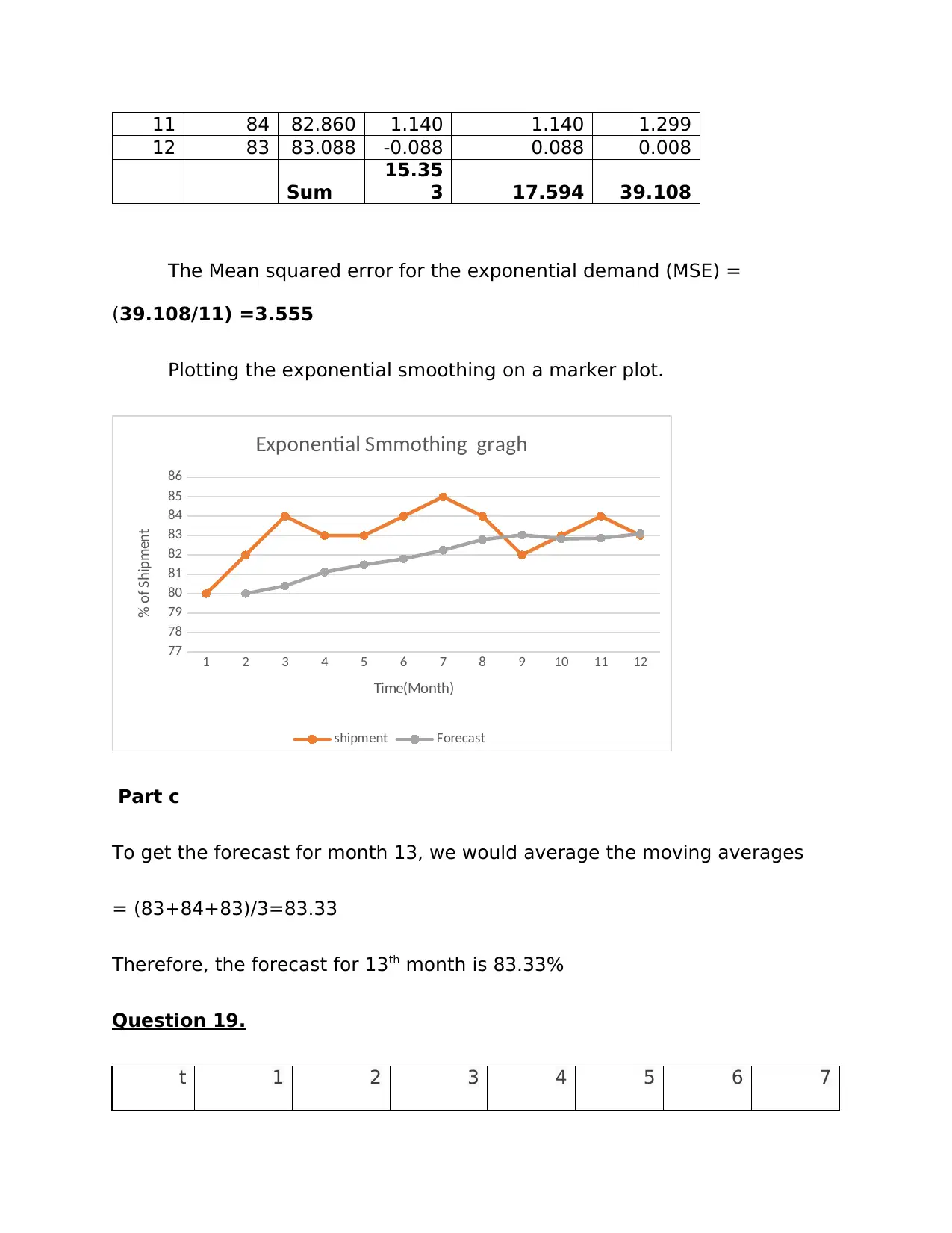
The Mean squared error for the exponential demand (MSE) =
(39.108/11) =3.555
Plotting the exponential smoothing on a marker plot.
1 2 3 4 5 6 7 8 9 10 11 12
77
78
79
80
81
82
83
84
85
86
Exponential Smmothing gragh
shipment Forecast
Time(Month)
% of Shipment
Part c
To get the forecast for month 13, we would average the moving averages
= (83+84+83)/3=83.33
Therefore, the forecast for 13th month is 83.33%
Question 19.
t 1 2 3 4 5 6 7
12 83 83.088 -0.088 0.088 0.008
Sum
15.35
3 17.594 39.108
The Mean squared error for the exponential demand (MSE) =
(39.108/11) =3.555
Plotting the exponential smoothing on a marker plot.
1 2 3 4 5 6 7 8 9 10 11 12
77
78
79
80
81
82
83
84
85
86
Exponential Smmothing gragh
shipment Forecast
Time(Month)
% of Shipment
Part c
To get the forecast for month 13, we would average the moving averages
= (83+84+83)/3=83.33
Therefore, the forecast for 13th month is 83.33%
Question 19.
t 1 2 3 4 5 6 7
Y
t
12
0
11
0
10
0
9
6
9
4
9
2
8
8
Part a
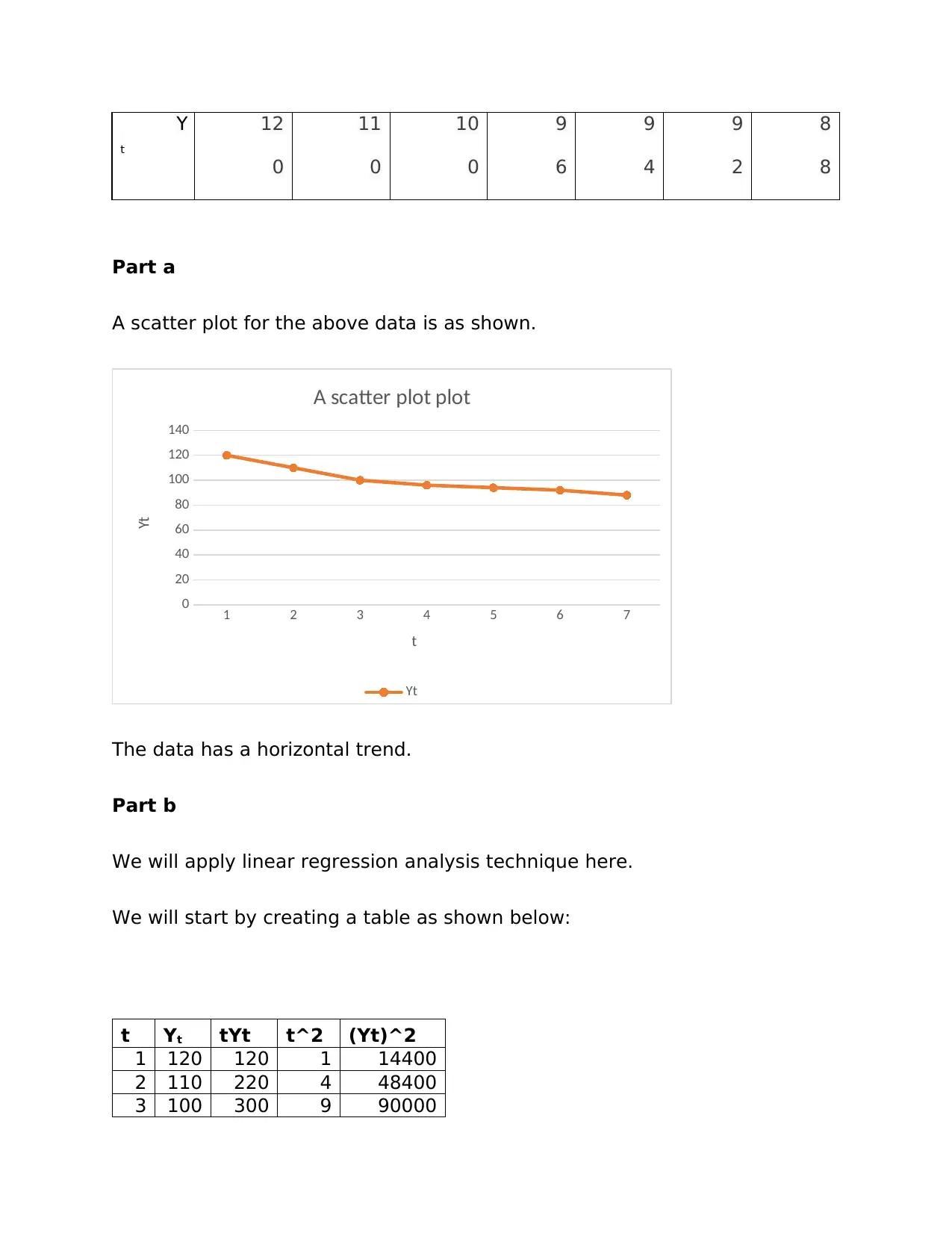
A scatter plot for the above data is as shown.
1 2 3 4 5 6 7
0
20
40
60
80
100
120
140
A scatter plot plot
Yt
t
Yt
The data has a horizontal trend.
Part b
We will apply linear regression analysis technique here.
We will start by creating a table as shown below:
t Yt tYt t^2 (Yt)^2
1 120 120 1 14400
2 110 220 4 48400
3 100 300 9 90000
t
12
0
11
0
10
0
9
6
9
4
9
2
8
8
Part a
A scatter plot for the above data is as shown.
1 2 3 4 5 6 7
0
20
40
60
80
100
120
140
A scatter plot plot
Yt
t
Yt
The data has a horizontal trend.
Part b
We will apply linear regression analysis technique here.
We will start by creating a table as shown below:
t Yt tYt t^2 (Yt)^2
1 120 120 1 14400
2 110 220 4 48400
3 100 300 9 90000
Secure Best Marks with AI Grader
Need help grading? Try our AI Grader for instant feedback on your assignments.
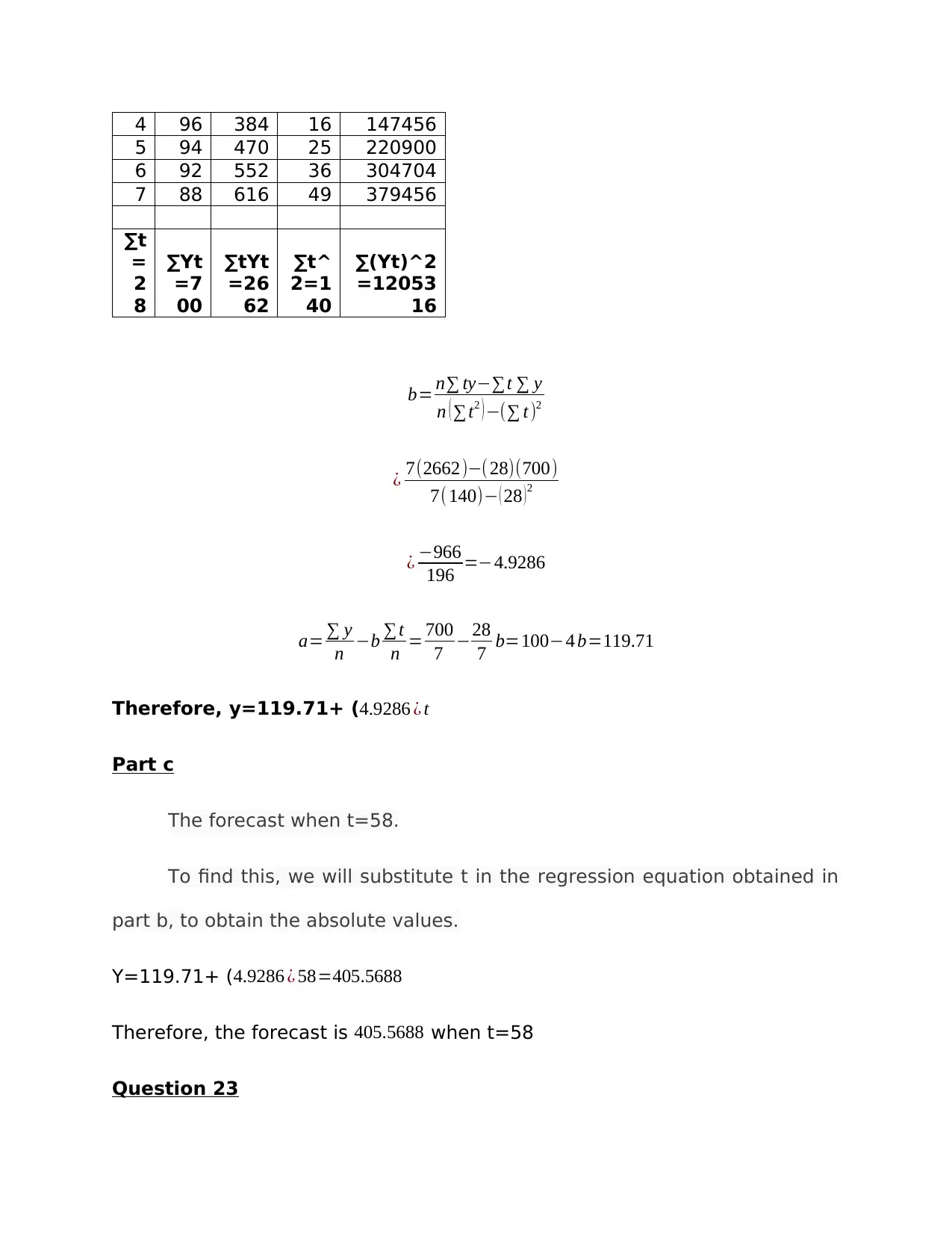
4 96 384 16 147456
5 94 470 25 220900
6 92 552 36 304704
7 88 616 49 379456
∑t
=
2
8
∑Yt
=7
00
∑tYt
=26
62
∑t^
2=1
40
∑(Yt)^2
=12053
16
b= n∑ ty−∑t ∑ y
n ( ∑ t2 ) −(∑ t)2
¿ 7(2662)−( 28)(700)
7(140)− ( 28 )2
¿ −966
196 =−4.9286
a= ∑ y
n −b ∑ t
n = 700
7 −28
7 b=100−4 b=119.71
Therefore, y=119.71+ ( 4.9286 ¿ t
Part c
The forecast when t=58.
To find this, we will substitute t in the regression equation obtained in
part b, to obtain the absolute values.
Y=119.71+ ( 4.9286 ¿ 58=405.5688
Therefore, the forecast is 405.5688 when t=58
Question 23
5 94 470 25 220900
6 92 552 36 304704
7 88 616 49 379456
∑t
=
2
8
∑Yt
=7
00
∑tYt
=26
62
∑t^
2=1
40
∑(Yt)^2
=12053
16
b= n∑ ty−∑t ∑ y
n ( ∑ t2 ) −(∑ t)2
¿ 7(2662)−( 28)(700)
7(140)− ( 28 )2
¿ −966
196 =−4.9286
a= ∑ y
n −b ∑ t
n = 700
7 −28
7 b=100−4 b=119.71
Therefore, y=119.71+ ( 4.9286 ¿ t
Part c
The forecast when t=58.
To find this, we will substitute t in the regression equation obtained in
part b, to obtain the absolute values.
Y=119.71+ ( 4.9286 ¿ 58=405.5688
Therefore, the forecast is 405.5688 when t=58
Question 23
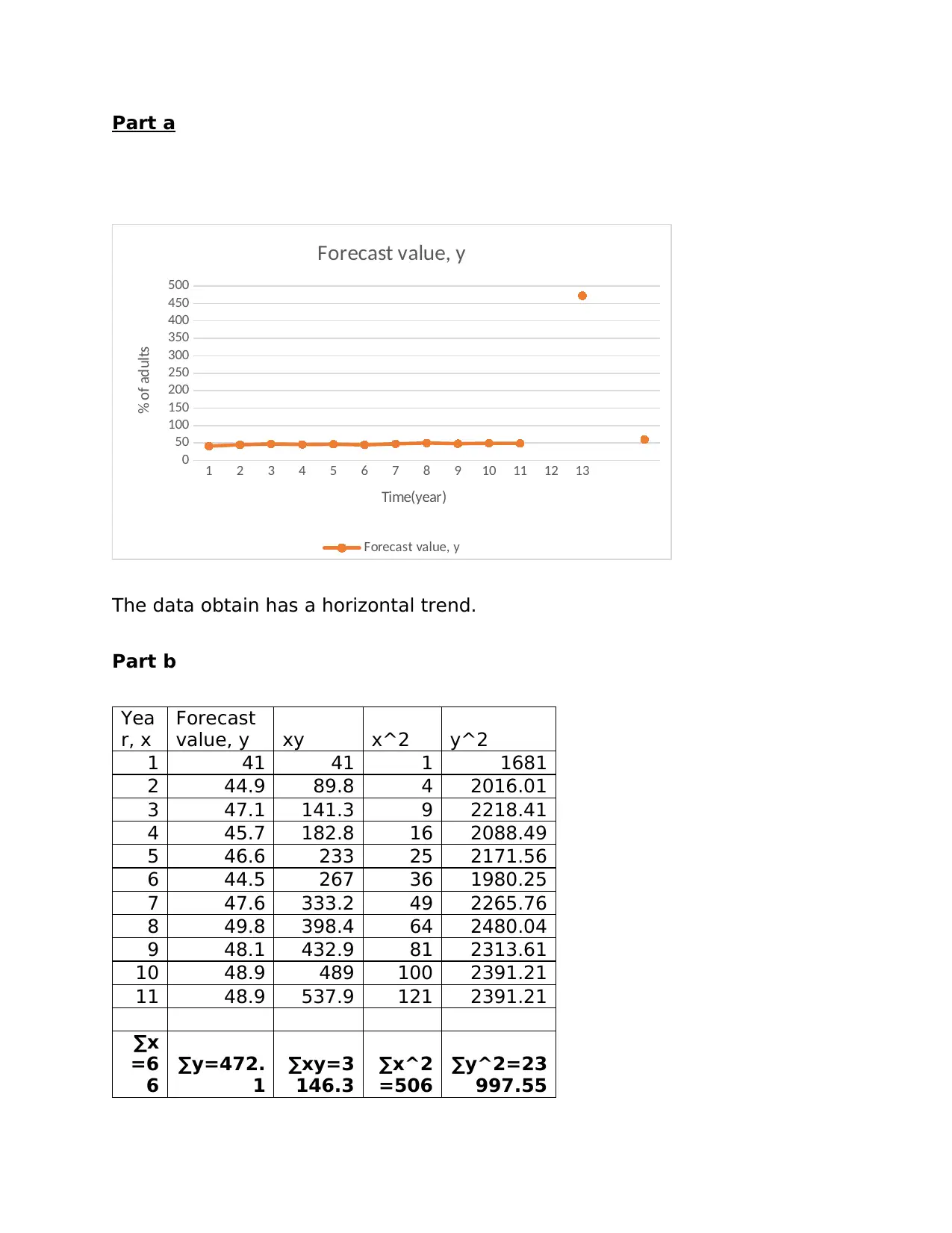
Part a
1 2 3 4 5 6 7 8 9 10 11 12 13
0
50
100
150
200
250
300
350
400
450
500
Forecast value, y
Forecast value, y
Time(year)
% of adults
The data obtain has a horizontal trend.
Part b
Yea
r, x
Forecast
value, y xy x^2 y^2
1 41 41 1 1681
2 44.9 89.8 4 2016.01
3 47.1 141.3 9 2218.41
4 45.7 182.8 16 2088.49
5 46.6 233 25 2171.56
6 44.5 267 36 1980.25
7 47.6 333.2 49 2265.76
8 49.8 398.4 64 2480.04
9 48.1 432.9 81 2313.61
10 48.9 489 100 2391.21
11 48.9 537.9 121 2391.21
∑x
=6
6
∑y=472.
1
∑xy=3
146.3
∑x^2
=506
∑y^2=23
997.55
1 2 3 4 5 6 7 8 9 10 11 12 13
0
50
100
150
200
250
300
350
400
450
500
Forecast value, y
Forecast value, y
Time(year)
% of adults
The data obtain has a horizontal trend.
Part b
Yea
r, x
Forecast
value, y xy x^2 y^2
1 41 41 1 1681
2 44.9 89.8 4 2016.01
3 47.1 141.3 9 2218.41
4 45.7 182.8 16 2088.49
5 46.6 233 25 2171.56
6 44.5 267 36 1980.25
7 47.6 333.2 49 2265.76
8 49.8 398.4 64 2480.04
9 48.1 432.9 81 2313.61
10 48.9 489 100 2391.21
11 48.9 537.9 121 2391.21
∑x
=6
6
∑y=472.
1
∑xy=3
146.3
∑x^2
=506
∑y^2=23
997.55
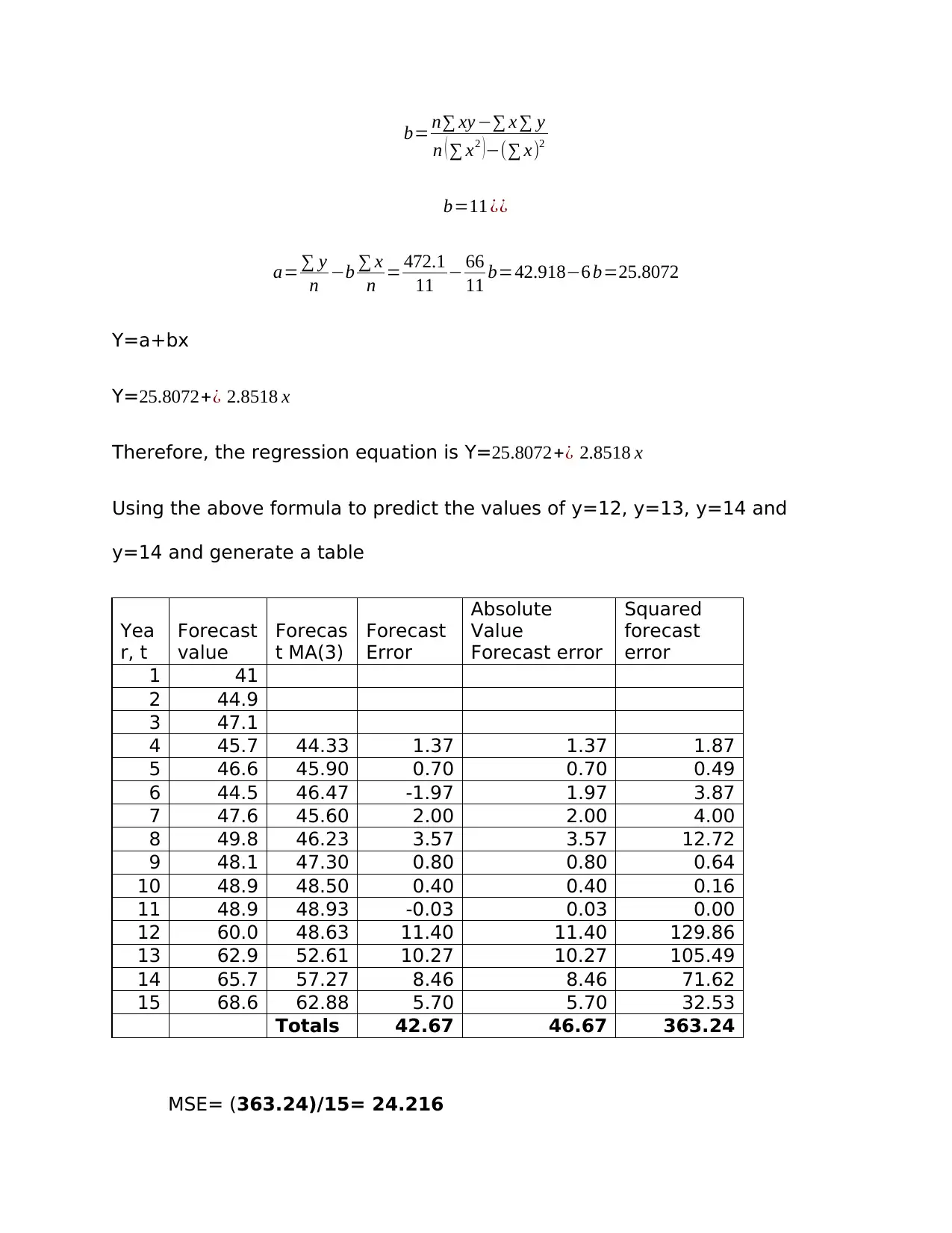
b= n∑ xy −∑ x ∑ y
n ( ∑ x2 )−(∑ x)2
b=11 ¿¿
a= ∑ y
n −b ∑ x
n = 472.1
11 − 66
11 b=42.918−6 b=25.8072
Y=a+bx
Y=25.8072+¿ 2.8518 x
Therefore, the regression equation is Y=25.8072+¿ 2.8518 x
Using the above formula to predict the values of y=12, y=13, y=14 and
y=14 and generate a table
Yea
r, t
Forecast
value
Forecas
t MA(3)
Forecast
Error
Absolute
Value
Forecast error
Squared
forecast
error
1 41
2 44.9
3 47.1
4 45.7 44.33 1.37 1.37 1.87
5 46.6 45.90 0.70 0.70 0.49
6 44.5 46.47 -1.97 1.97 3.87
7 47.6 45.60 2.00 2.00 4.00
8 49.8 46.23 3.57 3.57 12.72
9 48.1 47.30 0.80 0.80 0.64
10 48.9 48.50 0.40 0.40 0.16
11 48.9 48.93 -0.03 0.03 0.00
12 60.0 48.63 11.40 11.40 129.86
13 62.9 52.61 10.27 10.27 105.49
14 65.7 57.27 8.46 8.46 71.62
15 68.6 62.88 5.70 5.70 32.53
Totals 42.67 46.67 363.24
MSE= (363.24)/15= 24.216
n ( ∑ x2 )−(∑ x)2
b=11 ¿¿
a= ∑ y
n −b ∑ x
n = 472.1
11 − 66
11 b=42.918−6 b=25.8072
Y=a+bx
Y=25.8072+¿ 2.8518 x
Therefore, the regression equation is Y=25.8072+¿ 2.8518 x
Using the above formula to predict the values of y=12, y=13, y=14 and
y=14 and generate a table
Yea
r, t
Forecast
value
Forecas
t MA(3)
Forecast
Error
Absolute
Value
Forecast error
Squared
forecast
error
1 41
2 44.9
3 47.1
4 45.7 44.33 1.37 1.37 1.87
5 46.6 45.90 0.70 0.70 0.49
6 44.5 46.47 -1.97 1.97 3.87
7 47.6 45.60 2.00 2.00 4.00
8 49.8 46.23 3.57 3.57 12.72
9 48.1 47.30 0.80 0.80 0.64
10 48.9 48.50 0.40 0.40 0.16
11 48.9 48.93 -0.03 0.03 0.00
12 60.0 48.63 11.40 11.40 129.86
13 62.9 52.61 10.27 10.27 105.49
14 65.7 57.27 8.46 8.46 71.62
15 68.6 62.88 5.70 5.70 32.53
Totals 42.67 46.67 363.24
MSE= (363.24)/15= 24.216
Paraphrase This Document
Need a fresh take? Get an instant paraphrase of this document with our AI Paraphraser

Part c
Given x=16
When forecasting for this year, we will use the regression formula
obtained in b and substitute the value of x=16.
Y=25.8072+¿ 2.8518 ( 16 ) =¿71.436
Therefore, the percentage of adults next year when x=16 is 71.436%
Part d
Yes, the trend of equation in part b can equally predict the percentage of
adults participating in a 30-minute exercise.
Given x=16
When forecasting for this year, we will use the regression formula
obtained in b and substitute the value of x=16.
Y=25.8072+¿ 2.8518 ( 16 ) =¿71.436
Therefore, the percentage of adults next year when x=16 is 71.436%
Part d
Yes, the trend of equation in part b can equally predict the percentage of
adults participating in a 30-minute exercise.

Reference
Academy, J. (2008). Retrieved from https://www.youtube.com/user/rdjalayer:
https://www.youtube.com/watch?v=gHdYEZA50KE&t=29s
Davis, B. &. (1991). Times Series Thoery and Methods. In Times Series
Thoery and Methods. New York: Springer-Verlag.
Academy, J. (2008). Retrieved from https://www.youtube.com/user/rdjalayer:
https://www.youtube.com/watch?v=gHdYEZA50KE&t=29s
Davis, B. &. (1991). Times Series Thoery and Methods. In Times Series
Thoery and Methods. New York: Springer-Verlag.
1 out of 15
Your All-in-One AI-Powered Toolkit for Academic Success.
+13062052269
info@desklib.com
Available 24*7 on WhatsApp / Email
![[object Object]](/_next/static/media/star-bottom.7253800d.svg)
Unlock your academic potential
© 2024 | Zucol Services PVT LTD | All rights reserved.





