Linear Algebra Assignment: Vectors, Dot Products and Geometry

This document presents a comprehensive solution to a linear algebra assignment, focusing on vector operations, dot products, and geometric vector applications. The assignment includes problems on vector addition, subtraction, and scalar multiplication, as well as calculating vector magnitudes and unit vectors. It explores the concept of dot products, determining angles between vectors, and solving equations involving vector components. The solutions demonstrate how to express vectors in terms of other vectors, find distances between points, determine collinearity, and analyze geometric shapes formed by vectors. Furthermore, the document includes detailed calculations, step-by-step explanations, and clear diagrams to illustrate the concepts and solutions effectively.

Running head: LINEAR ALGEBRA 1
Linear Algebra
Name
Institution

LINEAR ALGEBRA 2
Linear Algebra 1
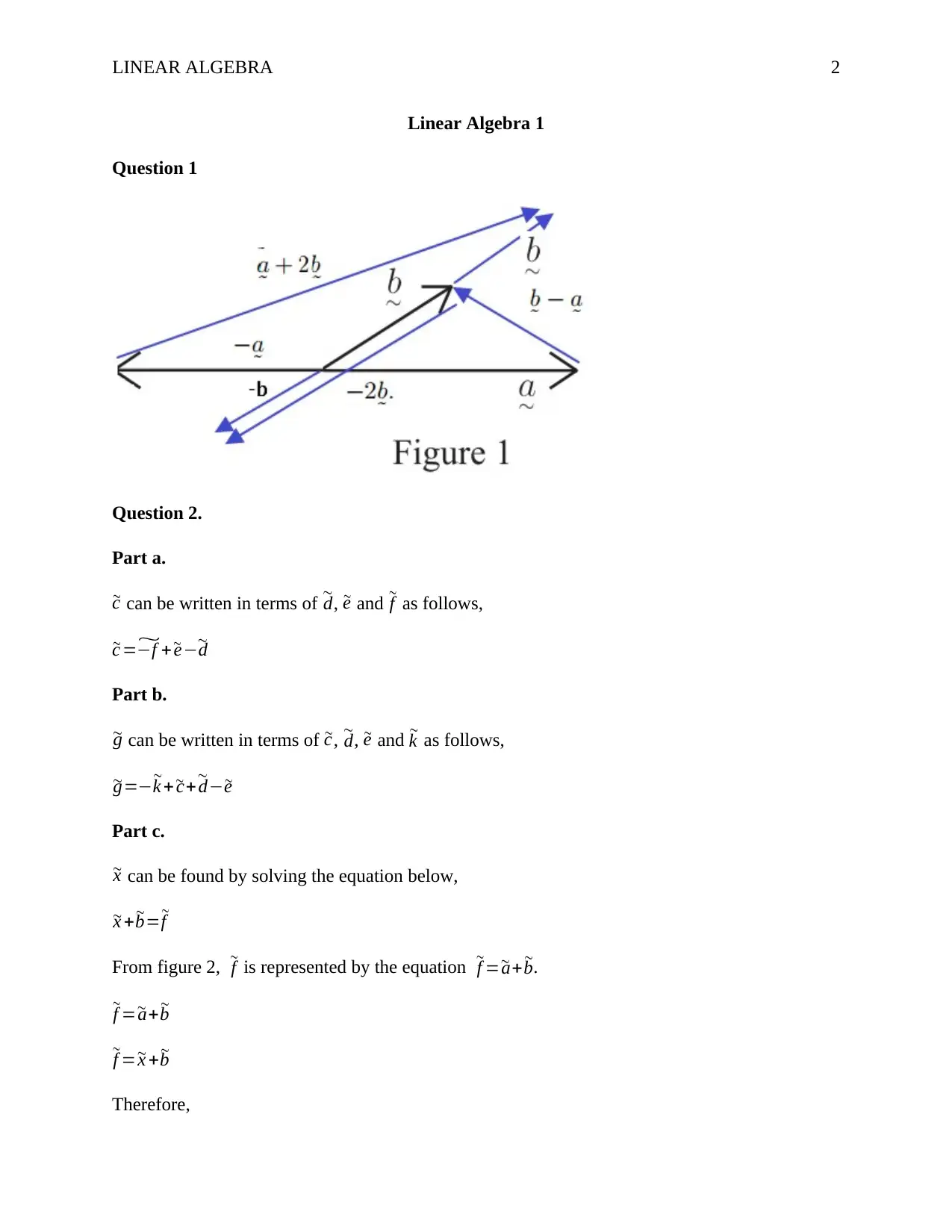
Question 1
Question 2.
Part a.
~c can be written in terms of ~
d, ~e and ~
f as follows,
~c =
~
−f + ~e−~d
Part b.
~g can be written in terms of ~c, ~
d, ~e and ~
k as follows,
~g=− ~
k + ~c+ ~
d− ~e
Part c.
~x can be found by solving the equation below,
~x + ~b= ~
f
From figure 2, ~
f is represented by the equation ~
f = ~a+ ~b.
~
f = ~a+ ~b
~
f = ~x + ~b
Therefore,

LINEAR ALGEBRA 3
~x + ~
b=¿ ~a+ ~
b
~x= ~a+ ~
b− ~
b
Hence,
~x= ~a
Part d
~x can be found by solving the equation below,
~x + ~
h=¿ ~
d− ~e
~
h=¿ ~
d− ~e− ~x
From figure 2, ~
h is represented by the equation ~
h=¿ ~
d− ~e− ~g
Therefore,
~
h=¿ ~
d− ~e− ~x
~
h=¿ ~
d− ~e− ~g
~
d− ~e− ~x= ~
d − ~e− ~g
− ~x=− ~g
Hence.
~x= ~g
Part e.
~x can be found by solving the equation below,
~x= ~e−~a− ~
b− ~
d− ~
k
Making ~
b the subject of the formula, ~
b= ~e− ~a− ~
d − ~
k −~x
From figure 2, ~
b is represented by the equation,
~
b=− ~a+ ~e− ~
d− ~c

LINEAR ALGEBRA 4
Therefore,
− ~a+ ~e− ~
d− ~c= ~e− ~a− ~
d− ~
k− ~x
~c =
~
k− ~x
From figure 2, ~c is represented by the equation,
~c =
~
k− ~
h
hence,
~
k − ~x= ~
k − ~
h
− ~x=− ~
h
~x= ~
h
Question 3.
Part a.
For the function ~a= ~
i+2 ~
j− ~
k
||~a||= √12+22 +12
||~a||= √ 6
Part b.
The unit vector withthe same direction as ~a is given by : ~a
||~a ||
¿
~
i+2 ~
j− ~
k
√ 6
¿ 1
√ 6
~
i+ 2
√ 6
~
j− 1
√ 6
~
k
Part c
The vector will be given by multiplying the unit vector by 5

LINEAR ALGEBRA 5
¿ 5 ( 1
√6
~i+ 2
√6
~j− 1
√6
~k )= 5
√6
~i+ 10
√6
~j− 5
√6
~k
Part d
The opposite direction for a vector ~a= ~
i+2 ~
j− ~
k is given as − ~a
The unit vector withthe same direction as ~a is 1
√6
~i+ 2
√6
~j− 1
√6
~k
The unit vector withopposite direction as ~a is−( 1
√6
~i + 2
√6
~j− 1
√6
~k )
The vector of length 2∈opposite direction=−2 ( 1
√6
~i+ 2
√ 6
~j− 1
√ 6
~k )
¿ −2
√ 6
~i− 4
√ 6
~j+ 2
√ 6
~k
Question 4
Part a
Considering the points P= (1,0,2 ) and Q= (−1,2,5 )
i.⃗ OP= ~
i+0 ~
j+ 2 ~
k= ~
i+2 ~
k⃗
OQ=− ~
i+ 2 ~
j+5 ~
k
ii. To find⃗ PQ, subtract⃗ OPfrom⃗ OQ⃗
PQ =⃗ OQ−⃗ OP⃗
PQ = (−1−1,2−0,5−2 ) = (−2,2,3 )⃗
PQ = (−2,2,3 )
¿−2 ~i+2 ~j+3 ~
k
iii. ¿⃗ PQ= √ (−22 ) + ( 22 )+ ( 32 )= √17=4.1231
Part b
The value of t can be calculated from the two points R= ( 2, t ,3 ) and S= ( 1,1, 11 ) as follows;
First we find ⃗ RS, given by,⃗
RS= ( 1−2,1−t , 11−3 )

LINEAR ALGEBRA 6⃗
RS= (−1 ,1−t , 8 )
d (⃗ RS ) =9
Hence the distance 9 is given by finding the magnitude,
9= √ (−12 ) + ( 1−t )2+ ( 82 )
92 =1+ ( 1−t )2+ 64
81−1−64= ( 1−t ) 2
16= ( 1−t )2
16=1 ( 1−t ) −t ( 1−t )
t2−2 t−15=0
Solving the quadratic equation by the use of quadratic formula,
−b ± √ b2−4 ac
2a
a=1 , b=−2 , c=−15
−2± √ −22 + ( 60 )
2
−2± 8
2
Hence t=3∨t=−5
Part c
the distance from the starting point can be calculated by the use of vectors,
let ~arepresent a vector direction through which the crawl travelled
hence ~a= ( 4 ~i−12 ~j+3 ~k ) in component notation
~a= ( 4 ,−12 , 3 )

LINEAR ALGEBRA 7
Distance is given by the magnitude of ~a
||~a||= √42±122+ 32= √169=13
Therefore, the distance from the starting point is 13 cm.
Part d
Whent=1 , r ( t ) =3 ~
i +12 ~
j−3 ( 1 ) ~
k=3 ~
i+ ~
j−3 ~
k
When t=3 , r ( t ) =3 ~
i+32 ~
j −3 ( 3 ) ~
k=3 ~
i+9 ~
j−9 ~
k
Displacement= ( 3 ~
i+9 ~
j−9 ~
k ) − ( 3 ~
i+ ~
j−3 ~
k )=8 ~
j−6 ~
k
scalar displacement = √ 82 +(−6)2=10
Question 5
Part a
P= (1,2,1 ) , Q= ( 3 , 3 , 6 )∧R (−3 , 0 ,−9 )⃗
PQ =Q−P= (3−1 , 3−2 , 6−1 )= ( 2 ,1 , 5 )⃗
QR =R−Q= (−3−3 , 0−3 ,−9−6 )= (−6 ,−3 ,−15 )⃗
PR=R−P= (−3−1 , 0−2 ,−9−1 )= (−4 ,−2,−10 )
Part b
The three points are collinear since:⃗
QR =−3⃗ PQ ∧⃗ PR=−2⃗ PQ
Question 6
Part a
For the following points in space
P= (1,2,1 ) , Q= ( 3 , 3 , 4 ) , R ( 4 ,3 , 5 ) and S ( 2 ,2 , 2 )
The direction ⃗ PQ ,⃗ QR , ,⃗ SR∧⃗ PS are given as follows;

LINEAR ALGEBRA 8⃗
PQ =Q−P= (3−1 , 3−2 , 4−1 )= (2 , 1 ,3 )⃗
QR =R−Q= ( 4−3 , 3−3 , 5−4 )= ( 1 , 0 ,1 )⃗
SR=R−S= ( 4−2 , 3−2 , 5−2 ) = ( 2 ,1 , 3 )⃗
PS=S−P= ( 2−1 , 2−2 , 2−1 ) = ( 1 , 0 , 1 )
Part b
The quadrilateral is a parallelogram since two opposite sides are equal as we can see from the
vectors calculated above.
Part c ⃗
PQ =Q−P= (3−1 , 3−2 , 4−1 )= (2 , 1 ,3 )⃗
QS=S−Q= ( 2−3 , 2−3 , 2−4 )= (−1 ,−1 ,−2 ) ,
1
2⃗ QS= 1
2 ( −1 ,−1 ,−2 ) = ( −1
2 ,− 1
2 ,−1 )⃗
PM =⃗ PQ+ 1
2⃗ QS⃗
PM = ( 2 ,1 , 3 ) +(−1
2 ,− 1
2 ,−1 )= (2− 1
2 ,1− 1
2 ,3−1)=( 3
2 , 1
2 , 2 )
Part d.
To the direction of ⃗ PN can be determined using the routes,⃗
PN =⃗ PS+ 1
2⃗ SQ and ⃗ PN =⃗ PQ+ 1
2⃗ QS⃗
PS=S−P= ( 2−1 , 2−2 , 2−1 ) = ( 1 , 0 , 1 )
1
2⃗ SQ=1
2 ( Q−S ) =1
2 ( 3−2 , 3−2 , 4−2 ) =1
2 ( 1 , 1 ,2 ) =( 1
2 , 1
2 , 1 )⃗
PN =⃗ PS+ 1
2⃗ SQ

LINEAR ALGEBRA 9⃗
PN = ( 1 ,0 , 1 ) +( 1
2 , 1
2 , 1 )=(1+ 1
2 ,0+ 1
2 ,1+1 )= (3
2 , 1
2 ,2 )
The mid points M=N this is because ⃗
PS+ 1
2⃗ SQ=⃗ PQ + 1
2⃗ QS= (3
2 , 1
2 ,2 )
Since ⃗ PM =⃗ PN then M=N.
Question 7
x , y ∈ R3 having ||~x + ~y||=||~x||+||~y || cab be proved as a false statement as follows
Let ~x be (−1 , 0 ,−2 ) and ~y be (2 , 1 ,3 )
Therefore ~x + ~y= (−1, 0 ,−2 )+( 2 , 1, 3 ) = ( 2−1 ,1+ 0 ,3−2 ) = ( 1 , 1, 1 )
||~x + ~y||= √ 12+12+12= √ 3=1.7321
||~x||= √−12+02 +−22= √5=2.2361
||~y||= √ 22 +12+32= √ 15=3.8730
Hence ||~x||+||~y||=2.2361+3.8730=6.1091
From the above analysis, ||~x + ~y||≠ ||~x||+||~y||
This justifies that the statement is false
LINEAR ALGEBRA 2
QUESTION 1
Part a
~u . ~v =¿ ||u||.||v||. cos θ
¿ 2 ×3 ×cos ( π
3 )=6 × 0.5=3
Part b

LINEAR ALGEBRA 10
Since ~u . ~v =¿ ||u||.||v ||. cos θ
||v||= ~u . ~v
||u||. cos θ
Since ~u=3∧ ~u . ~v =¿ 6, having given θ= π
4
||v||= 6
3 cos ( π
4 )=2.8293
Part c
When ~u . ~v =0 , this indicates that
cos θ=0 ,
Therefore, θ=cos−1 0=90 °
θ=90 °= π
2
Part d
When ~u . ~v =6 ,||v||=3∧||u||=2 , θwould be calculated as;
Making cos θ the subject of the formular
~u . ~v
||u||.||v|| =¿ cos θ
Substituting 6
2× 3 =¿ cos θ
cos θ=1
θ=cos−1 0=0 °=π
Question 2
Part a
~a= ~
i−2 ~
j∧
~
b=3 ~
i+6 ~
j
~a . ~
b= ( ~i−2 ~j ) .(3 ~i+6 ~j)
¿ ( 1 ×3 ) + (−2 ×6 )=−9
Part b
~a=3 ~
i−5 ~
j+4 ~
k

LINEAR ALGEBRA 11
~
b= ~i+ ~j+2 ~
k
~a . ~
b=(3 ~
i−5 ~
j+4 ~
k).( ~
i+ ~
j +2 ~
k )
~a . ~
b= ( 3 ×1 ) + (−5 ×1 ) + ( 4 ×2 ) =6
Part c
~a= ~
i− ~
j+2 ~
k
~
b= ~
i− ~
j− ~
k
~a . ~
b= ( ~
i− ~
j+ 2 ~
k ) .( ~
i− ~
j−
~
k )
~a . ~
b= ( 1× 1 ) + ( −1×−1 ) + ( 2×−1 ) =0
Question 3
Part a
~a= ~
i−2 ~
j
||~a||= √12+−22 = √5=2.2361
||~a||=2.2361
~
b=3 ~i+6 ~j
||~
b||= √ 32+62= √ 25=5
~a . ~
b=¿ ||~a||. ||~
b||. cos θ
From question above part a, ~a . ~
b=−9
Substituting each component to the formula,
−9=¿ 2.2239 ×5 cos θ
cos θ=¿ −9
11.1195 =−0.8094 ¿
θ=cos−1−0.8094=144.04 °
Part b
~a= ( 3 ,−5 , 4 )
||~a||= √32+−52 + 42 = √50=7.0711
||~a||=7.0711
~
b= ( 1 ,1 , 2 )

LINEAR ALGEBRA 12
||~b||= √12 +12 +22= √6=2.4495
||~
b||=2.4495
~a . ~
b=¿ ||~a||. ||~
b||. cos θ
From question above part b, ~a . ~
b=6
Substituting each component to the formula,
6=¿ 7.0711× 2.4495 cos θ
cos θ=¿ 6
17.3207 =0.3464 ¿
θ=cos−1 0.3464=69.73 °
Part c
~a= ( 1 ,−1 , 2 )
||~a||= √12+12 +22= √6=2.4495
||~a||=2.4495
~
b= ( 1 ,−1 ,−1 )
||~
b||= √12 +−12 +12= √3=1.7321
||~b||=1.7321
~a . ~
b=¿ ||~a||. ||~b||. cos θ
From question above part c, ~a . ~
b=0
Substituting each component to the formula,
0=¿ 2.4495 ×1.7321 cos θ
cos θ=¿ 0
4.2428 =0 ¿
θ=cos−1 0=90 °
Question 4
Part a
Finding ~u . ( 3 ~v +5 ~w ) if ~u . ~v =4∧ ~u . ~w=−2

LINEAR ALGEBRA 13
~u . ( 3 ~v +5 ~w )=3 ( ~u . ~v ) +5 ( ~u .~w )
¿ 3 ( 4 ) +5 (−2 )=2
Part b
~u . ( a ~v +2 ~w ) =4, ~u . ~v =2, and ~u . ~w=−3
~u . ( a ~v +2 ~w )=a ( ~u . ~v ) +2 ( ~u . ~w )
¿ a ( 2 ) +2 (−3 )=2a−6=4
a= 4+ 6
2 =5
Part c
Finding ~u . ( 4 ~v +6 ~w ) if ~u . ~v =3∧ ~uis perpendicular to ~w
For a non-zero vectors ~uand ~w to be perpendicular to each
~u . ~w=0
~u . ( 4 ~v +6 ~w )=4 ( ~u . ~v )+ 6 ( ~u . ~w )
¿ 4 ( 3 ) +6 ( 0 ) =12
Part c
~u . ( 5 ~u−3 ~v ) if ~u . ~v =7and ~u as a length of 2
~u . ( 5 ~u−3 ~v )=5 ( ~u . ~u )−3 ( ~u . ~v )
¿ 5 ( 2× 2 )−3 ( 7 )=−1
Part e
finding out ~a . ( ~
b ∥+ ~
b ⊥ ) given that ~
b ∥=2 ~a
length of ¿|~a|∨¿ 5
implying that ¿|~a|∨¿ √ 52
simplify a2=52

LINEAR ALGEBRA 14
~a=5
~
b ∥=2 ~a
~
b ⊥meaning its perpendicular to ~a
Therefore ~
b . ~a=0
~b ⊥=0
~a
Substituting 0
~a and 10 to the function
~a . ( ~b ∥+ ~b ⊥ )= ~a× ( 0
~a +2 ~a )=2 a2 =50
Question 5
Part a

LINEAR ALGEBRA 15
Part b
~
b∨¿ ¿
~
b × ~a
~a × ~a ~a
From part a above,
~
b=4 ~i+3 ~j
~a=4 ~
i−2 ~
j
~
b . ~a= ( 4 ,3 ) × ( 4 ,−2 )= ( 4 × 4 )+ (3 ×−2 )=10
~
b . ~a=10
~a . ~a= ( 4 ,−2 ) × ( 4 ,−2 )= ( 4 × 4 ) + (−2 ×−2 )=20
~a . ~a=20
Hence
~
b ∥= 10
20 ( 4 ,−2 )= ( 2 ,−1 )
~
b ∥= ( 2 ,−1 )
The function is drawn on the graph in part a.
Part c
Calculating the vector
~
b ⊥=
~
b− ~
b ∥
~
b=4 ~
i+3 ~
j
~
i=4∧ ~
j=3
~
b= ( 4 , 3 ) and ~
b ∥= ( 2 ,−1 )
Therefore
~
b− ~
b ∥= ( 4 ,3 )− ( 2 ,−1 ) = ( 4−2 , 3+1 )= ( 2 , 4 )

LINEAR ALGEBRA 16
~
b ⊥= ( 2 , 4 ) and ~a= ( 4 ,−2 )
Verifying that, ~
b ⊥ and ~a are perpendicular
~
b ⊥ . ~a=0 implying that
( 2 , 4 ) × ( 4 ,−2 )= ( 4 ×2 ) + (−2× 4 )=0
Hence this proves that ~
b ⊥ and ~a are perpendicular.
Question 6
Part a
~a=4 ~
i− ~
j+ ~
k , ~
b=−2 ~
i− ~
j−2 ~
k
we take ~
b¿∨¿= −1
2
~i+ 1
4
~j− 1
2
~
k∧ ~
b¿=−3
2
~i− 5
4
~j− 1
2
~
k ¿
Part b
~
b ∥=λ ~a
In the above case λ is the Scalar constant that gives the multiplication ratios.
λ< 0 this implies the scalar is a negative value which is less than zero implying that its
multiplication will switch the direction of the vector point in an opposite direction making it gain
a stretch or shrink on the magnitude of the vector. This depends on the size of the constant.
Question 7
Part a
For the quadrilateral ABCD with vertices A= (−1 ,2 , 3 ), B= ( 0 , 4 , 1 ) , C= ( 3 , 4 ,1 ) and
d= ( 2, 2 , 3 )
About the origin O= ( 0 ,0 , 0 )⃗
OA = A−O= ( −1−0,2−0 , 3−0 ) = ( −1 , 2 ,3 )⃗
OA = (−1 , 2, 3 )

LINEAR ALGEBRA 17⃗
OB =B−O= ( 0−0,4−0 , 1−0 )= ( 0 , 4 ,1 )⃗
OB = ( 0 , 4 , 1 )⃗
OC=C−O= (3−0,4−0 , 1−0 )= ( 3 , 4 ,1 )⃗
OC= ( 3 , 4 ,1 )⃗
OD=D−O= ( 2−0,2−0 ,3−0 ) = ( 2 ,2 , 3 )
Part b
Finding⃗ AC and⃗ BD⃗
AC=⃗ OC−⃗ OA = ( 3 , 4 ,1 ) − (−1 ,2 , 3 )= ( 3+1 , 4−2 , 1−3 )= ( 4 ,2 ,−2 )⃗
AC= ( 4 , 2,−2 )⃗
BD=⃗ OD−⃗ OB= ( 2, 2 , 3 )− ( 0 , 4 ,1 )= ( 2−0,2−4 ,3−1 )= ( 2 ,−2 ,2 )⃗
BD= ( 2 ,−2 ,2 )
Part c⃗
AC and⃗ BD are the diagonals of the quadrilateral
If⃗ AC and⃗ BD are perpendicular to each other, the⃗
AC .⃗ BD=0⃗
AC= ( 4 , 2,−2 )⃗
BD= ( 2 ,−2 ,2 )
Hence⃗ AC .⃗ BD=0
This proves that the diagonals are
perpendicular
QUESTION 8

LINEAR ALGEBRA 18
The parallelogram above ABCD have the vectors ⃗
AB= ~a, ⃗ BC = ~
b ,⃗ AD= ~
b, ⃗ DC= ~a
´BD, and ´AC are the diagonals
´AC=⃗ AB+⃗ BC= ~a+ ~
b
´BD=−⃗ AB+,⃗ AD= ~
b−¿ ~a
´AC . ´BD= ( ~a+ ~
b ) . ( ~
b− ~a )
¿ ¿ ~
b∨¿2−|~a|2=0 ¿
This implies that ´AC is perpendicular ¿ ´BD , hence it is a rhombus