Operations Research and Logistics: Leach Distributors Case Study

This assignment presents a comprehensive case study on Leach Distributors, focusing on the application of linear programming to optimize container usage for packaging. The student formulates the optimization problem by defining decision variables, an objective function (maximizing profit), and cons...Read More

OPERATIONS RESEARCH
AND LOGISTICS CASE
STUDY TASK REFERRED
DEFERRED
CASE STUDY A – LEACH DISTRIBUTORS

TABLE OF CONTENTS
• INTRODUCTION
• Formulation of Optimizing problem
• Decision variables
• Objective function
• Constraints
• Non-negativity restrictions
• Statement of assumption
• Steps involved in Excel Solver
• Solution obtained through Microsoft Solver
• Implications of resource restrictions for Leach Distributors
• Selection and analysis of linear programming application
• Benefits of Linear programming in Manufacturing
• Conclusion
• References

INTRODUCTION
• Linear programming is a mathematical model through which the best outcome whether it
to maximize profit or minimize costs is achieved by representing the linear relationships
between objective function and constraints.
• In this presentation, by applying the linear programming model, the number of each class
of containers to be used by Leach Distributors for packaging during standard shipment
will be determined.

Formulation of optimization problem by
defining objective function and constraint
equations

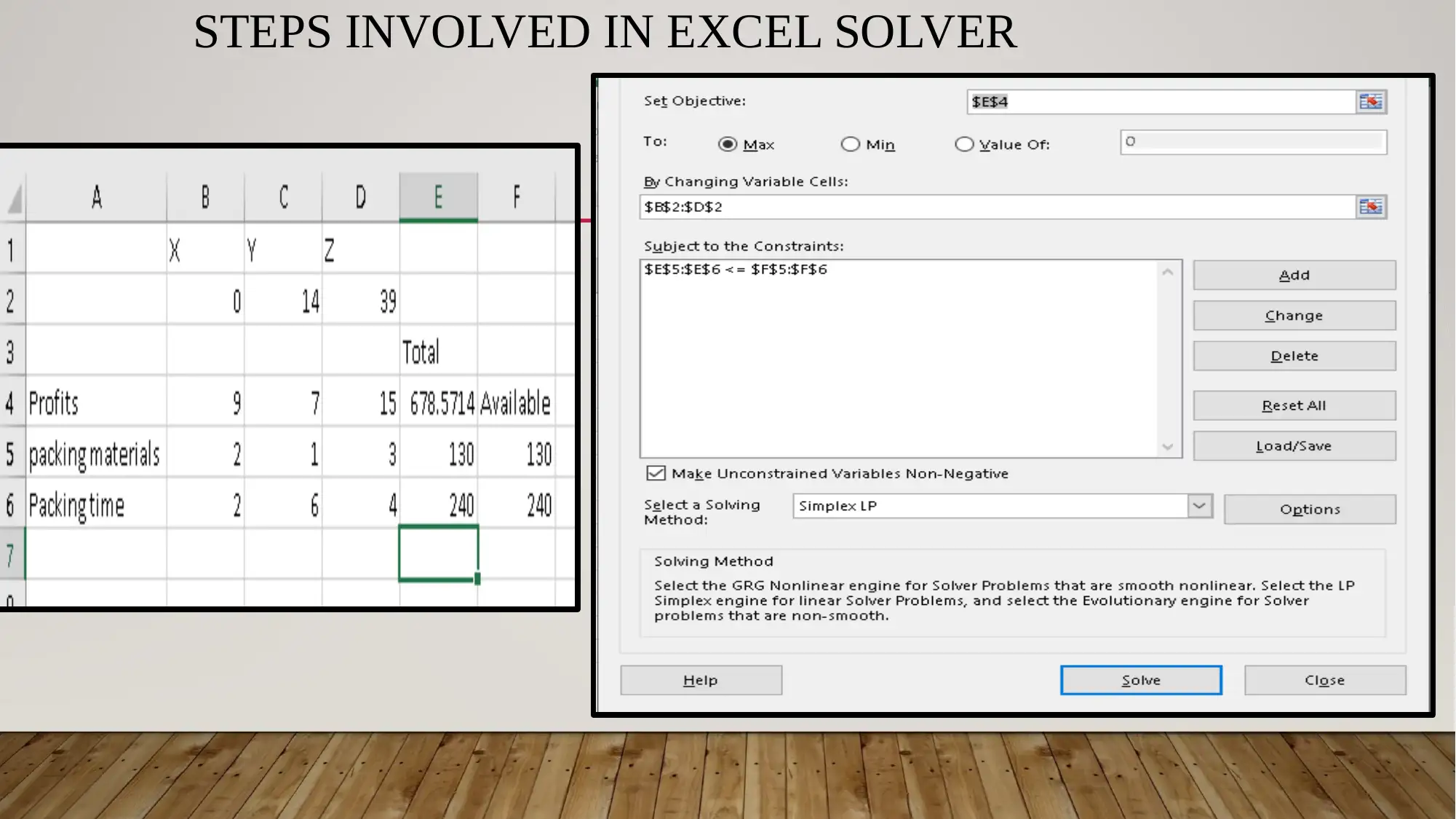
PROBLEM FORMULATION
Decision variables
• X = Number of class A container to be used
• Y = Number of class K container to be used
• Z = Number of class T container to be used
Objective function
• Maximize,
• Z = $9X + $7Y + $15Z
Constraints
• Subject to,
• Packing material
• 2X + 1Y + 3Z </= 130
• Packing time
• 2X + 6Y + 4Z </= 240

NON – NEGATIVITY RESTRICTIONS
X>/= 0, Y>/= 0, Z>/= 0

STATEMENT OF ASSUMPTIONS
• Conditions of certainty
• Linearity
• Additivity
• Divisibility
• Conditions for non – negativity variables
• Finiteness
• Condition for optimal solution

STEPS INVOLVED IN EXCEL SOLVER

USING MICROSOFT SOLVER FOR SOLVING
FORMULATED LINEAR PROGRAMMING PROBLEM

IMPLICATIONS OF LEACH DISTRIBUTOR’S
RESOURCE RESTRICTION
• Resource restrictions for the Leach Distributor are as follows:
• Total available packing material = 130 pounds
• Total available packing time = 240 hours
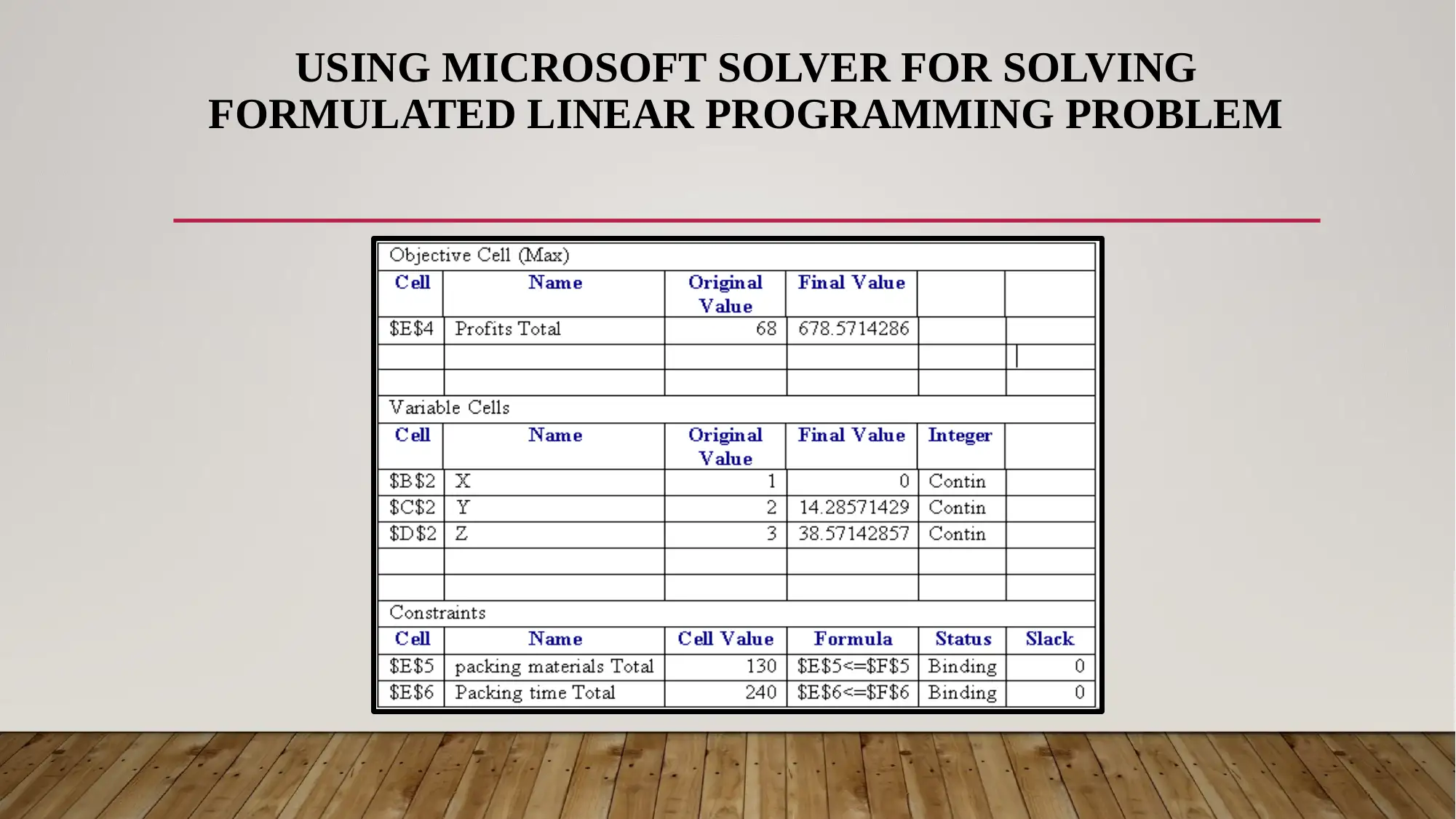
• Optimal number of each class of container to pack each week for Leach Distributor are as follows:
• A class “A” container = 0
• A class “K” container = 14
• A class “T” container = 39

CONT.
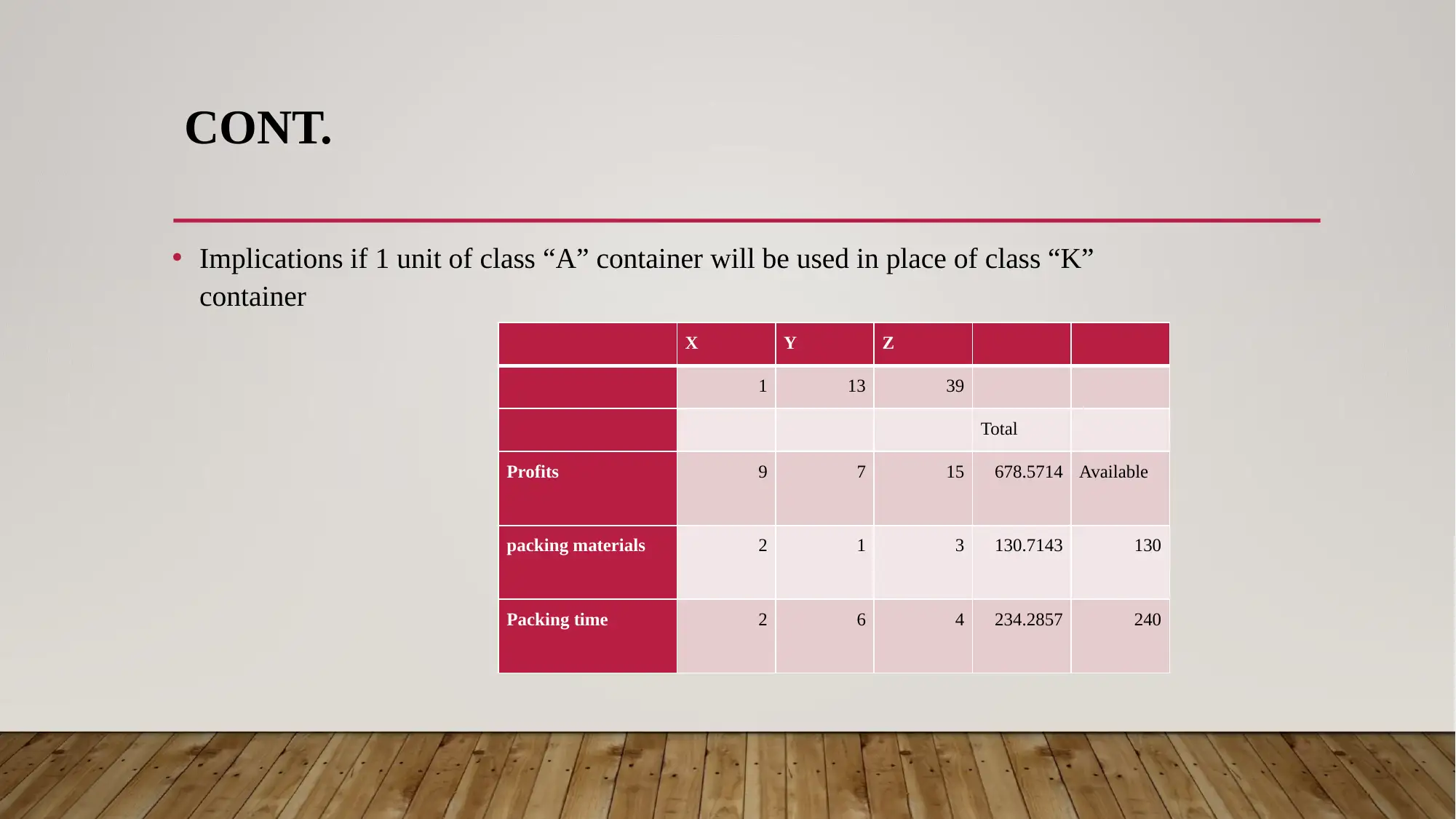
X Y Z
1 13 39
Total
Profits 9 7 15 678.5714 Available
packing materials 2 1 3 130.7143 130
Packing time 2 6 4 234.2857 240
• Implications if 1 unit of class “A” container will be used in place of class “K”
container

CONT.
• Implications if 1 unit of class “A” container will be used in place of class “T” container
X Y Z
1 14 38
Total
Profits 9 7 15 677 Available
packing
materials
2 1 3 130 130
Packing time 2 6 4 238 240

SELECTION AND ANALYSIS OF ONE
LINEAR PROGRAMMING APPLICATION
• Linear programming can be best applied in a manufacturing setting where raw materials
transformed into finished products that could lead to maximization of company’s revenue
and profits accordingly.
• For maximizing profit and minimizing the consumption of resources, it is necessary that
each step of the manufacturing process must be contributing efficiently towards the
achievement of objective function. For instance, in an assembly line, the raw materials
are expected to pass through several machines for a fixed amount of time.

BENEFITS OF APPLYING LP MODEL IN
MANUFACTURING
The following benefits are offered by linear programming tool to attain the objective of profit maximization:
• How much raw material should be used can be determined in alignment of the profit maximizing objective
function through linear programming tool.
• By including constraints in terms of availability of machine hours and labor hours, it can be ensured that the
entire manufacturing process would be efficient and would result in minimum consumption of resources and
incurrence of costs.
• Any hindrance or bottlenecks created by the available machines can be easily addressed.
• At last, the amount of products to be produced can be determined in alignment of the availability of time &
resources to ensure that the optimal quantity of products can be determined which lead to maximization of
profits.

CONCLUSION
• The above presentation involves the application of linear programming model for
determining the number of each class of container to be used by Leach Distributors for
packaging while standard shipment.
• Accordingly, it has been determined that the optimal number of each class of container
that is A, K and T are found out as 0, 14 and 39 respectively.
• At this level of using container, the profit would be maximum along with satisfying the
resource restrictions of imposed on Leach Distributors.

REFERENCES
Sangaiah, A. K., and et.al., 2020. Robust optimization and mixed-integer linear programming model for LNG
supply chain planning problem. Soft computing, 24(11), pp.7885-7905.
Samsatli, S. and Samsatli, N. J., 2018. A general mixed integer linear programming model for the design and
operation of integrated urban energy systems. Journal of Cleaner Production, 191, pp.458-479.
Kang, J., Ng, T. S. and Su, B., 2020. Optimizing electricity mix for CO2 emissions reduction: A robust input-output
linear programming model. European Journal of Operational Research, 287(1), pp.280-292.
Feng, Z. K., and et.al., 2019. A mixed integer linear programming model for unit commitment of thermal plants
with peak shaving operation aspect in regional power grid lack of flexible hydropower energy. Energy, 175,
pp.618-629.
Vimal, K. E. K., Rajak, S. and Kandasamy, J., 2018. Analysis of network design for a circular production system
using multi-objective mixed integer linear programming model. Journal of Manufacturing Technology
Management.

THANK YOU