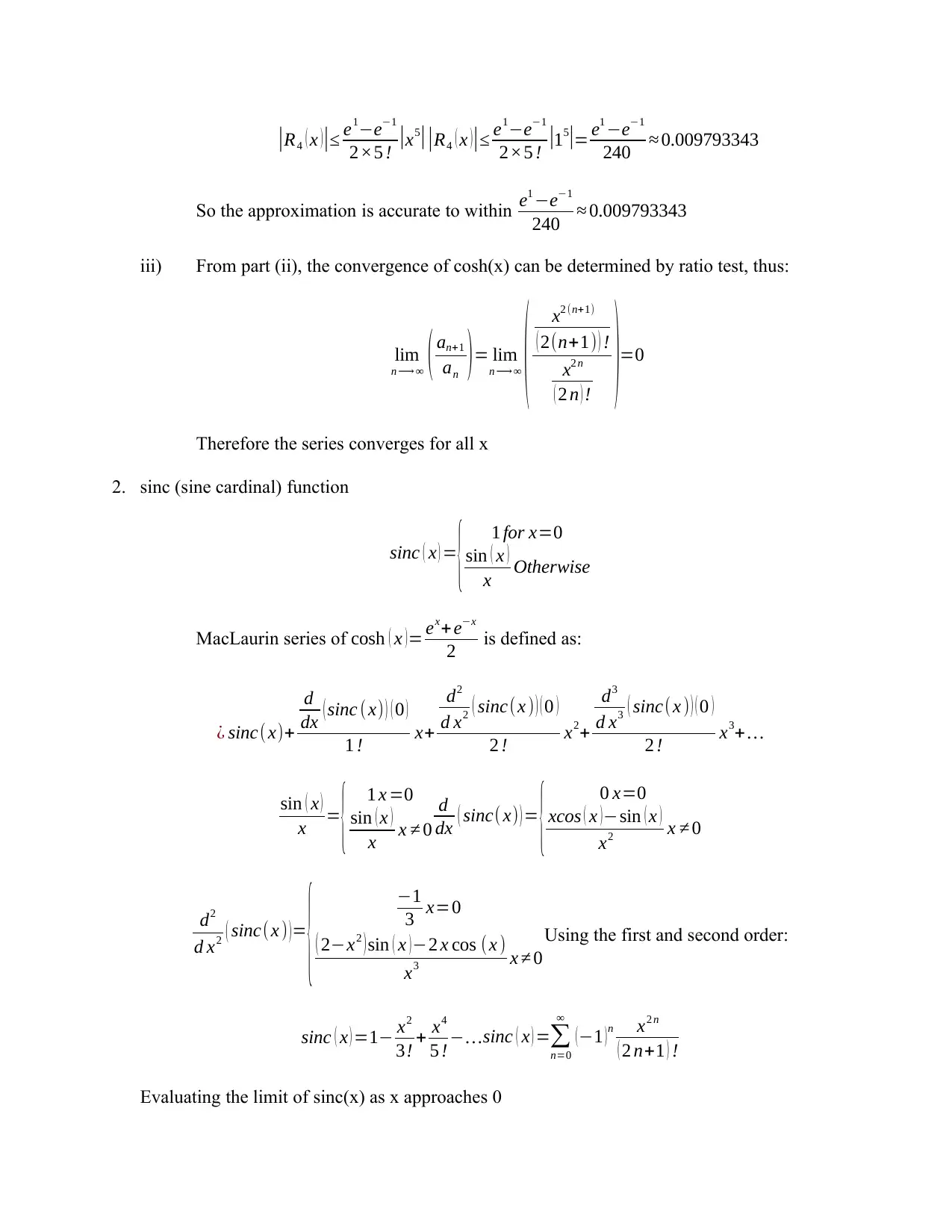
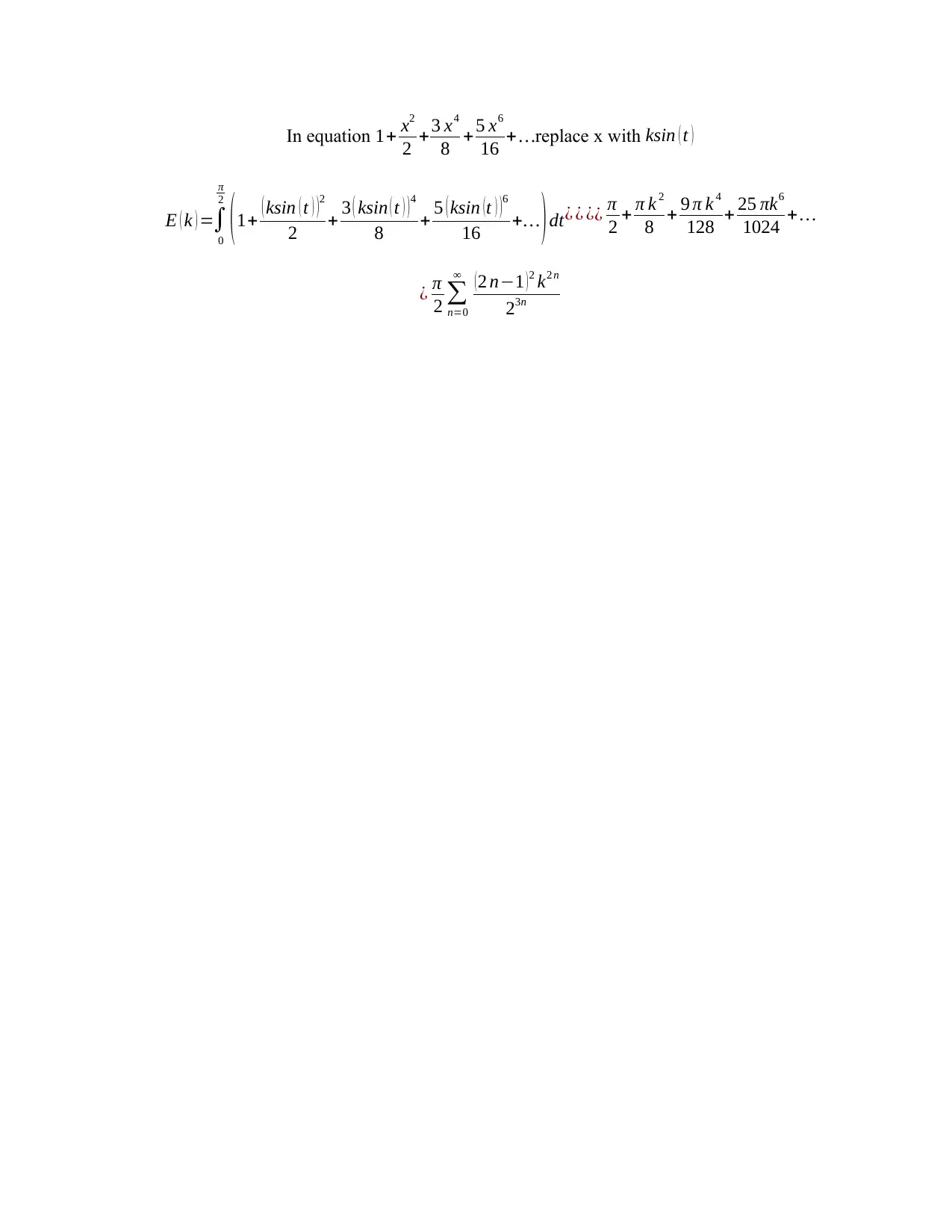
MacLaurin series for cosh(x), Taylor's inequality, sinc function, binomial series for sqrt(1-x^2)

This text covers MacLaurin series for cosh(x), Taylor's inequality, sinc function, and binomial series for sqrt(1-x^2) with their respective applications. It also mentions Desklib as an online library for solved assignments, essays, dissertations, and more.

Order Id 814870
1. .
i) MacLaurin series for cosh(x)
cosh ( x )= ex+ e−x
2
MacLaurin series of cosh ( x ) = ex+ e−x
2 is defined as:
¿ e0+ e−0
2 +
d
dx ( ex +e−x
2 ) ( 0 )
1 ! x+
d2
d x2 ( e x+ e−x
2 ) ( 0 )
2 ! x2+
d3
d x3 ( ex+e−x
2 ) ( 0 )
2! x3 +…
e0+ e−0
2 =1
d
dx ( ex +e− x
2 ) ( 0 ) =0 d2
d x2 ( ex+ e−x
2 ) ( 0 )=1 d3
d x3 ( ex+ e−x
2 ) ( 0 )=0 d4
d x4 ( ex +e− x
2 ) ( 0 )=1
d5
d x5 ( ex+ e−x
2 ) ( 0 )=0 d6
d x6 ( ex+ e−x
2 ) ( 0 ) =1 d7
d x7 ( ex+ e−x
2 ) ( 0 )=0 d8
d x8 ( ex+ e−x
2 ) ( 0 ) =1
¿ 1+ 0
1 ! x+ 1
2 ! x2 + 0
3 ! x3+ 1
4 ! x4 + 0
5 ! x5 + 1
6 ! x6 + 0
7 ! x7 + 1
8 ! x8 +…¿ 1+ 1
2! x2+ 1
4 ! x4 + 1
6 ! x6 + 1
8 ! x8 +…
Thus the series representation is;
∑
n=0
∞ x2n
( 2 n ) !
ii) Taylor’s inequality says
|R4 ( x )|=|f ( x ) −T 4 ( x )|≤ M
( 4 +1 ) ! x4 +1T 4 ( x )=1+ 1
2 ! x2 + 1
4 ! x4
f 5 ( x )= d5
d x5 ( ex−e−x
2 )= 1
2 ( ex−e−x ) f 5 ( 1 ) =M = e1−e−1
2
The Taylor’s inequality becomes:

|R4 ( x )|≤ e1−e−1
2×5 ! |x5
| |R4 ( x )|≤ e1−e−1
2×5 ! |15
|= e1 −e−1
240 ≈ 0.009793343
So the approximation is accurate to within e1 −e−1
240 ≈ 0.009793343
iii) From part (ii), the convergence of cosh(x) can be determined by ratio test, thus:
lim
n ⟶ ∞ ( an+1
an ) = lim
n ⟶ ∞
( x2 (n+1)
( 2(n+1) ) !
x2 n
( 2 n ) ! )=0
Therefore the series converges for all x
2. sinc (sine cardinal) function
sinc ( x ) = { 1 for x=0
sin ( x )
x Otherwise
MacLaurin series of cosh ( x ) = ex+ e−x
2 is defined as:
¿ sinc(x)+
d
dx ( sinc ( x) ) ( 0 )
1 ! x+
d2
d x2 ( sinc(x ) ) ( 0 )
2 ! x2+
d3
d x3 ( sinc( x ) ) ( 0 )
2 ! x3+ …
sin ( x )
x = { 1 x =0
sin ( x )
x x ≠ 0
d
dx ( sinc( x) ) =
{ 0 x=0
xcos ( x )−sin ( x )
x2 x ≠ 0
d2
d x2 ( sinc( x ) )=
{ −1
3 x=0
( 2−x2 ) sin ( x )−2 x cos (x )
x3 x ≠ 0
Using the first and second order:
sinc ( x ) =1− x2
3! + x4
5 ! −… sinc ( x ) =∑
n=0
∞
( −1 ) n x2 n
( 2 n+1 ) !
Evaluating the limit of sinc(x) as x approaches 0

lim
n ⟶ 0
sinc ( x ) = lim
n ⟶ 0 ( sin ( x )
x )= lim
n ⟶ 0 (1− x2
3! + x4
5 ! … )=1
3. MacLaurin series for sin(s^2)
¿ sin ( t2 ) +
d
dx ( sin ( t2 ) ) ( 0 )
1 ! x+
d2
d x2 ( sin ( t2 ) ) ( 0 )
2 ! x2+
d3
d x3 ( sin ( t2 ) ) ( 0 )
2 ! x3+ …
sin ( 0 ) =0
d
dx (sin ( t2 ) ) ( 0 ) =0 d2
d x2 ( sin ( t2 ) ) ( 0 ) =2 d3
d x3 ( sin ( t2 ) ) ( 0 )=0 d4
d x4 (sin ( t2 ) ) ( 0 )=0 d5
d x5 ( sin ( t2 ) ) ( 0 )=0
d6
d x6 ( sin ( t2 ) ) ( 0 )=−120 d7
d x7 ( sin ( t2 ) ) ( 0 )=0 d8
d x8 ( sin ( t2 ) ) ( 0 )=0 d9
d x9 ( sin ( t2 ) ) ( 0 ) =0
d10
d x10 (sin ( t2 ) ) ( 0 )=30240
¿ 1+ 0
1 ! x+ 2
2 ! x2 + 0
3 ! x3+ 0
4 ! x4 + 0
5 ! x5 +−120
6 ! x6+ 0
7 ! x7 + 0
8 ! x8 + +0
9! x9 + 30240
10 ! x10 …
¿ t 2− 1
6 t6 + 1
120 t10 …The Fresnel-sine integral:
∫
0
x
sin ( t2 ) dt=∫
0
x
( t2− 1
6 t6 + 1
120 t10 … … ) ¿ x3
3 − x7
42 + x11
1320 −…
4. Binomial series for 1
√1−x2
1
√1−x2 = ( 1−x2 )
−1
2
1
√1−x2 =1+ −1
2 (−x2 ) +
−1
2 (−3
2 ) (−x2 )2
2 ! +
−1
2 (−3
2 )(−5
2 ) (−x2 )3
3 ! +… ¿ 1+ x2
2 + 3 x4
8 +5 x6
16 + …
Now to evaluate E (k) let x2=k2 sin2 ( t ) ⟹ x=ksin(t)E ( k ) =∫
0
π
2
1
√1−k2 sin2 ( t ) dt

In equation 1+ x2
2 + 3 x4
8 +5 x6
16 + …replace x with ksin ( t )
E ( k ) =∫
0
π
2
( 1+ ( ksin ( t ) )
2
2 + 3 ( ksin ( t ) ) 4
8 + 5 ( ksin ( t ) )
6
16 +… ) dt¿ ¿ ¿¿ π
2 + π k 2
8 + 9 π k 4
128 + 25 πk6
1024 +…
¿ π
2 ∑
n=0
∞ ( 2 n−1 ) 2 k2 n
23n