Business Numeracy Assignment - Module Two

This numeracy assignment comprises three tasks. Task 1 focuses on calculating the Net Present Value (NPV) of a project using a discount rate. Task 2 requires students to complete an online numerical skills assessment and provide a screenshot of their results. Finally, Task 3 involves maintaining a reflective log throughout the module, documenting learning experiences, strengths, and areas for improvement.

Student Name
Student ID
Tutor
Portfolio Contents
1
Numeracy 2 (MAII3007) Portfolio
Autumn 2017

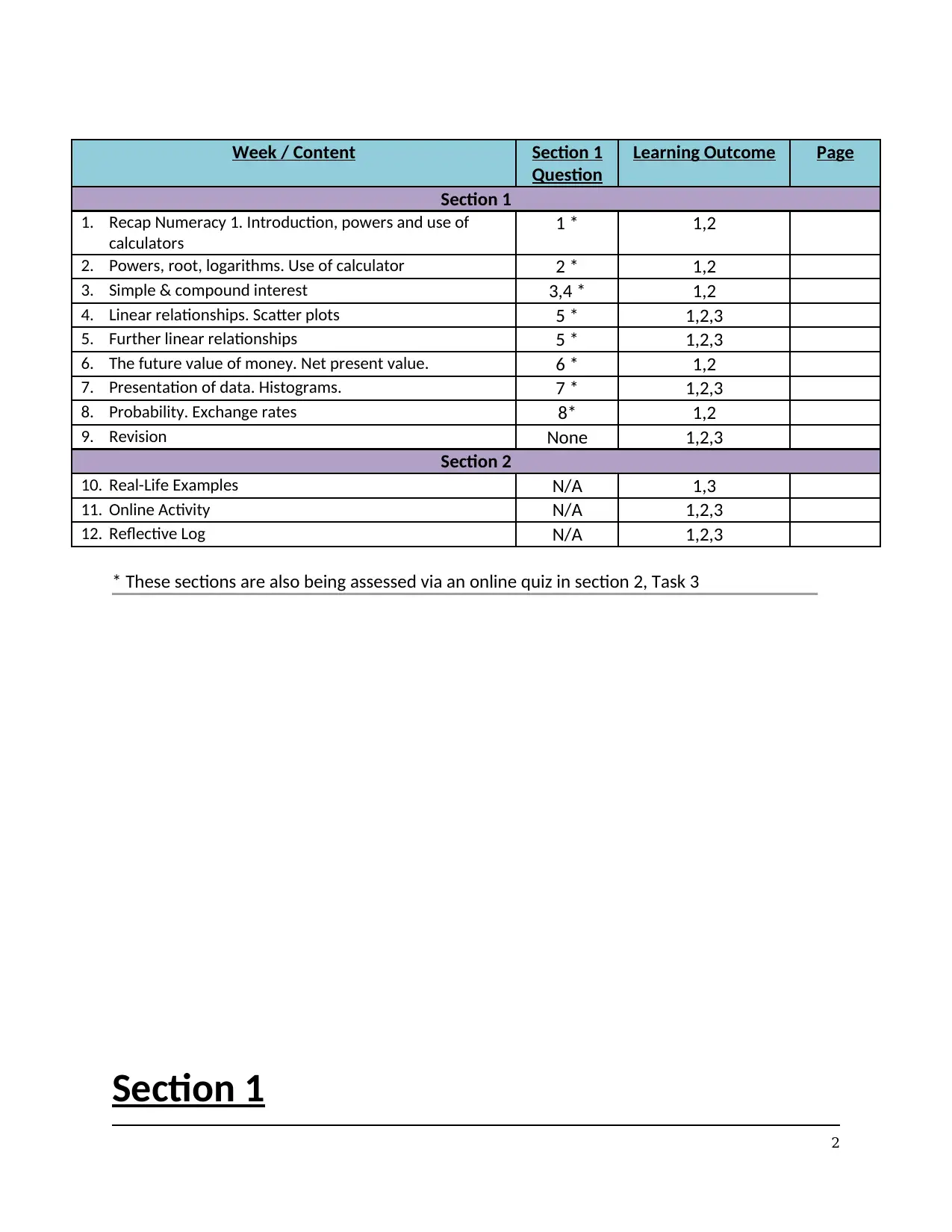
Week / Content Section 1
Question
Learning Outcome Page
Section 1
1. Recap Numeracy 1. Introduction, powers and use of
calculators
1 * 1,2
2. Powers, root, logarithms. Use of calculator 2 * 1,2
3. Simple & compound interest 3,4 * 1,2
4. Linear relationships. Scatter plots 5 * 1,2,3
5. Further linear relationships 5 * 1,2,3
6. The future value of money. Net present value. 6 * 1,2
7. Presentation of data. Histograms. 7 * 1,2,3
8. Probability. Exchange rates 8* 1,2
9. Revision None 1,2,3
Section 2
10. Real-Life Examples N/A 1,3
11. Online Activity N/A 1,2,3
12. Reflective Log N/A 1,2,3
* These sections are also being assessed via an online quiz in section 2, Task 3
Section 1
2

You are required to complete this section immediately after completing the class sessions
related to each question.
Answer all questions and show your workings and/or explain your results.
Marks will be awarded for good presentation.
You may use your calculator as required.
You must show your workings.
QUESTION 1 [6 marks]
State the Power rule for each part and simplify:
a) Simplify 73÷ 72(1 mark)
b) Simplify 1505 ×1502.5 (3 marks)
c) Evaluate ( 33 ) 4 (2 marks)
[TYPE YOUR ANSWER HERE]
a. Simplification of 73÷ 72
73÷ 72= 7*7*7 ÷ 7*7 = 343 / 49 = 7
b. Simplification of 1505 X 1502.5
1505 X 1502.5 = 75937500000 X 275567.5961 = 20,925,914,326,042,200
c. Evaluation of (33)4
(33)4 = (3*3*3)4 = 274 = 531441
3

QUESTION 2 [6 marks]
a) Find the root of this fractional power 641/ 3 (2 marks)
b) Evaluate 6
√ 1,000,000 (2 marks)
c) Express 100,640 in a scientific notation (2 marks)
TYPE YOUR ANSWER HERE
a. Exponent 1/3 means third root. Therefore, 641/3 means 4*4*4 = 64
Therefore, the answer is 4.
b. 6
√ 1,000,000 = 3∗2
√1,000,000 = 2
√100 = 10
c. 100,640 in scientific notation = 1.0064 x 105
SKILLS AUDIT: WEEKS 1 – 2
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
4

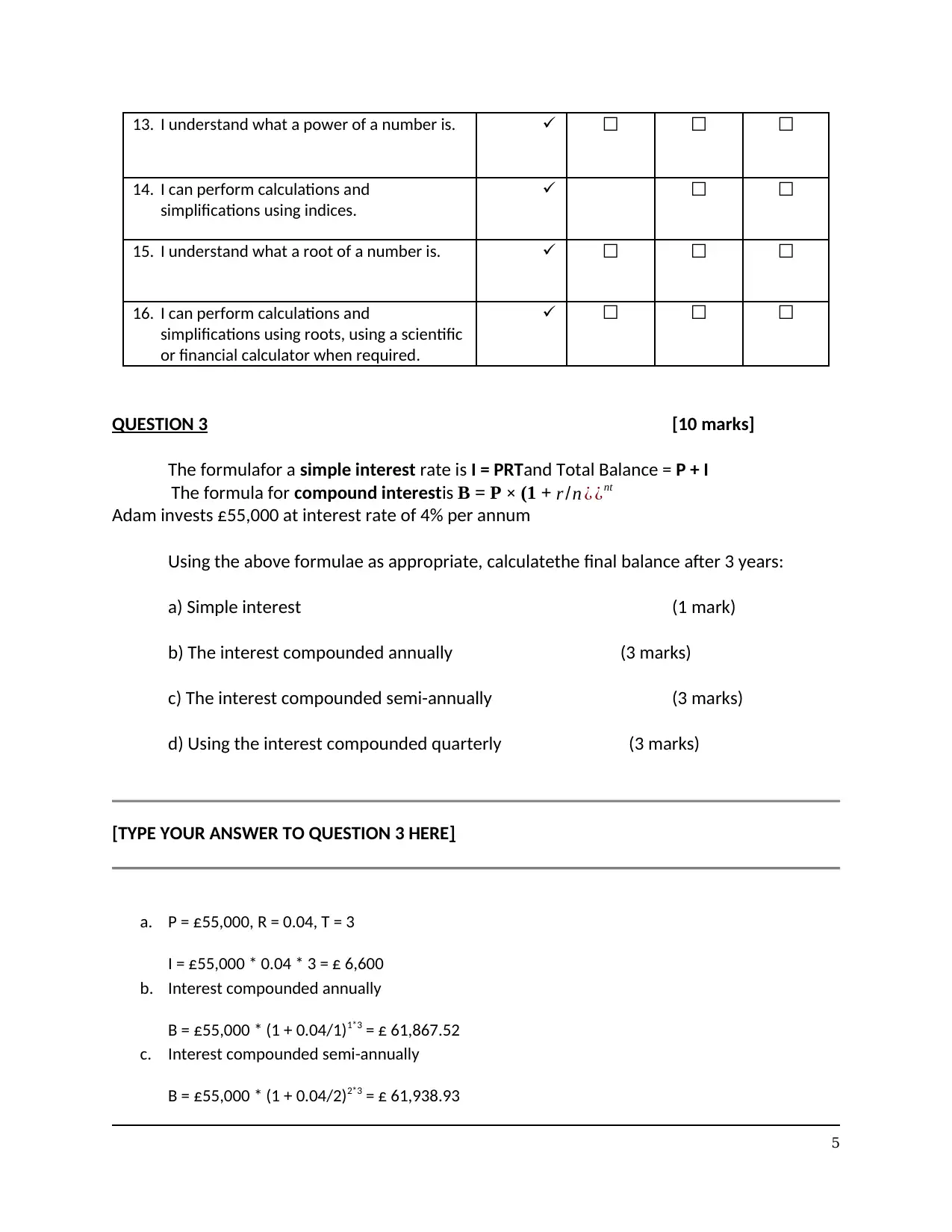
13. I understand what a power of a number is.  ☐ ☐ ☐
14. I can perform calculations and
simplifications using indices.
 ☐ ☐
15. I understand what a root of a number is.  ☐ ☐ ☐
16. I can perform calculations and
simplifications using roots, using a scientific
or financial calculator when required.
 ☐ ☐ ☐
QUESTION 3 [10 marks]
The formulafor a simple interest rate is I = PRTand Total Balance = P + I
The formula for compound interestis B = P × (1 + r /n ¿ ¿nt
Adam invests £55,000 at interest rate of 4% per annum
Using the above formulae as appropriate, calculatethe final balance after 3 years:
a) Simple interest (1 mark)
b) The interest compounded annually (3 marks)
c) The interest compounded semi-annually (3 marks)
d) Using the interest compounded quarterly (3 marks)
[TYPE YOUR ANSWER TO QUESTION 3 HERE]
a. P = £55,000, R = 0.04, T = 3
I = £55,000 * 0.04 * 3 = £ 6,600
b. Interest compounded annually
B = £55,000 * (1 + 0.04/1)1*3 = £ 61,867.52
c. Interest compounded semi-annually
B = £55,000 * (1 + 0.04/2)2*3 = £ 61,938.93
5

d. Interest compounded quarterly
B = £55,000 * (1 + 0.04/4)4*3 = £ 61,975.38
6

QUESTION 4 [10 marks]
a) Junior invests £160,000 at a5 1
8 % interest rate annually.
Compounding the interest annually, how long will it take his investment
to double?
(4 marks)
b) Using Rule 72, how long will it take Junior to double his investment?
(2 marks)
b) Caesar deposits £3,500 in a bank savings account and after 10 years his balance
is £4,266.50
Calculate the compound interest he received and give your answer in three
significant figures.
(4 marks)
[TYPE YOUR ANSWER TO QUESTION 4 HERE]
a. Interest compounded annually
P = £160,000, R = 5*1/8%, T = ?, B = £320,000
B = £160,000 * (1 + 0.05125/1)1*T = £ 320,000
Therefore, T = 13.8685 years
b. Under rule 72, 72 is divided by the increase per year that is 5*1/8% that is 5.125% to get the
year by which the amount will get double.
Therefore, the time will be 72 / 5.125 = 13.8685 years.
c. Rate of compound interest
P = £3500, R = ?, T = 10, B = £4266.50
B = £ 3500 * (1 + R)10 = £ 4,266.50
Therefore, T = 2.00 years
7

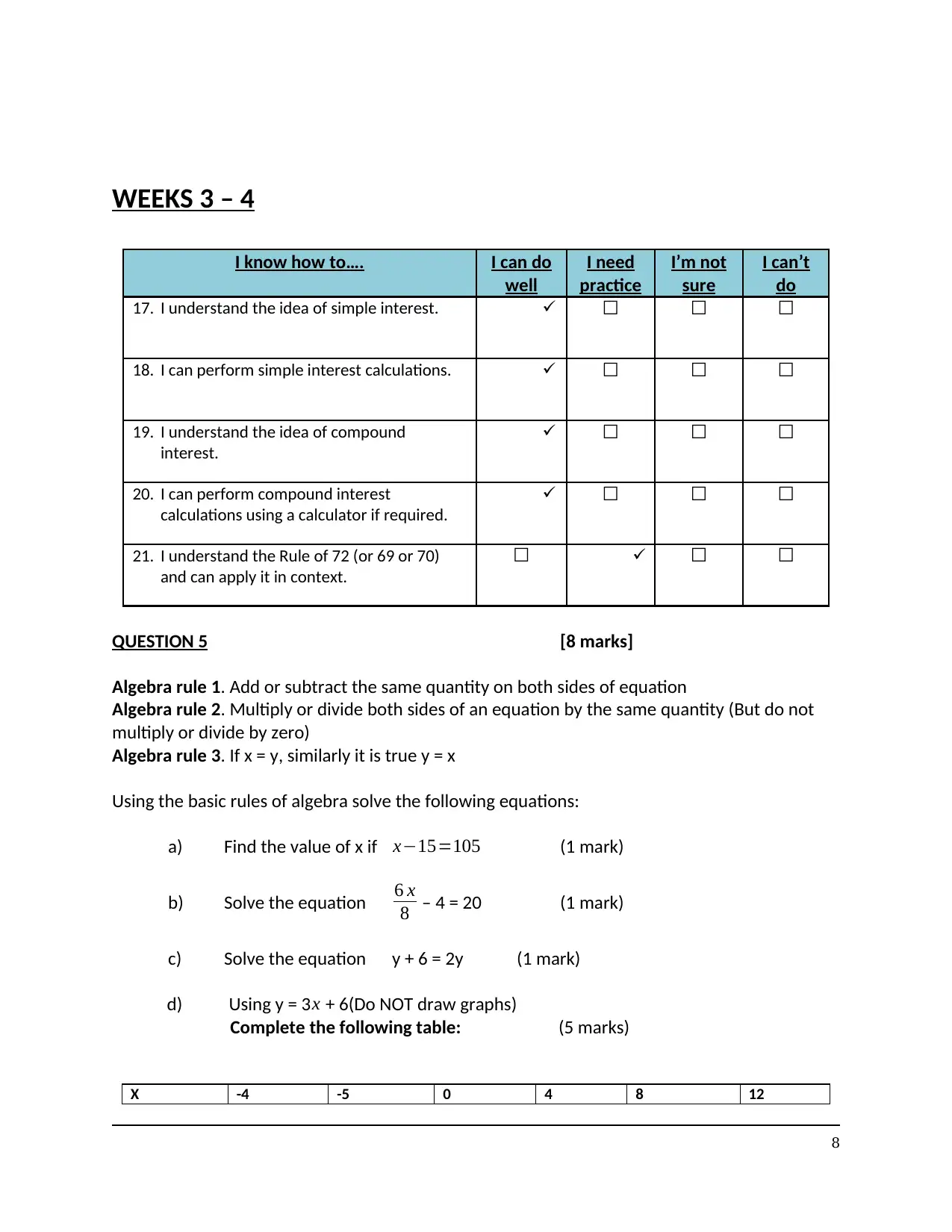
WEEKS 3 – 4
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
17. I understand the idea of simple interest.  ☐ ☐ ☐
18. I can perform simple interest calculations.  ☐ ☐ ☐
19. I understand the idea of compound
interest.
 ☐ ☐ ☐
20. I can perform compound interest
calculations using a calculator if required.
 ☐ ☐ ☐
21. I understand the Rule of 72 (or 69 or 70)
and can apply it in context.
☐  ☐ ☐
QUESTION 5 [8 marks]
Algebra rule 1. Add or subtract the same quantity on both sides of equation
Algebra rule 2. Multiply or divide both sides of an equation by the same quantity (But do not
multiply or divide by zero)
Algebra rule 3. If x = y, similarly it is true y = x
Using the basic rules of algebra solve the following equations:
a) Find the value of x if x−15=105 (1 mark)
b) Solve the equation 6 x
8 – 4 = 20 (1 mark)
c) Solve the equation y + 6 = 2y (1 mark)
d) Using y = 3 x + 6(Do NOT draw graphs)
Complete the following table: (5 marks)
X -4 -5 0 4 8 12
8

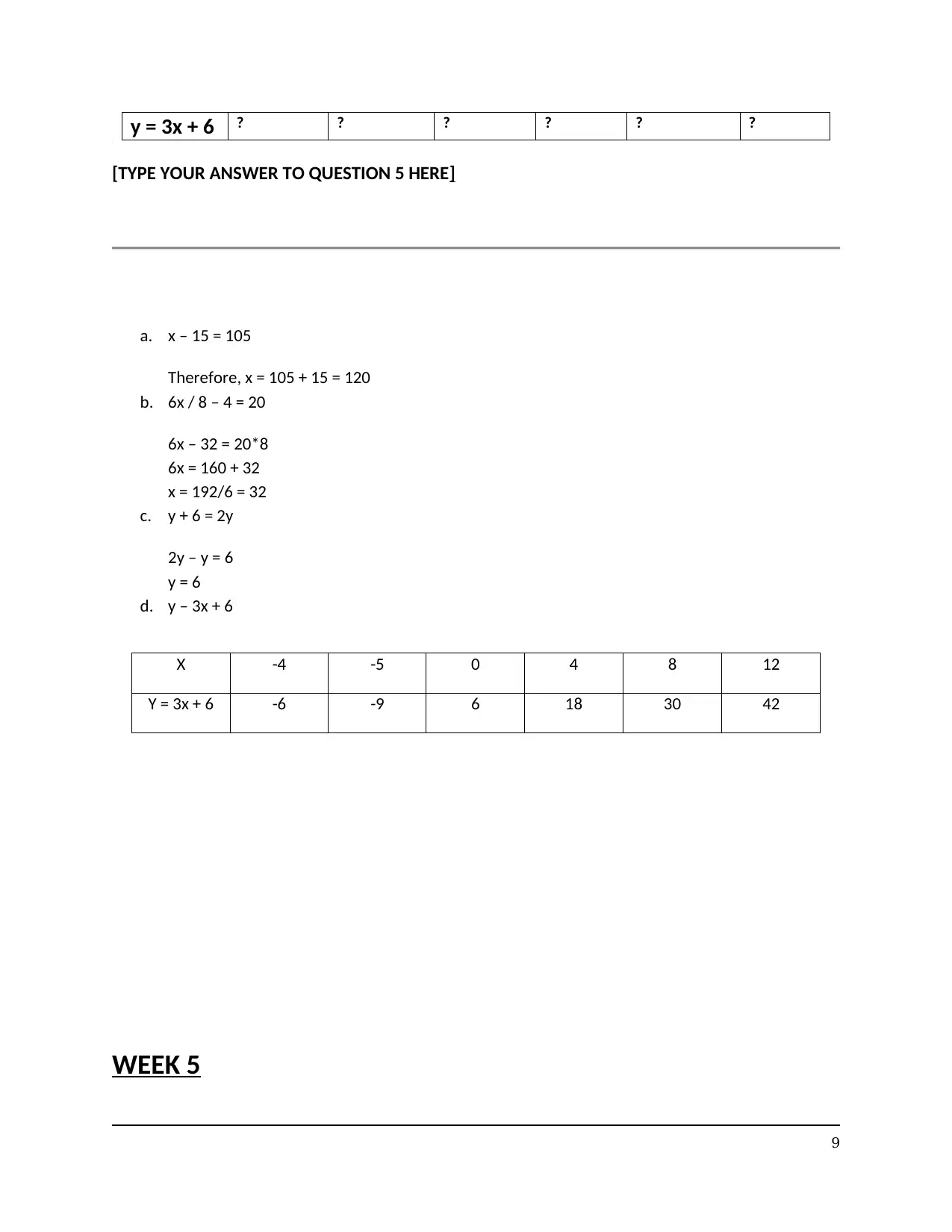
y = 3x + 6 ? ? ? ? ? ?
[TYPE YOUR ANSWER TO QUESTION 5 HERE]
a. x – 15 = 105
Therefore, x = 105 + 15 = 120
b. 6x / 8 – 4 = 20
6x – 32 = 20*8
6x = 160 + 32
x = 192/6 = 32
c. y + 6 = 2y
2y – y = 6
y = 6
d. y – 3x + 6
X -4 -5 0 4 8 12
Y = 3x + 6 -6 -9 6 18 30 42
WEEK 5
9

I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
22. I understand linear relationship between
two variables.
 ☐ ☐ ☐
23. I can work out linear equation problems
given in this session.
 ☐ ☐ ☐
24. I can plot a scatter graph from a set of data.  ☐ ☐ ☐
25. I understand the y-intercept and slope
(gradient) of a line graph and interpret their
meaning in real situations ( y=mx+c).
 ☐ ☐ ☐
26. I can use a scatter plot to derive a linear
relationship between two variables (
y=mx+c).
☐  ☐ ☐
27. I am able to explain the use of scatter
graphs in business context.
☐  ☐ ☐
10

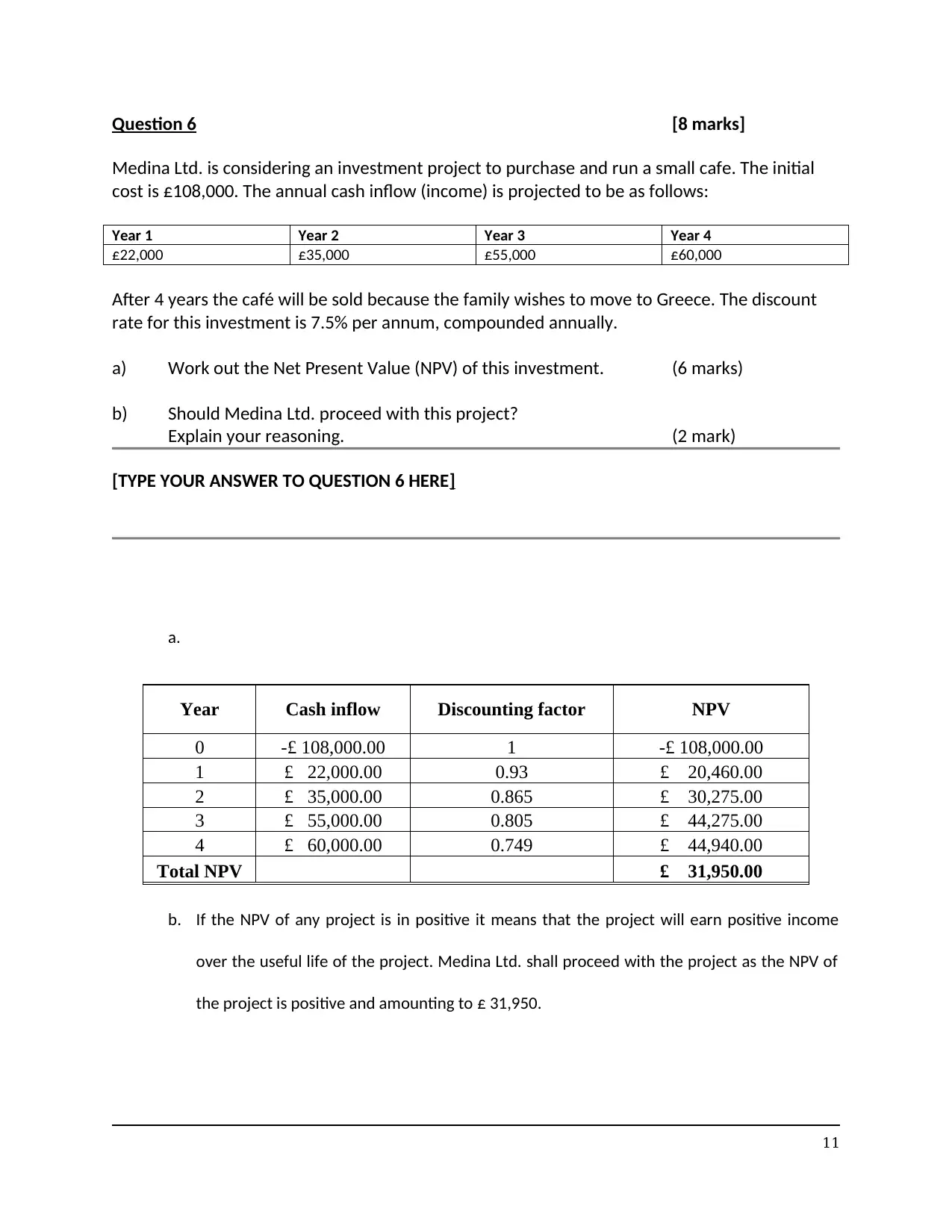
Question 6 [8 marks]
Medina Ltd. is considering an investment project to purchase and run a small cafe. The initial
cost is £108,000. The annual cash inflow (income) is projected to be as follows:
Year 1 Year 2 Year 3 Year 4
£22,000 £35,000 £55,000 £60,000
After 4 years the café will be sold because the family wishes to move to Greece. The discount
rate for this investment is 7.5% per annum, compounded annually.
a) Work out the Net Present Value (NPV) of this investment. (6 marks)
b) Should Medina Ltd. proceed with this project?
Explain your reasoning. (2 mark)
[TYPE YOUR ANSWER TO QUESTION 6 HERE]
a.
Year Cash inflow Discounting factor NPV
0 -£ 108,000.00 1 -£ 108,000.00
1 £ 22,000.00 0.93 £ 20,460.00
2 £ 35,000.00 0.865 £ 30,275.00
3 £ 55,000.00 0.805 £ 44,275.00
4 £ 60,000.00 0.749 £ 44,940.00
Total NPV £ 31,950.00
b. If the NPV of any project is in positive it means that the project will earn positive income
over the useful life of the project. Medina Ltd. shall proceed with the project as the NPV of
the project is positive and amounting to £ 31,950.
11

WEEK 6
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
28. I understand the concept of the future
value of money.
 ☐ ☐ ☐
29. I understand the net present value (NPV) of
a project.
 ☐ ☐ ☐
30. I can understandthe steps involved in
NPV(net present value) calculations, using a
calculator as required.
 ☐ ☐ ☐
Question 7 (The material for this question is covered in week 8) [9 marks]
Below are results of a Numeracy 2 assignment:
68 90 87 40 68
48 45 93 92 66
63 70 90 92 96
58 98 64 78 96
64 64 86 66 75
a) Produce a tally of this data set suitable for the production of a histogram.
(3 marks)
b) Plot a histogram from the above data. (5 marks)
c) Comment on the distribution of these marks. (1 mark)
[TYPE YOUR ANSWER TO QUESTION 7 HERE]
a.
12

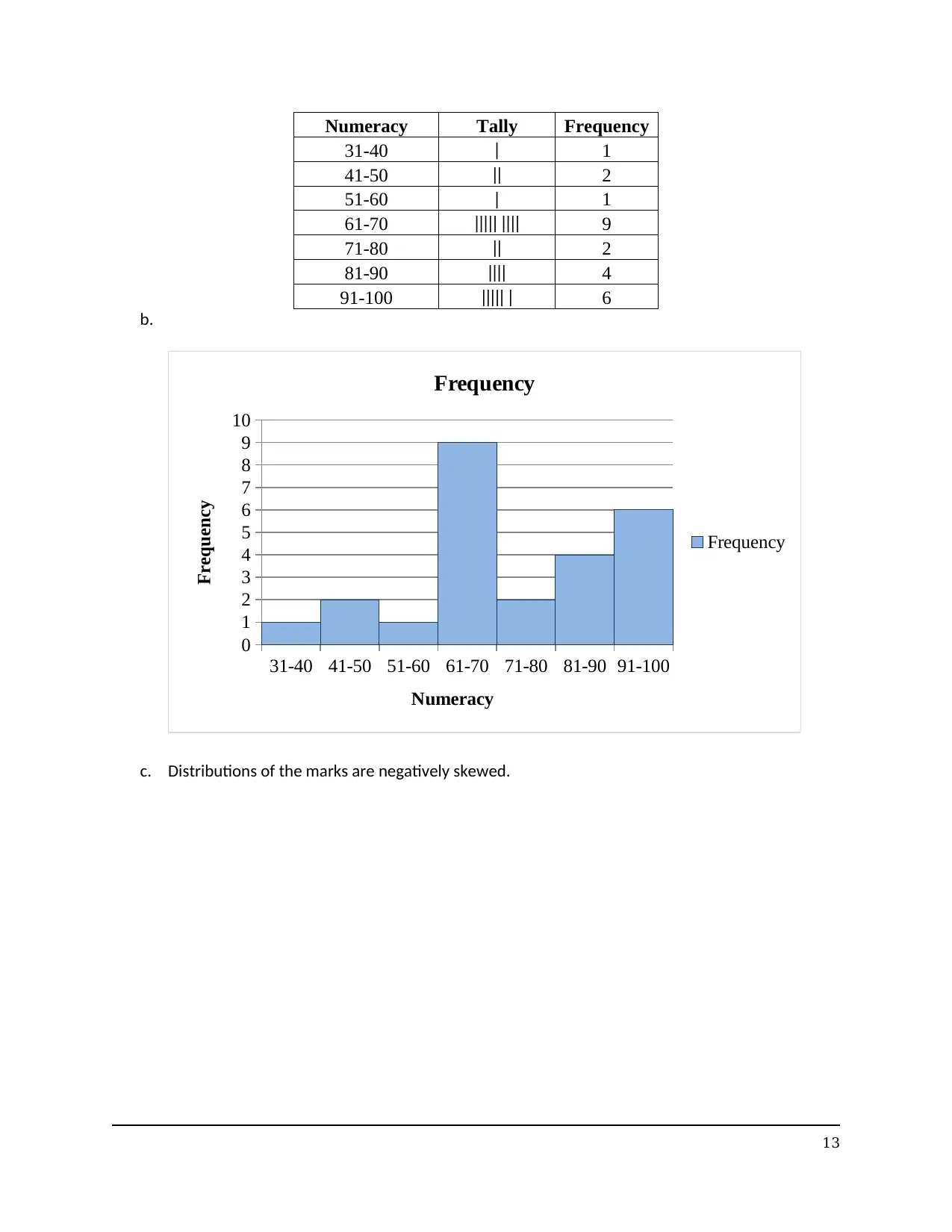
Numeracy Tally Frequency
31-40 ǀ 1
41-50 ǀǀ 2
51-60 ǀ 1
61-70 ǀǀǀǀǀ ǀǀǀǀ 9
71-80 ǀǀ 2
81-90 ǀǀǀǀ 4
91-100 ǀǀǀǀǀ ǀ 6
b.
31-40 41-50 51-60 61-70 71-80 81-90 91-100
0
1
2
3
4
5
6
7
8
9
10
Frequency
Frequency
Numeracy
Frequency
c. Distributions of the marks are negatively skewed.
13

WEEK 7
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
31. I understand the idea of frequency
distribution.
☐  ☐ ☐
32. I can read and interpret a histogram. ☐  ☐ ☐
33. I can construct a histogram from a set of
data.
☐  ☐ ☐
Question 8 [8 marks]
Probability is a branch of mathematics that deals with the study of chance. If one card is drawn
from a normal pack of 52 playing cards:
a) What is the probability of getting an ace? (2 marks)
b) What is the probability of getting a spade? (2 marks)
c) What is the probability of ace of spades? (2 marks)
d) What is the probability of ace or spade? (2 marks)
[TYPE YOUR ANSWER TO QUESTION 8 HERE]
a. Probability of getting an ace
Total 4 aces are there that can be drawn from 52 cards.
14

Therefore, n (S) = 52
Now, if A is the required event, then A = (club ace, spade ace, heart ace, diamond ace)
n (A) = 4
P (A) = n (A) / n (S) = 4/52
P (A) = 1/13
b. Probability of getting spade
n (S) = 52
Now, if A is the required event, then A = (Spade)
n (A) = 13
P (A) = n (A) / n (S) = 13/52
P (A) = 1/4
c. Probability of getting ace of spade
n (S) = 52
Now, if A is the required event, then A = (Ace of Spade)
n (A) = 1
P (A) = n (A) / n (S) = 1/52
P (A) = 1/52
d. Probability of getting ace or spade
n (S) = 52
Now, if A is the required event, then A = (Ace, Spade)
n (A) = (4,13)
WEEK 8
I know how to…. I can do
well
I need
practice
I’m not
sure
I can’t
do
34. I understand simple probabilities.  ☐ ☐ ☐
35. I can perform probability calculations, using
a calculator if required.
☐  ☐ ☐
36. I understand and can perform exchange ☐  ☐ ☐
15

rate calculations.
16

MODULE CODE MODULE TITLE SUMMER 2017 COURSEWORK BRIEF
Section 2
Task 1 - Two Real Life Examples (100 words each – 10 marks)
Give two real-life examples or problems in businesses that involve the topics studied in
this module (e.g. powers and roots, simple and compound interests, linear
relationships, graphs, probabilities and Net Present values (NPV)).
[TYPE YOUR ANSWERS TO TASK 1 HERE]
(i)
Simple interest example – simple interest is the quick and easy method of
calculating interest charged on the loan. The simple interest is determined through
multiplying daily interest rate by principle and by number of days, counted between
the payments. During the year 2017, Tesco PLC borrowed from USD bond amounting
to £ 709 million at the rate of 5.5% simple interest. Therefore, the amount of interest
for the year will be = £ 709 * 0.055 * 1 = £ 39 million where, P = 709 million, R =
0.055 and t = 1 year.
(ii)
Net present value – the NPV is the difference among the PV of the cash inflows and
the PV of the cash outflows. It is used to evaluate the investment’s profitability over
the useful life of the project. For the financial year 2017 Tesco PLC invested £ 64
million in the property. At the discount rate of 7.5%, the NPV will be as follows –
Year Cash inflow
Discounting
factor @
7.5%
NPV
0 -£ 64.00 1 -£ 64.00
1 £ 24.00 0.93 £ 22.32
2 £ 20.00 0.865 £ 17.30
3 £ 18.00 0.805 £ 14.49
4 £ 16.00 0.749 £ 11.98
Total NPV £ 2.09
Task 2 - Online Activity (10 marks)
You must complete and pass the online activity; take a screenshot and paste it onto
the portfolio. Ensure the following are visible before the screenshot:
GSM LONDON Page 1 of 18